Chemistry
An aqueous solution of HCl with pH 1.0 is diluted by adding equal volume of water (ignoring dissociation of water). The pH of HCl solution would
$($ Given $\log 2=0.30)$
A person's wound was exposed to some bacteria and then bacterial growth started to happen at the same place. The wound was later treated with some antibacterial medicine and the rate of bacterial decay(r) was found to be proportional with the square of the existing number of bacteria at any instance. Which of the following set of graphs correctly represents the 'before' and 'after' situation of the application of the medicine?
[Given: $N=$ No. of bacteria, $t=$ time, bacterial growth follows $1^{\text {st }}$ order kinetics.]
Total enthalpy change for freezing of 1 mol of water at $10^{\circ} \mathrm{C}$ to ice at $-10^{\circ} \mathrm{C}$ is ________
(Given: $\Delta_{\text {fus }} \mathrm{H}=x \mathrm{~kJ} / \mathrm{mol}$
$$\begin{aligned} & \mathrm{C}_{\mathrm{p}}\left[\mathrm{H}_2 \mathrm{O}(\mathrm{l})\right]=y \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \\ & \mathrm{C}_{\mathrm{p}}\left[\mathrm{H}_2 \mathrm{O}(\mathrm{~s})\right]=z \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \end{aligned}$$
Which of the following amine (s) show (s) positive carbylamine test?

B. $\left(\mathrm{CH}_3\right)_2 \mathrm{NH}$
C. $\mathrm{CH}_3 \mathrm{NH}_2$
D. $\left(\mathrm{CH}_3\right)_3 \mathrm{~N}$

Choose the correct answer from the options given below:
Which of the following compounds is least likely to give effervescence of $\mathrm{CO}_2$ in presence of aq. $\mathrm{NaHCO}_3 ?$
Match the List I with List II.
| List - I Molecule/Ion |
List - II Bond pair : lone pair (on the central atom) |
||
|---|---|---|---|
| (A) | $\mathrm{ICl}_2^{-}$ | (I) | $4:2$ |
| (B) | $\mathrm{H}_2 \mathrm{O}$ | (II) | $4:1$ |
| (C) | $\mathrm{SO_2}$ | (III) | $2:3$ |
| (D) | $\mathrm{XeF_4}$ | (IV) | $2:2$ |
Choose the correct answer from the options given below:
The first transition series metal ' $M$ ' has the highest enthalpy of atomisation in its series. One of its aquated ion $\left(\mathrm{M}^{\mathrm{n}+}\right)$ exists in green colour. The nature of the oxide formed by the above $\mathrm{M}^{\mathrm{n}+}$ ion is:
The number of valence electrons present in the metal among $\mathrm{Cr}, \mathrm{Co}, \mathrm{Fe}$ and Ni which has the lowest enthalpy of atomisation is :
Which of the following is the correct IUPAC name of given organic compound (X)?

At the sea level, the dry air mass percentage composition is given as nitrogen gas: 70.0 , oxygen gas: 27.0 and argon gas: 3.0 . If total pressure is 1.15 atm , then calculate the ratio of following respectively:
(i) partial pressure of nitrogen gas to partial pressure of oxygen gas
(ii) partial pressure of oxygen gas to partial pressure of argon gas
(Given: Molar mass of N, O and Ar are 14, 16 and $40 \mathrm{~g} \mathrm{~mol}^{-1}$ respectively.)
Given below are two statements :
Statement I : Dimethyl ether is completely soluble in water. However, diethyl ether is soluble in water to a very small extent.
Statement II : Sodium metal can be used to dry diethyl ether and not ethyl alcohol.
In the light of given statements. choose the correct answer from the options given below
Given below are two statements :
Statement I : Mohr's salt is composed of only three types of ions-ferrous, ammonium and sulfate.
Statement II : If the molar conductance at infinite dilution for ferrous, ammonium and sulfate ions are $x_1, x_2$ and $x_3 \mathrm{~S} \mathrm{~cm}^2 \mathrm{~mol}^{-1}$, respectively then the molar conductance for Mohr's salt solution at infinite dilution would be given by $x_1+x_2+2 x_3$
In the light of the given statements, choose the correct answer from the options given below :
Given below are two statements:
Statement I: D-(+)-glucose + D-(+) fructose $\xrightarrow{-\mathrm{H}_2 \mathrm{O}}$ Sucrose
$$\text { sucrose } \xrightarrow{\text { hydrolysis }} \mathrm{D}-(+) \text { glucose }+\mathrm{D}-(+) \text { fructose }$$
Statement II: Invert sugar is formed during sucrose hydrolysis
In the light of the above statements, choose the correct answer from the options given below
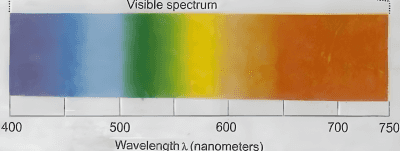
Which of the following statements are correct, if the threshold frequency of caesium is $5.16 \times$ $10^{14} \mathrm{~Hz}$ ?
A. When Cs is placed inside a vacuum chamber with an ammeter connected to it and yellow light is focused on Cs , the ammeter shows the presence of current.
B. When the brightness of the yellow light is dimmed, the value of the current in the ammeter is reduced.
C. When a red light is used instead of the yellow light, the current produced is higher with respect to the yellow light.
D. When a blue light is used, the ammeter shows the formation of current.
E. When a white light is used. the ammeter shows formation of current.
Choose the correct answer from the options given below:
The group 14 elements $A$ and $B$ have the first ionisation enthalpy values of 708 and $715 \mathrm{~kJ} \mathrm{~mol}^{-1}$ respectively. The above values are lowest among their group members. The nature of their ions $\mathrm{A}^{2+}$ and $\mathrm{B}^{4+}$ respectively is
The reactions which cannot be applied to prepare an alkene by elimination, are

Choose the correct answer from the options given below:
When a salt is treated with sodium hydroxide solution it gives gas X . On passing gas X through reagent Y a brown coloured precipitate is formed. X and Y respectively, are
Reaction $\mathrm{A}(\mathrm{g}) \rightarrow 2 \mathrm{~B}(\mathrm{~g})+\mathrm{C}(\mathrm{g})$ is a first order reaction. It was started with pure A
| t/min | Pressure of system at time t/mm Hg |
|---|---|
| 10 | 160 |
| $\infty$ | 240 |
Which of the following option is incorrect?
An octahedral complex having molecular composition $\mathrm{Co} \cdot 5 \mathrm{NH}_3 \cdot \mathrm{Cl}^2 . \mathrm{SO}_4$ has two isomers A and B. The solution of A gives a white precipitate with $\mathrm{AgNO}_3$ solution and the solution of B gives white precipitate with $\mathrm{BaCl}_2$ solution. The type of isomerism exhibited by the complex is,
Given below are two statements:
Statement I : Ozonolysis followed by treatment with $\mathrm{Zn}, \mathrm{H}_2 \mathrm{O}$ of cis-2-butene gives ethanal.
Statement II : The product obtained by ozonolysis followed by treatment with $\mathrm{Zn}, \mathrm{H}_2 \mathrm{O}$ of 3, 6-dimethyloct-4-ene has no chiral carbon atom.
In the light of the above statements, choose the correct answer from the options given below
An organic compound weighing 500 mg , produced 220 mg of $\mathrm{CO}_2$, on complete combustion. The percentage composition of carbon in the compound is _________ $\%$. (nearest integer)
(Given molar mass in $\mathrm{g} \mathrm{mol}^{-1}$ of $\mathrm{C}: 12, \mathrm{O}: 16$ )
The number of paramagnetic complexes among $\left[\mathrm{FeF}_6\right]^{3-},\left[\mathrm{Fe}(\mathrm{CN})_6\right]^{3-},\left[\mathrm{Mn}(\mathrm{CN})_6\right]^{3-}$, $\left[\mathrm{Co}\left(\mathrm{C}_2 \mathrm{O}_4\right)_3\right]^{3-},\left[\mathrm{MnCl}_6\right]^{3-}$, and $\left[\mathrm{CoF}_6\right]^{3-}$, which involved $\mathrm{d}^2 \mathrm{sp}^3$ hybridization is _________.
1 Faraday electricity was passed through $\mathrm{Cu}^{2+}(1.5 \mathrm{M}, 1 \mathrm{~L}) / \mathrm{Cu}$ and 0.1 Faraday was passed through $\mathrm{Ag}^{+}(0.2 \mathrm{M}, 1 \mathrm{~L}) / \mathrm{Ag}$ electrolytic cells. After this the two cells were connected as shown below to make an electrochemical cell. The emf of the cell thus formed at 298 K is __________ mV (nearest integer)

$$\begin{aligned} \text { Given : } \mathrm{E}^{\circ} \mathrm{Cu}^{2+} / \mathrm{Cu} & =0.34 \mathrm{~V} \\\\ \mathrm{E}^{\circ} \mathrm{Ag}^{+} / \mathrm{Ag} & =0.8 \mathrm{~V} \\\\ \frac{2 \cdot 303 \mathrm{RT}}{\mathrm{~F}} & =0.06 \mathrm{~V} \end{aligned}$$
The percentage dissociation of a salt $\left(\mathrm{MX}_3\right)$ solution at given temperature (van't Hoff factor $\mathrm{i}=2$ ) is ___________ %(Nearest integer)
Thyroxine, the hormone has given below structure

The percentage of iodine in thyroxine is __________ %. (nearest integer)
(Given molar mass in $\mathrm{g} \mathrm{mol}^{-1} \mathrm{C}: 12, \mathrm{H}: 1, \mathrm{O}: 16, \mathrm{~N}: 14, \mathrm{I}: 127$ )
Mathematics
From a group of 7 batsmen and 6 bowlers, 10 players are to be chosen for a team, which should include atleast 4 batsmen and atleast 4 bowlers. One batsmen and one bowler who are captain and vice-captain respectively of the team should be included. Then the total number of ways such a selection can be made, is
Let $C_1$ be the circle in the third quadrant of radius 3 , that touches both coordinate axes. Let $C_2$ be the circle with centre $(1,3)$ that touches $\mathrm{C}_1$ externally at the point $(\alpha, \beta)$. If $(\beta-\alpha)^2=\frac{m}{n}$ , $\operatorname{gcd}(m, n)=1$, then $m+n$ is equal to
Let P be the parabola, whose focus is $(-2,1)$ and directrix is $2 x+y+2=0$. Then the sum of the ordinates of the points on P, whose abscissa is $-$2, is
The mean and standard deviation of 100 observations are 40 and 5.1 , respectively. By mistake one observation is taken as 50 instead of 40 . If the correct mean and the correct standard deviation are $\mu$ and $\sigma$ respectively, then $10(\mu+\sigma)$ is equal to
Let ABC be the triangle such that the equations of lines AB and AC be $3 y-x=2$ and $x+y=2$, respectively, and the points B and C lie on $x$-axis. If P is the orthocentre of the triangle ABC , then the area of the triangle PBC is equal to
$\lim _\limits{x \rightarrow 0^{+}} \frac{\tan \left(5(x)^{\frac{1}{3}}\right) \log _e\left(1+3 x^2\right)}{\left(\tan ^{-1} 3 \sqrt{x}\right)^2\left(e^{5(x)^{\frac{4}{3}}}-1\right)}$ is equal to
Let $y=y(x)$ be the solution curve of the differential equation
$x\left(x^2+e^x\right) d y+\left(\mathrm{e}^x(x-2) y-x^3\right) \mathrm{d} x=0, x>0$, passing through the point $(1,0)$. Then $y(2)$ is equal to :
Let $A$ be a $3 \times 3$ matrix such that $|\operatorname{adj}(\operatorname{adj}(\operatorname{adj} \mathrm{A}))|=81$.
If $S=\left\{n \in \mathbb{Z}:(|\operatorname{adj}(\operatorname{adj} A)|)^{\frac{(n-1)^2}{2}}=|A|^{\left(3 n^2-5 n-4\right)}\right\}$, then $\sum_\limits{n \in S}\left|A^{\left(n^2+n\right)}\right|$ is equal to :
The remainder when $\left((64)^{(64)}\right)^{(64)}$ is divided by 7 is equal to
If for $\theta \in\left[-\frac{\pi}{3}, 0\right]$, the points $(x, y)=\left(3 \tan \left(\theta+\frac{\pi}{3}\right), 2 \tan \left(\theta+\frac{\pi}{6}\right)\right)$ lie on $x y+\alpha x+\beta y+\gamma=0$, then $\alpha^2+\beta^2+\gamma^2$ is equal to :
Let the system of equations :
$$ \begin{aligned} & 2 x+3 y+5 z=9 \\ & 7 x+3 y-2 z=8 \\ & 12 x+3 y-(4+\lambda) z=16-\mu \end{aligned}$$
have infinitely many solutions. Then the radius of the circle centred at $(\lambda, \mu)$ and touching the line $4 x=3 y$ is :
Let the set of all values of $p \in \mathbb{R}$, for which both the roots of the equation $x^2-(p+2) x+(2 p+9)=0$ are negative real numbers, be the interval $(\alpha, \beta]$. Then $\beta-2 \alpha$ is equal to
Among the statements
(S1) : The set $\left\{z \in \mathbb{C}-\{-i\}:|z|=1\right.$ and $\frac{z-i}{z+i}$ is purely real $\}$ contains exactly two elements, and
(S2) : The set $\left\{z \in \mathbb{C}-\{-1\}:|z|=1\right.$ and $\frac{z-1}{z+1}$ is purely imaginary $\}$ contains infinitely many elements.
The integral $\int_0^\pi \frac{(x+3) \sin x}{1+3 \cos ^2 x} d x$ is equal to
Let the line L pass through $(1,1,1)$ and intersect the lines $\frac{x-1}{2}=\frac{y+1}{3}=\frac{z-1}{4}$ and $\frac{x-3}{1}=\frac{y-4}{2}=\frac{z}{1}$. Then, which of the following points lies on the line $L$ ?
If the area of the region bounded by the curves $y=4-\frac{x^2}{4}$ and $y=\frac{x-4}{2}$ is equal to $\alpha$, then $6 \alpha$. equals
If the shortest distance between the lines $\frac{x-1}{2}=\frac{y-2}{3}=\frac{z-3}{4}$ and $\frac{x}{1}=\frac{y}{\alpha}=\frac{z-5}{1}$ is $\frac{5}{\sqrt{6}}$, then the sum of all possible values of $\alpha$ is
Let $x=-1$ and $x=2$ be the critical points of the function $f(x)=x^3+a x^2+b \log _{\mathrm{e}}|x|+1, x \neq 0$. Let $m$ and M respectively be the absolute minimum and the absolute maximum values of $f$ in the interval $\left[-2,-\frac{1}{2}\right]$. Then $|\mathrm{M}+m|$ is equal to $\left(\right.$ Take $\left.\log _{\mathrm{e}} 2=0.7\right):$
Let the angle $\theta, 0<\theta<\frac{\pi}{2}$ between two unit vectors $\hat{a}$ and $\hat{b}$ be $\sin ^{-1}\left(\frac{\sqrt{65}}{9}\right)$. If the vector $\vec{c}=3 \hat{a}+6 \hat{b}+9(\hat{a} \times \hat{b})$, then the value of $9(\vec{c} \cdot \hat{a})-3(\vec{c} \cdot \hat{b})$ is
Let $x_1, x_2, x_3, x_4$ be in a geometric progression. If $2,7,9,5$ are subtracted respectively from $x_1, x_2, x_3, x_4$, then the resulting numbers are in an arithmetic progression. Then the value of $\frac{1}{24}\left(x_1 x_2 x_3 x_4\right)$ is:
The number of singular matrices of order 2 , whose elements are from the set $\{2,3,6,9\}$, is __________.
The number of relations on the set $A=\{1,2,3\}$, containing at most 6 elements including $(1,2)$, which are reflexive and transitive but not symmetric, is __________.
The number of points of discontinuity of the function $f(x)=\left[\frac{x^2}{2}\right]-[\sqrt{x}], x \in[0,4]$, where $[\cdot]$ denotes the greatest integer function, is ________.
For $n \geq 2$, let $S_n$ denote the set of all subsets of $\{1,2, \ldots, n\}$ with no two consecutive numbers. For example $\{1,3,5\} \in S_6$, but $\{1,2,4\} \notin S_6$. Then $n\left(S_5\right)$ is equal to ________
Consider the hyperbola $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ having one of its focus at $\mathrm{P}(-3,0)$. If the latus ractum through its other focus subtends a right angle at P and $a^2 b^2=\alpha \sqrt{2}-\beta, \alpha, \beta \in \mathbb{N}$, then $\alpha+\beta$ is _________ .
Physics
If $\epsilon_0$ denotes the permittivity of free space and $\Phi_E$ is the flux of the electric field through the area bounded by the closed surface, then dimensions of $\left(\epsilon_0 \frac{d \phi_E}{d t}\right)$ are that of :
The percentage increase in magnetic field (B) when space within a current carrying solenoid is filled with magnesium (magnetic susceptibility $\chi_{\mathrm{Mg}}=1.2 \times 10^{-5}$ ) is :
An object of mass 1000 g experiences a time dependent force $\vec{F}=\left(2 t \hat{i}+3 t^2 \hat{j}\right) N$. The power generated by the force at time $t$ is:
A rod of length 5 L is bent right angle keeping one side length as 2 L .

The position of the centre of mass of the system :
(Consider $\mathrm{L}=10 \mathrm{~cm}$)
An ac current is represented as
$$i=5 \sqrt{2}+10 \cos \left(650 \pi t+\frac{\pi}{6}\right) A m p$$
The r.m.s value of the current is
Two harmonic waves moving in the same direction superimpose to form a wave $x=\mathrm{a} \cos (1.5 \mathrm{t}) \cos (50.5 \mathrm{t})$ where t is in seconds. Find the period with which they beat. (close to nearest integer)
A particle of charge $q$, mass $m$ and kinetic energy $E$ enters in magnetic field perpendicular to its velocity and undergoes a circular arc of radius (r). Which of the following curves represents the variation of $r$ with $E$ ?
A wire of resistance $R$ is bent into a triangular pyramid as shown in figure with each segment having same length. The resistance between points $A$ and $B$ is $R / n$. The value of $n$ is :

Two wires A and B are made of same material having ratio of lengths $\frac{L_A}{L_B}=\frac{1}{3}$ and their diameters ratio $\frac{d_A}{d_B}=2$. If both the wires are stretched using same force, what would be the ratio of their respective elongations?
Two plane polarized light waves combine at a certain point whose electric field components are
$$\begin{aligned} & E_1=E_0 \operatorname{Sin} \omega t \\ & E_2=E_0 \operatorname{Sin}\left(\omega t+\frac{\pi}{3}\right) \end{aligned}$$
Find the amplitude of the resultant wave.
Uniform magnetic fields of different strengths $\left(B_1\right.$ and $\left.B_2\right)$, both normal to the plane of the paper exist as shown in the figure. A charged particle of mass $m$ and charge $q$, at the interface at an instant, moves into the region 2 with velocity $v$ and returns to the interface. It continues to move into region 1 and finally reaches the interface. What is the displacement of the particle during this movement along the interface?

(Consider the velocity of the particle to be normal to the magnetic field and $\mathrm{B}_2>\mathrm{B}_1$ )
Two projectiles are fired from ground with same initial speeds from same point at angles $\left(45^{\circ}+\right.$ $\alpha)$ and $\left(45^{\circ}-\alpha\right)$ with horizontal direction. The ratio of their times of flights is
In a hydrogen like ion, the energy difference between the $2^{\text {nd }}$ excitation energy state and ground is 108.8 eV . The atomic number of the ion is:
Two thin convex lenses of focal lengths 30 cm and 10 cm are placed coaxially, 10 cm apart. The power of this combination is:
Two charges $q_1$ and $q_2$ are separated by a distance of 30 cm . A third charge $q_3$ initially at ' C ' as shown in the figure, is moved along the circular path of radius 40 cm from C to D . If the difference in potential energy due to movement of $q_3$ from C to D is given by $\frac{q_3 \mathrm{~K}}{4 \pi \epsilon_0}$, the value of K is :

A lens having refractive index 1.6 has focal length of 12 cm , when it is in air. Find the focal length of the lens when it is placed in water. (Take refractive index of water as 1.28)
For a hydrogen atom, the ratio of the largest wavelength of Lyman series to that of the Balmer series is
In the following circuit, the reading of the ammeter will be
$($ Take Zener breakdown voltage $=4 \mathrm{~V})$

A cubic block of mass $m$ is sliding down on an inclined plane at $60^{\circ}$ with an acceleration of $\frac{g}{2}$, the value of coefficient of kinetic friction is
Match the List I with List II
| List - I | List - II | ||
|---|---|---|---|
| (A) | Triatomic rigid gas | (I) | $\frac{C_p}{C_v}=\frac{5}{3}$ |
| (B) | Diatomic non-rigid gas | (II) | $\frac{C_p}{C_v}=\frac{7}{5}$ |
| (C) | Monoatomic gas | (III) | $\frac{C_p}{C_v}=\frac{4}{3}$ |
| (D) | Diatomic rigid gas | (IV) | $\frac{C_p}{C_v}=\frac{9}{7}$ |
Choose the correct answer from the options given below:
A wire of length 10 cm and diameter 0.5 mm is used in a bulb. The temperature of the wire is $1727^{\circ} \mathrm{C}$ and power radiated by the wire is 94.2 W . Its emissivity is $\frac{x}{8}$ where $x=$ _________.
(Given $\sigma=6.0 \times 10^{-8} \mathrm{~W} \mathrm{~m}^{-2} \mathrm{~K}^{-4}, \pi=3.14$ and assume that the emissivity of wire material is same at all wavelength.)
$\mathrm{A}, \mathrm{B}$ and C are disc, solid sphere and spherical shell respectively with same radii and masses. These masses are placed as shown in figure.

The moment of inertia of the given system about PQ axis is $\frac{x}{15} \mathrm{I}$, where I is the moment of inertia of the disc about its diameter. The value of $x$ is ____________.
For ac circuit shown in figure, $\mathrm{R}=100 \mathrm{k} \Omega$ and $\mathrm{C}=100 \mathrm{pF}$ and the phase difference between $\mathrm{V}_{\text {in }}$ and $\left(\mathrm{V}_{\mathrm{B}}-\mathrm{V}_{\mathrm{A}}\right)$ is $90^{\circ}$. The input signal frequency is $10^x \mathrm{rad} / \mathrm{sec}$, where ' $x$ ' is __________ .

A container contains a liquid with refractive index of 1.2 up to a height of 60 cm and another liquid having refractive index 1.6 is added to height H above first liquid. If viewed from above, the apparent shift in the position of bottom of container is 40 cm . The value of H is ________ cm . (Consider liquids are immisible)
An ideal gas has undergone through the cyclic process as shown in the figure. Work done by the gas in the entire cycle is ________ $\times 10^{-1} \mathrm{~J}$. (Take $\pi=3.14$ )
