When a hydrocarbon A undergoes complete combustion it requires 11 equivalents of oxygen and produces 4 equivalents of water. What is the molecular formula of $A$ ?

Cyclohexylamine when treated with nitrous acid yields $(\mathrm{P})$. On treating $(\mathrm{P})$ with $\mathrm{PCC}$ results in (Q). When $(\mathrm{Q})$ is heated with dil. $\mathrm{NaOH}$ we get $(\mathrm{R})$ The final product (R) is :

Compound $\mathrm{A}, \mathrm{C}_{5} \mathrm{H}_{10} \mathrm{O}_{5}$, given a tetraacetate with $\mathrm{Ac}_{2} \mathrm{O}$ and oxidation of $\mathrm{A}$ with $\mathrm{Br}_{2}-\mathrm{H}_{2} \mathrm{O}$ gives an acid, $\mathrm{C}_{5} \mathrm{H}_{10} \mathrm{O}_{6}$. Reduction of $\mathrm{A}$ with $\mathrm{HI}$ gives isopentane. The possible structure of $\mathrm{A}$ is

An organic compound $[\mathrm{A}]\left(\mathrm{C}_{4} \mathrm{H}_{11} \mathrm{~N}\right)$, shows optical activity and gives $\mathrm{N}_{2}$ gas on treatment with $\mathrm{HNO}_{2}$. The compound $[\mathrm{A}]$ reacts with $\mathrm{PhSO}_{2} \mathrm{Cl}$ producing a compound which is soluble in $\mathrm{KOH}$. The structure of $\mathrm{A}$ is :

Which of the following elements have half-filled f-orbitals in their ground state?

(Given : atomic number $\mathrm{Sm}=62 ; \mathrm{Eu}=63 ; \mathrm{Tb}=65 ; \mathrm{Gd}=64, \mathrm{Pm}=61$ )

A. $\mathrm{Sm}$

B. Eu

C. $\mathrm{Tb}$

D. Gd

E. $\mathrm{Pm}$

Choose the correct answer from the options given below :

Evaluate the following statements for their correctness.

A. The elevation in boiling point temperature of water will be same for $0.1 \mathrm{M} \, \mathrm{NaCl}$ and $0.1 \mathrm{M}$ urea.

B. Azeotropic mixtures boil without change in their composition.

C. Osmosis always takes place from hypertonic to hypotonic solution.

D. The density of $32 \% \, \mathrm{H}_{2} \mathrm{SO}_{4}$ solution having molarity $4.09 ~\mathrm{M}$ is approximately $1.26 \mathrm{~g} \mathrm{~mL}^{-1}$

E. A negatively charged sol is obtained when KI solution is added to silver nitrate solution.

Choose the correct answer from the options given below :

Arrange the following orbitals in decreasing order of energy.

A. $\mathrm{n}=3, \mathrm{l}=0, \mathrm{~m}=0$

B. $\mathrm{n}=4, \mathrm{l}=0, \mathrm{~m}=0$

C. $\mathrm{n}=3, \mathrm{l}=1, \mathrm{~m}=0$

D. $\mathrm{n}=3, \mathrm{l}=2, \mathrm{~m}=1$

The correct option for the order is :

In Dumas method for the estimation of $\mathrm{N}_{2}$, the sample is heated with copper oxide and the gas evolved is passed over :

Given below are two statements : one is labelled as Assertion (A) and the other is labelled as Reason (R)

Assertion (A): The first ionization enthalpy of $3 \mathrm{~d}$ series elements is more than that of group 2 metals

Reason (R): In 3d series of elements successive filling of d-orbitals takes place.

In the light of the above statements, choose the correct answer from the options given below :

A hydrocarbon ' $\mathrm{X}$ ' with formula $\mathrm{C}_{6} \mathrm{H}_{8}$ uses two moles of $\mathrm{H}_{2}$ on catalytic hydrogenation of its one mole. On ozonolysis, ' $\mathrm{X}$ ' yields two moles of methane dicarbaldehyde. The hydrocarbon ' $\mathrm{X}$ ' is:

Given below are two statements :

Statement I: Upon heating a borax bead dipped in cupric sulphate in a luminous flame, the colour of the bead becomes green

Statement II: The green colour observed is due to the formation of copper(I) metaborate

In light of the above statements, choose the most appropriate answer from the options given below :

In the following halogenated organic compounds, the one with the maximum number of chlorine atoms in its structure is :

The incorrect statement for the use of indicators in acid-base titration is :

Assume carbon burns according to the following equation :

$2 \mathrm{C}_{(\mathrm{s})}+\mathrm{O}_{2(\mathrm{~g})} \rightarrow 2 \mathrm{CO}(\mathrm{g})$

when $12 \mathrm{~g}$ carbon is burnt in $48 \mathrm{~g}$ of oxygen, the volume of carbon monoxide produced is ___________ $\times 10^{-1} \mathrm{~L}$ at STP [nearest integer]

[Given: Assume $\mathrm{CO}$ as ideal gas, Mass of $\mathrm{C}$ is $12 \mathrm{~g} \mathrm{~mol}^{-1}$, Mass of $\mathrm{O}$ is $16 \mathrm{~g} \mathrm{~mol}^{-1}$ and molar volume of an ideal gas at STP is $22.7 \mathrm{~L} \mathrm{~mol}^{-1}$ ]

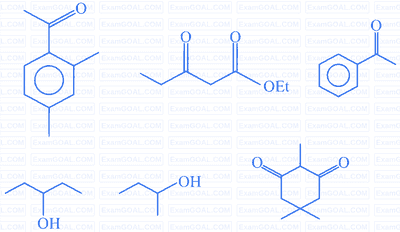
The number of molecules which gives the haloform test among the following molecules is ________.

Amongst the following, the number of species having the linear shape is _________.

$\mathrm{XeF}_{2}, \mathrm{I}_{3}^{+}, \mathrm{C}_{3} \mathrm{O}_{2}, \mathrm{I}_{3}^{-}, \mathrm{CO}_{2}, \mathrm{SO}_{2}, \mathrm{BeCl}_{2}$ and $\mathrm{BCl}_{2}^{\ominus}$

Enthalpies of formation of $\mathrm{CCl}_{4}(\mathrm{~g}), \mathrm{H}_{2} \mathrm{O}(\mathrm{g}), \mathrm{CO}_{2}(\mathrm{~g})$ and $\mathrm{HCl}(\mathrm{g})$ are $-105,-242,-394$ and $-92 ~\mathrm{kJ}$ $\mathrm{mol}^{-1}$ respectively. The magnitude of enthalpy of the reaction given below is _________ $\mathrm{kJ} ~\mathrm{mol}^{-1}$. (nearest integer)

$\mathrm{CCl}_{4}(\mathrm{~g})+2 \mathrm{H}_{2} \mathrm{O}(\mathrm{g}) \rightarrow \mathrm{CO}_{2}(\mathrm{~g})+4 \mathrm{HCl}(\mathrm{g})$

At $298 \mathrm{~K}$, the solubility of silver chloride in water is $1.434 \times 10^{-3} \mathrm{~g} \mathrm{~L}^{-1}$. The value of $-\log \mathrm{K}_{\mathrm{sp}}$ for silver chloride is _________.

(Given mass of $\mathrm{Ag}$ is $107.9 \mathrm{~g} \mathrm{~mol}^{-1}$ and mass of $\mathrm{Cl}$ is $35.5 \mathrm{~g} \mathrm{~mol}^{-1}$ )

The resistivity of a $0.8 \mathrm{M}$ solution of an electrolyte is $5 \times 10^{-3} \Omega~ \mathrm{cm}$.

Its molar conductivity is _________ $\times 10^{4}~ \Omega^{-1} \mathrm{~cm}^{2} \mathrm{~mol}^{-1}$. (Nearest integer)

A sample of a metal oxide has formula $\mathrm{M}_{0.83} \mathrm{O}_{1.00}$. The metal $\mathrm{M}$ can exist in two oxidation states $+2$ and $+3$.

In the sample of $\mathrm{M}_{0.83} \mathrm{O}_{1.00}$, the percentage of metal ions existing in $+2$ oxidation state is __________ $\%$. (nearest integer)

If the CFSE of $\left[\mathrm{Ti}\left(\mathrm{H}_{2} \mathrm{O}\right)_{6}\right]^{3+}$ is $-96.0 \mathrm{~kJ} / \mathrm{mol}$, this complex will absorb maximum at wavelength ___________ $\mathrm{nm}$. (nearest integer)

Assume Planck's constant (h) $=6.4 \times 10^{-34} \mathrm{Js}$, Speed of light $(\mathrm{c})=3.0 \times 10^{8} \mathrm{~m} / \mathrm{s}$ and Avogadro's

Constant $\left(\mathrm{N}_{\mathrm{A}}\right)=6 \times 10^{23} / \mathrm{mol}$

The rate constant for a first order reaction is $20 \mathrm{~min}^{-1}$. The time required for the initial concentration of the reactant to reduce to its $\frac{1}{32}$ level is _______ $\times 10^{-2} \mathrm{~min}$. (Nearest integer)

(Given : $\ln 10=2.303$ and $$ \log 2=0.3010 \text { )}$$

Let $\vec{a}=\hat{i}+2 \hat{j}+3 \hat{k}, \vec{b}=\hat{i}-\hat{j}+2 \hat{k}$ and $\vec{c}=5 \hat{i}-3 \hat{j}+3 \hat{k}$ be three vectors. If $\vec{r}$ is a vector such that, $\vec{r} \times \vec{b}=\vec{c} \times \vec{b}$ and $\vec{r} \cdot \vec{a}=0$, then $25|\vec{r}|^{2}$ is equal to :

Let $f: \mathbb{R}-\{2,6\} \rightarrow \mathbb{R}$ be real valued function

defined as $f(x)=\frac{x^2+2 x+1}{x^2-8 x+12}$.

Then range of $f$ is

The equation $\mathrm{e}^{4 x}+8 \mathrm{e}^{3 x}+13 \mathrm{e}^{2 x}-8 \mathrm{e}^{x}+1=0, x \in \mathbb{R}$ has :

Let (a, b) $\subset(0,2 \pi)$ be the largest interval for which $\sin ^{-1}(\sin \theta)-\cos ^{-1}(\sin \theta)>0, \theta \in(0,2 \pi)$, holds.

If $\alpha x^{2}+\beta x+\sin ^{-1}\left(x^{2}-6 x+10\right)+\cos ^{-1}\left(x^{2}-6 x+10\right)=0$ and $\alpha-\beta=b-a$, then $\alpha$ is equal to :

Let $\alpha>0$. If $\int\limits_0^\alpha \frac{x}{\sqrt{x+\alpha}-\sqrt{x}} \mathrm{~d} x=\frac{16+20 \sqrt{2}}{15}$, then $\alpha$ is equal to :

Let the mean and standard deviation of marks of class A of 100 students be respectively 40 and $\alpha(>$ 0 ), and the mean and standard deviation of marks of class $B$ of $n$ students be respectively 55 and 30 $-\alpha$. If the mean and variance of the marks of the combined class of $100+\mathrm{n}$ studants are respectively 50 and 350 , then the sum of variances of classes $A$ and $B$ is :

Let $a_1, a_2, a_3, \ldots$ be an A.P. If $a_7=3$, the product $a_1 a_4$ is minimum and the sum of its first $n$ terms is zero, then $n !-4 a_{n(n+2)}$ is equal to :

The complex number $z=\frac{i-1}{\cos \frac{\pi}{3}+i \sin \frac{\pi}{3}}$ is equal to :

Among the relations

$\mathrm{S}=\left\{(\mathrm{a}, \mathrm{b}): \mathrm{a}, \mathrm{b} \in \mathbb{R}-\{0\}, 2+\frac{\mathrm{a}}{\mathrm{b}}>0\right\}$

and $\mathrm{T}=\left\{(\mathrm{a}, \mathrm{b}): \mathrm{a}, \mathrm{b} \in \mathbb{R}, \mathrm{a}^{2}-\mathrm{b}^{2} \in \mathbb{Z}\right\}$,

The absolute minimum value, of the function

$f(x)=\left|x^{2}-x+1\right|+\left[x^{2}-x+1\right]$,

where $[t]$ denotes the greatest integer function, in the interval $[-1,2]$, is :

If $\phi(x)=\frac{1}{\sqrt{x}} \int\limits_{\frac{\pi}{4}}^x\left(4 \sqrt{2} \sin t-3 \phi^{\prime}(t)\right) d t, x>0$,

then $\emptyset^{\prime}\left(\frac{\pi}{4}\right)$ is equal to :

Let $\mathrm{H}$ be the hyperbola, whose foci are $(1 \pm \sqrt{2}, 0)$ and eccentricity is $\sqrt{2}$. Then the length of its latus rectum is :

$$ \lim\limits_{x \rightarrow \infty} \frac{(\sqrt{3 x+1}+\sqrt{3 x-1})^6+(\sqrt{3 x+1}-\sqrt{3 x-1})^6}{\left(x+\sqrt{x^2-1}\right)^6+\left(x-\sqrt{x^2-1}\right)^6} x^3 $$

Let $y=y(x)$ be the solution of the differential equation

$\left(3 y^{2}-5 x^{2}\right) y \mathrm{~d} x+2 x\left(x^{2}-y^{2}\right) \mathrm{d} y=0$

such that $y(1)=1$. Then $\left|(y(2))^{3}-12 y(2)\right|$ is equal to :

The set of all values of $a^{2}$ for which the line $x+y=0$ bisects two distinct chords drawn from a point $\mathrm{P}\left(\frac{1+a}{2}, \frac{1-a}{2}\right)$ on the circle $2 x^{2}+2 y^{2}-(1+a) x-(1-a) y=0$, is equal to :

Let the area of the region

$\left\{(x, y):|2 x-1| \leq y \leq\left|x^{2}-x\right|, 0 \leq x \leq 1\right\}$ be $\mathrm{A}$.

Then $(6 \mathrm{~A}+11)^{2}$ is equal to

The coefficient of $x^{-6}$, in the

expansion of $\left(\frac{4 x}{5}+\frac{5}{2 x^{2}}\right)^{9}$, is

Let $\mathrm{A}=\left[\mathrm{a}_{i j}\right], \mathrm{a}_{i j} \in \mathbb{Z} \cap[0,4], 1 \leq i, j \leq 2$.

The number of matrices A such that the sum of all entries is a prime number $\mathrm{p} \in(2,13)$ is __________.

If ${ }^{2 n+1} \mathrm{P}_{n-1}:{ }^{2 n-1} \mathrm{P}_{n}=11: 21$,

then $n^{2}+n+15$ is equal to :

Let $\vec{a}, \vec{b}, \vec{c}$ be three vectors such that

$|\vec{a}|=\sqrt{31}, 4|\vec{b}|=|\vec{c}|=2$ and $2(\vec{a} \times \vec{b})=3(\vec{c} \times \vec{a})$.

If the angle between $\vec{b}$ and $\vec{c}$ is $\frac{2 \pi}{3}$, then $\left(\frac{\vec{a} \times \vec{c}}{\vec{a} \cdot \vec{b}}\right)^{2}$ is equal to __________.

Let A be the event that the absolute difference between two randomly choosen real numbers in the sample space $[0,60]$ is less than or equal to a . If $\mathrm{P}(\mathrm{A})=\frac{11}{36}$, then $\mathrm{a}$ is equal to _______.

If the constant term in the binomial expansion of $\left(\frac{x^{\frac{5}{2}}}{2}-\frac{4}{x^{l}}\right)^{9}$ is $-84$ and the coefficient of $x^{-3 l}$ is $2^{\alpha} \beta$, where $\beta<0$ is an odd number, then $|\alpha l-\beta|$ is equal to ________.

For a solid rod, the Young's modulus of elasticity is $3.2 \times 10^{11} \mathrm{Nm}^{-2}$ and density is $8 \times 10^3 \mathrm{~kg} \mathrm{~m}^{-3}$. The velocity of longitudinal wave in the rod will be.

Heat energy of $735 \mathrm{~J}$ is given to a diatomic gas allowing the gas to expand at constant pressure. Each gas molecule rotates around an internal axis but do not oscillate. The increase in the internal energy of the gas will be :

The radius of electron's second stationary orbit in Bohr's atom is R. The radius of 3rd orbit will be

If the two metals $\mathrm{A}$ and $\mathrm{B}$ are exposed to radiation of wavelength $350 \mathrm{~nm}$. The work functions of metals $\mathrm{A}$ and $\mathrm{B}$ are $4.8 \mathrm{eV}$ and $2.2 \mathrm{eV}$. Then choose the correct option.

The number of turns of the coil of a moving coil galvanometer is increased in order to increase current sensitivity by $50 \%$. The percentage change in voltage sensitivity of the galvanometer will be :

Match List I with List II

LIST I		LIST II
A.	Microwaves	I.	Physiotherapy
B.	UV rays	II.	Treatment of cancer
C.	Infra-red light	III.	Lasik eye surgery
D.	X-ray	IV.	Aircraft navigation

Choose the correct answer from the options given below:

The $\mathrm{H}$ amount of thermal energy is developed by a resistor in $10 \mathrm{~s}$ when a current of $4 \mathrm{~A}$ is passed through it. If the current is increased to $16 \mathrm{~A}$, the thermal energy developed by the resistor in $10 \mathrm{~s}$ will be :

A body weight $\mathrm{W}$, is projected vertically upwards from earth's surface to reach a height above the earth which is equal to nine times the radius of earth. The weight of the body at that height will be :

Considering a group of positive charges, which of the following statements is correct ?

A body of mass $10 \mathrm{~kg}$ is moving with an initial speed of $20 \mathrm{~m} / \mathrm{s}$. The body stops after $5 \mathrm{~s}$ due to friction between body and the floor. The value of the coefficient of friction is:

(Take acceleration due to gravity $g=10 \mathrm{~ms}^{-2}$ )

A hypothetical gas expands adiabatically such that its volume changes from 08 litres to 27 litres. If the ratio of final pressure of the gas to initial pressure of the gas is $\frac{16}{81}$. Then the ratio of $\frac{\mathrm{Cp}}{\mathrm{Cv}}$ will be.

A long conducting wire having a current I flowing through it, is bent into a circular coil of $\mathrm{N}$ turns. Then it is bent into a circular coil of $\mathrm{n}$ turns. The magnetic field is calculated at the centre of coils in both the cases. The ratio of the magnetic field in first case to that of second case is :

A microscope is focused on an object at the bottom of a bucket. If liquid with refractive index $\frac{5}{3}$ is poured inside the bucket, then the microscope has to be raised by $30 \mathrm{~cm}$ to focus the object again. The height of the liquid in the bucket is :

Match List I with List II

LIST I		LIST II
A.	Angular momentum	I.	$\left[\mathrm{ML}^{2} \mathrm{~T}^{-2}\right]$
B.	Torque	II.	$\left[\mathrm{ML}^{-2} \mathrm{~T}^{-2}\right]$
C.	Stress	III	$\left[\mathrm{ML}^{2} \mathrm{~T}^{-1}\right]$
D.	Pressure gradient	IV.	$\left[\mathrm{ML}^{-1} \mathrm{~T}^{-2}\right]$

Choose the correct answer from the options given below:

Under the same load, wire A having length $5.0 \mathrm{~m}$ and cross section $2.5 \times 10^{-5} \mathrm{~m}^{2}$ stretches uniformly by the same amount as another wire B of length $6.0 \mathrm{~m}$ and a cross section of $3.0 \times 10^{-5}$ $\mathrm{m}^{2}$ stretches. The ratio of the Young's modulus of wire A to that of wire $B$ will be :

An alternating voltage source $\mathrm{V}=260 \sin (628 \mathrm{t}$ ) is connected across a pure inductor of $5 \mathrm{mH}$ Inductive reactance in the circuit is :

A stone of mass $1 \mathrm{~kg}$ is tied to end of a massless string of length $1 \mathrm{~m}$. If the breaking tension of the string is $400 \mathrm{~N}$, then maximum linear velocity, the stone can have without breaking the string, while rotating in horizontal plane, is :

A body is moving with constant speed, in a circle of radius $10 \mathrm{~m}$. The body completes one revolution in $4 \mathrm{~s}$. At the end of 3rd second, the displacement of body (in $\mathrm{m}$ ) from its starting point is :

A water heater of power $2000 \mathrm{~W}$ is used to heat water. The specific heat capacity of water is $4200 \mathrm{~J}$ $\mathrm{kg}^{-1} \mathrm{~K}^{-1}$. The efficiency of heater is $70 \%$. Time required to heat $2 \mathrm{~kg}$ of water from $10^{\circ} \mathrm{C}$ to $60^{\circ} \mathrm{C}$ is _________ s.

(Assume that the specific heat capacity of water remains constant over the temperature range of the water).

A series $\mathrm{LCR}$ circuit consists of $\mathrm{R}=80 \Omega, \mathrm{X}_{\mathrm{L}}=100 \Omega$, and $\mathrm{X}_{\mathrm{C}}=40 \Omega$. The input

voltage is 2500 $\cos (100 \pi \mathrm{t}) \mathrm{V}$. The amplitude of current, in the circuit, is _________ A.

Two light waves of wavelengths 800 and $600 \mathrm{~nm}$ are used in Young's double slit experiment to obtain interference fringes on a screen placed $7 \mathrm{~m}$ away from plane of slits. If the two slits are separated by $0.35 \mathrm{~mm}$, then shortest distance from the central bright maximum to the point where the bright fringes of the two wavelength coincide will be ______ $\mathrm{mm}$.

Two bodies are projected from ground with same speeds $40 \mathrm{~ms}^{-1}$ at two different angles with respect to horizontal. The bodies were found to have same range. If one of the body was projected at an angle of $60^{\circ}$, with horizontal then sum of the maximum heights, attained by the two projectiles, is $\mathrm{m}$. (Given $\mathrm{g}=10 \mathrm{~ms}^{-2}$ )

The displacement equations of two interfering waves are given by

$y_{1}=10 \sin \left(\omega t+\frac{\pi}{3}\right) \mathrm{cm}, y_{2}=5[\sin \omega t+\sqrt{3} \cos \omega t] \mathrm{cm}$ respectively.

The amplitude of the resultant wave is _______ $\mathrm{cm}$.

For the given circuit, in the steady state, $\left|\mathrm{V}_{\mathrm{B}}-\mathrm{V}_{\mathrm{D}}\right|=$ ________ V.

Two discs of same mass and different radii are made of different materials such that their thicknesses are $1 \mathrm{~cm}$ and $0.5 \mathrm{~cm}$ respectively. The densities of materials are in the ratio $3: 5$. The moment of inertia of these discs respectively about their diameters will be in the ratio of $\frac{x}{6}$. The value of $x$ is ________.

A ball is dropped from a height of $20 \mathrm{~m}$. If the coefficient of restitution for the collision between ball and floor is $0.5$, after hitting the floor, the ball rebounds to a height of ________ $\mathrm{m}$.

If the binding energy of ground state electron in a hydrogen atom is $13.6\, \mathrm{eV}$, then, the energy required to remove the electron from the second excited state of $\mathrm{Li}^{2+}$ will be : $x \times 10^{-1} \mathrm{eV}$. The value of $x$ is ________.

Two parallel plate capacitors $C_{1}$ and $C_{2}$ each having capacitance of $10 \mu \mathrm{F}$ are individually charged by a 100 V D.C. source. Capacitor $C_{1}$ is kept connected to the source and a dielectric slab is inserted between it plates. Capacitor $\mathrm{C}_{2}$ is disconnected from the source and then a dielectric slab is inserted in it. Afterwards the capacitor $C_{1}$ is also disconnected from the source and the two capacitors are finally connected in parallel combination. The common potential of the combination will be ________ V.

(Assuming Dielectric constant $=10$ )