Chemistry
Which of the following statements are correct?
A. The process of adding an electron to a neutral gaseous atom is always exothermic.
B. The process of removing an electron from an isolated gaseous atom is always endothermic.
C. The $1^{\text {st }}$ ionization energy of boron is less than that of beryllium.
D. The electronegativity of C is 2.5 in $\mathrm{CH}_4$ and $\mathrm{CCl}_4$
E. Li is the most electropositive among elements of group I.
Choose the correct answer from the options given below:
$$ \text {Identify }[\mathrm{A}],[\mathrm{B}] \text { and }[\mathrm{C}] \text {, respectively in the following reaction sequence : } $$

$$ \text { Match the LIST-I with LIST-II } $$
| LIST-I (Molecules/ion) |
LIST-II (Hybridisation of central atom) |
||
|---|---|---|---|
| A. | $$ \mathrm{PF}_5 $$ |
I | $$ \mathrm{dsp}^2 $$ |
| B | $$ \mathrm{SF}_6 $$ |
II | $$ \mathrm{sp}^3 \mathrm{~d} $$ |
| C | $$ \mathrm{Ni}(\mathrm{CO})_4 $$ |
III | $$ \mathrm{sp}^3 \mathrm{~d}^2 $$ |
| D | $$ \left[\mathrm{PtCl}_4\right]^{2-} $$ |
IV | $$ \mathrm{sp}^3 $$ |
$$ \text { Choose the correct answer from the options given below: } $$
$$ \text { In the following reactions, which one is NOT correct? } $$
Given below are two statements :
Statement I : The N - N single bond is weaker and longer than that of P - P single bond.
Statement II : Compounds of group 15 elements in +3 oxidation states readily undergo disproportionation reactions.
In the light of the above statements, choose the correct answer from the options given below
$$ \text { Which compound would give 3-methyl-6-oxoheptanal upon ozonolysis? } $$
Which of the following properties will change when system containing solution 1 will become solution 2 ?

$$ \text {Identify the correct statements from the following. } $$

$$ \text {Choose the correct answer from the options given below: } $$
$$ \text { The least acidic compound, among the following is: } $$

Among $10^{-9} \mathrm{~g}$ (each) of the following elements, which one will have the highest number of atoms?
Element: $\mathrm{Pb}, \mathrm{Po}, \mathrm{Pr}$ and Pt
The metal ions that have the calculated spin-only magnetic moment value of 4.9 B.M. are :
A. $\mathrm{Cr}^{2+}$
B. $\mathrm{Fe}^{2+}$
C. $\mathrm{Fe}^{3+}$
D. $\mathrm{Co}^{2+}$
E. $\mathrm{Mn}^{3+}$
Choose the correct answer from the options given below:
$$ \text { Which of the following is the correct structure of L-Fructose? } $$
Given below are two statements :
Statement I : A catalyst cannot alter the equilibrium constant $\left(\mathrm{K}_{\mathrm{c}}\right)$ of the reaction, temperature remaining constant.
Statement II : A homogenous catalyst can change the equilibrium composition of a system, temperature remaining constant.
In the light of the above statements, choose the correct answer from the options given below
In a reaction $A+B \rightarrow C$, initial concentrations of $A$ and $B$ are related as $[A]_0=8[B]_0$. The half lives of $A$ and $B$ are 10 min and 40 min , respectively. If they start to disappear at the same time, both following first order kinetics, after how much time will the concentration of both the reactants be same?
Number of molecules from below which cannot give iodoform reaction is :
Ethanol, Isopropyl alcohol, Bromoacetone, 2-Butanol, 2-Butanone, Butanal, 2-Pentanone, 3-Pentanone, Pentanal and 3-Pentanol.
$$ \text {During estimation of nitrogen by Dumas' method of compound } \mathrm{X}(0.42 \mathrm{~g}) $$
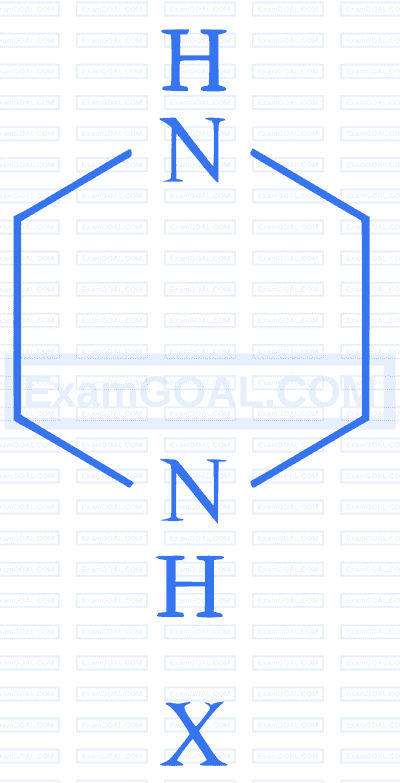
_________mL of $\mathrm{N}_2$ gas will be liberated at STP. (nearest integer)
(Given molar mass in $\mathrm{g}~ \mathrm{mol}^{-1}: \mathrm{C}: 12, \mathrm{H}: 1, \mathrm{~N}: 14$ )Consider the following reactions
$$ \begin{aligned} & \mathrm{A}+\underset{\substack{ \text { Little } \\ \text { amount }}}{\mathrm{NaCl}}+\mathrm{H}_2 \mathrm{SO}_4 \rightarrow \mathrm{CrO}_2 \mathrm{Cl}_2+\text { Side Products } \\ & \mathrm{CrO}_2 \mathrm{Cl}_{2 \text { (Vapour) }}+\mathrm{NaOH} \rightarrow \mathrm{~B}+\mathrm{NaCl}+\mathrm{H}_2 \mathrm{O} \\ & \mathrm{~B}+\mathrm{H}^{+} \rightarrow \mathrm{C}+\mathrm{H}_2 \mathrm{O} \end{aligned} $$
The number of terminal ' $O$ ' present in the compound ' C ' is__________
0.5 g of an organic compound on combustion gave 1.46 g of $\mathrm{CO}_2$ and 0.9 g of $\mathrm{H}_2 \mathrm{O}$. The percentage of carbon in the compound is _______________. (Nearest integer)
[Given : Molar mass (in $\left.\mathrm{g} \mathrm{mol}^{-1}\right) \mathrm{C}: 12, \mathrm{H}: 1, \mathrm{O}: 16$ ]
The number of optical isomers exhibited by the iron complex $(\mathrm{A})$ obtained from the following reaction is___________.
$$ \mathrm{FeCl}_3+\mathrm{KOH}+\mathrm{H}_2 \mathrm{C}_2 \mathrm{O}_4 \rightarrow \mathrm{~A} $$
Given :
$$ \begin{aligned} & \left.\Delta \mathrm{H}^{\ominus}{ }_{\text {sub }}[\mathrm{C} \text { (graphite })\right]=710 \mathrm{~kJ} \mathrm{~mol}^{-1} \\ & \Delta_{\mathrm{C}-\mathrm{H}} \mathrm{H}^{\ominus}=414 \mathrm{~kJ} \mathrm{~mol}^{-1} \\ & \Delta_{\mathrm{H}-\mathrm{H}} \mathrm{H}^{\ominus}=436 \mathrm{~kJ} \mathrm{~mol}^{-1} \\ & \Delta_{\mathrm{C}}=\mathrm{C} \mathrm{H}^{\ominus}=611 \mathrm{~kJ} \mathrm{~mol}^{-1} \end{aligned} $$
The $\Delta \mathrm{H}_{\mathrm{f}} \ominus$ for $\mathrm{CH}_2=\mathrm{CH}_2$ is_________ $\mathrm{kJ} \mathrm{mol}^{-1}$ (nearest integer value)
Mathematics
Let $\mathrm{A}=\{-3,-2,-1,0,1,2,3\}$. Let R be a relation on A defined by $x \mathrm{R} y$ if and only if $0 \leq x^2+2 y \leq 4$. Let $l$ be the number of elements in R and $m$ be the minimum number of elements required to be added in R to make it a reflexive relation. Then $l+m$ is equal to
$$ \text { Let } f(x)=\int x^3 \sqrt{3-x^2} d x \text {. If } 5 f(\sqrt{2})=-4 \text {, then } f(1) \text { is equal to } $$
Let the domain of the function $f(x)=\log _2 \log _4 \log _6\left(3+4 x-x^2\right)$ be $(a, b)$. If $\int_0^{b-a}\left[x^2\right] d x=p-\sqrt{q}-\sqrt{r}, p, q, r \in \mathbb{N}, \operatorname{gcd}(p, q, r)=1$, where $[\cdot]$ is the greatest integer function, then $p+q+r$ is equal to
Let $\alpha$ and $\beta$ be the roots of $x^2+\sqrt{3} x-16=0$, and $\gamma$ and $\delta$ be the roots of $x^2+3 x-1=0$. If $P_n=$ $\alpha^n+\beta^n$ and $Q_n=\gamma^n+\hat{o}^n$, then $\frac{P_{25}+\sqrt{3} P_{24}}{2 P_{23}}+\frac{Q_{25}-Q_{23}}{Q_{24}}$ is equal to
Let a line passing through the point $(4,1,0)$ intersect the line $\mathrm{L}_1: \frac{x-1}{2}=\frac{y-2}{3}=\frac{z-3}{4}$ at the point $A(\alpha, \beta, \gamma)$ and the line $\mathrm{L}_2: x-6=y=-z+4$ at the point $B(a, b, c)$. Then $\left|\begin{array}{lll}1 & 0 & 1 \\ \alpha & \beta & \gamma \\ a & b & c\end{array}\right|$ is equal to
Let $\quad f(x)= \begin{cases}(1+a x)^{1 / x} & , x<0 \\ 1+b, & x=0 \\ \frac{(x+4)^{1 / 2}-2}{(x+c)^{1 / 3}-2}, & x>0\end{cases}$ be continuous at $x=0$. Then $e^a b c$ is equal to:
A line passing through the point $P(\sqrt{5}, \sqrt{5})$ intersects the ellipse $\frac{x^2}{36}+\frac{y^2}{25}=1$ at $A$ and $B$ such that $(P A) \cdot(P B)$ is maximum. Then $5\left(P A^2+P B^2\right)$ is equal to :
Line $L_1$ passes through the point $(1,2,3)$ and is parallel to $z$-axis. Line $L_2$ passes through the point $(\lambda, 5,6)$ and is parallel to $y$-axis. Let for $\lambda=\lambda_1, \lambda_2, \lambda_2<\lambda_1$, the shortest distance between the two lines be 3 . Then the square of the distance of the point $\left(\lambda_1, \lambda_2, 7\right)$ from the line $L_1$ is
If $\sum\limits_{r=1}^9\left(\frac{r+3}{2^r}\right) \cdot{ }^9 C_r=\alpha\left(\frac{3}{2}\right)^9-\beta, \alpha, \beta \in \mathbb{N}$, then $(\alpha+\beta)^2$ is equal to
A line passes through the origin and makes equal angles with the positive coordinate axes. It intersects the lines $\mathrm{L}_1: 2 x+y+6=0$ and $\mathrm{L}_2: 4 x+2 y-p=0, p>0$, at the points A and B , respectively. If $A B=\frac{9}{\sqrt{2}}$ and the foot of the perpendicular from the point $A$ on the line $L_2$ is $M$, then $\frac{A M}{B M}$ is equal to
Let $A$ be a matrix of order $3 \times 3$ and $|A|=5$. If $|2 \operatorname{adj}(3 A \operatorname{adj}(2 A))|=2^\alpha \cdot 3^\beta \cdot 5^\gamma, \alpha, \beta, \gamma \in N$, then $\alpha+\beta+\gamma$ is equal to
All five letter words are made using all the letters A, B, C, D, E and arranged as in an English dictionary with serial numbers. Let the word at serial number $n$ be denoted by $\mathrm{W}_{\mathrm{n}}$. Let the probability $\mathrm{P}\left(\mathrm{W}_{\mathrm{n}}\right)$ of choosing the word $\mathrm{W}_{\mathrm{n}}$ satisfy $\mathrm{P}\left(\mathrm{W}_{\mathrm{n}}\right)=2 \mathrm{P}\left(\mathrm{W}_{\mathrm{n}-1}\right), \mathrm{n}>1$.
If $\mathrm{P}(\mathrm{CDBEA})=\frac{2^\alpha}{2^\beta-1}, \alpha, \beta \in \mathbb{N}$, then $\alpha+\beta$ is equal to :____________
Let $\vec{a}=\hat{i}+\hat{j}+\hat{k}, \vec{b}=3 \hat{i}+2 \hat{j}-\hat{k}, \vec{c}=\lambda \hat{j}+\mu \hat{k}$ and $\hat{d}$ be a unit vector such that $\vec{a} \times \hat{d}=\vec{b} \times \hat{d}$ and $\vec{c} \cdot \hat{d}=1$. If $\vec{c}$ is perpendicular to $\vec{a}$, then $|3 \lambda \hat{d}+\mu \vec{c}|^2$ is equal to________
Let the product of the focal distances of the point $\mathbf{P}(4,2 \sqrt{3})$ on the hyperbola $\mathrm{H}: \frac{x^2}{a^2}-\frac{y^2}{b^2}=1$ be 32 . Let the length of the conjugate axis of H be $p$ and the length of its latus rectum be $q$. Then $p^2+q^2$ is equal to__________
The area of the region bounded by the curve $y=\max \{|x|, x|x-2|\}$, the $x$-axis and the lines $x=-2$ and $x=4$ is equal to__________
If the number of seven-digit numbers, such that the sum of their digits is even, is $m \cdot n \cdot 10^n ; m, n \in\{1,2,3, \ldots, 9\}$, then $m+n$ is equal to__________
Physics
A gas is kept in a container having walls which are thermally non-conducting. Initially the gas has a volume of $800 \mathrm{~cm}^3$ and temperature $27^{\circ} \mathrm{C}$. The change in temperature when the gas is adiabatically compressed to $200 \mathrm{~cm}^3$ is:
(Take $\gamma=1.5 ; \gamma$ is the ratio of specific heats at constant pressure and at constant volume)
A person measures mass of 3 different particles as $435.42 \mathrm{~g}, 226.3 \mathrm{~g}$ and 0.125 g . According to the rules for arithmetic operations with significant figures, the addition of the masses of 3 particles will be.
The angle of projection of a particle is measured from the vertical axis as $\phi$ and the maximum height reached by the particle is $\mathrm{h}_{\mathrm{m}}$. Here $\mathrm{h}_{\mathrm{m}}$ as function of $\phi$ can be presented as
$$ \text { Match the LIST-I with LIST-II } $$
| List - I |
List - II |
||
|---|---|---|---|
| A. | $$ \text { Gravitational constant } $$ |
I. | $$ \left[\mathrm{LT}^{-2}\right] $$ |
| B. | $$ \text { Gravitational potential energy } $$ |
II. | $$ \left[\mathrm{L}^2 \mathrm{~T}^{-2}\right] $$ |
| C. | $$ \text { Gravitational potential } $$ |
III. | $$ \left[\mathrm{ML}^2 \mathrm{~T}^{-2}\right] $$ |
| D. | $$ \text { Acceleration due to gravity } $$ |
IV. | $$ \left[\mathrm{M}^{-1} \mathrm{~L}^3 \mathrm{~T}^{-2}\right] $$ |
Consider a completely full cylindrical water tank of height 1.6 m and of cross-sectional area $0.5 \mathrm{~m}^2$. It has a small hole in its side at a height 90 cm from the bottom. Assume, the crosssectional area of the hole to be negligibly small as compared to that of the water tank. If a load 50 kg is applied at the top surface of the water in the tank then the velocity of the water coming out at the instant when the hole is opened is:
$$ \left(\mathrm{g}=10 \mathrm{~m} / \mathrm{s}^2\right) $$
Consider following statements for refraction of light through prism, when angle of deviation is minimum.
A. The refracted ray inside prism becomes parallel to the base.
B. Larger angle prisms provide smaller angle of minimum deviation.
C. Angle of incidence and angle of emergence becomes equal.
D. There are always two sets of angle of incidence for which deviation will be same except at minimum deviation setting.
E. Angle of refraction becomes double of prism angle.
Choose the correct answer from the options given below :
A parallel plate capacitor is filled equally(half) with two dielectrics of dielectric constants $\varepsilon_1$ and $\varepsilon_2$, as shown in figures. The distance between the plates is $d$ and area of each plate is $A$. If capacitance in first configuration and second configuration are $\mathrm{C}_1$ and $\mathrm{C}_2$ respectively, then $\frac{C_1}{C_2}$ is:
First Configuration

Second Configuration

A force of 49 N acts tangentially at the highest point of a sphere (solid) of mass 20 kg , kept on a rough horizontal plane. If the sphere rolls without slipping, then the acceleration of the center of the sphere is


A piston of mass $M$ is hung from a massless spring whose restoring force law goes as $F=-k x^3$, where k is the spring constant of appropriate dimension. The piston separates the vertical chamber into two parts, where the bottom part is filled with ' $n$ ' moles of an ideal gas. An external work is done on the gas isothermally (at a constant temperature T) with the help of a heating filament (with negligible volume) mounted in lower part of the chamber, so that the piston goes up from a height $\mathrm{L}_0$ to $\mathrm{L}_1$, the total energy delivered by the filament is:(Assume spring to be in its natural length before heating)
$$ \text { Match the LIST-I with LIST-II } $$
| List - I |
List - II |
||
|---|---|---|---|
| A. | $$ { }_0^1 \mathrm{n}+{ }_{92}^{235} \mathrm{U} \rightarrow{ }_{54}^{140} \mathrm{Xe}+{ }_{38}^{94} \mathrm{Sr}+2{ }_0^1 \mathrm{n} $$ |
I. | $$ \text { Chemical reaction } $$ |
| B. | $$ 2 \mathrm{H}_2+\mathrm{O}_2 \rightarrow 2 \mathrm{H}_2 \mathrm{O} $$ |
II. | $$ \text { Fusion with +ve } \mathrm{Q} \text { value } $$ |
| C. | $$ { }_1^2 \mathrm{H}+{ }_1^2 \mathrm{H} \rightarrow{ }_2^3 \mathrm{He}+{ }_0^1 \mathrm{n} $$ |
III. | $$ \text { Fission } $$ |
| D. | $$ { }_1^1 \mathrm{H}+{ }_1^3 \mathrm{H} \rightarrow{ }_1^2 \mathrm{H}+{ }_1^2 \mathrm{H} $$ |
IV. | $$ \text { Fusion with -ve } Q \text { value } $$ |

Two blocks of masses $m$ and $M,(M>m)$, are placed on a frictionless table as shown in figure. A massless spring with spring constant k is attached with the lower block. If the system is slightly displaced and released, then ( $\mu=$ coefficient of friction between the two blocks)
A. The time period of small oscillation of the two blocks is $T=2 \pi \sqrt{\frac{(m+M)}{k}}$
B. The acceleration of the blocks is $a=-\frac{k x}{M+m}$ ( $x=$ displacement of the blocks from the mean position)
C. The magnitude of the frictional force on the upper block is $\frac{m \mu|x|}{M+m}$
D. The maximum amplitude of the upper block, if it does not slip, is $\frac{\mu(M+m) g}{k}$
E. Maximum frictional force can be $\mu(\mathrm{M}+\mathrm{m}) \mathrm{g}$.
Choose the correct answer from the options given below :
$$ \text {Which of the following curves possibly represent one-dimensional motion of a particle? } $$




Choose the correct answer from the options given below :
The electrostatic potential on the surface of uniformly charged spherical shell of radius $\mathrm{R}=10 \mathrm{~cm}$ is 120 V . The potential at the centre of shell, at a distance $\mathrm{r}=5 \mathrm{~cm}$ from centre, and at a distance $\mathrm{r}=15$ cm from the centre of the shell respectively, are:
$$ \text { Choose the correct logic circuit for the given truth table having inputs } A \text { and } B \text {. } $$
| Inputs | Output | ||
|---|---|---|---|
| A | B | Y | |
| 0 | 0 | 0 | |
| 0 | 1 | 0 | |
| 1 | 0 | 1 | |
| 1 | 1 | 1 | |
In the figure shown below, a resistance of $150.4 \Omega$ is connected in series to an ammeter A of resistance $240 \Omega$. A shunt resistance of $10 \Omega$ is connected in parallel with the ammeter. The reading of the ammeter is___________mA .

A loop ABCDA , carrying current $\mathrm{I}=12 \mathrm{~A}$, is placed in a plane, consists of two semi-circular segments of radius $R_1=6 \pi \mathrm{~m}$ and $\mathrm{R}_2=4 \pi \mathrm{~m}$. The magnitude of the resultant magnetic field at center O is $\mathrm{k} \times 10^{-7} \mathrm{~T}$. The value of k is_________.
( Given $\mu_0=4 \pi \times 10^{-7} \mathrm{Tm} \mathrm{A}^{-1}$ )
