JEE Main 2019 (Online) 12th January Evening Slot
Paper was held on
Sat, Jan 12, 2019 9:30 AM
Chemistry
1
The magnetic moment of an octahedral homoleptic Mn(II) complex is 5.9 BM. The suitable ligand for this complex is -
2
The major product of the following reaction is –
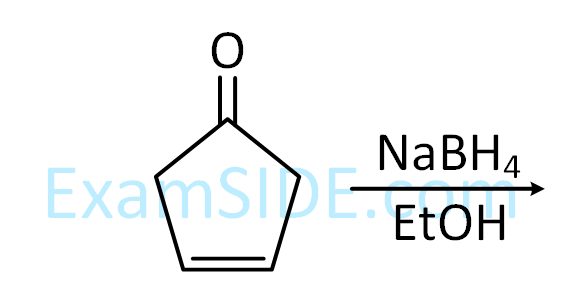

3
The correct statement (s) among I to III with respect to potassium ions that are abundant within the cell fluid
is /are
I. They activate many enzymes
II. They participate in the oxidation of glucose to produce ATP
III. Along with sodium ions they are responsible for the transmission of nerve signals
I. They activate many enzymes
II. They participate in the oxidation of glucose to produce ATP
III. Along with sodium ions they are responsible for the transmission of nerve signals
4
The major product of the following reaction is –
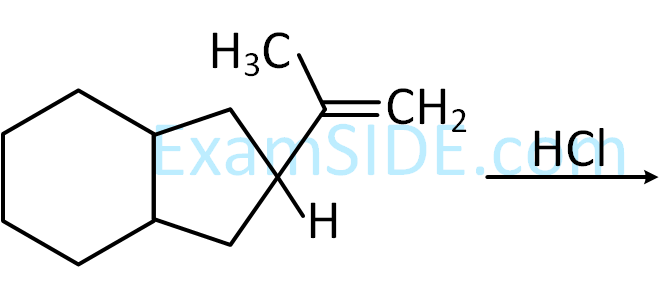
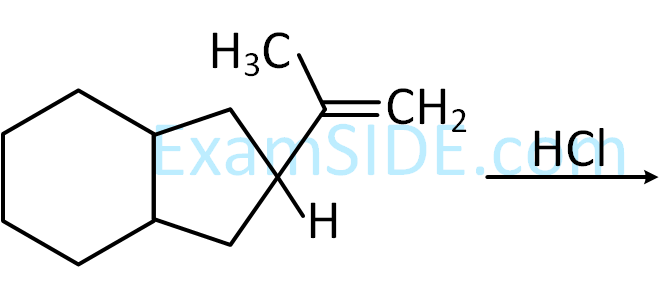
5
The correct order of atomic radii is :
6
The major product of the following reaction is :
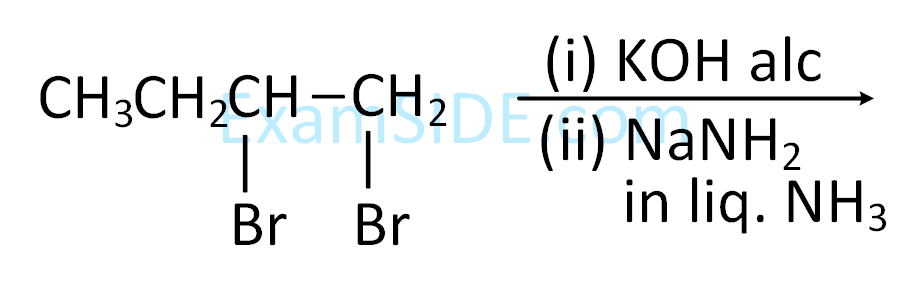
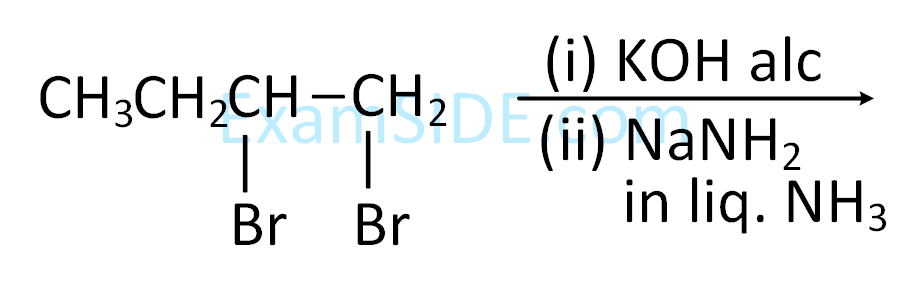
7
The element that shows greater ability to form p$$\pi $$-p$$\pi $$ multiple bonds, is :
8
The correct structure of histidine in a strongly acidic solution (pH = 2) is -
9
If Ksp of Ag2CO3 is 8 $$ \times $$ 10–12, the molar solubility of Ag2CO3 in 0.1 M AgNO3 is -
10
For a reaction consider the plot of $$\ell $$n k versus 1/T given in the figure. If the rate constant of this reaction at 400 K is 10–5 s–1, then the rate constant at 500 K is –
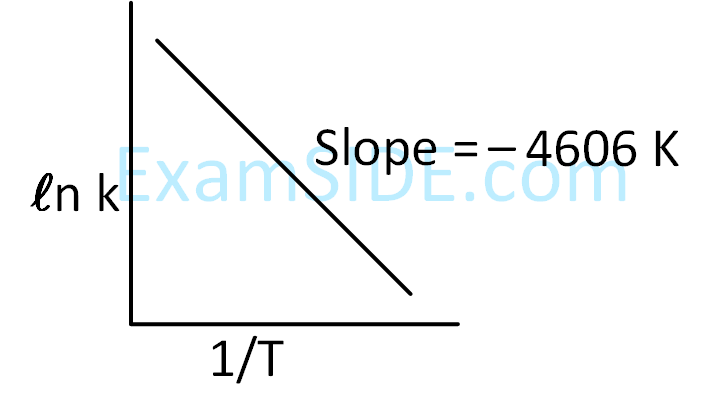
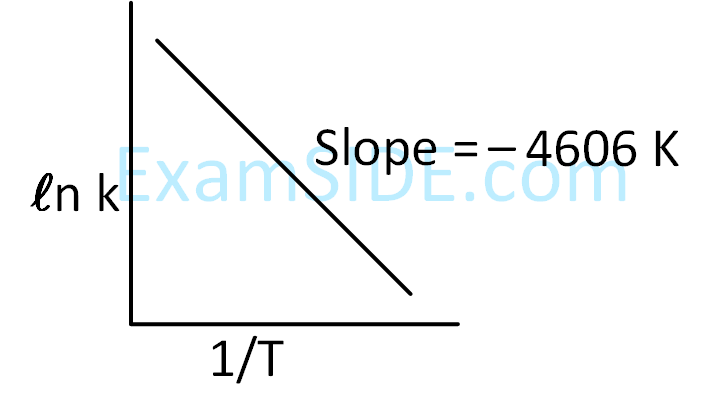
11
The increasing order of the reactivity of the following with LiAlH4 is
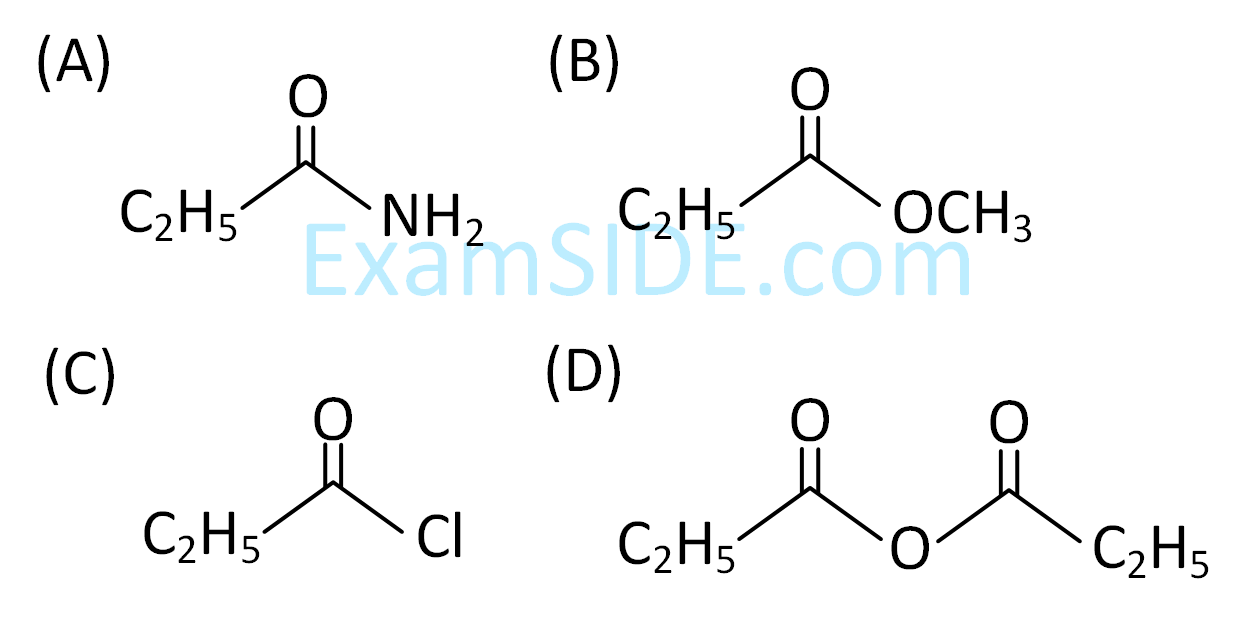

12
8 g of NaOH is dissolved in 18g of H2O. Mole fraction of NaOH in solution and molality (in mol kg–1) of the solution respectively are -
13
The upper stratosphere consisting of the ozone layer protects us from sun's radiation that falls in the wavelength region of -
14
Given
(i) C (graphite) + O2(g) $$ \to $$ CO2(g); $$\Delta $$rH$$^\Theta $$ = x kJ mol$$-$$1
(ii) C(graphite) + $${1 \over 2}$$O2(g) $$ \to $$ CO(g); $$\Delta $$rH$$^\Theta $$ = y kJ mol$$-$$1
(iii) CO(g) + $${1 \over 2}$$ O2(g) $$ \to $$ CO2(g); $$\Delta $$rH$$^\Theta $$ = z kJ mol$$-$$1
Based on the above thermochemical equations, find out which one of the following algebraic relationships is correct?
(i) C (graphite) + O2(g) $$ \to $$ CO2(g); $$\Delta $$rH$$^\Theta $$ = x kJ mol$$-$$1
(ii) C(graphite) + $${1 \over 2}$$O2(g) $$ \to $$ CO(g); $$\Delta $$rH$$^\Theta $$ = y kJ mol$$-$$1
(iii) CO(g) + $${1 \over 2}$$ O2(g) $$ \to $$ CO2(g); $$\Delta $$rH$$^\Theta $$ = z kJ mol$$-$$1
Based on the above thermochemical equations, find out which one of the following algebraic relationships is correct?
15
If the de Broglie wavelength of the electron in nth Bohr orbit in a hydrogenic atom is equal to 1.5 $$\pi $$a0 (a0 is Bohr radius), then the value of n/z is -
16
The aldehydes which will not form Grignard product with one equivalent Grignard reagents are -
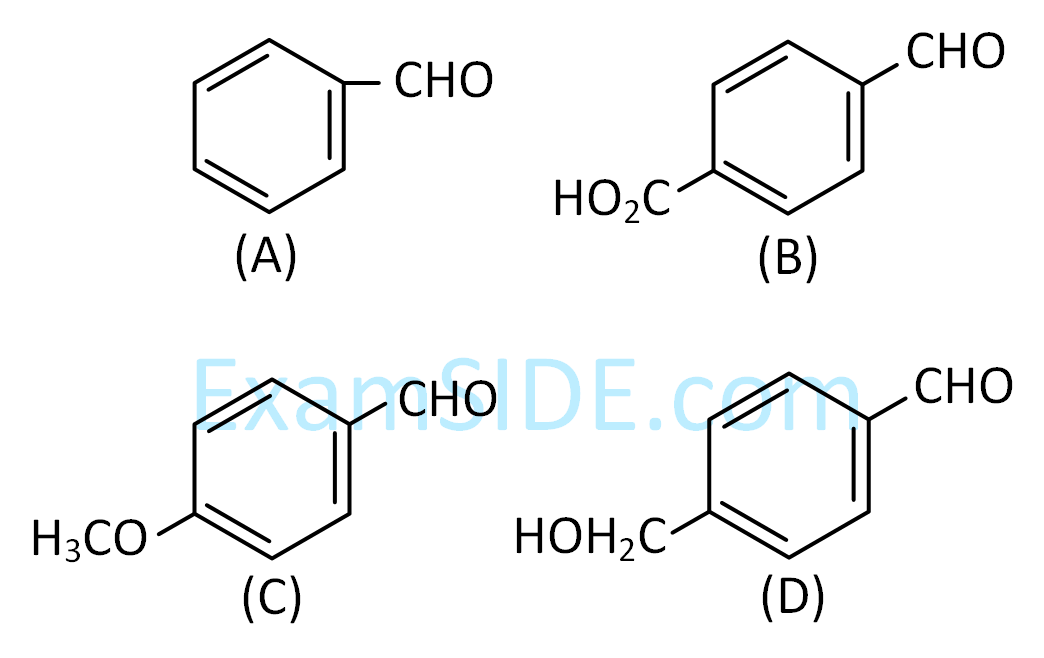

17
The element that does NOT show catenation is :
18
The combination of plots which does not represent isothermal expansion of an ideal gas is –
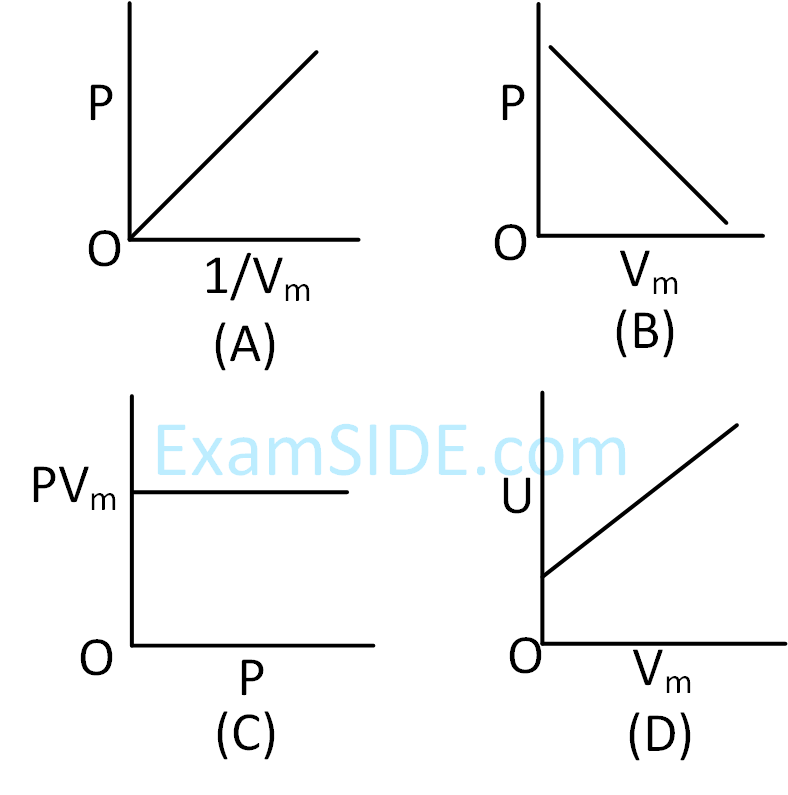

19
Molecules of benzoic acid (C6H5COOH) dimerise in benzene. 'w' g of the acid dissolved in 30 g of benzene shows a depression in freezing point equal to 2K. If the percentage association of the acid to form dimmer in the solution is 80, then w is – (Its given that Kf = 5 K kg mol–1, Molar mass of benzoic acid = 122 g mol–1)
20
The major product of the following reaction is –
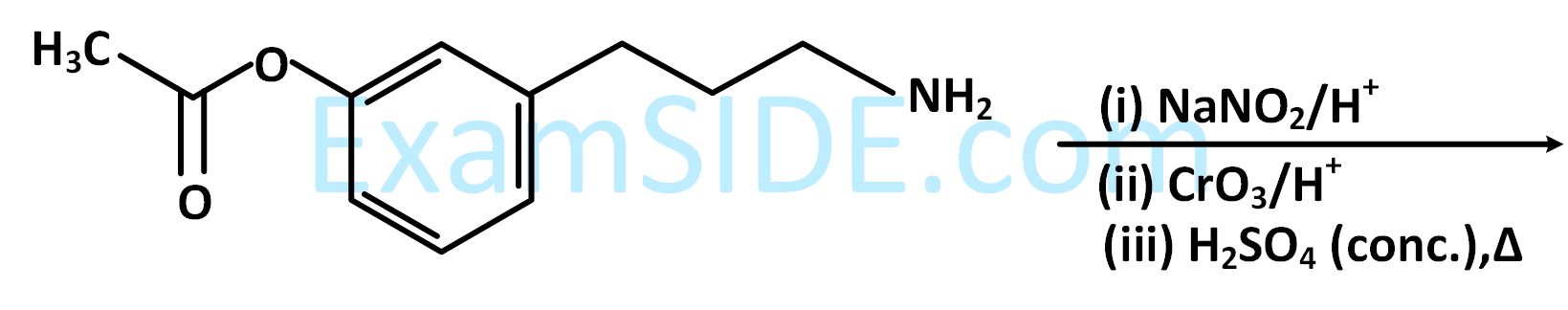

21
The major product in the following conversion is –
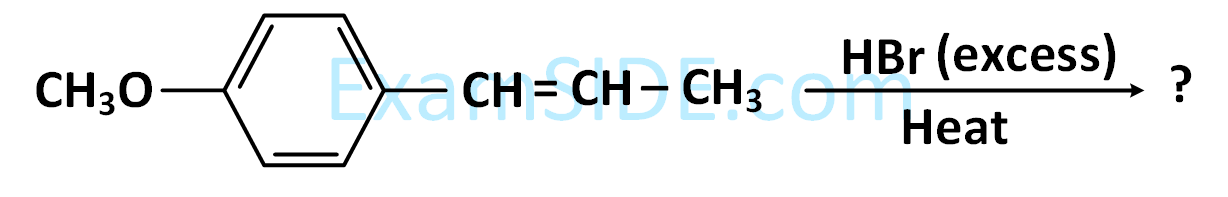
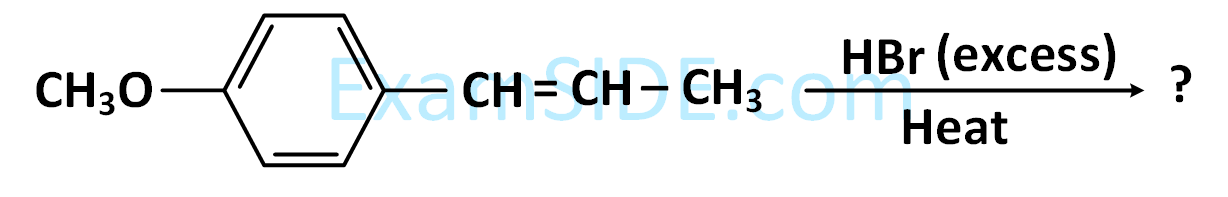
22
The major product of the following reaction is –
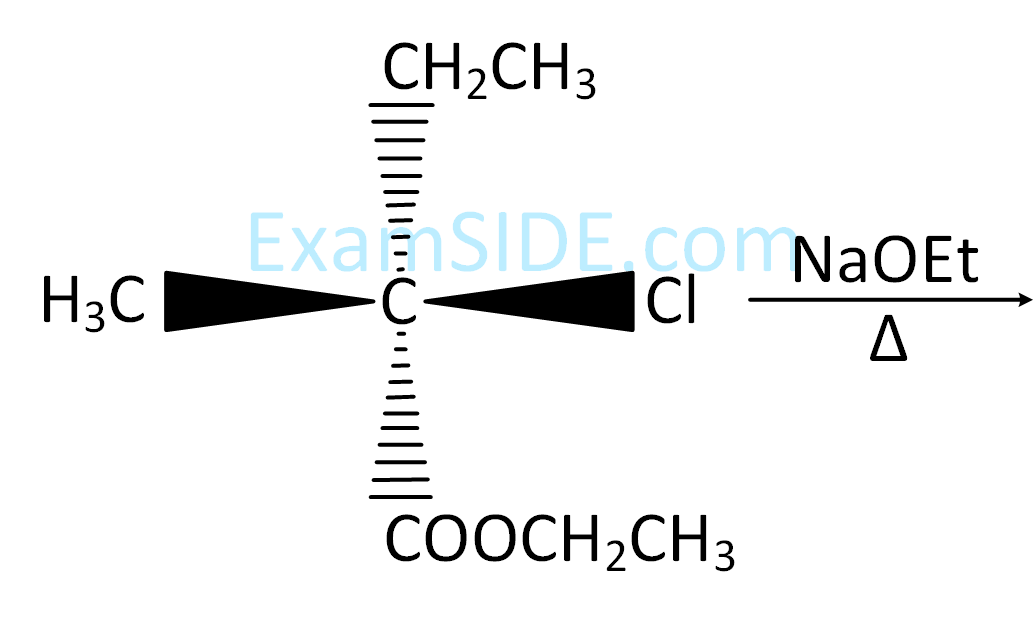

23
$$ \wedge _m^ \circ $$ for NaCl, HCl and NaA are 126.4, 425.9 and 100.5 S cm2 mol–1, respectively. If the conductivity of 0.001 M HA is-
5 $$ \times $$ 10–5 S cm–1, degree of dissociation of HA is -
5 $$ \times $$ 10–5 S cm–1, degree of dissociation of HA is -
Mathematics
1
The integral $$\int\limits_1^e {\left\{ {{{\left( {{x \over e}} \right)}^{2x}} - {{\left( {{e \over x}} \right)}^x}} \right\}} \,$$ loge x dx is equal to :
2
In a game, a man wins Rs. 100 if he gets 5 or 6 on a throw of a fair die and loses Rs. 50 for getting any other number on the die. If he decides to throw the die either till he gets a five or a six or to a maximum of three throws, then his expected gain/loss (in rupees) is :
3
If a curve passes through the point (1, –2) and has slope of the tangent at any point (x, y) on it as $${{{x^2} - 2y} \over x}$$, then the curve also passes through the point :
4
There are m men and two women participating in a chess tournament. Each participant plays two games with every other participant. If the number of games played by the men between themselves exceeds the number of games played between the men and the women by 84, then the value of m is :
5
$$\mathop {\lim }\limits_{x \to {1^ - }} {{\sqrt \pi - \sqrt {2{{\sin }^{ - 1}}x} } \over {\sqrt {1 - x} }}$$ is equal to :
6
If a circle of radius R passes through the origin O and intersects the coordinates axes at A and B, then the
locus of the foot of perpendicular from O on AB is :
7
If nC4, nC5 and nC6 are in A.P., then n can be :
8
The total number of irrational terms in the binomial expansion of (71/5 – 31/10)60 is :
9
The number of integral values of m for which the quadratic expression, (1 + 2m)x2 – 2(1 + 3m)x + 4(1 + m), x $$ \in $$ R, is always positive, is :
10
The set of all values of $$\lambda $$ for which the system of linear equations
x – 2y – 2z = $$\lambda $$x
x + 2y + z = $$\lambda $$y
– x – y = $$\lambda $$z
has a non-trivial solutions :
x – 2y – 2z = $$\lambda $$x
x + 2y + z = $$\lambda $$y
– x – y = $$\lambda $$z
has a non-trivial solutions :
11
The mean and the variance of five observations are 4 and 5.20, respectively. If three of the observations are
3, 4 and 4 ; then the absolute value of the difference of the other two observations, is :
12
If a straight line passing through the point P(–3, 4) is such that its intercepted portion between the coordinate axes is bisected at P, then its equation is :
13
Let f be a differentiable function such that f(1) = 2 and f '(x) = f(x) for all x $$ \in $$ R R. If h(x) = f(f(x)), then h'(1) is equal to :
14
Let z1 and z2 be two complex numbers satisfying | z1 | = 9 and | z2 – 3 – 4i | = 4. Then the minimum value of
| z1 – z2 | is :
15
If A = $$\left[ {\matrix{
1 & {\sin \theta } & 1 \cr
{ - \sin \theta } & 1 & {\sin \theta } \cr
{ - 1} & { - \sin \theta } & 1 \cr
} } \right]$$;
then for all $$\theta $$ $$ \in $$ $$\left( {{{3\pi } \over 4},{{5\pi } \over 4}} \right)$$, det (A) lies in the interval :
then for all $$\theta $$ $$ \in $$ $$\left( {{{3\pi } \over 4},{{5\pi } \over 4}} \right)$$, det (A) lies in the interval :
16
In a class of 60 students, 40 opted for NCC, 30 opted for NSS and 20 opted for both NCC and NSS. If one of these students is selected at random, then the probability that the students selected has opted neither for NCC
nor for NSS is :
17
Let Z be the set of integers.
If A = {x $$ \in $$ Z : 2(x + 2) (x2 $$-$$ 5x + 6) = 1} and
B = {x $$ \in $$ Z : $$-$$ 3 < 2x $$-$$ 1 < 9},
then the number of subsets of the set A $$ \times $$ B, is
If A = {x $$ \in $$ Z : 2(x + 2) (x2 $$-$$ 5x + 6) = 1} and
B = {x $$ \in $$ Z : $$-$$ 3 < 2x $$-$$ 1 < 9},
then the number of subsets of the set A $$ \times $$ B, is
18
If the function f given by f(x) = x3 – 3(a – 2)x2 + 3ax + 7, for some a$$ \in $$R is increasing in (0, 1] and decreasing in [1, 5), then a root of the equation, $${{f\left( x \right) - 14} \over {{{\left( {x - 1} \right)}^2}}} = 0\left( {x \ne 1} \right)$$ is :
19
The integral $$\int {{{3{x^{13}} + 2{x^{11}}} \over {{{\left( {2{x^4} + 3{x^2} + 1} \right)}^4}}}} \,dx$$ is equal to : (where C is a constant of integration)
20
Let S and S' be the foci of an ellipse and B be any one of the extremities of its minor axis. If $$\Delta $$S'BS is a right angled triangle with right angle at B and area ($$\Delta $$S'BS) = 8 sq. units, then the length of a latus rectum of the ellipse is :
21
If sin4$$\alpha $$ + 4 cos4$$\beta $$ + 2 = 4$$\sqrt 2 $$ sin $$\alpha $$ cos $$\beta $$; $$\alpha $$, $$\beta $$ $$ \in $$ [0, $$\pi $$],
then cos($$\alpha $$ + $$\beta $$) $$-$$ cos($$\alpha $$ $$-$$ $$\beta $$) is equal to :
then cos($$\alpha $$ + $$\beta $$) $$-$$ cos($$\alpha $$ $$-$$ $$\beta $$) is equal to :
Physics
1
A load of mass M kg is suspended from a steel wire of length 2m and radius 1.0 mm in Searle's apparatus
experiment. The increase in length produced in the wire is 4.0 mm. Now the load is fully immersed in a liquid of relative density 2. The relative density of the material of load is 8.
The new value of increase in length of the steel wire is:
The new value of increase in length of the steel wire is:
2
A long cylindrical vessel is half filled with a liquid. When the vessel is rotated about its own vertical axis, the liquid rises up near the wall. If the radius of vessel is 5 cm and its rotational speed is 2 rotations per second, then the difference in the heights between the centre and the sides, in cm, will be :
3
The charge on a capacitor plate in a circuit, as a function of time, is shown in the figure :
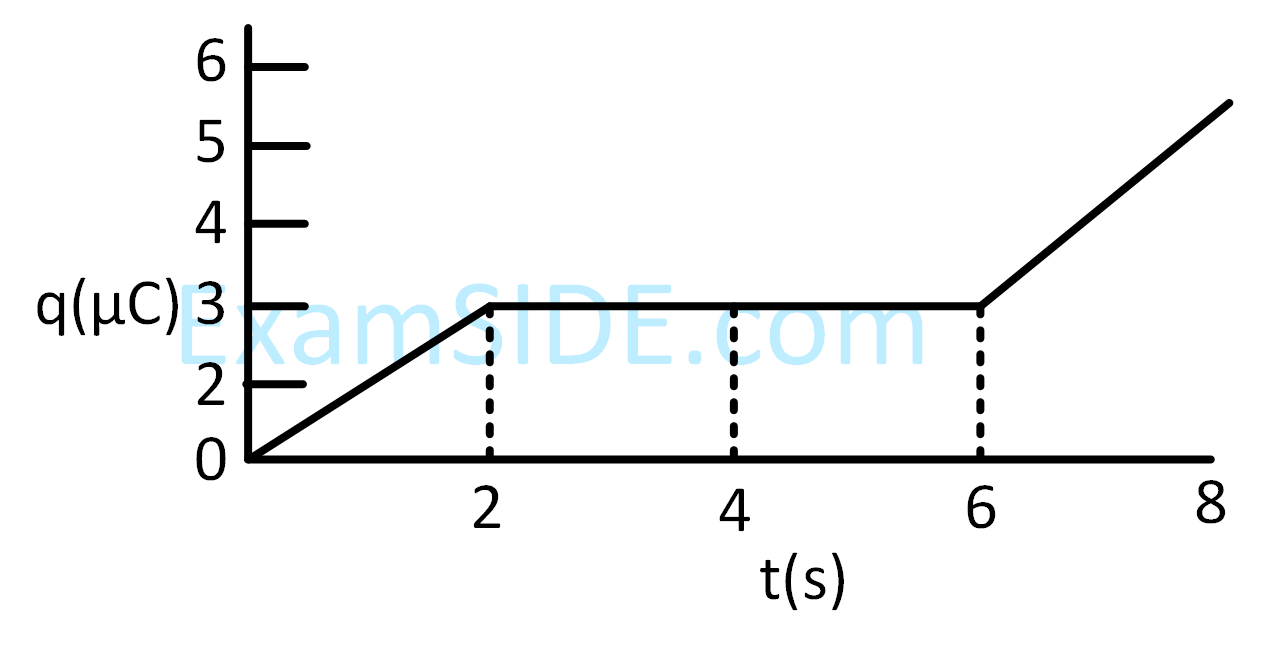
When is the value of current at t = 4 s ?

When is the value of current at t = 4 s ?
4
Two particles A, B are moving on two concentric circles of radii R1 and R2 with equal angular speed $$\omega $$. At t = 0, their positions and direction of motion are shown in the figure. :
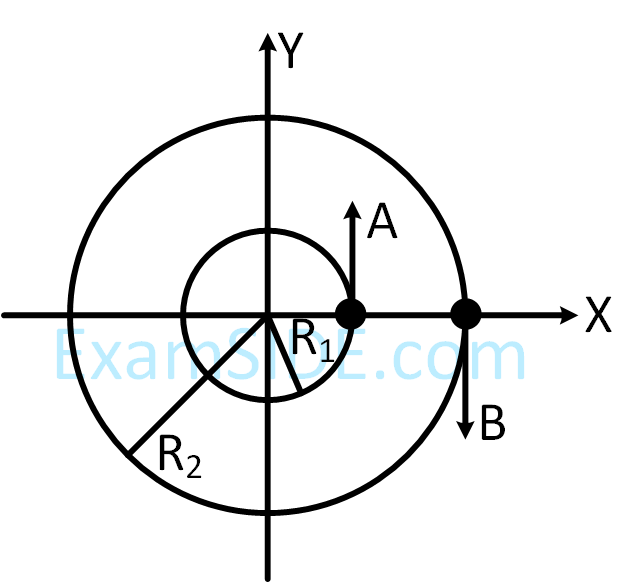
The relative velocity $${\overrightarrow V _A} - {\overrightarrow V _B}$$ at t = $${\pi \over {2\omega }}$$ is given by :

The relative velocity $${\overrightarrow V _A} - {\overrightarrow V _B}$$ at t = $${\pi \over {2\omega }}$$ is given by :
5
The mean intensity of radiation on the surface of the Sun is about 108 W/m2 . The rms value of the corresponding magnetic field is closet to :
6
A 10 m long horizontal wire extends from North East to South West. It is falling with a speed of 5.0 ms–1, at right angles to the horizontal component of the earth's magnetic field of 0.3 $$ \times $$ 10–4 Wb/m2. The value of the induced emf in wire is :
7
An alpha-particle of mass m suffers 1-dimensional elastic collision with a nucleus at rest of unknown mass. It is scattered directly backwards losing, 64% of its initial kinetic energy. The mass of the nucleus is
8
A resonance tube is old and has jagged end. It is still used in the laboratory to determine velocity of sound in air. A tuning fork of frequency 512 Hz produces first resonance when the tube is filled with water to a mark 11 cm below a reference mark, near the open end of the tube. The experiment is repeated with another fork of frequency 256 Hz which produces first resonance when water reaches a mark 27 cm below the reference mark. The velocity of sound in air, obtained in the experiment, is close to :
9
A vertical closed cylinder is separated into two parts by a frictionless piston of mass m and of negligible thickness. The piston is free to move along the length of the cylinder. The length of the cylinder above the piston is $$\ell $$1, and that below the piston is $$\ell $$2, such that $$\ell $$1 > $$\ell $$2. Each part of the cylinder contains n moles of an ideal gas at equal temperature T. If the piston is stationary, its mass, m, will be given by :
(R is universal gas constant and g is the acceleration due to gravity)
(R is universal gas constant and g is the acceleration due to gravity)
10
A parallel plate capacitor with plates of area 1 m2 each, are at a separation of 0.1 m. If the electric field between the plates is 100 N/C, the magnitude of charge on each plate is :
(Take $$\varepsilon $$0 = 8.85 $$ \times $$ 10$$-$$12 $${{{C^2}} \over {N - {m^2}}}$$)
(Take $$\varepsilon $$0 = 8.85 $$ \times $$ 10$$-$$12 $${{{C^2}} \over {N - {m^2}}}$$)
11
When a certain photosensitive surface is illuminated with monochromatic light of frequency v, the stopping
potential for the current is –V0/2. When the surface is illuminated by monochromatic light of frequency v/2, the stopping potential is – V0. The threshold frequency for photoelectric emission is :
12
A paramagnetic material has 1028 atoms/m3. Its magnetic susceptibility at temperature 350 K is 2.8 $$ \times $$ 10–4. Its susceptibility at 300 K is :
13
In a Frank-Hertz experiment, an electron of energy 5.6 eV passes through mercury vapour and emerges with an energy 0.7 eV. The minimum wavelength of photons emitted by mercury atoms is close to :
14
The moment of inertia of a solid sphere, about an axis parallel to its diameter and at a distance of x from it, is 'I(x)'. Which one of the graphs represents the variation of I(x) with x correctly ?
15
In the given circuit diagram, the currents, I1 = – 0.3 A, I4 = 0.8 A and I5 = 0.4 A, are flowing as shown. The currents I2, I3 and I6, respectively, are :
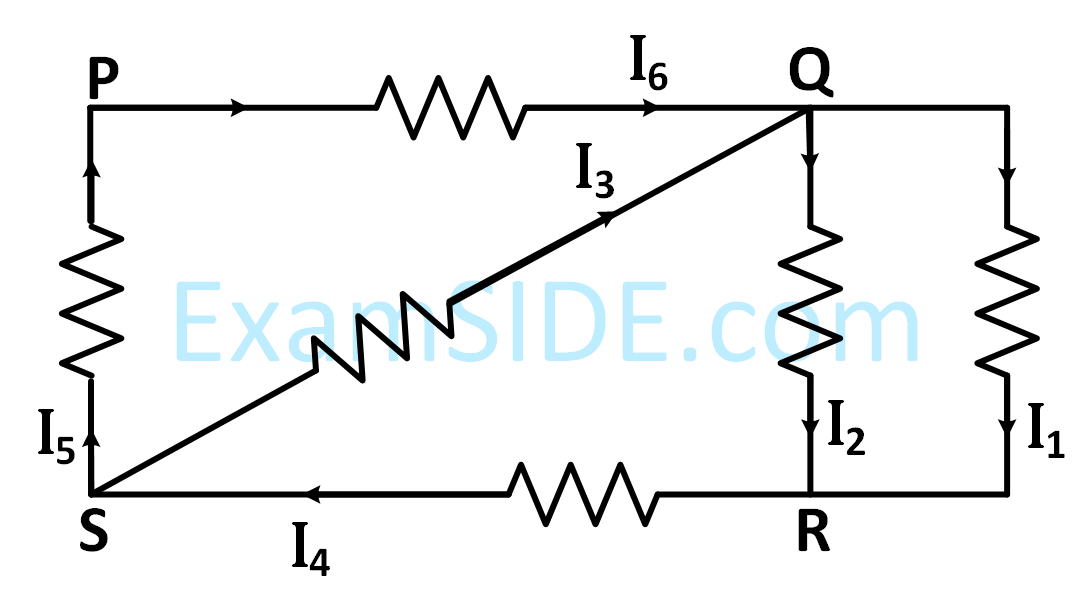

16
A plano-convex lens (focal length f2, refractive index $$\mu $$2, radius of curvature R) fits exactly into a plano-concave lens (focal length f1, refractive index $$\mu $$1, radius of curvature R). Their plane surfaces are parallel to each other. Then, the focal length of the combination will be :
17
A block kept on a rough inclined plane, as shown in the figure, remains at rest upto a maximum force 2 N down the inclined plane. The maximum external force up the inclined plane that does not move the block is 10 N. The coefficient of static friction between the block and the plane is : [Take g = 10 m/s2]


18
Let $$\ell $$, r, C and V represent inductance, resistance, capacitance and voltage, respectively. The dimension of $${\ell \over {rCV}}$$ in SI units will be :
19
A simple harmonic motion is represented by :
y = 5 (sin 3 $$\pi $$ t + $$\sqrt 3 $$ cos 3 $$\pi $$t) cm
The amplitude and time period of the motion are :
y = 5 (sin 3 $$\pi $$ t + $$\sqrt 3 $$ cos 3 $$\pi $$t) cm
The amplitude and time period of the motion are :
20
Two satellites, A and B, have masses m and 2m respectively. A is in a circular orbit of radius R, and B is in a circular orbit of radius 2R around the earth. The ratio of their kinetic energies, TA/TB, is ;
21
A galvanometer, whose resistance is 50 ohm, has 25 divisions in it. When a current of 4 $$ \times $$ 10–4 A passes through it, its needle ( pointer) deflects by one division. To use this galvanometer as a voltmeter of range 2.5 V, it should be connected to a resistance of :
22
A particle of mass 20 g is released with an initial velocity 5 m/s along the curve from the point A, as shown in the figure. The point A is at height h from point B. The particle slides along the frictionless surface. when the particle reaches point b, its angular momentum about O will be :
(Take g = 10 m/s2)
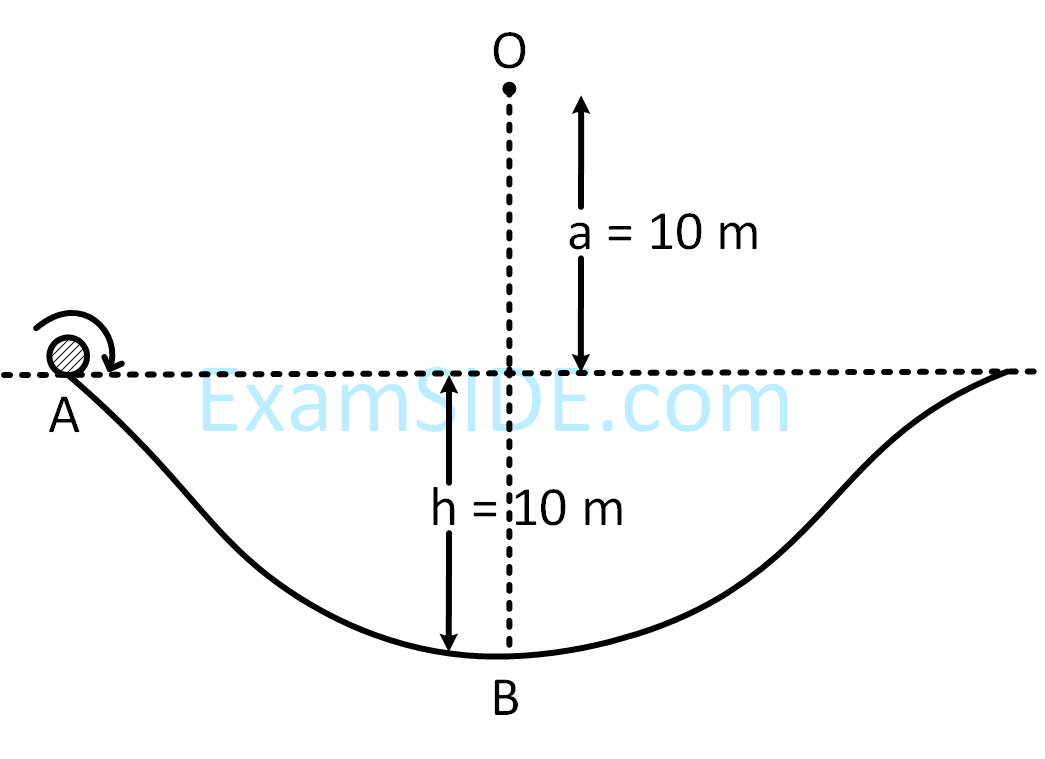
(Take g = 10 m/s2)

23
An ideal gas is enclosed in a cylinder at pressure of 2 atm and temperature 300 K. The mean time between two successive collisions is 6 $$ \times $$ 10–8 s. If the pressure is doubled and temperature is increased to 500 K, the mean time between two successive collisions will be close to
24
In the circuit shown, find C if the effective capacitance of the whole circuit is to be 0.5 $$\mu $$F. All values in the circuit are in $$\mu $$F.


25
Formation of real image using a biconvex lens is shown below :

If the whole set up is immersed in water without disturbing the object and the screen positions, what will one observe on the screen ?

If the whole set up is immersed in water without disturbing the object and the screen positions, what will one observe on the screen ?
26
In the above circuit, C = $${{\sqrt 3 } \over 2}$$$$\mu $$F, R2 = 20 $$\Omega $$, L = $${{\sqrt 3 } \over {10}}$$ H and R1 = 10 $$\Omega $$. Current in L-R1 path is I1 and in C-R2 path it is I2 . The voltage of A.C. source is given by, V = 200 $${\sqrt 2 }$$ sin (100 t) volts . The phase difference between I1 and I2 is :
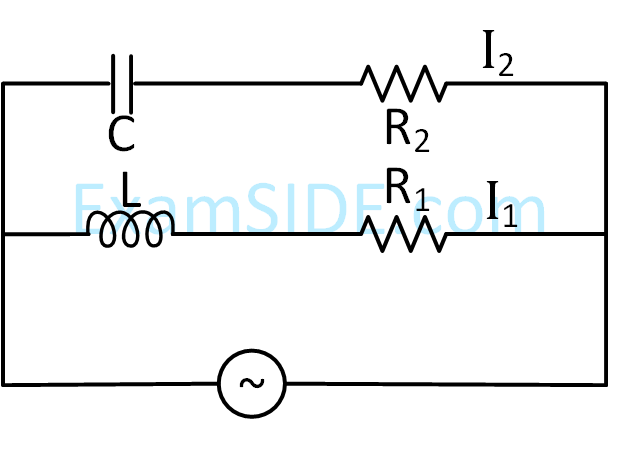

27
A soap bubble, blown by a mechanical pump at the mouth of a tube, increases in volume, with time, at a constant rate. The graph that correctly depicts the time dependence of pressure inside the bubble is given by :