Chemistry
Assertion A:
 can be easily reduced using $\mathrm{Zn}-\mathrm{Hg} / \mathrm{HCl}$ to
can be easily reduced using $\mathrm{Zn}-\mathrm{Hg} / \mathrm{HCl}$ to 
Reason $\mathrm{R}: \mathrm{Zn}-\mathrm{Hg} / \mathrm{HCl}$ is used to reduce carbonyl group to $-\mathrm{CH}_{2}-$ group.
In the light of the above statements, choose the correct answer from the options given below:
Match List I with List II:
| List I (Complexes) | List II (Hybridisation) | ||
|---|---|---|---|
| A. | $\left[\mathrm{Ni}(\mathrm{CO})_{4}\right]$ | I. | $\mathrm{sp}^{3}$ |
| B. | $\left[\mathrm{Cu}\left(\mathrm{NH}_{3}\right)_{4}\right]^{2+}$ | II. | dsp$^{2}$ |
| C. | $\left[\mathrm{Fe}\left(\mathrm{NH}_{3}\right)_{6}\right]^{2+}$ | III. | $\mathrm{sp}^{3}\mathrm{d}^{2}$ |
| D. | $\left[\mathrm{Fe}\left(\mathrm{H}_{2} \mathrm{O}\right)_{6}\right]^{2+}$ | IV. | $\mathrm{d}^{2} \mathrm{sp}^{3}$ |
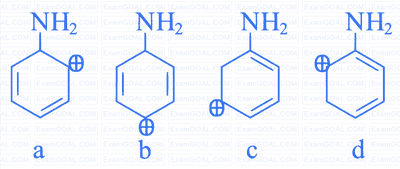
Decreasing order towards SN 1 reaction for the following compounds is:


In the above conversion of compound $(\mathrm{X})$ to product $(\mathrm{Y})$, the sequence of reagents to be used will be:
Match List I with List II:
| List I (Mixture) | List II (Separation Technique) | ||
|---|---|---|---|
| A. | $\mathrm{CHCl}_3+\mathrm{C}_6 \mathrm{H}_5 \mathrm{NH}_2$ | I. | Steam distillation |
| B. | $\mathrm{C}_6 \mathrm{H}_{14}+\mathrm{C}_5 \mathrm{H}_{12}$ | II. | Differential extraction |
| C. | $\mathrm{C}_6 \mathrm{H}_5 \mathrm{NH}_2+\mathrm{H}_2 \mathrm{O}$ | III. | Distillation |
| D. | $\text { Organic compound in } \mathrm{H}_2 \mathrm{O}$ | IV. | Fractional distillation |
A. $\mathrm{O}$
B. $\mathrm{S}$
C. Se
D. $\mathrm{Te}$
Choose the correct from the options given below:

$1 \mathrm{~L}$ Solution $(\mathrm{X})+\mathrm{AgNO}_{3}$ solution (excess) $\longrightarrow \mathrm{Y}$
$1 \mathrm{~L}$ Solution $(\mathrm{X})+\mathrm{BaCl}_{2}$ solution (excess) $\longrightarrow \mathrm{Z}$
The number of moles of $\mathrm{Y}$ and $\mathrm{Z}$ respectively are
$$ \Psi_{2 \mathrm{~s}}=\frac{1}{2 \sqrt{2 \pi}}\left(\frac{1}{a_0}\right)^{1 / 2}\left(2-\frac{r}{a_0}\right) e^{-r / 2 a_0} $$
At $r=r_0$, radial node is formed. Thus, $r_0$ in terms of $a_0$
The strength of 50 volume solution of hydrogen peroxide is ______ $\mathrm{g} / \mathrm{L}$ (Nearest integer).
Given:Molar mass of $\mathrm{H}_{2} \mathrm{O}_{2}$ is $34 \mathrm{~g} \mathrm{~mol}^{-1}$
Molar volume of gas at $\mathrm{STP}=22.7 \mathrm{~L}$
Number of compounds from the following which will not dissolve in cold $\mathrm{NaHCO}_{3}$ and $\mathrm{NaOH}$ solutions but will dissolve in hot $\mathrm{NaOH}$ solution is ________.

Given: $\ln 10=2.3 ; \log 2=0.3$
Given $\mathrm{C_V}=20 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}$
$\mathrm{X}\left|\mathrm{X}^{2+}(0.001 \mathrm{M}) \| \mathrm{Y}^{2+}(0.01 \mathrm{M})\right| \mathrm{Y}$ is _______ $\times 10^{-2} \mathrm{~V}$ (Nearest integer)
Given: $\mathrm{E}^{0} _ {\mathrm{X}^{2+} \mid \mathrm{X}}=-2.36 \mathrm{~V}$
$\mathrm{E}_{\mathrm{Y}^{2+} \mid \mathrm{Y}}^{0}=+0.36 \mathrm{~V}$
$\frac{2.303 \mathrm{RT}}{\mathrm{F}}=0.06 \mathrm{~V}$
$2 \mathrm{SO}_{2}(g)+\mathrm{O}_{2}(g) \rightleftharpoons 2 \mathrm{SO}_{3}(g), \Delta H=-190 \mathrm{~kJ}$
The number of factors which will increase the yield of $\mathrm{SO}_{3}$ at equilibrium from the following is _______.
A. Increasing temperature
B. Increasing pressure
C. Adding more $\mathrm{SO}_{2}$
D. Adding more $\mathrm{O}_{2}$
E. Addition of catalyst
Given $\mathrm{K}_{f}=1.8 \mathrm{~K} \mathrm{~kg} \mathrm{~mol}^{-1}$
Mathematics
Then $\tan ^{-1}\left(\frac{1}{1+a_{1} a_{2}}\right)+\tan ^{-1}\left(\frac{1}{1+a_{2} a_{3}}\right)+\ldots . .+\tan ^{-1}\left(\frac{1}{1+a_{2021} a_{2022}}\right)$ is equal to :
$$ \begin{aligned} & x-y+z=5 \\ & 2 x+2 y+\alpha z=8 \\ & 3 x-y+4 z=\beta \end{aligned} $$
has infinitely many solutions. Then $\alpha$ and $\beta$ are the roots of :
$f(x)=\left\{\begin{array}{cc}\frac{x}{|x|}, & x \neq 0 \\ 1, & x=0\end{array}\right.$
$g(x)=\left\{\begin{array}{cc}\frac{\sin (x+1)}{(x+1)}, & x \neq-1 \\ 1, & x=-1\end{array}\right.$
and $h(x)=2[x]-f(x)$, where $[x]$ is the greatest integer $\leq x$. Then the
value of $\lim\limits_{x \rightarrow 1} g(h(x-1))$ is :
and $g(x)=\frac{x^3}{3}+a x+b x^2, a \neq 2 b$
have a common extreme point, then $a+2 b+7$ is equal to :
$\frac{d y}{d x}=-\left(\frac{x^2+3 y^2}{3 x^2+y^2}\right), y(1)=0$ is :
seven digits 1, 2, 2, 2, 3, 3, 5 is ____________.
$\left\{(x, y): y \geq x^2, y \geq(1-x)^2, y \leq 2 x(1-x)\right\}$.
Then $540 \mathrm{~A}$ is equal to :
have a common real root is $\frac{3}{\sqrt{2 \beta}}$ then $\beta$ is equal to ___________.
Physics
Match List I with List II:
| List I | List II | ||
|---|---|---|---|
| A. | Torque | I. | $\mathrm{kg} \mathrm{m}^{-1} \mathrm{~s}^{-2}$ |
| B. | Energy density | II. | $\mathrm{kg} \,\mathrm{ms}^{-1}$ |
| C. | Pressure gradient | III. | $\mathrm{kg}\, \mathrm{m}^{-2} \mathrm{~s}^{-2}$ |
| D. | Impulse | IV. | $\mathrm{kg} \,\mathrm{m}^{2} \mathrm{~s}^{-2}$ |
Choose the correct answer from the options given below:

A thin prism $P_1$ with an angle $6^{\circ}$ and made of glass of refractive index $1.54$ is combined with another prism $P_2$ made from glass of refractive index $1.72$ to produce dispersion without average deviation. The angle of prism $P_2$ is
$(\mathrm{I}: r < a, \mathrm{II}: a < r < b$, III: $r>b$ )
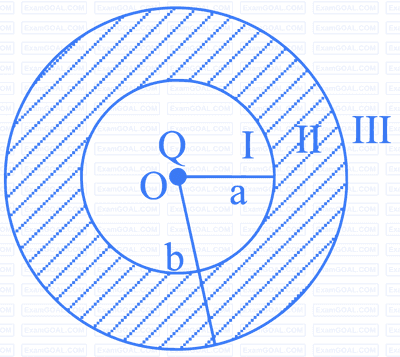
As shown in the figure, a current of $2 \mathrm{~A}$ flowing in an equilateral triangle of side $4 \sqrt{3} \mathrm{~cm}$. The magnetic field at the centroid $\mathrm{O}$ of the triangle is

(Neglect the effect of earth's magnetic field)
The output $Y$ for the inputs $A$ and $B$ of circuit is given by

Truth table of the shown circuit is:
A current carrying rectangular loop PQRS is made of uniform wire. The length $P R=Q S=5 \mathrm{~cm}$ and $P Q=R S=100 \mathrm{~cm}$. If ammeter current reading changes from I to $2 I$, the ratio of magnetic forces per unit length on the wire $P Q$ due to wire $R S$ in the two cases respectively $\left(f_{P Q}^I: f_{P Q}^{2 t}\right)$ is:

For a simple harmonic motion in a mass spring system shown, the surface is frictionless. When the mass of the block is $1 \mathrm{~kg}$, the angular frequency is $\omega_{1}$. When the mass block is $2 \mathrm{~kg}$ the angular frequency is $\omega_{2}$. The ratio $\omega_{2} / \omega_{1}$ is

$\left(\right.$ Take $\left.\pi=\frac{22}{7}\right)$
In a Young's double slit experiment, the intensities at two points, for the path differences $\frac{\lambda}{4}$ and $\frac{\lambda}{3}$ ( $\lambda$ being the wavelength of light used) are $I_{1}$ and $I_{2}$ respectively. If $I_{0}$ denotes the intensity produced by each one of the individual slits, then $\frac{I_{1}+I_{2}}{I_{0}}=$ __________.
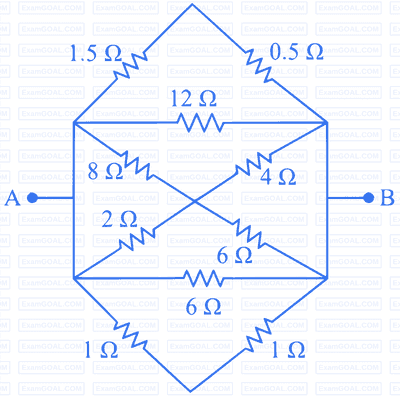
If the potential difference between $\mathrm{B}$ and $\mathrm{D}$ is zero, the value of $x$ is $\frac{1}{n} \Omega$. The value of $n$ is __________.

A uniform disc of mass $0.5 \mathrm{~kg}$ and radius $r$ is projected with velocity $18 \mathrm{~m} / \mathrm{s}$ at $\mathrm{t}=0$ s on a rough horizontal surface. It starts off with a purely sliding motion at $\mathrm{t}=0 \mathrm{~s}$. After $2 \mathrm{~s}$ it acquires a purely rolling motion (see figure). The total kinetic energy of the disc after $2 \mathrm{~s}$ will be __________ $\mathrm{J}$ (given, coefficient of friction is $0.3$ and $g=10 \mathrm{~m} / \mathrm{s}^{2}$ ).

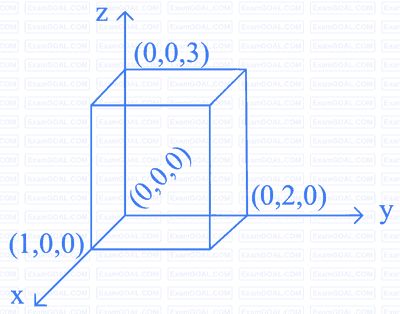
The value of $n$ is _________ (if dimension of cuboid is $1 \times 2 \times 3 \mathrm{~m}^{3}$ )