Chemistry
The product B formed in the following reaction sequence is:

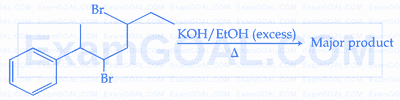
The major product of the following reaction is:
Given below are two statements:
Statement I: and
and  are isomeric compounds.
are isomeric compounds.
Statement II:  and
and  are functional group isomers.
are functional group isomers.
In the light of the above statements, choose the correct answer from the options given below:
Match List - I with List - II.
| List - I (Complex) | List - II (Hybridisation of central metal ion) |
|---|---|
| (A) [CoF6]3- | (I) d2sp3 |
| (B) [NiCl4]2- | (II) sp3 |
| (C) [Co(NH3)6]3+ | (III) sp3d2 |
| (D) [Ni(CN)4]2- | (IV) dsp2 |
Arrange the following in increasing order of solubility product :
$\mathrm{Ca}(\mathrm{OH})_2, \mathrm{AgBr}, \mathrm{PbS}, \mathrm{HgS}$
Match List-I with List-II.
| List - I (Saccharides) | List - II (Glycosidic-linkages found) |
|---|---|
| (A) Sucrose | (I) α 1 $-$ 4 |
| (B) Maltose | (II) α 1 $-$ 4 and α 1 $-$ 6 |
| (C) Lactose | (III) α 1 $-$ β 2 |
| (D) Amylopectin | (IV) β 1 $-$ 4 |
Choose the correct answer from the options given below :

An ideal gas undergoes a cyclic transformation starting from the point A and coming back to the same point by tracing the path A→B→C→D→A as shown in the three cases above.
Choose the correct option regarding ΔU :
Which of the following is/are not correct with respect to energy of atomic orbitals of hydrogen atom?
(A) 1s < 2p < 3d < 4s
(B) 1s < 2s = 2p < 3s = 3p
(C) 1s < 2s < 2p < 3s < 3p
(D) 1s < 2s < 4s < 3d
Choose the correct answer from the options given below :
Identify product [A], [B] and [C] in the following reaction sequence.
$\mathrm{CH}_3-\mathrm{C} \equiv \mathrm{CH} \xrightarrow[\mathrm{H}_2]{\mathrm{Pd} / \mathrm{C}}[\mathrm{A}] \xrightarrow[\text { (ii) } \mathrm{Zn}, \mathrm{H}_2 \mathrm{O}]{\text { (i) } \mathrm{O}_3}[\mathrm{~B}]+[\mathrm{C}]$
Identify correct conversion during acidic hydrolysis from the following :
(A) starch gives galactose.
(B) cane sugar gives equal amount of glucose and fructose.
(C) milk sugar gives glucose and galactose.
(D) amylopectin gives glucose and fructose.
(E) amylose gives only glucose.
Choose the correct answer from the options given below :
The total number of compounds from below when treated with hot KMnO4, giving benzoic acid is:


Assume a living cell with 0.9% (w/w) of glucose solution (aqueous). This cell is immersed in another solution having equal mole fraction of glucose and water.
(Consider the data upto first decimal place only)
The cell will :
The purification method based on the following physical transformation is :
$\underset{(X)}{\text { Solid }} \xrightarrow[]{\text { Heat }} \underset{(X)}{\text { Vapour }} \xrightarrow{\text { Cool }} \underset{(X)}{\text { Solid }}$Identify correct statements :
(A) Primary amines do not give diazonium salts when treated with $\mathrm{NaNO}_2$ in acidic condition.
(B) Aliphatic and aromatic primary amines on heating with $\mathrm{CHCl}_3$ and ethanolic KOH form carbylamines.
(C) Secondary and tertiary amines also give carbylamine test.
(D) Benzenesulfonyl chloride is known as Hinsberg's reagent.
(E) Tertiary amines reacts with benzenesulfonyl chloride very easily.
Choose the correct answer from the options given below :
Identify the inorganic sulphides that are yellow in colour :
(A) $(NH_4)_2S$
(B) $PbS$
(C) $CuS$
(D) $As_2S_3$
(E) $As_2S_5$
Choose the correct answer from the options given below :
Consider an elementary reaction
$$ \mathrm{A}(\mathrm{~g})+\mathrm{B}(\mathrm{~g}) \rightarrow \mathrm{C}(\mathrm{~g})+\mathrm{D}(\mathrm{~g}) $$
If the volume of reaction mixture is suddenly reduced to $\frac{1}{3}$ of its initial volume, the reaction rate will become ' $x^{\prime}$ times of the original reaction rate. The value of $x$ is :
Given below are two statements :
Statement I : According to the Law of Octaves, the elements were arranged in the increasing order of their atomic number.
Statement II : Meyer observed a periodically repeated pattern upon plotting physical properties of certain elements against their respective atomic numbers.
In the light of the above statements, choose the correct answer from the options given below :
Concentrated nitric acid is labelled as $75 \%$ by mass. The volume in mL of the solution which contains 30 g of nitric acid is ______________.
Given : Density of nitric acid solution is $1.25 \mathrm{~g} / \mathrm{mL}$.
For bacterial growth in a cell culture, growth law is very similar to the law of radioactive decay. Which of the following graphs is most suitable to represent bacterial colony growth ?
Where N - Number of Bacteria at any time, $\mathrm{N}_0$ - Initial number of Bacteria.
$\mathrm{O}_2, \mathrm{O}_2^{+}, \mathrm{O}_2^{-}, \mathrm{NO}, \mathrm{NO}_2, \mathrm{CO}, \mathrm{K}_2\left[\mathrm{NiCl}_4\right],\left[\mathrm{Co}\left(\mathrm{NH}_3\right)_6\right] \mathrm{Cl}_3, \mathrm{~K}_2\left[\mathrm{Ni}(\mathrm{CN})_4\right]$
The current in Amperes used for the given electrolysis is ___________ . (Nearest integer).
Consider the following data :
Heat of formation of $\mathrm{CO}_2(\mathrm{g})=-393.5 \mathrm{~kJ} \mathrm{~mol}{ }^{-1}$
Heat of formation of $\mathrm{H}_2 \mathrm{O}(\mathrm{l})=-286.0 \mathrm{~kJ} \mathrm{~mol}{ }^{-1}$
Heat of combustion of benzene $=-3267.0 \mathrm{~kJ} \mathrm{~mol}^{-1}$
The heat of formation of benzene is __________ $\mathrm{kJ} \mathrm{mol}^{-1}$. (Nearest integer)
A group 15 element forms $\mathrm{d} \pi-\mathrm{d} \pi$ bond with transition metals. It also forms hydride, which is a strongest base among the hydrides of other group members that form $d \pi-d \pi$ bond.
The atomic number of the element is _______ .
Mathematics
The area of the region bounded by the curves $x(1+y^2)=1$ and $y^2=2x$ is:
Bag $B_1$ contains 6 white and 4 blue balls, Bag $B_2$ contains 4 white and 6 blue balls, and Bag $B_3$ contains 5 white and 5 blue balls. One of the bags is selected at random and a ball is drawn from it. If the ball is white, then the probability that the ball is drawn from Bag $B_2$ is:
Let $\mathrm{f}: \mathrm{R} \rightarrow \mathrm{R}$ be a twice differentiable function such that $f(2)=1$. If $\mathrm{F}(\mathrm{x})=\mathrm{x} f(\mathrm{x})$ for all $\mathrm{x} \in \mathrm{R}$, $\int\limits_0^2 x F^{\prime}(x) d x=6$ and $\int\limits_0^2 x^2 F^{\prime \prime}(x) d x=40$, then $F^{\prime}(2)+\int\limits_0^2 F(x) d x$ is equal to :
The square of the distance of the point $ \left( \frac{15}{7}, \frac{32}{7}, 7 \right) $ from the line $ \frac{x + 1}{3} = \frac{y + 3}{5} = \frac{z + 5}{7} $ in the direction of the vector $ \hat{i} + 4\hat{j} + 7\hat{k} $ is:
Two equal sides of an isosceles triangle are along $ -x + 2y = 4 $ and $ x + y = 4 $. If $ m $ is the slope of its third side, then the sum, of all possible distinct values of $ m $, is:
Let the coefficients of three consecutive terms $T_r$, $T_{r+1}$ and $T_{r+2}$ in the binomial expansion of $(a + b)^{12}$ be in a G.P. and let $p$ be the number of all possible values of $r$. Let $q$ be the sum of all rational terms in the binomial expansion of $(\sqrt[4]{3}+\sqrt[3]{4})^{12}$. Then $p + q$ is equal to:
If the components of $\vec{a}=\alpha \hat{i}+\beta \hat{j}+\gamma \hat{k}$ along and perpendicular to $\vec{b}=3 \hat{i}+\hat{j}-\hat{k}$ respectively, are $\frac{16}{11}(3 \hat{i}+\hat{j}-\hat{k})$ and $\frac{1}{11}(-4 \hat{i}-5 \hat{j}-17 \hat{k})$, then $\alpha^2+\beta^2+\gamma^2$ is equal to :
Let [x] denote the greatest integer less than or equal to x. Then the domain of $ f(x) = \sec^{-1}(2[x] + 1) $ is:
Let S be the set of all the words that can be formed by arranging all the letters of the word GARDEN. From the set S, one word is selected at random. The probability that the selected word will NOT have vowels in alphabetical order is:
If A and B are the points of intersection of the circle $x^2 + y^2 - 8x = 0$ and the hyperbola $\frac{x^2}{9} - \frac{y^2}{4} = 1$ and a point P moves on the line $2x - 3y + 4 = 0$, then the centroid of $\Delta PAB$ lies on the line :
If $\alpha + i\beta$ and $\gamma + i\delta$ are the roots of $x^2 - (3 - 2i)x - (2i - 2) = 0$, $i = \sqrt{-1}$, then $\alpha \gamma + \beta \delta$ is equal to:
Let $f$ be a real valued continuous function defined on the positive real axis such that $g(x)=\int\limits_0^x t f(t) d t$. If $g\left(x^3\right)=x^6+x^7$, then value of $\sum\limits_{r=1}^{15} f\left(r^3\right)$ is :
Let $f(x)=\lim \limits_{n \rightarrow \infty} \sum\limits_{r=0}^n\left(\frac{\tan \left(x / 2^{r+1}\right)+\tan ^3\left(x / 2^{r+1}\right)}{1-\tan ^2\left(x / 2^{r+1}\right)}\right)$ Then $\lim\limits_{x \rightarrow 0} \frac{e^x-e^{f(x)}}{(x-f(x))}$ is equal to ___________.
Let $A$ and $B$ be the two points of intersection of the line $y+5=0$ and the mirror image of the parabola $y^2=4 x$ with respect to the line $x+y+4=0$. If $d$ denotes the distance between $A$ and $B$, and a denotes the area of $\triangle S A B$, where $S$ is the focus of the parabola $y^2=4 x$, then the value of $(a+d)$ is __________.
If $y=y(x)$ is the solution of the differential equation, $\sqrt{4-x^2} \frac{\mathrm{~d} y}{\mathrm{~d} x}=\left(\left(\sin ^{-1}\left(\frac{x}{2}\right)\right)^2-y\right) \sin ^{-1}\left(\frac{x}{2}\right),-2 \leq x \leq 2, y(2)=\frac{\pi^2-8}{4}$, then $y^2(0)$ is equal to ___________.
The interior angles of a polygon with n sides, are in an A.P. with common difference 6°. If the largest interior angle of the polygon is 219°, then n is equal to _______.
The number of natural numbers, between 212 and 999, such that the sum of their digits is 15, is _______.
Physics
A concave mirror produces an image of an object such that the distance between the object and image is 20 cm. If the magnification of the image is –3, then the magnitude of the radius of curvature of the mirror is :
A uniform rod of mass 250 g having length 100 cm is balanced on a sharp edge at 40 cm mark. A mass of 400 g is suspended at 10 cm mark. To maintain the balance of the rod, the mass to be suspended at 90 cm mark, is

In the circuit shown here, assuming threshold voltage of diode is negligibly small, then voltage $ V_{AB} $ is correctly represented by:
A uniform magnetic field of 0.4 T acts perpendicular to a circular copper disc 20 cm in radius. The disc is having a uniform angular velocity of 10 $ \pi $ rad s-1 about an axis through its centre and perpendicular to the disc. What is the potential difference developed between the axis of the disc and the rim? $(\pi=3.14)$
The velocity-time graph of an object moving along a straight line is shown in the figure. What is the distance covered by the object between $t = 0$ to $t = 4s$?

The magnetic field of an E.M. wave is given by $\vec{B} = \left( \frac{\sqrt{3}}{2} \hat{i} + \frac{1}{2} \hat{j} \right) 30 \sin \left[ \omega \left( t - \frac{z}{c} \right) \right]$ (S.I. Units).
The corresponding electric field in S.I. units is:
A balloon and its content having mass M is moving up with an acceleration ‘a’. The mass that must be released from the content so that the balloon starts moving up with an acceleration ‘3a’ will be
(Take ‘g’ as acceleration due to gravity)
The ratio of vapour densities of two gases at the same temperature is $ \frac{4}{25} $, then the ratio of r.m.s. velocities will be :

An infinite wire has a circular bend of radius a, and carrying a current I as shown in the figure. The magnitude of magnetic field at the origin O of the arc is given by:
The frequency of revolution of the electron in Bohr's orbit varies with n, the principal quantum number as:
Earth has mass 8 times and radius 2 times that of a planet. If the escape velocity from the earth is 11.2 km/s, the escape velocity in km/s from the planet will be:
Which of the following phenomena cannot be explained by wave theory of light?
Match List - I with List - II.
| List - I | List - II |
|---|---|
| (A) Angular Impulse | (I) M0 L2 T-2 |
| (B) Latent Heat | (II) M L2 T-3 A-1 |
| (C) Electrical resistivity | (III) M L2 T-1 |
| (D) Electromotive force | (IV) M L3 T-3 A-2 |
Choose the correct answer from the options given below:
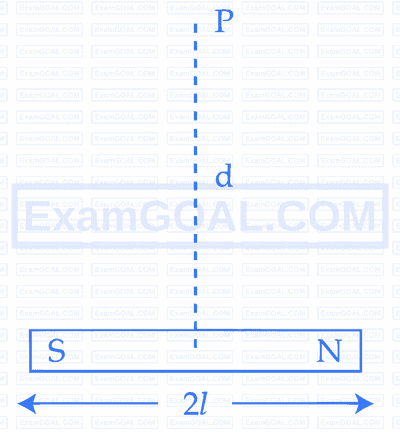
A bar magnet has total length $2 l=20$ units and the field point P is at a distance $\mathrm{d}=10$ units from the centre of the magnet. If the relative uncertainty of length measurement is $1 \%$, then uncertainty of the magnetic field at point P is :
The kinetic energy of translation of the molecules in 50 g of $ \text{CO}_2 $ gas at 17°C is :
A 400 g solid cube having an edge of length 10 cm floats in water. How much volume of the cube is outside the water?
(Given: density of water = 1000 kg m-3)
Given below are two statements. One is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A) : Knowing initial position $\mathrm{x}_0$ and initial momentum $p_0$ is enough to determine the position and momentum at any time $t$ for a simple harmonic motion with a given angular frequency $\omega$.
Reason (R) : The amplitude and phase can be expressed in terms of $\mathrm{X}_0$ an $\mathrm{p}_0$.
In the light of the above statements, choose the correct answer from the options given below :
A parallel plate capacitor of capacitance 1 µF is charged to a potential difference of 20 V. The distance between plates is 1 µm. The energy density between plates of capacitor is :
The volume contraction of a solid copper cube of edge length 10 cm , when subjected to a hydraulic pressure of $7 \times 10^{6} ~\mathrm{Pa}$, would be __________ $\mathrm{mm}{ }^3$.
(Given bulk modulus of copper $=1.4 \times 10^{11} \mathrm{~N} \mathrm{~m}^{-2}$ )
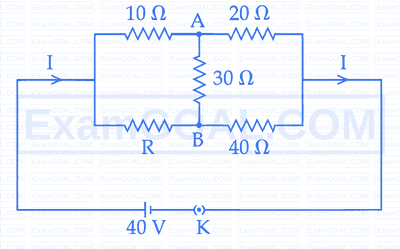
The value of current I in the electrical circuit as given below, when potential at A is equal to the potential at B, will be _______ A.

A conducting bar moves on two conducting rails as shown in the figure. A constant magnetic field B exists into the page. The bar starts to move from the vertex at time t = 0 with a constant velocity. If the induced EMF is E ∝ tn, then value of n is _________.