JEE Main 2021 (Online) 26th August Evening Shift
Paper was held on
Thu, Aug 26, 2021 9:30 AM
Chemistry
1
Which one of the following phenols does not give colour when condensed with phthalic anhydride in presence of conc. H2SO4 ?
2
The bond order and magnetic behaviour of $$O_2^ - $$ ion are respectively :
3
Given below are two statements : one is labelled as Assertion (A) and other is labelled as Reason (R).
Assertion (A) : Sucrose is a disaccharide and a non-reducing sugar.
Reason (R) : Sucrose involves glycosidic linkage between C1 of $$\beta$$-glucose and C2 of $$\alpha$$-fructose.
Choose the most appropriate answer from the options given below :
Assertion (A) : Sucrose is a disaccharide and a non-reducing sugar.
Reason (R) : Sucrose involves glycosidic linkage between C1 of $$\beta$$-glucose and C2 of $$\alpha$$-fructose.
Choose the most appropriate answer from the options given below :
4
Match List - I with List - II :

Choose the most appropriate match :

Choose the most appropriate match :
5
Given below are two statements : one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A) : Barium carbonate is insoluble in water and is highly stable.
Reason (R) : The thermal stability of the carbonates increases with increasing cationic size.
Assertion (A) : Barium carbonate is insoluble in water and is highly stable.
Reason (R) : The thermal stability of the carbonates increases with increasing cationic size.
6

The major product in the above reaction is :
7
Indicate the complex/complex ion which did not show any geometrical isomerism :
8
Arrange the following Cobalt complexes in the order of increasing Crystal Field Stabilization Energy (CFSE) value.
Complexes : $$\mathop {{{[Co{F_6}]}^{3 - }}}\limits_A ,\mathop {{{[Co{{({H_2}O)}_6}]}^{2 + }}}\limits_B ,\mathop {{{[Co{{(N{H_3})}_6}]}^{3 + }}}\limits_C and \mathop {{{[Co{{({en})}_3}]}^{3 + }}}\limits_D $$
Choose the correct option :
Complexes : $$\mathop {{{[Co{F_6}]}^{3 - }}}\limits_A ,\mathop {{{[Co{{({H_2}O)}_6}]}^{2 + }}}\limits_B ,\mathop {{{[Co{{(N{H_3})}_6}]}^{3 + }}}\limits_C and \mathop {{{[Co{{({en})}_3}]}^{3 + }}}\limits_D $$
Choose the correct option :
9
Which one of the following compounds is not aromatic?
10
The number of stereoisomers possible for 1, 2-dimethyl cyclopropane is :
11

Consider the given reaction, identify 'X' and 'Y' :
12

Consider the given reaction, the product A is :
13
In the sulphur estimation, 0.471 g of an organic compound gave 1.44 g of barium sulphate. The percentage of sulphur in the compound is ____________%. (Nearest integer)
(Atomic Mass of Ba = 137 u)
(Atomic Mass of Ba = 137 u)
14
The equilibrium constant Kc at 298 K for the reaction A + B $$\rightleftharpoons$$ C + D is 100. Starting with an equimolar solution with concentrations of A, B, C and D all equal to 1M, the equilibrium concentration of D is ___________ $$\times$$ 10$$-$$2 M. (Nearest integer)
15
For water $$\Delta$$vap H = 41 kJ mol$$-$$1 at 373 K and 1 bar pressure. Assuming that water vapour is an ideal gas that occupies a much larger volume than liquid water, the internal energy change during evaporation of water is ___________ kJ mol$$-$$1
[Use : R = 8.3 J mol$$-$$1 K$$-$$1]
[Use : R = 8.3 J mol$$-$$1 K$$-$$1]
16
A metal surface is exposed to 500 nm radiation. The threshold frequency of the metal for photoelectric current is 4.3 $$\times$$ 1014 Hz. The velocity of ejected electron is ____________ $$\times$$ 105 ms$$-$$1 (Nearest integer)
[Use : h = 6.63 $$\times$$ 10$$-$$34 Js, me = 9.0 $$\times$$ 10$$-$$31 kg]
[Use : h = 6.63 $$\times$$ 10$$-$$34 Js, me = 9.0 $$\times$$ 10$$-$$31 kg]
17
For the galvanic cell,
Zn(s) + Cu2+ (0.02 M) $$\to$$ Zn2+ (0.04 M) + Cu(s),
Ecell = ______________ $$\times$$ 10$$-$$2 V. (Nearest integer)
[Use : $$E_{Cu/C{u^{2 + }}}^0$$ = $$-$$ 0.34 V, $$E_{Zn/Z{n^{2 + }}}^0$$ = + 0.76 V, $${{2.303RT} \over F} = 0.059\,V$$]
Zn(s) + Cu2+ (0.02 M) $$\to$$ Zn2+ (0.04 M) + Cu(s),
Ecell = ______________ $$\times$$ 10$$-$$2 V. (Nearest integer)
[Use : $$E_{Cu/C{u^{2 + }}}^0$$ = $$-$$ 0.34 V, $$E_{Zn/Z{n^{2 + }}}^0$$ = + 0.76 V, $${{2.303RT} \over F} = 0.059\,V$$]
18
100 mL of Na3PO4 solution contains 3.45 g of sodium. The molarity of the solution is _____________ $$\times$$ 10$$-$$2 mol L$$-$$1. (Nearest integer)
[Atomic Masses - Na : 23.0 u, O : 16.0 u, P : 31.0 u]
[Atomic Masses - Na : 23.0 u, O : 16.0 u, P : 31.0 u]
19
The overall stability constant of the complex ion [Cu(NH3)4]2+ is 2.1 $$\times$$ 1013. The overall dissociations constant is y $$\times$$ 10$$-$$14. Then y is __________. (Nearest integer)
20
83 g of ethylene glycol dissolved in 625 g of water. The freezing point of the solution is ____________ K. (Nearest integer)
[Use : Molal Freezing point depression constant of water = 1.86 K kg mol$$-$$1]
Freezing Point of water = 273 K
Atomic masses : C : 12.0 u, O : 16.0 u, H : 1.0 u]
[Use : Molal Freezing point depression constant of water = 1.86 K kg mol$$-$$1]
Freezing Point of water = 273 K
Atomic masses : C : 12.0 u, O : 16.0 u, H : 1.0 u]
21
The reaction rate for the reaction
[PtCl4]2$$-$$ + H2O $$\rightleftharpoons$$ [Pt(H2O)Cl3]$$-$$ + Cl$$-$$
was measured as a function of concentrations of different species. It was observed that $${{ - d\left[ {{{\left[ {PtC{l_4}} \right]}^{2 - }}} \right]} \over {dt}} = 4.8 \times {10^{ - 5}}\left[ {{{\left[ {PtC{l_4}} \right]}^{2 - }}} \right] - 2.4 \times {10^{ - 3}}\left[ {{{\left[ {Pt({H_2}O)C{l_3}} \right]}^ - }} \right]\left[ {C{l^ - }} \right]$$.
where square brackets are used to denote molar concentrations. The equilibrium constant Kc = ____________ . (Nearest integer)
[PtCl4]2$$-$$ + H2O $$\rightleftharpoons$$ [Pt(H2O)Cl3]$$-$$ + Cl$$-$$
was measured as a function of concentrations of different species. It was observed that $${{ - d\left[ {{{\left[ {PtC{l_4}} \right]}^{2 - }}} \right]} \over {dt}} = 4.8 \times {10^{ - 5}}\left[ {{{\left[ {PtC{l_4}} \right]}^{2 - }}} \right] - 2.4 \times {10^{ - 3}}\left[ {{{\left[ {Pt({H_2}O)C{l_3}} \right]}^ - }} \right]\left[ {C{l^ - }} \right]$$.
where square brackets are used to denote molar concentrations. The equilibrium constant Kc = ____________ . (Nearest integer)
22
A chloro compound "A".
(i) forms aldehydes on ozonolysis followed by the hydrolysis.
(ii) when vaporized completely 1.53 g of A, gives 448 mL of vapour at STP.
The number of carbon atoms in a molecule of compound A is ___________.
(i) forms aldehydes on ozonolysis followed by the hydrolysis.
(ii) when vaporized completely 1.53 g of A, gives 448 mL of vapour at STP.
The number of carbon atoms in a molecule of compound A is ___________.
Mathematics
1
Let [t] denote the greatest integer less than or equal to t. Let
f(x) = x $$-$$ [x], g(x) = 1 $$-$$ x + [x], and h(x) = min{f(x), g(x)}, x $$\in$$ [$$-$$2, 2]. Then h is :
f(x) = x $$-$$ [x], g(x) = 1 $$-$$ x + [x], and h(x) = min{f(x), g(x)}, x $$\in$$ [$$-$$2, 2]. Then h is :
2
Let $$A = \left( {\matrix{
1 & 0 & 0 \cr
0 & 1 & 1 \cr
1 & 0 & 0 \cr
} } \right)$$. Then A2025 $$-$$ A2020 is equal to :
3
The local maximum value of the function $$f(x) = {\left( {{2 \over x}} \right)^{{x^2}}}$$, x > 0, is
4
If the value of the integral
$$\int\limits_0^5 {{{x + [x]} \over {{e^{x - [x]}}}}dx = \alpha {e^{ - 1}} + \beta } $$, where $$\alpha$$, $$\beta$$ $$\in$$ R, 5$$\alpha$$ + 6$$\beta$$ = 0, and [x] denotes the greatest integer less than or equal to x; then the value of ($$\alpha$$ + $$\beta$$)2 is equal to :
$$\int\limits_0^5 {{{x + [x]} \over {{e^{x - [x]}}}}dx = \alpha {e^{ - 1}} + \beta } $$, where $$\alpha$$, $$\beta$$ $$\in$$ R, 5$$\alpha$$ + 6$$\beta$$ = 0, and [x] denotes the greatest integer less than or equal to x; then the value of ($$\alpha$$ + $$\beta$$)2 is equal to :
5
Let y(x) be the solution of the differential equation
2x2 dy + (ey $$-$$ 2x)dx = 0, x > 0. If y(e) = 1, then y(1) is equal to :
2x2 dy + (ey $$-$$ 2x)dx = 0, x > 0. If y(e) = 1, then y(1) is equal to :
6
The domain of the function $${{\mathop{\rm cosec}\nolimits} ^{ - 1}}\left( {{{1 + x} \over x}} \right)$$ is :
7
A fair die is tossed until six is obtained on it. Let x be the number of required tosses, then the conditional probability P(x $$\ge$$ 5 | x > 2) is :
8
If $$\sum\limits_{r = 1}^{50} {{{\tan }^{ - 1}}{1 \over {2{r^2}}} = p} $$, then the value of tan p is :
9
Two fair dice are thrown. The numbers on them are taken as $$\lambda$$ and $$\mu$$, and a system of linear equations
x + y + z = 5
x + 2y + 3z = $$\mu$$
x + 3y + $$\lambda$$z = 1
is constructed. If p is the probability that the system has a unique solution and q is the probability that the system has no solution, then :
x + y + z = 5
x + 2y + 3z = $$\mu$$
x + 3y + $$\lambda$$z = 1
is constructed. If p is the probability that the system has a unique solution and q is the probability that the system has no solution, then :
10
The locus of the mid points of the chords of the hyperbola x2 $$-$$ y2 = 4, which touch the parabola y2 = 8x, is :
11
The value of
$$2\sin \left( {{\pi \over 8}} \right)\sin \left( {{{2\pi } \over 8}} \right)\sin \left( {{{3\pi } \over 8}} \right)\sin \left( {{{5\pi } \over 8}} \right)\sin \left( {{{6\pi } \over 8}} \right)\sin \left( {{{7\pi } \over 8}} \right)$$ is :
$$2\sin \left( {{\pi \over 8}} \right)\sin \left( {{{2\pi } \over 8}} \right)\sin \left( {{{3\pi } \over 8}} \right)\sin \left( {{{5\pi } \over 8}} \right)\sin \left( {{{6\pi } \over 8}} \right)\sin \left( {{{7\pi } \over 8}} \right)$$ is :
12
If $${\left( {\sqrt 3 + i} \right)^{100}} = {2^{99}}(p + iq)$$, then p and q are roots of the equation :
13
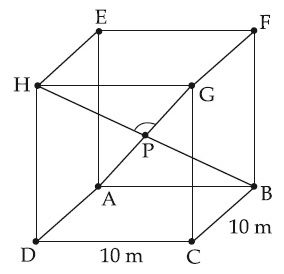
A hall has a square floor of dimension 10 m $$\times$$ 10 m (see the figure) and vertical walls. If the angle GPH between the diagonals AG and BH is $${\cos ^{ - 1}}{1 \over 5}$$, then the height of the hall (in meters) is :

14
The value of $$\int\limits_{ - {\pi \over 2}}^{{\pi \over 2}} {\left( {{{1 + {{\sin }^2}x} \over {1 + {\pi ^{\sin x}}}}} \right)} \,dx$$ is
15
A circle C touches the line x = 2y at the point (2, 1) and intersects the circle
C1 : x2 + y2 + 2y $$-$$ 5 = 0 at two points P and Q such that PQ is a diameter of C1. Then the diameter of C is :
C1 : x2 + y2 + 2y $$-$$ 5 = 0 at two points P and Q such that PQ is a diameter of C1. Then the diameter of C is :
16
$$\mathop {\lim }\limits_{x \to 2} \left( {\sum\limits_{n = 1}^9 {{x \over {n(n + 1){x^2} + 2(2n + 1)x + 4}}} } \right)$$ is equal to :
17
The sum of all 3-digit numbers less than or equal to 500, that are formed without using the digit "1" and they all are multiple of 11, is _____________.
18
Let a and b respectively be the points of local maximum and local minimum of the function f(x) = 2x3 $$-$$ 3x2 $$-$$ 12x. If A is the total area of the region bounded by y = f(x), the x-axis and the lines x = a and x = b, then 4A is equal to ______________.
19
If the projection of the vector $$\widehat i + 2\widehat j + \widehat k$$ on the sum of the two vectors $$2\widehat i + 4\widehat j - 5\widehat k$$ and $$ - \lambda \widehat i + 2\widehat j + 3\widehat k$$ is 1, then $$\lambda$$ is equal to __________.
20
Let a1, a2, ......., a10 be an AP with common difference $$-$$ 3 and b1, b2, ........., b10 be a GP with common ratio 2. Let ck = ak + bk, k = 1, 2, ......, 10. If c2 = 12 and c3 = 13, then $$\sum\limits_{k = 1}^{10} {{c_k}} $$ is equal to _________.
21
Let $$\lambda$$ $$\ne$$ 0 be in R. If $$\alpha$$ and $$\beta$$ are the roots of the equation x2 $$-$$ x + 2$$\lambda$$ = 0, and $$\alpha$$ and $$\gamma$$ are the roots of equation 3x2 $$-$$ 10x + 27$$\lambda$$ = 0, then $${{\beta \gamma } \over \lambda }$$ is equal to ____________.
22
Let the mean and variance of four numbers 3, 7, x and y(x > y) be 5 and 10 respectively. Then the mean of four numbers 3 + 2x, 7 + 2y, x + y and x $$-$$ y is ______________.
23
The least positive integer n such that $${{{{(2i)}^n}} \over {{{(1 - i)}^{n - 2}}}},i = \sqrt { - 1} $$ is a positive integer, is ___________.
Physics
1
The temperature of equal masses of three different liquids x, y and z are 10$$^\circ$$C, 20$$^\circ$$C and 30$$^\circ$$C respectively. The temperature of mixture when x is mixed with y is 16$$^\circ$$C and that when y is mixed with z is 26$$^\circ$$C. The temperature of mixture when x and z are mixed will be :
2
The de-Broglie wavelength of a particle having kinetic energy E is $$\lambda$$. How much extra energy must be given to this particle so that the de-Broglie wavelength reduces to 75% of the initial value?
3
A particle of mass m is suspended from a ceiling through a string of length L. The particle moves in a horizontal circle of radius r such that $$r = {L \over {\sqrt 2 }}$$. The speed of particle will be :
4
A cylindrical container of volume 4.0 $$\times$$ 10$$-$$3 m3 contains one mole of hydrogen and two moles of carbon dioxide. Assume the temperature of the mixture is 400 K. The pressure of the mixture of gases is :
[Take gas constant as 8.3 J mol$$-$$1 K$$-$$1]
[Take gas constant as 8.3 J mol$$-$$1 K$$-$$1]
5
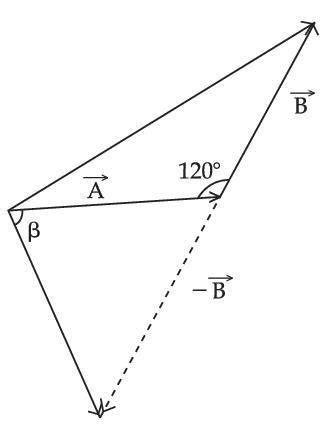
The angle between vector $$\left( {\overrightarrow A } \right)$$ and $$\left( {\overrightarrow A - \overrightarrow B } \right)$$ is :

6
A light beam is described by $$E = 800\sin \omega \left( {t - {x \over c}} \right)$$. An electron is allowed to move normal to the propagation of light beam with a speed of 3 $$\times$$ 107 ms$$-$$1. What is the maximum magnetic force exerted on the electron?
7
The two thin coaxial rings, each of radius 'a' and having charges +Q and $$-$$Q respectively are separated by a distance of 's'. The potential difference between the centres of the two rings is :
8
If you are provided a set of resistances 2$$\Omega$$, 4$$\Omega$$, 6$$\Omega$$ and 8$$\Omega$$. Connect these resistances so as to obtain an equivalent resistance of $${{46} \over 3}$$$$\Omega$$.
9
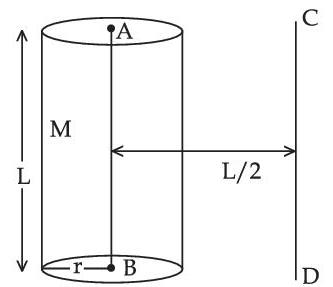
The solid cylinder of length 80 cm and mass M has a radius of 20 cm. Calculate the density of the material used if the moment of inertia of the cylinder about an axis CD parallel to AB as shown in figure is 2.7 kg m2.

10
A parallel plate capacitor with plate area A has separation d between the plates. Two dielectric slabs of dielectric constant K1 and K2 of same area A/2 and thickness d/2 are inserted in the space between the plates. The capacitance of the capacitor will be given by:


11
A bomb is dropped by fighter plane flying horizontally. To an observer sitting in the plane, the trajectory of the bomb is a :
12
An electric bulb of 500 watt at 100 volt is used in a circuit having a 200 V supply. Calculate the resistance R to be connected in series with the bulb so that the power delivered by the bulb is 500 W.
13
Four NOR gates are connected as shown in figure. The truth table for the given figure is :


14
Match List - I with List - II

Choose the most appropriate answer from the options given below :

Choose the most appropriate answer from the options given below :
15
In the given circuit the AC source has $$\omega$$ = 100 rad s-1. Considering the inductor and capacitor to be ideal, what will be the current I flowing through the circuit?


16
If the length of the pendulum in pendulum clock increases by 0.1%, then the error in time per day is :
17
Two blocks of masses 3 kg and 5 kg are connected by a metal wire going over a smooth pulley. The breaking stress of the metal is $${{24} \over \pi } \times {10^2}$$ Nm-2. What is the minimum radius of the wire ? (Take g = 10 ms-2)
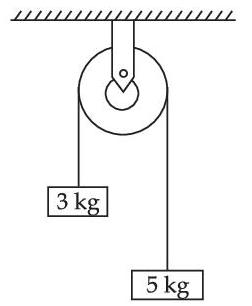

18
Two waves are simultaneously passing through a string and their equations are :
y1 = A1 sin k(x $$-$$ vt), y2 = A2 sin k(x $$-$$ vt + x0). Given amplitudes A1 = 12 mm and A2 = 5 mm, x0 = 3.5 cm and wave number k = 6.28 cm$$-$$1. The amplitude of resulting wave will be ................ mm.
y1 = A1 sin k(x $$-$$ vt), y2 = A2 sin k(x $$-$$ vt + x0). Given amplitudes A1 = 12 mm and A2 = 5 mm, x0 = 3.5 cm and wave number k = 6.28 cm$$-$$1. The amplitude of resulting wave will be ................ mm.
19
A source of light is placed in front of a screen. Intensity of light on the screen is I. Two Polaroids P1 and P2 are so placed in between the source of light and screen that the intensity of light on screen is I/2. P2 should be rotated by an angle of (degrees) so that the intensity of light on the screen becomes $${{3I} \over 8}$$.
20
If the maximum value of accelerating potential provided by a ratio frequency oscillator is 12 kV. The number of revolution made by a proton in a cyclotron to achieve one sixth of the speed of light is ...............
[mp = 1.67 $$\times$$ 10$$-$$27 kg, e = 1.6 $$\times$$ 10$$-$$19C, Speed of light = 3 $$\times$$ 108 m/s]
[mp = 1.67 $$\times$$ 10$$-$$27 kg, e = 1.6 $$\times$$ 10$$-$$19C, Speed of light = 3 $$\times$$ 108 m/s]
21
The acceleration due to gravity is found upto an accuracy of 4% on a planet. The energy supplied to a simple pendulum to known mass 'm' to undertake oscillations of time period T is being estimated. If time period is measured to an accuracy of 3%, the accuracy to which E is known as ..............%
22
A circular coil of radius 8.0 cm and 20 turns is rotated about its vertical diameter with an angular speed of 50 rad s$$-$$1 in a uniform horizontal magnetic field of 3.0 $$\times$$ 10$$-$$2 T. The maximum emf induced the coil will be ................. $$\times$$ 10$$-$$2 volt (rounded off to the nearest integer)
23
Two simple harmonic motions are represented by the equations
$${x_1} = 5\sin \left( {2\pi t + {\pi \over 4}} \right)$$ and $${x_2} = 5\sqrt 2 (\sin 2\pi t + \cos 2\pi t)$$. The amplitude of second motion is ................ times the amplitude in first motion.
$${x_1} = 5\sin \left( {2\pi t + {\pi \over 4}} \right)$$ and $${x_2} = 5\sqrt 2 (\sin 2\pi t + \cos 2\pi t)$$. The amplitude of second motion is ................ times the amplitude in first motion.
24
A coil in the shape of an equilateral triangle of side 10 cm lies in a vertical plane between the pole pieces of permanent magnet producing a horizontal magnetic field 20 mT. The torque acting on the coil when a current of 0.2 A is passed through it and its plane becomes parallel to the magnetic field will be $$\sqrt x $$ $$\times$$ 10$$-$$5 Nm. The value of x is .................
25
For the given circuit, the power across Zener diode is .............. mW.


26
An object is placed at a distance of 12 cm from a convex lens. A convex mirror of focal length 15 cm is placed on other side of lens at 8 cm as shown in the figure. Image of object coincides with the object.

When the convex mirror is removed, a real and inverted image is formed at a position. The distance of the image from the object will be .............. (cm)

When the convex mirror is removed, a real and inverted image is formed at a position. The distance of the image from the object will be .............. (cm)
27
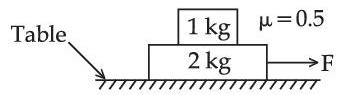
The coefficient of static friction between two blocks is 0.5 and the table is smooth. The maximum horizontal force that can be applied to move the blocks together is .................. N. (take g = 10 ms-2)