JEE Main 2020 (Online) 6th September Evening Slot
Paper was held on
Sun, Sep 6, 2020 9:30 AM
Chemistry
1
The correct match between Item-I (starting
material) and Item-II (reagent) for the
preparation of benzaldehyde is :
| Item-I | Item-II |
|---|---|
| (I) Benzene | (P) HCl and SnCl2, H3O+ |
| (II) Benzonitrile | (Q) H2, Pd-BaSO4, S and quinoline |
| (III) Benzoyl Chloride | (R) CO, HCl and AlCl3 |
2
The average molar mass of chlorine is
35.5 g mol–1. The ratio of 35Cl to 37Cl in naturally
occuring chlorine is close to :
3
Mischmetal is an alloy consisting mainly of :
4
Which of the following compounds can be
prepared in good yield by Gabriel phthalimide
synthesis?
5
For a reaction,
4M(s) + nO2(g) $$ \to $$ 2M2On(s)
the free energy change is plotted as a function of temperature. The temperature below which the oxide is stable could be inferred from the plot as the point at which :
4M(s) + nO2(g) $$ \to $$ 2M2On(s)
the free energy change is plotted as a function of temperature. The temperature below which the oxide is stable could be inferred from the plot as the point at which :
6
The IUPAC name of the following compound is :


7
The rate of a reaction decreased by 3.555
times when the temperature was changed
from 40oC to 30oC. The activation energy
(in kJ mol–1) of the reaction is _______.
Take; R = 8.314 J mol–1 K–1 ln 3.555 = 1.268
Take; R = 8.314 J mol–1 K–1 ln 3.555 = 1.268
8
The atomic number of Unnilunium is _______.
9
If the solubility product of AB2 is 3.20 $$ \times $$ 10–11 M3,
then the solubility of AB2 in pure water is
_____ $$ \times $$ 10–4 mol L–1.
[Assuming that neither kind of ion reacts with water]
[Assuming that neither kind of ion reacts with water]
10
A solution of phenol in chloroform when treated
with aqueous NaOH gives compound P as a
major product. The mass percentage of carbon
in P is ______. (to the nearest integer)
(Atomic mass : C = 12; H = 1; O = 16)
(Atomic mass : C = 12; H = 1; O = 16)
11
For a d4 metal ion in an octahedral field, the
correct electronic configuration is :
12
The increasing order of the boiling points of
the major products A, B and C of the following
reactions will be :
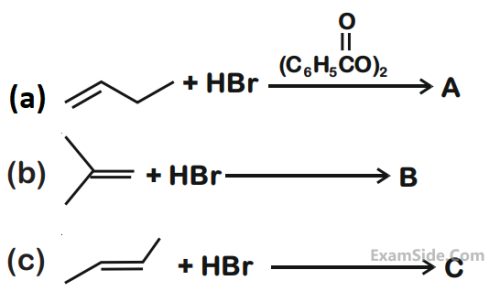

13
Match the following :
| Test/Method | Reagent |
|---|---|
| (i) Lucas Test | (a) C6H5SO2Cl/ aq. KOH |
| (ii) Dumas method | (b) HNO3/ AgNO3 |
| (iii) Kjeldahl’s method | (c) CuO/CO2 |
| (iv) Hinsberg test | (d) Conc. HCl and ZnCl2 |
| (e) H2SO4 |
14
The value of KC is 64 at 800 K for the reaction
N2(g) + 3H2(g) ⇌ 2NH3(g)
The value of KC for the following reaction is :
NH3(g) ⇌ $${1 \over 2}$$N2(g) + $${3 \over 2}$$H2(g)
N2(g) + 3H2(g) ⇌ 2NH3(g)
The value of KC for the following reaction is :
NH3(g) ⇌ $${1 \over 2}$$N2(g) + $${3 \over 2}$$H2(g)
15
A set of solutions is prepared using 180 g of
water as a solvent and 10 g of different nonvolatile solutes A, B and C. The relative
lowering of vapour pressure in the presence of
these solutes are in the order :
[Given, molar mass of A = 100 g mol–1; B = 200 g mol–1; C = 10,000 g mol–1]
[Given, molar mass of A = 100 g mol–1; B = 200 g mol–1; C = 10,000 g mol–1]
16
For the given cell :
Cu(s) | Cu2+(C1M) || Cu2+(C2M) | Cu(s)
change in Gibbs energy ($$\Delta $$G) is negative, if :
Cu(s) | Cu2+(C1M) || Cu2+(C2M) | Cu(s)
change in Gibbs energy ($$\Delta $$G) is negative, if :
17
Which one of the following statements is not
true?
Mathematics
1
For a suitably chosen real constant a, let a
function, $$f:R - \left\{ { - a} \right\} \to R$$ be defined by
$$f(x) = {{a - x} \over {a + x}}$$. Further suppose that for any real number $$x \ne - a$$ and $$f(x) \ne - a$$,
(fof)(x) = x. Then $$f\left( { - {1 \over 2}} \right)$$ is equal to :
function, $$f:R - \left\{ { - a} \right\} \to R$$ be defined by
$$f(x) = {{a - x} \over {a + x}}$$. Further suppose that for any real number $$x \ne - a$$ and $$f(x) \ne - a$$,
(fof)(x) = x. Then $$f\left( { - {1 \over 2}} \right)$$ is equal to :
2
Let L denote the line in the xy-plane with x and
y intercepts as 3 and 1 respectively. Then the
image of the point (–1, –4) in this line is :
3
The area (in sq. units) of the region enclosed
by the curves y = x2 – 1 and y = 1 – x2 is equal to :
by the curves y = x2 – 1 and y = 1 – x2 is equal to :
4
Let z = x + iy be a non-zero complex number
such that $${z^2} = i{\left| z \right|^2}$$, where i = $$\sqrt { - 1} $$ , then z lies
on the :
5
The integral $$\int\limits_1^2 {{e^x}.{x^x}\left( {2 + {{\log }_e}x} \right)} dx$$ equals :
6
For all twice differentiable functions f : R $$ \to $$ R,
with f(0) = f(1) = f'(0) = 0
with f(0) = f(1) = f'(0) = 0
7
Let f : R $$ \to $$ R be a function defined by
f(x) = max {x, x2}. Let S denote the set of all points in R, where f is not differentiable. Then :
f(x) = max {x, x2}. Let S denote the set of all points in R, where f is not differentiable. Then :
8
The set of all real values of $$\lambda $$ for which the
function
$$f(x) = \left( {1 - {{\cos }^2}x} \right)\left( {\lambda + \sin x} \right),x \in \left( { - {\pi \over 2},{\pi \over 2}} \right)$$
has exactly one maxima and exactly one minima, is :
$$f(x) = \left( {1 - {{\cos }^2}x} \right)\left( {\lambda + \sin x} \right),x \in \left( { - {\pi \over 2},{\pi \over 2}} \right)$$
has exactly one maxima and exactly one minima, is :
9
The sum of distinct values of $$\lambda $$ for which the
system of equations
$$\left( {\lambda - 1} \right)x + \left( {3\lambda + 1} \right)y + 2\lambda z = 0$$
$$\left( {\lambda - 1} \right)x + \left( {4\lambda - 2} \right)y + \left( {\lambda + 3} \right)z = 0$$
$$2x + \left( {3\lambda + 1} \right)y + 3\left( {\lambda - 1} \right)z = 0$$
has non-zero solutions, is ________ .
$$\left( {\lambda - 1} \right)x + \left( {3\lambda + 1} \right)y + 2\lambda z = 0$$
$$\left( {\lambda - 1} \right)x + \left( {4\lambda - 2} \right)y + \left( {\lambda + 3} \right)z = 0$$
$$2x + \left( {3\lambda + 1} \right)y + 3\left( {\lambda - 1} \right)z = 0$$
has non-zero solutions, is ________ .
10
Consider the data on x taking the values
0, 2, 4, 8,....., 2n with frequencies
nC0 , nC1 , nC2 ,...., nCn respectively. If the
mean of this data is $${{728} \over {{2^n}}}$$, then n is equal to _________ .
0, 2, 4, 8,....., 2n with frequencies
nC0 , nC1 , nC2 ,...., nCn respectively. If the
mean of this data is $${{728} \over {{2^n}}}$$, then n is equal to _________ .
11
If $$\overrightarrow x $$ and $$\overrightarrow y $$ be two non-zero vectors such that
$$\left| {\overrightarrow x + \overrightarrow y } \right| = \left| {\overrightarrow x } \right|$$ and $${2\overrightarrow x + \lambda \overrightarrow y }$$ is perpendicular to $${\overrightarrow y }$$,
then the value of $$\lambda $$ is _________ .
12
Suppose that a function f : R $$ \to $$ R satisfies
f(x + y) = f(x)f(y) for all x, y $$ \in $$ R and f(1) = 3.
If $$\sum\limits_{i = 1}^n {f(i)} = 363$$ then n is equal to ________ .
f(x + y) = f(x)f(y) for all x, y $$ \in $$ R and f(1) = 3.
If $$\sum\limits_{i = 1}^n {f(i)} = 363$$ then n is equal to ________ .
13
The number of words (with or without meaning)
that can be formed from all the letters of the
word “LETTER” in which vowels never come
together is ________ .
14
The common difference of the A.P.
b1, b2, … , bm is 2 more than the common
difference of A.P. a1, a2, …, an. If
a40 = –159, a100 = –399 and b100 = a70, then b1 is equal to :
b1, b2, … , bm is 2 more than the common
difference of A.P. a1, a2, …, an. If
a40 = –159, a100 = –399 and b100 = a70, then b1 is equal to :
15
If $$\alpha $$ and $$\beta $$ are the roots of the equation
2x(2x + 1) = 1, then $$\beta $$ is equal to :
2x(2x + 1) = 1, then $$\beta $$ is equal to :
16
The probabilities of three events A, B and C are
given by
P(A) = 0.6, P(B) = 0.4 and P(C) = 0.5.
If P(A$$ \cup $$B) = 0.8, P(A$$ \cap $$C) = 0.3, P(A$$ \cap $$B$$ \cap $$C) = 0.2, P(B$$ \cap $$C) = $$\beta $$
and P(A$$ \cup $$B$$ \cup $$C) = $$\alpha $$, where 0.85 $$ \le \alpha \le $$ 0.95, then $$\beta $$ lies in the interval :
P(A) = 0.6, P(B) = 0.4 and P(C) = 0.5.
If P(A$$ \cup $$B) = 0.8, P(A$$ \cap $$C) = 0.3, P(A$$ \cap $$B$$ \cap $$C) = 0.2, P(B$$ \cap $$C) = $$\beta $$
and P(A$$ \cup $$B$$ \cup $$C) = $$\alpha $$, where 0.85 $$ \le \alpha \le $$ 0.95, then $$\beta $$ lies in the interval :
17
If the constant term in the binomial expansion
of
$${\left( {\sqrt x - {k \over {{x^2}}}} \right)^{10}}$$ is 405, then |k| equals :
$${\left( {\sqrt x - {k \over {{x^2}}}} \right)^{10}}$$ is 405, then |k| equals :
18
Let $$\theta = {\pi \over 5}$$ and $$A = \left[ {\matrix{
{\cos \theta } & {\sin \theta } \cr
{ - \sin \theta } & {\cos \theta } \cr
} } \right]$$.
If B = A + A4 , then det (B) :
If B = A + A4 , then det (B) :
Physics
1
Assuming the nitrogen molecule is moving with r.m.s. velocity at 400 K, the de-Broglie wavelength
of nitrogen molecule is close to :
(Given : nitrogen molecule weight : 4.64 $$ \times $$ 10–26 kg,
Boltzman constant: 1.38 $$ \times $$ 10–23 J/K,
Planck constant : 6.63 $$ \times $$ 10–34 J.s)
(Given : nitrogen molecule weight : 4.64 $$ \times $$ 10–26 kg,
Boltzman constant: 1.38 $$ \times $$ 10–23 J/K,
Planck constant : 6.63 $$ \times $$ 10–34 J.s)
2
The linear mass density of a thin rod AB of length L varies from A to B as
$$\lambda \left( x \right) = {\lambda _0}\left( {1 + {x \over L}} \right)$$, where x is the distance from A. If M is the mass of the rod then its moment of inertia about an axis passing through A and perpendicular to the rod is :
$$\lambda \left( x \right) = {\lambda _0}\left( {1 + {x \over L}} \right)$$, where x is the distance from A. If M is the mass of the rod then its moment of inertia about an axis passing through A and perpendicular to the rod is :
3
Three rods of identical cross-section and lengths are made of three different materials of thermal
conductivity K1
, K2
and K3
, respecrtively. They are joined together at their ends to make a long rod
(see figure). One end of the long rod is maintained at 100oC and the other at 0oC (see figure). If
the joints of the rod are at 70oC and 20oC in steady state and there is no loss of energy from the
surface of the rod, the correct relationship between K1
, K2
and K3
is :
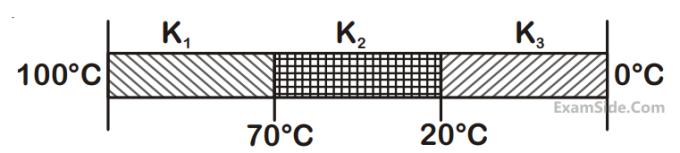

4
A fluid is flowing through a horizontal pipe of varying cross-section, with
speed v ms–1 at a point where the pressure is P pascal. At another point where pressure is $${P \over 2}$$ Pascal its speed is V ms–1. If the density of the fluid is $$\rho $$ kg m–3 and the flow is streamline, then V is equal to :
speed v ms–1 at a point where the pressure is P pascal. At another point where pressure is $${P \over 2}$$ Pascal its speed is V ms–1. If the density of the fluid is $$\rho $$ kg m–3 and the flow is streamline, then V is equal to :
5
When a particle of mass m is attached to a vertical spring of spring constant k and released, its
motion is described by
y(t) = y0 sin2 $$\omega $$t, where 'y' is measured from the lower end of unstretched spring. Then $$\omega $$ is:
y(t) = y0 sin2 $$\omega $$t, where 'y' is measured from the lower end of unstretched spring. Then $$\omega $$ is:
6
In a dilute gas at pressure P and temperature T, the mean time between successive collisions of a
molecule varies with T as :
7
A circuit to verify Ohm's law uses ammeter and voltmeter in series or parallel connected correctly
to the resistor. In the circuit :
8
A square loop of side 2$$a$$ and carrying current I is kept in xz plane with its centre at origin. A long
wire carrying the same current I is placed parallel to z-axis and passing through point (0, b, 0),
(b >> a). The magnitude of torque on the loop about z-axis will be :
9
A charged particle going around in a circle can be considered to be a current loop. A particle of
mass m carrying charge q is moving in a plane with speed v under the influence of magnetic field $$\overrightarrow B $$.
The magnetic moment of this moving particle:
10
A double convex lens has power P and same radii of curvature R of both the surfaces. The radius of
curvature of a surface of a plano-convex lens made of the same material with power 1.5 P is :
11
When a car is at rest, its driver sees rain drops falling on it vertically. When driving the car with
speed v, he sees that rain drops are coming at an angle 60° from the horizontal. On further
increasing the speed of the car to (1 + $$\beta $$)v, this angle changes to 45o. The value of $$\beta $$ is close to :
12
Particle A of mass m1
moving with velocity $$\left( {\sqrt3\widehat i + \widehat j} \right)m{s^{ - 1}}$$ collides with another particle B of mass m2
which is at rest initially. Let $$\overrightarrow {{V_1}} $$
and $$\overrightarrow {{V_2}} $$
be the velocities of particles A and B after collision
respectively. If m1
= 2m2
and after
collision $$\overrightarrow {{V_1}} = $$$$\left( {\widehat i + \sqrt 3 \widehat j} \right)$$ , the angle between $$\overrightarrow {{V_1}} $$ and $$\overrightarrow {{V_2}} $$ is :
collision $$\overrightarrow {{V_1}} = $$$$\left( {\widehat i + \sqrt 3 \widehat j} \right)$$ , the angle between $$\overrightarrow {{V_1}} $$ and $$\overrightarrow {{V_2}} $$ is :
13
A particle moving in the xy plane experiences a velocity dependent force
$$\overrightarrow F = k\left( {{v_y}\widehat i + {v_x}\widehat j} \right)$$ , where vx and vy are the
x and y components of its velocity $$\overrightarrow v $$ . If $$\overrightarrow a $$ is the
acceleration of the particle, then
which of the following statements is true for the particle?
$$\overrightarrow F = k\left( {{v_y}\widehat i + {v_x}\widehat j} \right)$$ , where vx and vy are the
x and y components of its velocity $$\overrightarrow v $$ . If $$\overrightarrow a $$ is the
acceleration of the particle, then
which of the following statements is true for the particle?
14
In a series LR circuit, power of 400W is dissipated from a source of 250 V, 50 Hz. The power factor
of the circuit is 0.8. In order to bring the power factor to unity, a capacitor of value C is added in
series to the L and R. Taking the value of C as $$\left( {{n \over {3\pi }}} \right)$$ $$\mu $$F, then value of n is __________.
15
A Young's double-slit experiment is performed using monochromatic light of wavelength $$\lambda $$. The
intensity of light at a point on the screen, where the path difference is $$\lambda $$, is K units. The intensity
of light at a point where the path difference is $${\lambda \over 6}$$ is given by $${{nK} \over {12}}$$, where n is an integer. The value
of n is __________.
16
The centre of mass of solid hemisphere of radius 8 cm is x from the centre of the flat surface. Then
value of x is __________.
17
For a plane electromagnetic wave, the magnetic field at a point x and time t is
$$\overrightarrow B \left( {x,t} \right)$$ = $$\left[ {1.2 \times {{10}^{ - 7}}\sin \left( {0.5 \times {{10}^3}x + 1.5 \times {{10}^{11}}t} \right)\widehat k} \right]$$ T
The instantaneous electric field $$\overrightarrow E $$ corresponding to $$\overrightarrow B $$ is :
(speed of light c = 3 × 108 ms–1)
$$\overrightarrow B \left( {x,t} \right)$$ = $$\left[ {1.2 \times {{10}^{ - 7}}\sin \left( {0.5 \times {{10}^3}x + 1.5 \times {{10}^{11}}t} \right)\widehat k} \right]$$ T
The instantaneous electric field $$\overrightarrow E $$ corresponding to $$\overrightarrow B $$ is :
(speed of light c = 3 × 108 ms–1)
18
Given the masses of various atomic particles
mp = 1.0072 u, mn = 1.0087 u, me = 0.000548 u,
$${m_{\overline v }}$$ = 0, md = 2.0141 u, where p $$ \equiv $$ proton, n $$ \equiv $$ neutron,
e $$ \equiv $$ electron, $$\overline v $$ $$ \equiv $$ antineutrino and d $$ \equiv $$ deuteron. Which of the following process is allowed by momentum and energy conservation?
mp = 1.0072 u, mn = 1.0087 u, me = 0.000548 u,
$${m_{\overline v }}$$ = 0, md = 2.0141 u, where p $$ \equiv $$ proton, n $$ \equiv $$ neutron,
e $$ \equiv $$ electron, $$\overline v $$ $$ \equiv $$ antineutrino and d $$ \equiv $$ deuteron. Which of the following process is allowed by momentum and energy conservation?
19
Two planets have masses M and 16 M and their radii are $$a$$ and 2$$a$$, respectively. The separation
between the centres of the planets is 10$$a$$. A body of mass m is fired from the surface of the larger
planet towards the smaller planet along the line joining their centres. For the body to be able to
reach at the surface of smaller planet, the minimum firing speed needed is :
20
Consider the force F on a charge 'q' due to a uniformly charged spherical shell of radius R carrying
charge Q distributed uniformly over it. Which one of the following statements is true for F, if 'q' is
placed at distance r from the centre of the shell?
21
In the figure shown, the current in the 10 V battery is close to :
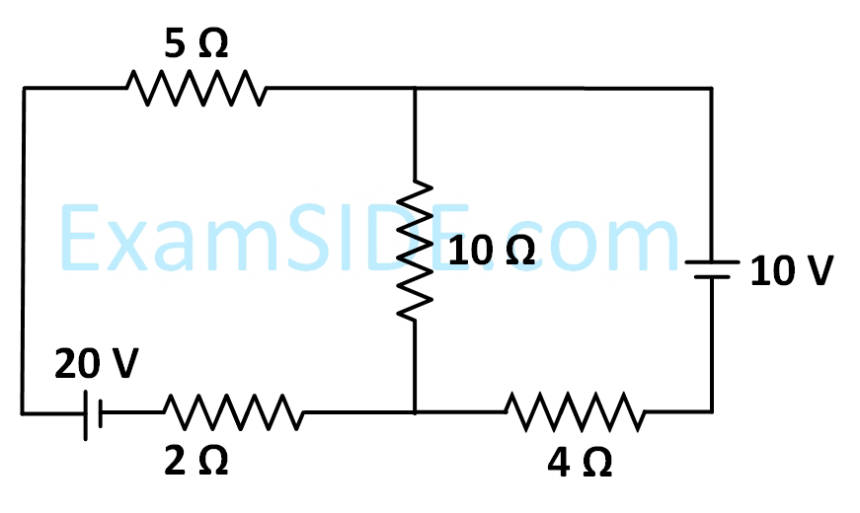

22
A student measuring the diameter of a pencil of circular cross-section with the help of a vernier
scale records the following four readings 5.50 mm, 5.55 mm, 5.45 mm, 5.65 mm. The average of
these four readings is 5.5375 mm and the standard deviation of the data is 0.07395 mm. The
average diameter
of the pencil should therefore be recorded as :
of the pencil should therefore be recorded as :
23
Two identical electric point dipoles have dipole moments $${\overrightarrow p _1} = p\widehat i$$ and $${\overrightarrow p _2} = - p\widehat i$$ and are held on the x
axis at distance '$$a$$' from each other. When released, they move along the x-axis with the direction
of their dipole moments remaining unchanged. If the mass of each dipole is 'm', their speed when
they are infinitely far apart is :