JEE Main 2017 (Online) 8th April Morning Slot
Paper was held on
Sat, Apr 8, 2017 3:30 AM
Chemistry
1
The major product of the following reaction is :


2
The major product of the following reaction is :


3
The IUPAC name of the following compound is :


4
The major product expected from the following reaction is :
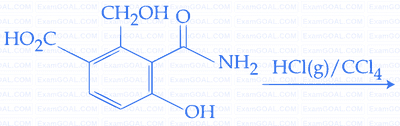

5
Which of the following compounds will not undergo Friedel Craft’s reaction with benzene ?
6
Among the following, the essential amino acid is :
7
A solution containing a group-IV cation gives a precipitate on passing H2S. A solution of this precipitate in dil.HCl produces a white precipitate with NaOH solution and bluish-white precipitate with basic potassium ferrocyanide. The cation is :
8
A mixture containing the following four compounds is extracted with $$1M$$ $$HCl.$$ The compound that goes to aqueous layer is :


9
Which of the following statements is not true about partition chromatography?
10
Consider the following standard electrode potentials (Eo in volts) in aqueous solution :
Based on these data, which of the following statements is correct ?
| Element | M3+ /M | M+ /M |
|---|---|---|
| A1 | -1.66 | + 0.55 |
| T1 | +1.26 | - 0.34 |
Based on these data, which of the following statements is correct ?
11
Consider the following ionization enthalpies of two elements 'A' and 'B' .
Which of the following statements is correct ?
| Element | Ionization enthalpy (kJ/mol) | ||
|---|---|---|---|
| 1st | 2nd | 3rd | |
| A | 899 | 1757 | 14847 |
| B | 737 | 1450 | 7731 |
Which of the following statements is correct ?
12
The major product of the following reaction is :


13
A metal ‘M’ reacts with nitrogen gas to afford ‘M3N’. ‘M3N’ on heating at high temperature gives back ‘M’ and on
reaction with water produces a gas ‘B’. Gas ‘B’ reacts with aqueous
solution of CuSO4 to form a deep blue compound. ‘M’ and
‘B’ respectively are :
14
What is the standard reduction potential (Eo) for Fe3+ $$ \to $$ Fe ?
Given that :
Fe2+ + 2e$$-$$ $$ \to $$ Fe; $$E_{F{e^{2 + }}/Fe}^o$$ = $$-$$0.47 V
Fe3+ + e$$-$$ $$ \to $$ Fe2+; $$E_{F{e^{3 + }}/F{e^{2 + }}}^o$$ = +0.77 V
Given that :
Fe2+ + 2e$$-$$ $$ \to $$ Fe; $$E_{F{e^{2 + }}/Fe}^o$$ = $$-$$0.47 V
Fe3+ + e$$-$$ $$ \to $$ Fe2+; $$E_{F{e^{3 + }}/F{e^{2 + }}}^o$$ = +0.77 V
15
sp3d2 hybridization is not displayed by :
16
Which of the following is paramagnetic ?
17
The enthalpy change on freezing of 1 mol of water at 5oC to ice at −5oC is :
(Given $$\Delta $$fusH = 6 kJ mol$$-$$1 at 0oC,
Cp(H2O, $$\ell $$ = 75.3J mol$$-$$1 K$$-$$1)
Cp(H2O s) =36.8 J mol$$-$$1 K$$-$$1)
(Given $$\Delta $$fusH = 6 kJ mol$$-$$1 at 0oC,
Cp(H2O, $$\ell $$ = 75.3J mol$$-$$1 K$$-$$1)
Cp(H2O s) =36.8 J mol$$-$$1 K$$-$$1)
18
The pair of compounds having metals in their highest oxidation state is :
19
The rate of a reaction A doubles on increasing the temperature from 300 to 310 K. By how much, the temperature of reaction B should be Increased from 300 K so that rate doubles if activation energy of the reaction B is twice to that of reaction A.
20
Additin of sodium hydroxide solution to a weak acid (HA)results in a buffer of pH 6. If ionition constant of HA is 10$$-$$5, the ratio of salt to acid concentration in the buffer solution will be :
21
5 g of Na2SO4 was dissolved in x g of H2O. The change in freezing point was found to be
3.82oC. If Na2SO4 is 81.5% ionised,
the value of x
(Kf for water=1.86oC kg mol−1) is approximately :
(molar mass of S = 32 g mol−1 and that of Na = 23 g mol−1)
(Kf for water=1.86oC kg mol−1) is approximately :
(molar mass of S = 32 g mol−1 and that of Na = 23 g mol−1)
22
If the shortest wavelength in Lyman series of hydrogen atom is A, then the longest wavelength in Paschen series of He+ is :
23
For a reaction, A(g) $$ \to $$ A($$\ell $$); $$\Delta $$H= $$-$$ 3RT.
The correct statement for the reaction is :
The correct statement for the reaction is :
24
Excess of NaOH (aq) was added to 100 mL of FeCl3 (aq) resulting into 2.14 g of Fe(OH)3 . The molarity of FeCl3 (aq) is :
(Given molar mass of Fe = 56 g mol−1 and molar mass of Cl = 35.5 g mol−1)
(Given molar mass of Fe = 56 g mol−1 and molar mass of Cl = 35.5 g mol−1)
Mathematics
1
$$\mathop {\lim }\limits_{x \to 3} $$ $${{\sqrt {3x} - 3} \over {\sqrt {2x - 4} - \sqrt 2 }}$$ is equal to :
2
If all the words, with or without meaning, are written using the letters of the word QUEEN and are arranged as in English dictionary, then the position of the word QUEEN is :
3
If the arithmetic mean of two numbers a and b, a > b > 0, is five times their geometric mean, then $${{a + b} \over {a - b}}$$ is equal to :
4
If (27)999 is divided by 7, then the remainder is :
5
Let f(x) = 210.x + 1 and g(x)=310.x $$-$$ 1. If (fog) (x) = x, then x is equal to :
6
The number of real values of $$\lambda $$ for which the system of linear equations
2x + 4y $$-$$ $$\lambda $$z = 0
4x + $$\lambda $$y + 2z = 0
$$\lambda $$x + 2y + 2z = 0
has infinitely many solutions, is :
2x + 4y $$-$$ $$\lambda $$z = 0
4x + $$\lambda $$y + 2z = 0
$$\lambda $$x + 2y + 2z = 0
has infinitely many solutions, is :
7
Let A be any 3 $$ \times $$ 3 invertible matrix. Then which one of the following is not always true ?
8
Let p(x) be a quadratic polynomial such that p(0)=1. If p(x) leaves remainder 4 when divided by x$$-$$ 1 and it leaves remainder 6 when divided by x + 1; then :
9
Let z$$ \in $$C, the set of complex numbers. Then the equation, 2|z + 3i| $$-$$ |z $$-$$ i| = 0 represents :
10
Consider an ellipse, whose center is at the origin and its major axis is along the x-axis. If its eccentricity is $${3 \over 5}$$ and the distance between its foci is 6, then the area (in sq. units) of the quadrilatateral inscribed in the ellipse, with the vertices as the vertices of the ellipse, is :
11
The value of tan-1 $$\left[ {{{\sqrt {1 + {x^2}} + \sqrt {1 - {x^2}} } \over {\sqrt {1 + {x^2}} - \sqrt {1 - {x^2}} }}} \right],$$ $$\left| x \right| < {1 \over 2},x \ne 0,$$ is equal to :
12
If
$$S = \left\{ {x \in \left[ {0,2\pi } \right]:\left| {\matrix{ 0 & {\cos x} & { - \sin x} \cr {\sin x} & 0 & {\cos x} \cr {\cos x} & {\sin x} & 0 \cr } } \right| = 0} \right\},$$
then $$\sum\limits_{x \in S} {\tan \left( {{\pi \over 3} + x} \right)} $$ is equal to :
$$S = \left\{ {x \in \left[ {0,2\pi } \right]:\left| {\matrix{ 0 & {\cos x} & { - \sin x} \cr {\sin x} & 0 & {\cos x} \cr {\cos x} & {\sin x} & 0 \cr } } \right| = 0} \right\},$$
then $$\sum\limits_{x \in S} {\tan \left( {{\pi \over 3} + x} \right)} $$ is equal to :
13
Three persons P, Q and R independently try to hit a target. I the probabilities of their hitting the target are $${3 \over 4},{1 \over 2}$$ and $${5 \over 8}$$ respectively, then the probability that the target is hit by P or Q but not by R is :
14
An unbiased coin is tossed eight times. The probability of obtaining at least one head and at least one tail is :
15
The mean age of 25 teachers in a school is 40 years. A teacher retires at the age of 60 years and a new teacher is appointed in his place. If now the mean age of the teachers in this school is 39 years, then the age (in years) of the newly appointed teacher is :
16
The area (in sq. units) of the parallelogram whose diagonals are along the vectors $$8\widehat i - 6\widehat j$$ and $$3\widehat i + 4\widehat j - 12\widehat k,$$ is :
17
If two parallel chords of a circle, having diameter 4units, lie on the opposite sides of the center and subtend angles $${\cos ^{ - 1}}\left( {{1 \over 7}} \right)$$ and sec$$-$$1 (7) at the center respectivey, then the distance between these chords, is :
18
The area (in sq. units) of the smaller portion enclosed between the curves, x2 + y2 = 4 and y2 = 3x, is :
19
The integral $$\int_{{\pi \over {12}}}^{{\pi \over 4}} {\,\,{{8\cos 2x} \over {{{\left( {\tan x + \cot x} \right)}^3}}}} \,dx$$ equals :
20
The locus of the point of intersection of the straight lines,
tx $$-$$ 2y $$-$$ 3t = 0
x $$-$$ 2ty + 3 = 0 (t $$ \in $$ R), is :
tx $$-$$ 2y $$-$$ 3t = 0
x $$-$$ 2ty + 3 = 0 (t $$ \in $$ R), is :
21
The curve satisfying the differential equation, ydx $$-$$(x + 3y2)dy = 0 and passing through the point (1, 1), also passes through the point :
22
If a point P has co-ordinates (0, $$-$$2) and Q is any point on the circle, x2 + y2 $$-$$ 5x $$-$$ y + 5 = 0, then the maximum value of (PQ)2 is :
23
If y = $${\left[ {x + \sqrt {{x^2} - 1} } \right]^{15}} + {\left[ {x - \sqrt {{x^2} - 1} } \right]^{15}},$$
then (x2 $$-$$ 1) $${{{d^2}y} \over {d{x^2}}} + x{{dy} \over {dx}}$$ is equal to :
then (x2 $$-$$ 1) $${{{d^2}y} \over {d{x^2}}} + x{{dy} \over {dx}}$$ is equal to :
24
The integral
$$\int {\sqrt {1 + 2\cot x(\cos ecx + \cot x)\,} \,\,dx} $$
$$\left( {0 < x < {\pi \over 2}} \right)$$ is equal to :
(where C is a constant of integration)
$$\int {\sqrt {1 + 2\cot x(\cos ecx + \cot x)\,} \,\,dx} $$
$$\left( {0 < x < {\pi \over 2}} \right)$$ is equal to :
(where C is a constant of integration)
Physics
1
There is a uniform electrostatic field in a region. The potential at various points on a small sphere centred at $$P,$$ in the region, is found to vary between the limits 589.0 V to 589.8 V. What is the potential at a point on the sphere whose radius vector makes an angle of 60o with the direction of the field ?
2
A single slit of width b is illuminated by a coherent monochromatic light of wavelength $$\lambda $$. If the second and fourthminima in the diffraction pattern at a distance 1 m from the slit are at 3 cm and 6 cm respectively from the central maximum, what is the width of the central maximum ? (i.e. distance between first minimum on either side of the central maximum)
3
Let the refractive index of a denser medium with respect to a rarer medium be n12 and its critical angle be θC . At an angle of incidence A when light is travelling from
denser medium to rarer medium, a part of the light is reflected and the rest is refracted and the angle between reflected and refracted rays is 90o. Angle A is given by :
4
Magnetic field in a plane electromagnetic wave is given by
$$\overrightarrow B $$ = B0 sin (k x + $$\omega $$t) $$\widehat j\,T$$
Expression for corresponding electric field will be :
Where c is speed of light.
$$\overrightarrow B $$ = B0 sin (k x + $$\omega $$t) $$\widehat j\,T$$
Expression for corresponding electric field will be :
Where c is speed of light.
5
What is the conductivity of a semiconductor sale having electron concentration of $$5 \times {10^{18}}\,\,{m^{ - 3}},$$ hole concentration of $$5 \times {10^{19}}\,\,{m^{ - 3}},$$ electron mobility of 2.0 m2 V$$-$$1 s-1 and hole mobility of 0.01 m2 V$$-$$1 s$$-$$1 ?
(Take charge of electronas 1.6 $$ \times $$ 10 $$-$$19 c)
(Take charge of electronas 1.6 $$ \times $$ 10 $$-$$19 c)
6
A small circular loop of wire of radius a is located at the centre of a much larger circular wire loop of radius b. The two loops are in the same plane. The outer loop of radius b carries an alternating current I = Io cos ($$\omega $$t). The emf induced in the smaller inner loop is nearly :
7
A uniform disc of radius R and mass M is free to rotate only about its axis. A string is wrapped over its rim and a body of mass m is tied to the free end of the string as shown in the figure. The body is released from rest. Then the acceleration of the body is :
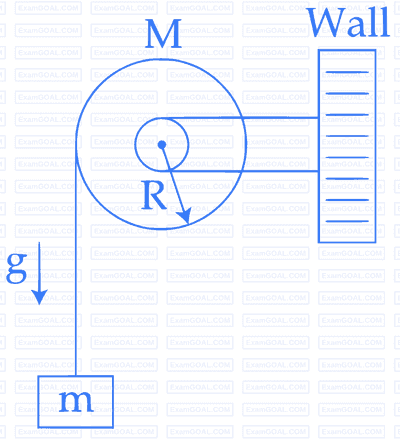

8
In a physical balance working on the principle of moments, when 5 mg weight is placed on the left pan, the beam becomes horizontal. Both the empty pans of the balance are of equal mass. Which of the following statements is correct ?
9
In a certain region static electric and magnetic fields exist. The magnetic field is given by $$\overrightarrow B = {B_0}\left( {\widehat i + 2\widehat j - 4\widehat k} \right)$$ . If a test charge moving with a velocity $$\overrightarrow \upsilon = {\upsilon _0}\left( {3\widehat i - \widehat j + 2\widehat k} \right)$$ experiences no force in that region, then the electric field in the region, in SI units, is :
10
A magnetic dipole in a constant magnetic field has :
11
The energy stored in the electric field produced by a metal sphere is 4.5 J. If the sphere contains 4 $$\mu $$C charge, its radius will be :
[ Take : $${1 \over {4\,\pi { \in _0}}} = $$ 9 $$ \times $$ 109 N $$-$$ m2/C2 ]
[ Take : $${1 \over {4\,\pi { \in _0}}} = $$ 9 $$ \times $$ 109 N $$-$$ m2/C2 ]
12
The maximum velocity of the photoelectrons emitted from the surface is v when light of frequency n falls on a metal surface. If the incident frequency is increased to 3n, the maximum velocity of the ejected photoelectrons will be :
13
Two wires W1 and W2 have the same radius r and respective densities $$\rho $$1 and $$\rho $$2 such that ρ2 = 4$$\rho $$1 . They are joined together at the point O, as shown in the figure. The combination is used as a sonometer wire and kept under tension T. The point O is midway between the two bridges. When a stationary wave is set up in the composite wire, the joint is found to be a node. The ratio of the number of antinodes formed in W1 to W2
is :
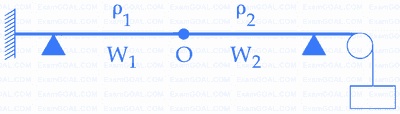

14
An ideal gas has molecules with 5 degrees of freedom. The ratio of specific heats at constant pressure (Cp ) and at constant volume (Cv) is :
15
The ratio of maximum acceleration to maximum velocity in a simple harmonic motion is 10 s−1 . At, t = 0 the displacement is 5 m. What is the maximum acceleration ? The initial phase is $${\pi \over 4}$$.
16
A compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temperature increases by $$\Delta $$T. The net change in its length is zero. Let $$\ell $$ be the length of the rod, A its area of cross-section,Y its Young’s modulus, and $$\alpha $$ its coefficient of linear expansion. Then, F is equal to :
17
An object is dropped from a height h from the ground. Every time it hits the ground it looses 50% of its kinetic energy. The total distance covered as t $$ \to $$ $$\infty $$ is :
18
In an experiment, a sphere of aluminium of mass 0.20 kg is heated upto 150oC. Immediately, it is put into water of volume 150 cc at 27oC kept in a calorimeter of water equivalent to 0.025 kg. Final temperature of the system is 40oC. The specific heat of aluminium is : (take 4.2 Joule = 1 calorie)
19
Moment of inertia of an equilateral triangular lamina ABC, about the axis
passing through its centre O and perpendicular to its plane is Io as shown in the figure. A cavity DEF is cut out from the lamina, where D, E, F are the mid points of the sides. Moment of inertia of the remaining part of lamina about the same axis is :
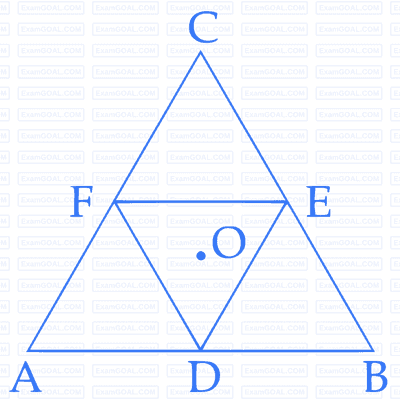

20
A 1 kg block attached to a spring vibrates with a frequency of 1 Hz on a frictionless horizontal table. Two springs identical to the original spring are attached in parallel to an 8 kg block placed on the same table. So, the frequency of vibration of the 8 kg block is :
21
Which graph corresponds to an object moving with a constant negative
acceleration and a positive velocity ?
22
Time (T), velocity (C) and angular momentum (h) are chosen as fundamentalquantities instead of mass, length and time. In terms of these, the dimensions of mass would be :
23
If the Earth has no rotational motion, the weight of a person on the equator is W. Determine the speed with which the earth would have to rotate about its axis so that the person at the equator will weigh $${3 \over 4}$$ W. Radius of the Earth is 6400 km and g=10 m/s2.
24

A 9 V battery with internal resistance of 0.5 $$\Omega $$ is connected across an infinite network as shown in the figure. All ammeters A1, A2, 3 and voltmeter V are ideal.
Choose correct statement.
25
The V-I characteristic of a diode is shown in the figure. The ratio of forward to reverse bias
resistance is :


26
Two deuterons undergo nuclear fusion to form a Helium nucleus. Energy released in this process is : (given binding energy per nucleon for deuteron = 1.1 MeV and for helium = 7.0 MeV)
27
According to Bohr’s theory, the time averaged magnetic field at the centre (i.e. nucleus) of a hydrogen atom due to the motion of electrons in the nth orbit is proportional to : (n = principal quantum number)