AIEEE 2006
Paper was held on
Sat, Apr 29, 2006 9:30 AM
Chemistry
1
The standard enthalpy of formation $$\Delta _fH^o$$ at 298 K for methane, CH4(g), is –74.8 kJ mol–1. The additional information required to determine the average energy for C – H bond formation would be :
2
An ideal gas is allowed to expand both reversibly and irreversibly in an isolated system. If Ti is the
initial temperature and Tf is the final temperature, which of the following statements is correct?
3
The enthalpy changes for the following processes are listed below :
Cl2(g) = 2Cl(g), 242.3 kJ mol–1
I2(g) = 2I(g), 151.0 kJ mol–1
ICl(g) = I(g) + Cl(g), 211.3 kJ mol–1
I2(s) = I2(g), 62.76 kJ mol–1
Given that the standard states for iodine and chlorine are I2(s) and Cl2(g), the standard enthalpy of formation for ICl(g) is :
Cl2(g) = 2Cl(g), 242.3 kJ mol–1
I2(g) = 2I(g), 151.0 kJ mol–1
ICl(g) = I(g) + Cl(g), 211.3 kJ mol–1
I2(s) = I2(g), 62.76 kJ mol–1
Given that the standard states for iodine and chlorine are I2(s) and Cl2(g), the standard enthalpy of formation for ICl(g) is :
4
($$\Delta H - \Delta U$$) for the formation of carbon monoxide (CO) from its elements at 298 K is : (R = 8.314 J K–1 mol–1)
5
Phosphorus pentachloride dissociates as follows, in a closed reaction vessel
PCl5 (g) $$\leftrightharpoons$$ PCl3 (g) + Cl2 (g)
If total pressure at equilibrium of the reaction mixture is P and degree of dissociation of PCl5 is x, the partial pressure of PCl3 will be
PCl5 (g) $$\leftrightharpoons$$ PCl3 (g) + Cl2 (g)
If total pressure at equilibrium of the reaction mixture is P and degree of dissociation of PCl5 is x, the partial pressure of PCl3 will be
6
The equilibrium constant for the reaction
SO3 (g) $$\leftrightharpoons$$ SO2 (g) + $$1 \over 2$$ O2 (g)
is Kc = 4.9 $$\times$$ 10–2. The value of Kc for the reaction
2SO2 (g) + O2 (g) $$\leftrightharpoons$$ 2SO3 (g) will be :
SO3 (g) $$\leftrightharpoons$$ SO2 (g) + $$1 \over 2$$ O2 (g)
is Kc = 4.9 $$\times$$ 10–2. The value of Kc for the reaction
2SO2 (g) + O2 (g) $$\leftrightharpoons$$ 2SO3 (g) will be :
7
Given the data at 25oC,
Ag + I- $$\to$$ AgI + e- , Eo = 0.152 V
Ag $$\to$$ Ag+ + e-, Eo = -0.800 V
What is the value of log Ksp for AgI? (2.303 RT/F = 0.059 V)
Ag + I- $$\to$$ AgI + e- , Eo = 0.152 V
Ag $$\to$$ Ag+ + e-, Eo = -0.800 V
What is the value of log Ksp for AgI? (2.303 RT/F = 0.059 V)
8
CH3Br + Nu- $$\to$$ CH3 - Nu + Br- The decreasing order of the rate of the above reaction with nucleophiles (Nu–) A to D is
[Nu– = (A) PhO–, (B) AcO–, (C) HO–, (D) CH3O–]
[Nu– = (A) PhO–, (B) AcO–, (C) HO–, (D) CH3O–]
9
The increasing order of stability of the following free radicals is
10
Increasing order of stability among the three main conformations (i.e. Eclipse, Anti, Gauche) of
2-fluoroethanol is
11
How many EDTA (ethylenediaminetetraacetic acid) molecules are required to make an octahedral
complex with a Ca2+ ion?
12
Fluorobenzene (C6H5F) can be synthesized in the laboratory
13
Phenyl magnesium bromide reacts with methanol to give
14
Reaction of trans-2-phenyl-1-bromocyclopentane on reaction with alcoholic KOH produces
15
HBr reacts with CH2 = CH – OCH3 under anhydrous conditions at room temperature to give
16
The increasing order of the rate of HCN addition to compounds A – D is
(A) HCHO
(B) CH3COCH3
(C) PhCOCH3
(D) PhCOPh
(A) HCHO
(B) CH3COCH3
(C) PhCOCH3
(D) PhCOPh
17
The term anomers of glucose refers to
18
The pyrimidine bases present in DNA are
19
In Fe(CO)5, the Fe – C bond possesses :
20
The $$IUPAC$$ name of the compound shown below is :
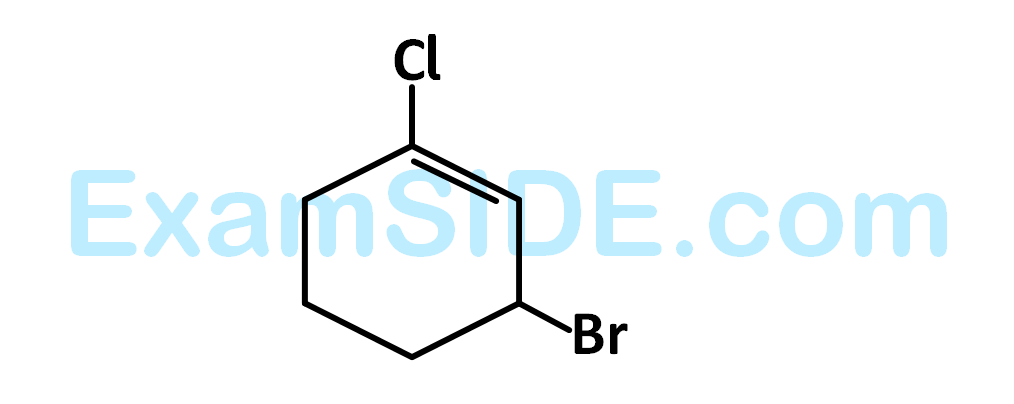

21
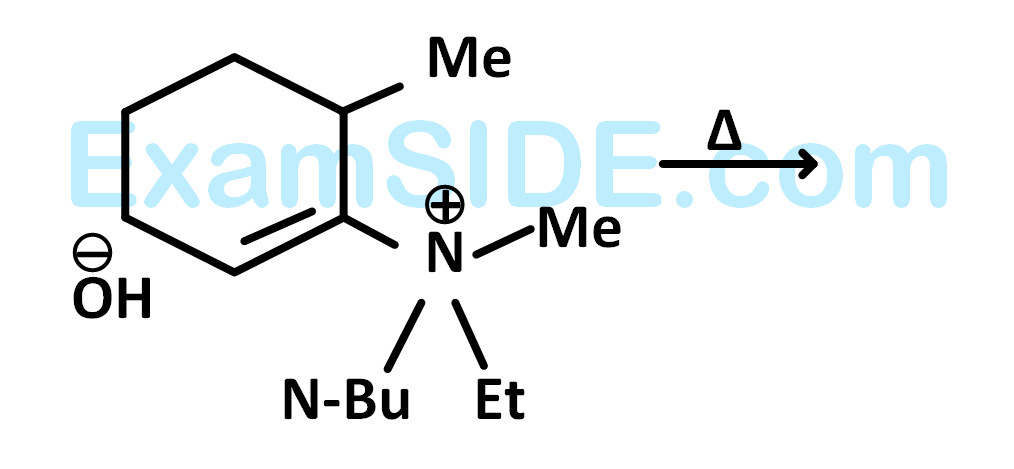
The alkene formed as a major product in the above elimination reaction is
22
The structure of the major product formed in the following reaction
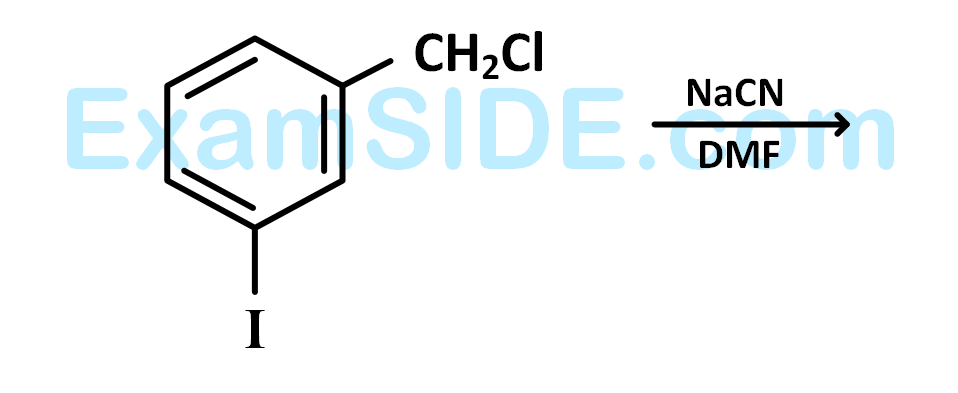
is

is
23
The structure of the compound that gives a tribromo derivative on treatment with bromine water is
24
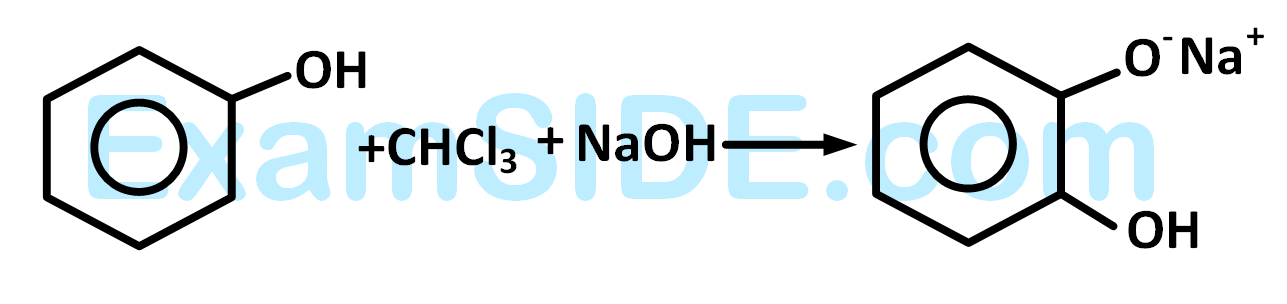
The electrophile involved in the above reaction is
25
Among the following the one that gives positives iodoform test upon reaction with $${I_2}$$ and $$NaOH$$ is
26
The correct order of increasing acid strength of the compounds


27
A metal, $$M$$ forms chlorides in its $$+2$$ and $$+4$$ oxidation states. Which of the following statements about these chlorides is correct?
28
In the transformation of $${}_{92}^{238}U$$ to $${}_{92}^{234}U$$, if one emission is an α-particle, what should be the other
emission(s)?
29
Among the following mixtures, dipole-dipole as the major interaction, is present in
30
18 g of glucose (C6H12O6) is added to 178.2 g of water. The vapour pressure of water for this aqueous solution at 100oC is
31
Which of the following chemical reactions depicts the oxidizing behaviour of H2SO4?
32
The molar conductivities $$ \wedge _{NaOAc}^o$$ and $$ \wedge _{HCl}^o$$ and at infinite dilution in water at 25oC are 91.0 and 426.2 Scm2/mol respectively. To calculate $$ \wedge _{HOAc}^o$$ , the additional value required is
33
Resistance of a conductivity cell filled with a solution of an electrolyte of concentration 0.1 M is 100$$\Omega $$.
The conductivity of this solution is 1.29 S m–1. Resistance of the same cell when filled with 0.2 M of
the same solution is 520 $$\Omega $$, The molar conductivity of 0.02 M solution of the electrolyte will be
34
Rate of a reaction can be expressed by Arrhenius equation as:
$$$k = A\,{e^{ - E/RT}}$$$ In this equation, E represents
$$$k = A\,{e^{ - E/RT}}$$$ In this equation, E represents
35
A reaction was found to be second order with respect to the concentration of carbon monoxide. If the
concentration of carbon monoxide is doubled, with everything else kept the same, the rate of reaction
will
36
The following mechanism has been proposed for the reaction of NO with Br2 to form NOBr:
NO(g) + Br2 (g) $$\leftrightharpoons$$ NOBr2 (g)
NOBr2 (g) + NO (g) $$\to$$ 2NOBr (g)
If the second step is the rate determining step, the order of the reaction with respect to NO(g) is
NO(g) + Br2 (g) $$\leftrightharpoons$$ NOBr2 (g)
NOBr2 (g) + NO (g) $$\to$$ 2NOBr (g)
If the second step is the rate determining step, the order of the reaction with respect to NO(g) is
37
The increasing order of the first ionization enthalpies of the elements B, P, S and F (lowest first) is :
38
Which of the following statements is true?
39
The “spin-only” magnetic moment [in units of Bohr magneton, (µB)] of Ni2+ in aqueous solution would
be : (Atomic number of Ni = 28)
40
Lanthanoid contraction is caused due to :
41
Nickel (Z = 28) combines with a uninegative monodentate ligand X– to form a paramagnetic complex [NiX4]2− . The number of unpaired electron(s) in the nickel and geometry of this complex ion are,
respectively
42
The IUPAC name for the complex [Co(NO2)(NH3)5]Cl2 is
43
How many moles of magnesium phosphate Mg3(PO4)2 will contain 0.25 mole of oxygen atoms?
44
Density of a 2.05M solution of acetic acid in water is 1.02 g/mL The molality of the solution is
45
Uncertainty in the position of an electron (mass = 9.1 $$\times$$ 10-31 kg) moving with a velocity 300 ms-1, accurate upto 0.001% will be (h = 6.63 $$\times$$ 10-34 Js)
46
According to Bohr's theory, the angular momentum of an electron in 5th orbit is
47
Which of the following sets of ions represents a collection of isoelectronic species?
48
Following statements regarding the periodic trends of chemical reactivity of the alkali metals and the
halogens are given. Which of these statements gives the correct picture?
49
Which of the following molecules/ions does not contain unpaired electrons?
50
In which of the following molecules/ions are all the bonds not equal?
51
The decreasing values of bond angles from NH3 (106o) to SbH3 (91o
) down group-15 of the periodic table is due to
Mathematics
1
Suppose a population A has 100 observations 101, 102,........, 200, and another
population B has 100 observations 151, 152,......., 250. If VA and VB represent the
variances of the two populations, respectively, then $${{{V_A}} \over {{V_B}}}$$ is
2
The value of $$\int\limits_1^a {\left[ x \right]} f'\left( x \right)dx,a > 1$$ where $${\left[ x \right]}$$ denotes the greatest integer not exceeding $$x$$ is
3
The set of points where $$f\left( x \right) = {x \over {1 + \left| x \right|}}$$ is differentiable is
4
If $${x^m}.{y^n} = {\left( {x + y} \right)^{m + n}},$$ then $${{{dy} \over {dx}}}$$ is
5
The function $$f\left( x \right) = {x \over 2} + {2 \over x}$$ has a local minimum at
6
A triangular park is enclosed on two sides by a fence and on the third side by a straight river bank. The two sides having fence are of same length $$x$$. The maximum area enclosed by the park is
7
If $$A$$ and $$B$$ are square matrices of size $$n\, \times \,n$$ such that
$${A^2} - {B^2} = \left( {A - B} \right)\left( {A + B} \right),$$ then which of the following will be always true?
$${A^2} - {B^2} = \left( {A - B} \right)\left( {A + B} \right),$$ then which of the following will be always true?
8
Let $$A = \left( {\matrix{
1 & 2 \cr
3 & 4 \cr
} } \right)$$ and $$B = \left( {\matrix{
a & 0 \cr
0 & b \cr
} } \right),a,b \in N.$$ Then
9
$$\int\limits_0^\pi {xf\left( {\sin x} \right)dx} $$ is equal to
10
$$\int\limits_{ - {{3\pi } \over 2}}^{ - {\pi \over 2}} {\left[ {{{\left( {x + \pi } \right)}^3} + {{\cos }^2}\left( {x + 3\pi } \right)} \right]} dx$$ is equal to
11
The differential equation whose solution is $$A{x^2} + B{y^2} = 1$$
where $$A$$ and $$B$$ are arbitrary constants is of
where $$A$$ and $$B$$ are arbitrary constants is of
12
At a telephone enquiry system the number of phone cells regarding relevant enquiry follow Poisson distribution with an average of $$5$$ phone calls during $$10$$ minute time intervals. The probability that there is at the most one phone call during a $$10$$-minute time period is :
13
The values of a, for which the points $$A, B, C$$ with position vectors $$2\widehat i - \widehat j + \widehat k,\,\,\widehat i - 3\widehat j - 5\widehat k$$ and $$a\widehat i - 3\widehat j + \widehat k$$ respectively are the vertices of a right angled triangle with $$C = {\pi \over 2}$$ are :
14
The two lines $$x=ay+b, z=cy+d;$$ and $$x=a'y+b' ,$$ $$z=c'y+d'$$ are perpendicular to each other if :
15
If $$0 < x < \pi $$ and $$\cos x + \sin x = {1 \over 2},$$ then $$\tan x$$ is :
16
If $${z^2} + z + 1 = 0$$, where z is complex number, then value of $${\left( {z + {1 \over z}} \right)^2} + {\left( {{z^2} + {1 \over {{z^2}}}} \right)^2} + {\left( {{z^3} + {1 \over {{z^3}}}} \right)^2} + .......... + {\left( {{z^6} + {1 \over {{z^6}}}} \right)^2}$$ is :
17
The value of $$\sum\limits_{k = 1}^{10} {\left( {\sin {{2k\pi } \over {11}} + i\,\,\cos {{2k\pi } \over {11}}} \right)} $$ is :
18
If the roots of the quadratic equation $${x^2} + px + q = 0$$ are $$\tan {30^ \circ }$$ and $$\tan {15^ \circ }$$, respectively, then the value of $$2 + q - p$$ is
19
All the values of $$m$$ for which both roots of the equation $${x^2} - 2mx + {m^2} - 1 = 0$$ are greater than $$ - 2$$ but less then 4, lie in the interval
20
If $$x$$ is real, the maximum value of $${{3{x^2} + 9x + 17} \over {3{x^2} + 9x + 7}}$$ is
21
At an election, a voter may vote for any number of candidates, not greater than the number to be elected. There are 10 candidates and 4 are of be selected, if a voter votes for at least one candidate, then the number of ways in which he can vote is
22
If the expansion in powers of $$x$$ of the function $${1 \over {\left( {1 - ax} \right)\left( {1 - bx} \right)}}$$ is $${a_0} + {a_1}x + {a_2}{x^2} + {a_3}{x^3}.....$$ then $${a_n}$$ is
23
If $${{a_1},{a_2},....{a_n}}$$ are in H.P., then the expression $${{a_1}\,{a_2} + \,{a_2}\,{a_3}\, + .... + {a_{n - 1}}\,{a_n}}$$ is equal to
24
Let $${a_1}$$, $${a_2}$$, $${a_3}$$.....be terms on A.P. If $${{{a_1} + {a_2} + .....{a_p}} \over {{a_1} + {a_2} + .....{a_q}}} = {{{p^2}} \over {{q^2}}},\,p \ne q,\,then\,{{{a_6}} \over {{a_{21}}}}\,$$ equals
25
A straight line through the point $$A (3, 4)$$ is such that its intercept between the axes is bisected at $$A$$. Its equation is :
26
If $$\left( {a,{a^2}} \right)$$ falls inside the angle made by the lines $$y = {x \over 2},$$ $$x > 0$$ and $$y = 3x,$$ $$x > 0,$$ then a belong to :
27
If the lines $$3x - 4y - 7 = 0$$ and $$2x - 3y - 5 = 0$$ are two diameters of a circle of area $$49\pi $$ square units, the equation of the circle is :
28
Let $$C$$ be the circle with centre $$(0, 0)$$ and radius $$3$$ units. The equation of the locus of the mid points of the chords of the circle $$C$$ that subtend an angle of $${{2\pi } \over 3}$$ at its center is :
29
In the ellipse, the distance between its foci is $$6$$ and minor axis is $$8$$. Then its eccentricity is :
30
The locus of the vertices of the family of parabolas
$$y = {{{a^3}{x^2}} \over 3} + {{{a^2}x} \over 2} - 2a$$ is :
$$y = {{{a^3}{x^2}} \over 3} + {{{a^2}x} \over 2} - 2a$$ is :
31
Let $W$ denote the words in the English dictionary. Define the relation $R$ by
$R=\{(x, y) \in W \times W \mid$ the words $x$ and $y$ have at least one letter in common}. Then, $R$ is
$R=\{(x, y) \in W \times W \mid$ the words $x$ and $y$ have at least one letter in common}. Then, $R$ is
Physics
1
The $$rms$$ value of the electric field of the light coming from the Sun is $$720$$ $$N/C.$$ The average total energy density of the electromagnetic wave is
2
The circuit has two oppositively connected ideal diodes in parallel. What is the current following in the circuit?
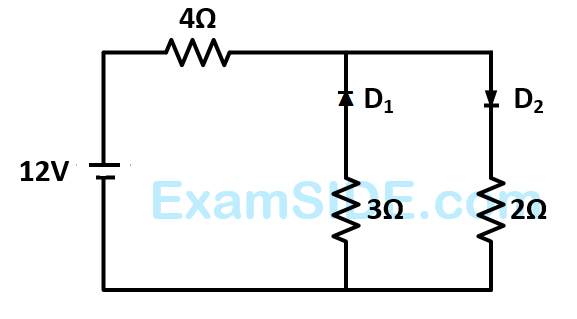

3
In the following, which one of the diodes is reverse biased?
4
The anode voltage of a photocell is kept fixed. The wavelength $$\lambda $$ of the light falling on the cathode is gradually changed. The plate current $${\rm I}$$ of the photocell varies as follows
5
If the lattice constant of this semiconductor is decreased, then which of the following is correct?
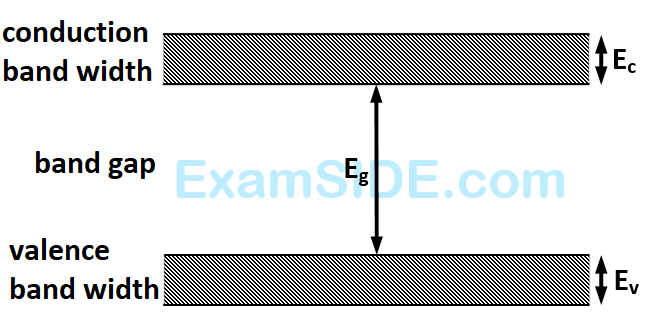

6
An inductor $$(L=100$$ $$mH)$$, a resistor $$\left( {R = 100\,\Omega } \right)$$ and a battery $$\left( {E = 100V} \right)$$ are initially connected in series as shown in the figure. After a long time the battery is disconnected after short circuiting the points $$A$$ and $$B$$. The current in the circuit $$1$$ $$ms$$ after the short circuit is


7
Needles $${N_1}$$, $${N_2}$$ and $${N_3}$$ are made of ferromagnetic, a paramagnetic and a diamagnetic substance respectively. A magnet when brought close to them will
8
In a region, steady and uniform electric and magnetic fields are present. These two fields are parallel to each other. A charged particle is released from rest in this region. The path of the particle will be a
9
A long solenoid has $$200$$ turns per $$cm$$ and carries a current $$i.$$ The magnetic field at its center is $$6.28 \times {10^{ - 2}}\,\,\,Weber/{m^2}.$$ Another long solenoid has $$100$$ turns per $$cm$$ and it carries a current $${i \over 3}$$. The value of the magnetic field at its center is
10
Which of the following units denotes the dimension $${{M{L^2}} \over {{Q^2}}}$$, where $$Q$$ denotes the electric charge?
11
In a series resonant $$LCR$$ circuit, the voltage across $$R$$ is $$100$$ volts and $$R = 1\,k\Omega $$ with $$C = 2\mu F.$$ The resonant frequency $$\omega $$ is $$200$$ $$rad/s$$. At resonance the voltage across $$L$$ is
12
In an $$AC$$ generator, a coil with $$N$$ turns, all of the same area $$A$$ and total resistance $$R,$$ rotates with frequency $$\omega $$ in a magnetic field $$B.$$ The maximum value of $$emf$$ generated in the coil is
13
The flux linked with a coil at any instant $$'t'$$ is given by
$$\phi = 10{t^2} - 50t + 250$$
The induced $$emf$$ at $$t=3s$$ is
$$\phi = 10{t^2} - 50t + 250$$
The induced $$emf$$ at $$t=3s$$ is
14
The refractive index of a glass is $$1.520$$ for red light and $$1.525$$ for blue light. Let $${D_1}$$ and $${D_2}$$ be angles of minimum deviation for red and blue light respectively in a prism of this glass. Then,
15
The threshold frequency for a metallic surface corresponds to an energy of $$6.2$$ $$eV$$ and the stopping potential for a radiation incident on this surface is $$5V.$$ The incident radiation lies in
16
The time taken by a photoelectron to come out after the photon strikes is approximately
17
An alpha nucleus of energy $${1 \over 2}m{v^2}$$ bombards a heavy nuclear target of charge $$Ze$$. Then the distance of closest approach for the alpha nucleus will be proportional to
18
If the ratio of the concentration of electrons to that of holes in a semiconductor is $${7 \over 5}$$ and the ratio of currents is $${7 \over 4},$$ then what is the ratio of their drift velocities?
19
A solid which is not transparent to visible light and whose conductivity increases with temperature is formed by
20
When $${}_3L{i^7}$$ nuclei are bombarded by protons, and the resultant nuclei are $${}_4B{e^8}$$, the emitted particles will be
21
If the binding energy per nucleon in $${}_3^7Li$$ and $${}_2^4He$$ nuclei are $$5.60$$ $$MeV$$ and $$7.06$$ $$MeV$$ respectively, then in the reaction
$$$p + {}_3^7Li \to 2\,{}_2^4He$$$
energy of proton must be
energy of proton must be
22
The resistance of bulb filmanet is $$100\Omega $$ at a temperature of $${100^ \circ }C.$$ If its temperature coefficient of resistance be $$0.005$$ per $$^ \circ C$$, its resistance will become $$200\,\Omega $$ at a temperature of
23
If the terminal speed of a sphere of gold (density $$ = 19.5\,\,kg/{m^3}$$) is $$0.2$$ $$m/s$$ in a viscous liquid (density $$ = 1.5\,\,kg/{m^3}$$, find the terminal speed of a sphere of silver (density $$ = 10.5\,\,kg/{m^3}$$) of the same size in the same liquid
24
A wire elongates by $$l$$ $$mm$$ when a LOAD $$W$$ is hanged from it. If the wire goes over a pulley and two weights $$W$$ each are hung at the two ends, the elongation of the wire will be (in $$mm$$)
25
The work of $$146$$ $$kJ$$ is performed in order to compress one kilo mole of gas adiabatically and in this process the temperature of the gas increases by $${7^ \circ }C.$$ The gas is $$\left( {R = 8.3J\,\,mo{l^{ - 1}}\,{K^{ - 1}}} \right)$$
26
Assuming the Sun to be a spherical body of radius $$R$$ at a temperature of $$TK$$, evaluate the total radiant powered incident of Earth at a distance $$r$$ from the Sun
Where r0 is the radius of the Earth and $$\sigma $$ is Stefan's constant.
27
Two rigid boxes containing different ideal gases are placed on a table. Box A contains one mole of nitrogen at temperature $${T_0},$$ while Box contains one mole of helium at temperature $$\left( {{7 \over 3}} \right){T_0}.$$ The boxes are then put into thermal contact with each other, and heat flows between them until the gases reach a common final temperature (ignore the heat capacity of boxes). Then, the final temperature of the gases, $${T_f}$$ in terms of $${T_0}$$ is
28
A force of $$ - F\widehat k$$ acts on $$O,$$ the origin of the coordinate system. The torque about the point $$(1, -1)$$ is

29
Starting from the origin a body oscillates simple harmonically with a period of $$2$$ $$s.$$ After what time will its kinetic energy be $$75\% $$ of the total energy?
30
The maximum velocity of a particle, executing simple harmonic motion with an amplitude $$7$$ $$mm,$$ is $$4.4$$ $$m/s.$$ The period of oscillation is
31
A string is stretched between fixed points separated by $$75.0$$ $$cm.$$ It is observed to have resonant frequencies of $$420$$ $$Hz$$ and $$315$$ $$Hz$$. There are no other resonant frequencies between these two. Then, the lowest resonant frequency for this string is
32
An electric dipole is placed at an angle of $${30^ \circ }$$ to a non-uniform electric field. The dipole will experience
33
Two spherical conductors $$A$$ and $$B$$ of radii $$1$$ $$mm$$ and $$2$$ $$mm$$ are separated by a distance of $$5$$ $$cm$$ and are uniformly charged. If the spheres are connected by a conducting wire then in equilibrium condition, the ratio of the magnitude of the electric fields at the surfaces of spheres $$A$$ and $$B$$ is
34
Two insulating plates are both uniformly charged in such a way that the potential difference between them is $${V_2} - {V_1} = 20\,V.$$ (i.e., plate $$2$$ is at a higher potential). The plates are separated by $$d=0.1$$ $$m$$ and can be treated as infinitely large. An electron is released from rest on the inner surface of plate $$1.$$ What is its speed when it hits plate $$2$$?
$$\left( {e = 1.6 \times {{10}^{ - 19}}\,C,\,\,{m_e} = 9.11 \times {{10}^{ - 31}}\,kg} \right)$$
$$\left( {e = 1.6 \times {{10}^{ - 19}}\,C,\,\,{m_e} = 9.11 \times {{10}^{ - 31}}\,kg} \right)$$

35
A thermocouple is made from two metals, Antimony and Bismuth. If one junction of the couple is kept hot and the other is kept cold, then, an electric current will
36
An electric bulb is rated $$220$$ volt - $$100$$ watt. The power consumed by it when operated on $$110$$ volt will be
37
In a Wheatstone's bridge, three resistance $$P, Q$$ and $$R$$ connected in the three arms and the fourth arm is formed by two resistances $${S_1}$$ and $${S_2}$$ connected in parallel. The condition for the bridge to be balanced will be
38
The current $${\rm I}$$ drawn from the $$5$$ volt source will be
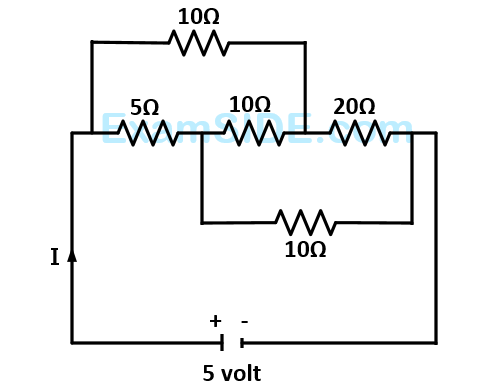

39
Four point masses, each of value $$m,$$ are placed at the corners of a square $$ABCD$$ of side $$l$$. The moment of inertia of this system about an axis passing through $$A$$ and parallel to $$BD$$ is
40
A particle located at x = 0 at time t = 0, starts moving along the positive x-direction with a velocity
'v' that varies as $$v = \alpha \sqrt x $$. The displacement of the particle varies with time as
41
A mass of $$M$$ $$kg$$ is suspended by a weightless string. The horizontal force that is required to displace it until the string makes an angle of $${45^ \circ }$$ with the initial vertical direction is
42
A ball of mass $$0.2$$ $$kg$$ is thrown vertically upwards by applying a force by hand. If the hand moves $$0.2$$ $$m$$ while applying the force and the ball goes upto $$2$$ $$m$$ height further, find the magnitude of the force. (consider $$g = 10\,m/{s^2}$$).
43
A player caught a cricket ball of mass $$150$$ $$g$$ moving at a rate of $$20$$ $$m/s.$$ If the catching process is completed in $$0.1s,$$ the force of the blow exerted by the ball on the hand of the player is equal to
44
A coin is placed on a horizontal platform which undergoes vertical simple harmonic motoin of angular frequency $$\omega .$$ The amplitude of oscillation is gradually increased. The coin will leave contact with the platform for the first time
45
A particle of mass $$100g$$ is thrown vertically upwards with a speed of $$5$$ $$m/s$$. The work done by the force of gravity during the time the particle goes up is
46
The potential energy of a $$1$$ $$kg$$ particle free to move along the $$x$$-axis is given by $$V\left( x \right) = \left( {{{{x^4}} \over 4} - {{{x^2}} \over 2}} \right)J$$.
The total mechanical energy of the particle is $$2J.$$ Then, the maximum speed (in $$m/s$$) is
47
A bomb of mass $$16kg$$ at rest explodes into two pieces of masses $$4$$ $$kg$$ and $$12$$ $$kg.$$ The velocity of the $$12$$ $$kg$$ mass is $$4\,\,m{s^{ - 1}}.$$ The kinetic energy of the other mass is
48
Consider a two particle system with particles having masses $${m_1}$$ and $${m_2}$$. If the first particle is pushed towards the center of mass through a distance $$d,$$ by what distance should the second particle is moved, so as to keep the center of mass at the same position?
49
A thin circular ring of mass $$m$$ and radius $$R$$ is rotating about its axis with a constant angular velocity $$\omega $$. Two objects each of mass $$M$$ are attached gently to the opposite ends of a diameter of the ring. The ring now rotates with an angular velocity $$\omega ' = $$