The linear mass density of a thin rod AB of length L varies from A to B as
$$\lambda \left( x \right) = {\lambda _0}\left( {1 + {x \over L}} \right)$$, where x is the distance from A. If M is the mass of the rod then its moment of inertia about an axis passing through A and perpendicular to the rod is :

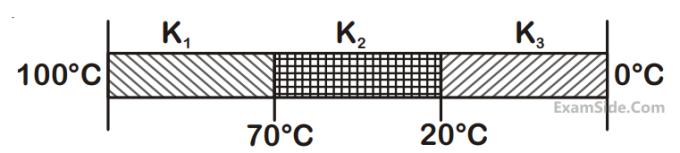
Three rods of identical cross-section and lengths are made of three different materials of thermal conductivity K₁ , K₂ and K₃ , respecrtively. They are joined together at their ends to make a long rod (see figure). One end of the long rod is maintained at 100^oC and the other at 0^oC (see figure). If the joints of the rod are at 70^oC and 20^oC in steady state and there is no loss of energy from the surface of the rod, the correct relationship between K₁ , K₂ and K₃ is :

A fluid is flowing through a horizontal pipe of varying cross-section, with
speed v ms^–1 at a point where the pressure is P pascal. At another point where pressure is $${P \over 2}$$ Pascal its speed is V ms^–1. If the density of the fluid is $$\rho $$ kg m^–3 and the flow is streamline, then V is equal to :

When a particle of mass m is attached to a vertical spring of spring constant k and released, its motion is described by
y(t) = y₀ sin² $$\omega $$t, where 'y' is measured from the lower end of unstretched spring. Then $$\omega $$ is: