A wheel is rotating freely with an angular speed $$\omega $$ on a shaft. The moment of inertia of the wheel is I and the moment of inertia of the shaft is negligible. Another wheel of moment of inertia 3I initially at rest is suddenly coupled to the same shaft. The resultant fractional loss in the kinetic energy of the system is :

Consider two uniform discs of the same thickness and different radii R₁ = R and
R₂ = $$\alpha $$R made of the same material. If the ratio of their moments of inertia I₁ and I₂ , respectively, about their axes is I₁ : I₂ = 1 : 16 then the value of $$\alpha $$ is :

For a uniform rectangular sheet shown in the figure, the ratio of moments of inertia about the axes perpendicular to the sheet and passing through O (the centre of mass) and O' (corner point) is :

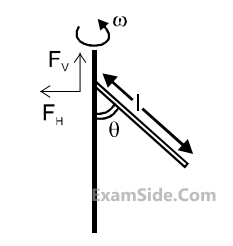
A uniform rod of length ‘$$l$$’ is pivoted at one of its ends on a vertical shaft of negligible radius. When the shaft rotates at angular speed $$\omega $$ the rod makes an angle $$\theta $$ with it (see figure). To find $$\theta $$ equate the rate of change of angular momentum (direction going into the paper) $${{m{l^2}} \over {12}}{\omega ^2}\sin \theta \cos \theta $$ about the centre of mass (CM) to the torque provided by the horizontal and vertical forces F_H and F_V about the CM. The value of $$\theta $$ is then such that :