Permutations and Combinations · Mathematics · JEE Main

A person has three different bags and four different books. The number of ways, in which he can put these books in the bags so that no bag is empty, is :

A building has ground floor and 10 more floors. Nine persons enter in a lift at the ground floor. The lift goes up to the $10^{\text {th }}$ floor. The number of ways, in which any 4 persons exit at a floor and the remaining 5 persons exit at a different floor, if the lift does not stop at the first and the second floors, is equal to :

The number of 4-letter words, with or without meaning, each consisting of two vowels and two consonants that can be formed from the letters of the word INCONSEQUENTIAL, without repeating any letter, is:

A box contains 5 blue, 6 yellow and 4 red balls. The number of ways, of drawing 8 balls containing at least two balls of each colour, is :

Let

$\mathrm{A}=\{(a, b, c): a, b, c$ are non-negative integers and $a+b+2 c=22\}$.

Then $n(\mathrm{~A})$ is equal to :

The number of ways, of forming a queue of 4 boys and 3 girls such that all the girls are not together, is:

Let $p_n$ denote the total number of triangles formed by joining the vertices of an $n$-side regular polygon.
If $p_{n+1} - p_n = 66$, then the sum of all distinct prime divisors of $n$ is :

The number of seven-digit numbers, that can be formed by using the digits 1, 2, 3, 5 and 7 such that each digit is used at least once, is:

The number of elements in the set $S = \left\{ (r, k) : k \in \mathbb{Z} \text{ and } ^{36}C_{r+1} = \frac{6\left(^{35}C_{r}\right)}{(k^2-3)} \right\}$ is :

Let $\mathrm{S}=\{1,2,3,4,5,6,7,8,9\}$. Let $x$ be the number of 9-digit numbers formed using the digits of the set S such that only one digit is repeated and it is repeated exactly twice. Let $y$ be the number of 9 -digit numbers formed using the digits of the set S such that only two digits are repeated and each of these is repeated exactly twice. Then,

The letters of the word "UDAYPUR" are written in all possible ways with or without meaning and these words are arranged as in a dictionary. The rank of the word "UDAYPUR" is

The largest value of $n$, for which $40^n$ divides $60!$, is

The number of ways, in which 16 oranges can be distributed to four children such that each child gets at least one orange, is

The largest $n \in \mathbb{N}$, for which $7^n$ divides $101!$, is :

The number of strictly increasing functions $f$ from the set $\{1,2,3,4,5,6\}$ to the set $\{1,2,3, \ldots ., 9\}$ such that $f(i) \neq i$ for $1 \leq i \leq 6$, is equal to :

There are 12 points in a plane, no three of which are in the same straight line, except 5 points which are collinear. Then the total number of triangles that can be formed with the vertices at any three of these 12 points is

From a group of 7 batsmen and 6 bowlers, 10 players are to be chosen for a team, which should include atleast 4 batsmen and atleast 4 bowlers. One batsmen and one bowler who are captain and vice-captain respectively of the team should be included. Then the total number of ways such a selection can be made, is

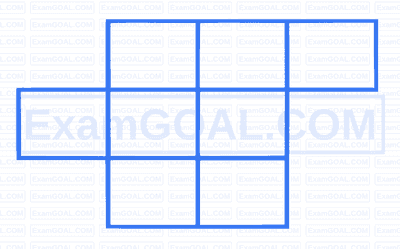
The number of sequences of ten terms, whose terms are either 0 or 1 or 2 , that contain exactly five 1 s and exactly three 2 s , is equal to :

If all the words with or without meaning made using all the letters of the word "KANPUR" are arranged as in a dictionary, then the word at 440^th position in this arrangement is :

Let $ P $ be the set of seven digit numbers with sum of their digits equal to 11. If the numbers in $ P $ are formed by using the digits 1, 2 and 3 only, then the number of elements in the set $ P $ is :

Let ${ }^n C_{r-1}=28,{ }^n C_r=56$ and ${ }^n C_{r+1}=70$. Let $A(4 \operatorname{cost}, 4 \sin t), B(2 \sin t,-2 \cos t)$ and $C\left(3 r-n, r^2-n-1\right)$ be the vertices of a triangle $A B C$, where $t$ is a parameter. If $(3 x-1)^2+(3 y)^2$ $=\alpha$, is the locus of the centroid of triangle ABC , then $\alpha$ equals

The number of different 5 digit numbers greater than 50000 that can be formed using the digits 0 , $1,2,3,4,5,6,7$, such that the sum of their first and last digits should not be more than 8 , is

Group A consists of 7 boys and 3 girls, while group B consists of 6 boys and 5 girls. The number of ways, 4 boys and 4 girls can be invited for a picnic if 5 of them must be from group $A$ and the remaining 3 from group $B$, is equal to :

The number of words, which can be formed using all the letters of the word "DAUGHTER", so that all the vowels never come together, is :

In a group of 3 girls and 4 boys, there are two boys $B_1$ and $B_2$. The number of ways, in which these girls and boys can stand in a queue such that all the girls stand together, all the boys stand together, but $B_1$ and $B_2$ are not adjacent to each other, is :

From all the English alphabets, five letters are chosen and are arranged in alphabetical order. The total number of ways, in which the middle letter is ' M ', is :

The number of ways five alphabets can be chosen from the alphabets of the word MATHEMATICS, where the chosen alphabets are not necessarily distinct, is equal to:

Let $$[t]$$ be the greatest integer less than or equal to $$t$$. Let $$A$$ be the set of all prime factors of 2310 and $$f: A \rightarrow \mathbb{Z}$$ be the function $$f(x)=\left[\log _2\left(x^2+\left[\frac{x^3}{5}\right]\right)\right]$$. The number of one-to-one functions from $$A$$ to the range of $$f$$ is

If all the words with or without meaning made using all the letters of the word "NAGPUR" are arranged as in a dictionary, then the word at $$315^{\text {th }}$$ position in this arrangement is :

Let $$0 \leq r \leq n$$. If $${ }^{n+1} C_{r+1}:{ }^n C_r:{ }^{n-1} C_{r-1}=55: 35: 21$$, then $$2 n+5 r$$ is equal to :

The number of triangles whose vertices are at the vertices of a regular octagon but none of whose sides is a side of the octagon is

Let the set $$S=\{2,4,8,16, \ldots, 512\}$$ be partitioned into 3 sets $$A, B, C$$ with equal number of elements such that $$\mathrm{A} \cup \mathrm{B} \cup \mathrm{C}=\mathrm{S}$$ and $$\mathrm{A} \cap \mathrm{B}=\mathrm{B} \cap \mathrm{C}=\mathrm{A} \cap \mathrm{C}=\phi$$. The maximum number of such possible partitions of $$S$$ is equal to:

60 words can be made using all the letters of the word $$\mathrm{BHBJO}$$, with or without meaning. If these words are written as in a dictionary, then the $$50^{\text {th }}$$ word is:

There are 5 points $$P_1, P_2, P_3, P_4, P_5$$ on the side $$A B$$, excluding $$A$$ and $$B$$, of a triangle $$A B C$$. Similarly there are 6 points $$\mathrm{P}_6, \mathrm{P}_7, \ldots, \mathrm{P}_{11}$$ on the side $$\mathrm{BC}$$ and 7 points $$\mathrm{P}_{12}, \mathrm{P}_{13}, \ldots, \mathrm{P}_{18}$$ on the side $$\mathrm{CA}$$ of the triangle. The number of triangles, that can be formed using the points $$\mathrm{P}_1, \mathrm{P}_2, \ldots, \mathrm{P}_{18}$$ as vertices, is:

The number of ways in which 21 identical apples can be distributed among three children such that each child gets at least 2 apples, is

If for some $$m, n ;{ }^6 C_m+2\left({ }^6 C_{m+1}\right)+{ }^6 C_{m+2}>{ }^8 C_3$$ and $${ }^{n-1} P_3:{ }^n P_4=1: 8$$, then $${ }^n P_{m+1}+{ }^{\mathrm{n}+1} C_m$$ is equal to

Number of ways of arranging 8 identical books into 4 identical shelves where any number of shelves may remain empty is equal to

Let $$\alpha=\frac{(4 !) !}{(4 !)^{3 !}}$$ and $$\beta=\frac{(5 !) !}{(5 !)^{4 !}}$$. Then :

All words, with or without meaning, are made using all the letters of the word MONDAY. These words are written as in a dictionary with serial numbers. The serial number of the word MONDAY is :

The number of five digit numbers, greater than 40000 and divisible by 5 , which can be formed using the digits $$0,1,3,5,7$$ and 9 without repetition, is equal to :

If the letters of the word MATHS are permuted and all possible words so formed are arranged as in a dictionary with serial numbers, then the serial number of the word THAMS is :

The number of triplets $$(x, \mathrm{y}, \mathrm{z})$$, where $$x, \mathrm{y}, \mathrm{z}$$ are distinct non negative integers satisfying $$x+y+z=15$$, is :

Eight persons are to be transported from city A to city B in three cars of different makes. If each car can accommodate at most three persons, then the number of ways, in which they can be transported, is :

If the number of words, with or without meaning, which can be made using all the letters of the word MATHEMATICS in which $$\mathrm{C}$$ and $$\mathrm{S}$$ do not come together, is $$(6 !) \mathrm{k}$$, then $$\mathrm{k}$$ is equal to :

The number of arrangements of the letters of the word "INDEPENDENCE" in which all the vowels always occur together is :

The number of ways, in which 5 girls and 7 boys can be seated at a round table so that no two girls sit together, is :

Let the number of elements in sets $$A$$ and $$B$$ be five and two respectively. Then the number of subsets of $$A \times B$$ each having at least 3 and at most 6 elements is :

All the letters of the word PUBLIC are written in all possible orders and these words are written as in a dictionary with serial numbers. Then the serial number of the word PUBLIC is :

The value of $$\frac{1}{1 ! 50 !}+\frac{1}{3 ! 48 !}+\frac{1}{5 ! 46 !}+\ldots .+\frac{1}{49 ! 2 !}+\frac{1}{51 ! 1 !}$$ is :

The letters of the word OUGHT are written in all possible ways and these words are arranged as in a dictionary, in a series. Then the serial number of the word TOUGH is :

The number of 3 digit numbers, that are divisible by either 3 or 4 but not divisible by 48, is :

The number of numbers, strictly between 5000 and 10000 can be formed using the digits 1, 3, 5, 7, 9 without repetition, is :

$$\sum\limits_{k = 0}^6 {{}^{51 - k}{C_3}} $$ is equal to :

The number of integers, greater than 7000 that can be formed, using the digits 3, 5, 6, 7, 8 without repetition is :

The number of square matrices of order 5 with entries from the set {0, 1}, such that the sum of all the elements in each row is 1 and the sum of all the elements in each column is also 1, is :

The number of ways to distribute 30 identical candies among four children C₁, C₂, C₃ and C₄ so that C₂ receives at least 4 and at most 7 candies, C₃ receives at least 2 and at most 6 candies, is equal to :

The total number of 5-digit numbers, formed by using the digits 1, 2, 3, 5, 6, 7 without repetition, which are multiple of 6, is :

Two players A and B play a series of games of badminton. The player, who wins 5 games first, wins the series. Assuming that no game ends in a draw, the number of ways, in which player A wins the series is $\_\_\_\_$ .

Three persons enter in a lift at the ground floor. The lift will go up to 10^th floor. The number of ways, in which the three persons can exit the lift at three different floors, if the lift does not stop at first, second and third floors, is equal to ________.

Let S denote the set of 4-digit numbers $a b c d$ such that $a>b>c>d$ and P denote the set of 5 -digit numbers having product of its digits equal to 20 . Then $n(\mathrm{~S})+n(\mathrm{P})$ is equal to $\_\_\_\_$

The number of 4 -letter words, with or without meaning, which can be formed using the letters PQRPQRSTUVP, is $\_\_\_\_$ .

Let ABC be a triangle. Consider four points $\mathrm{p}_1, \mathrm{p}_2, \mathrm{p}_3, \mathrm{p}_4$ on the side AB , five points $p_5, p_6, p_7, p_8, p_9$ on the side $B C$, and four points $p_{10}, p_{11}, p_{12}, p_{13}$ on the side AC . None of these points is a vertex of the triangle ABC . Then the total number of pentagons, that can be formed by taking all the vertices from the points $p_1, p_2, \ldots, p_{13}$, is $\_\_\_\_$

Let $S=\{(m, n): m, n \in\{1,2,3, \ldots . ., 50\}\}$. If the number of elements $(m, n)$ in $S$ such that $6^m+9^n$ is a multiple of 5 is $p$ and the number of elements ( $m, n$ ) in $S$ such that $m+n$ is a square of a prime number is q , then $\mathrm{p}+\mathrm{q}$ is equal to $\_\_\_\_$ .

Let m and $\mathrm{n},(\mathrm{m}<\mathrm{n})$, be two 2-digit numbers. Then the total numbers of pairs $(\mathrm{m}, \mathrm{n})$, such that $\operatorname{gcd}(m, n)=6$, is __________ .

All five letter words are made using all the letters A, B, C, D, E and arranged as in an English dictionary with serial numbers. Let the word at serial number $n$ be denoted by $\mathrm{W}_{\mathrm{n}}$. Let the probability $\mathrm{P}\left(\mathrm{W}_{\mathrm{n}}\right)$ of choosing the word $\mathrm{W}_{\mathrm{n}}$ satisfy $\mathrm{P}\left(\mathrm{W}_{\mathrm{n}}\right)=2 \mathrm{P}\left(\mathrm{W}_{\mathrm{n}-1}\right), \mathrm{n}>1$.

If $\mathrm{P}(\mathrm{CDBEA})=\frac{2^\alpha}{2^\beta-1}, \alpha, \beta \in \mathbb{N}$, then $\alpha+\beta$ is equal to :____________

If the number of seven-digit numbers, such that the sum of their digits is even, is $m \cdot n \cdot 10^n ; m, n \in\{1,2,3, \ldots, 9\}$, then $m+n$ is equal to__________

The number of 6-letter words, with or without meaning, that can be formed using the letters of the word MATHS such that any letter that appears in the word must appear at least twice, is _________.

The number of natural numbers, between 212 and 999, such that the sum of their digits is 15, is _______.

Number of functions $f:\{1,2, \ldots, 100\} \rightarrow\{0,1\}$, that assign 1 to exactly one of the positive integers less than or equal to 98 , is equal to ________.

The number of 3 -digit numbers, that are divisible by 2 and 3 , but not divisible by 4 and 9 , is _________.

The number of ways, 5 boys and 4 girls can sit in a row so that either all the boys sit together or no two boys sit together, is ________.

The number of integers, between 100 and 1000 having the sum of their digits equals to 14 , is __________.

The number of 3-digit numbers, formed using the digits 2, 3, 4, 5 and 7, when the repetition of digits is not allowed, and which are not divisible by 3 , is equal to ________.

The number of ways of getting a sum 16 on throwing a dice four times is ________.

There are 4 men and 5 women in Group A, and 5 men and 4 women in Group B. If 4 persons are selected from each group, then the number of ways of selecting 4 men and 4 women is ________.

The total number of words (with or without meaning) that can be formed out of the letters of the word 'DISTRIBUTION' taken four at a time, is equal to __________.

In an examination of Mathematics paper, there are 20 questions of equal marks and the question paper is divided into three sections : $$A, B$$ and $$C$$. A student is required to attempt total 15 questions taking at least 4 questions from each section. If section $$A$$ has 8 questions, section $$B$$ has 6 questions and section $$C$$ has 6 questions, then the total number of ways a student can select 15 questions is __________.

All the letters of the word "GTWENTY" are written in all possible ways with or without meaning and these words are written as in a dictionary. The serial number of the word "GTWENTY" is _________.

Total numbers of 3-digit numbers that are divisible by 6 and can be formed by using the digits $$1,2,3,4,5$$ with repetition, is _________.

The number of seven digit positive integers formed using the digits $$1,2,3$$ and $$4$$ only and sum of the digits equal to $$12$$ is ___________.

Let the digits a, b, c be in A. P. Nine-digit numbers are to be formed using each of these three digits thrice such that three consecutive digits are in A.P. at least once. How many such numbers can be formed?

In an examination, 5 students have been allotted their seats as per their roll numbers. The number of ways, in which none of the students sits on the allotted seat, is _________.

The sum of all the four-digit numbers that can be formed using all the digits 2, 1, 2, 3 is equal to __________.

The number of permutations, of the digits 1, 2, 3, ..., 7 without repetition, which neither contain the string 153 nor the string 2467, is ___________.

Some couples participated in a mixed doubles badminton tournament. If the number of matches played, so that no couple played in a match, is 840, then the total number of persons, who participated in the tournament, is ___________.

The number of 4-letter words, with or without meaning, each consisting of 2 vowels and 2 consonants, which can be formed from the letters of the word UNIVERSE without repetition is __________.

The number of ways of giving 20 distinct oranges to 3 children such that each child gets at least one orange is ___________.

Number of integral solutions to the equation $$x+y+z=21$$, where $$x \ge 1,y\ge3,z\ge4$$, is equal to ____________.

The total number of six digit numbers, formed using the digits 4, 5, 9 only and divisible by 6, is ____________.

The number of 3-digit numbers, that are divisible by either 2 or 3 but not divisible by 7, is ____________.

The number of words, with or without meaning, that can be formed using all the letters of the word ASSASSINATION so that the vowels occur together, is ___________.

Let 5 digit numbers be constructed using the digits $$0,2,3,4,7,9$$ with repetition allowed, and are arranged in ascending order with serial numbers. Then the serial number of the number 42923 is __________.

Number of 4-digit numbers that are less than or equal to 2800 and either divisible by 3 or by 11 , is equal to ____________.

Number of 4-digit numbers (the repetition of digits is allowed) which are made using the digits 1, 2, 3 and 5, and are divisible by 15, is equal to ___________.

The total number of 4-digit numbers whose greatest common divisor with 54 is 2, is __________.

If all the six digit numbers $$x_1\,x_2\,x_3\,x_4\,x_5\,x_6$$ with $$0< x_1 < x_2 < x_3 < x_4 < x_5 < x_6$$ are arranged in the increasing order, then the sum of the digits in the $$\mathrm{72^{th}}$$ number is _____________.

Five digit numbers are formed using the digits 1, 2, 3, 5, 7 with repetitions and are written in descending order with serial numbers. For example, the number 77777 has serial number 1. Then the serial number of 35337 is ____________.

A triangle is formed by X-axis, Y-axis and the line $$3x+4y=60$$. Then the number of points P(a, b) which lie strictly inside the triangle, where a is an integer and b is a multiple of a, is ____________.

Suppose Anil's mother wants to give 5 whole fruits to Anil from a basket of 7 red apples, 5 white apples and 8 oranges. If in the selected 5 fruits, at least 2 oranges, at least one red apple and at least one white apple must be given, then the number of ways, Anil's mother can offer 5 fruits to Anil is ____________

Let $$x$$ and $$y$$ be distinct integers where $$1 \le x \le 25$$ and $$1 \le y \le 25$$. Then, the number of ways of choosing $$x$$ and $$y$$, such that $$x+y$$ is divisible by 5, is ____________.

A boy needs to select five courses from 12 available courses, out of which 5 courses are language courses. If he can choose at most two language courses, then the number of ways he can choose five courses is __________

The number of 9 digit numbers, that can be formed using all the digits of the number 123412341 so that the even digits occupy only even places, is ______________.

The number of natural numbers lying between 1012 and 23421 that can be formed using the digits $$2,3,4,5,6$$ (repetition of digits is not allowed) and divisible by 55 is _________.

The number of matrices of order $$3 \times 3$$, whose entries are either 0 or 1 and the sum of all the entries is a prime number, is __________.

A class contains b boys and g girls. If the number of ways of selecting 3 boys and 2 girls from the class is 168 , then $$\mathrm{b}+3 \mathrm{~g}$$ is equal to ____________.

Let $$S$$ be the set of all passwords which are six to eight characters long, where each character is either an alphabet from $$\{A, B, C, D, E\}$$ or a number from $$\{1,2,3,4,5\}$$ with the repetition of characters allowed. If the number of passwords in $$S$$ whose at least one character is a number from $$\{1,2,3,4,5\}$$ is $$\alpha \times 5^{6}$$, then $$\alpha$$ is equal to ___________.

Numbers are to be formed between 1000 and 3000 , which are divisible by 4 , using the digits $$1,2,3,4,5$$ and 6 without repetition of digits. Then the total number of such numbers is ____________.

The number of 5-digit natural numbers, such that the product of their digits is 36 , is __________.

The letters of the word 'MANKIND' are written in all possible orders and arranged in serial order as in an English dictionary. Then the serial number of the word 'MANKIND' is _____________.

The number of 6-digit numbers made by using the digits 1, 2, 3, 4, 5, 6, 7, without repetition and which are multiple of 15 is ____________.

The total number of four digit numbers such that each of first three digits is divisible by the last digit, is equal to ____________.

Let b₁b₂b₃b₄ be a 4-element permutation with b_i $$\in$$ {1, 2, 3, ........, 100} for 1 $$\le$$ i $$\le$$ 4 and b_i $$\ne$$ b_j for i $$\ne$$ j, such that either b₁, b₂, b₃ are consecutive integers or b₂, b₃, b₄ are consecutive integers. Then the number of such permutations b₁b₂b₃b₄ is equal to ____________.

Let A be a matrix of order 2 $$\times$$ 2, whose entries are from the set {0, 1, 2, 3, 4, 5}. If the sum of all the entries of A is a prime number p, 2 < p < 8, then the number of such matrices A is ___________.

The number of ways, 16 identical cubes, of which 11 are blue and rest are red, can be placed in a row so that between any two red cubes there should be at least 2 blue cubes, is _____________.

The total number of 3-digit numbers, whose greatest common divisor with 36 is 2, is ___________.

There are ten boys B₁, B₂, ......., B₁₀ and five girls G₁, G₂, ........, G₅ in a class. Then the number of ways of forming a group consisting of three boys and three girls, if both B₁ and B₂ together should not be the members of a group, is ___________.

The total number of three-digit numbers, with one digit repeated exactly two times, is ______________.

The number of 3-digit odd numbers, whose sum of digits is a multiple of 7, is _____________.

Let A be a 3 $$\times$$ 3 matrix having entries from the set {$$-$$1, 0, 1}. The number of all such matrices A having sum of all the entries equal to 5, is ___________.

The number of 7-digit numbers which are multiples of 11 and are formed using all the digits 1, 2, 3, 4, 5, 7 and 9 is _____________.

In an examination, there are 5 multiple choice questions with 3 choices, out of which exactly one is correct. There are 3 marks for each correct answer, $$-$$2 marks for each wrong answer and 0 mark if the question is not attempted. Then, the number of ways a student appearing in the examination gets 5 marks is ____________.