Gravitation · Physics · JEE Main
MCQ (Single Correct Answer)
Given below are two statements: one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A): The radius vector from the Sun to a planet sweeps out equal areas in equal intervals of time and thus areal velocity of planet is constant.
Reason (R): For a central force field the angular momentum is a constant.
In the light of the above statements, choose the most appropriate answer from the options given below:
An object is kept at rest at a distance of $3 R$ above the earth's surface where $R$ is earth's radius. The minimum speed with which it must be projected so that it does not return to earth is : (Assume $\mathrm{M}=$ mass of earth, $\mathrm{G}=$ Universal gravitational constant)
Given below are two statements : one is labelled as Assertion A and the other is labelled as Reason $\mathbf{R}$
Assertion A : The kinetic energy needed to project a body of mass $m$ from earth surface to infinity is $\frac{1}{2} \mathrm{mgR}$, where R is the radius of earth.
Reason R : The maximum potential energy of a body is zero when it is projected to infinity from earth surface.
In the light of the above statements, choose the correct answer from the options given below
$$ \text { Match the LIST-I with LIST-II } $$
| List - I |
List - II |
||
|---|---|---|---|
| A. | $$ \text { Gravitational constant } $$ |
I. | $$ \left[\mathrm{LT}^{-2}\right] $$ |
| B. | $$ \text { Gravitational potential energy } $$ |
II. | $$ \left[\mathrm{L}^2 \mathrm{~T}^{-2}\right] $$ |
| C. | $$ \text { Gravitational potential } $$ |
III. | $$ \left[\mathrm{ML}^2 \mathrm{~T}^{-2}\right] $$ |
| D. | $$ \text { Acceleration due to gravity } $$ |
IV. | $$ \left[\mathrm{M}^{-1} \mathrm{~L}^3 \mathrm{~T}^{-2}\right] $$ |
Earth has mass 8 times and radius 2 times that of a planet. If the escape velocity from the earth is 11.2 km/s, the escape velocity in km/s from the planet will be:
If a satellite orbiting the Earth is 9 times closer to the Earth than the Moon, what is the time period of rotation of the satellite? Given rotational time period of Moon $=27$ days and gravitational attraction between the satellite and the moon is neglected.
A small point of mass $m$ is placed at a distance $2 R$ from the centre ' $O$ ' of a big uniform solid sphere of mass M and radius R . The gravitational force on ' m ' due to M is $\mathrm{F}_1$. A spherical part of radius $\mathrm{R} / 3$ is removed from the big sphere as shown in the figure and the gravitational force on m due to remaining part of $M$ is found to be $F_2$. The value of ratio $F_1: F_2$ is

A satellite of $$10^3 \mathrm{~kg}$$ mass is revolving in circular orbit of radius $$2 R$$. If $$\frac{10^4 R}{6} \mathrm{~J}$$ energy is supplied to the satellite, it would revolve in a new circular orbit of radius
(use $$g=10 \mathrm{~m} / \mathrm{s}^2, R=$$ radius of earth)
An astronaut takes a ball of mass $$m$$ from earth to space. He throws the ball into a circular orbit about earth at an altitude of $$318.5 \mathrm{~km}$$. From earth's surface to the orbit, the change in total mechanical energy of the ball is $$x \frac{\mathrm{GM}_{\mathrm{e}} \mathrm{m}}{21 \mathrm{R}_{\mathrm{e}}}$$. The value of $$x$$ is (take $$\mathrm{R}_{\mathrm{e}}=6370 \mathrm{~km})$$ :
Two satellite A and B go round a planet in circular orbits having radii 4R and R respectively. If the speed of $$\mathrm{A}$$ is $$3 v$$, the speed of $$\mathrm{B}$$ will be :
Two planets $$A$$ and $$B$$ having masses $$m_1$$ and $$m_2$$ move around the sun in circular orbits of $$r_1$$ and $$r_2$$ radii respectively. If angular momentum of $$A$$ is $$L$$ and that of $$B$$ is $$3 \mathrm{~L}$$, the ratio of time period $$\left(\frac{T_A}{T_B}\right)$$ is:
Assuming the earth to be a sphere of uniform mass density, a body weighed $$300 \mathrm{~N}$$ on the surface of earth. How much it would weigh at R/4 depth under surface of earth ?
To project a body of mass $$m$$ from earth's surface to infinity, the required kinetic energy is (assume, the radius of earth is $$R_E, g=$$ acceleration due to gravity on the surface of earth):
A satellite revolving around a planet in stationary orbit has time period 6 hours. The mass of planet is one-fourth the mass of earth. The radius orbit of planet is :
(Given $$=$$ Radius of geo-stationary orbit for earth is $$4.2 \times 10^4 \mathrm{~km}$$)
If $$\mathrm{G}$$ be the gravitational constant and $$\mathrm{u}$$ be the energy density then which of the following quantity have the dimensions as that of the $$\sqrt{\mathrm{uG}}$$ :
Match List I with List II :
| LIST I | LIST II | ||
|---|---|---|---|
| A. | Kinetic energy of planet | I. | $$ -\mathrm{GMm} / \mathrm{a} $$ |
| B. | Gravitation Potential energy of sun-planet system | II. | $$ \mathrm{GMm} / 2 \mathrm{a} $$ |
| C. | Total mechanical energy of planet | III. | $$ \frac{\mathrm{Gm}}{\mathrm{r}} $$ |
| D. | Escape energy at the surface of planet for unit mass object | IV. | $$ -\mathrm{GMm} / 2 \mathrm{a} $$ |
(Where $$\mathrm{a}=$$ radius of planet orbit, $$\mathrm{r}=$$ radius of planet, $$\mathrm{M}=$$ mass of Sun, $$\mathrm{m}=$$ mass of planet)
Choose the correct answer from the options given below :
A $$90 \mathrm{~kg}$$ body placed at $$2 \mathrm{R}$$ distance from surface of earth experiences gravitational pull of :
($$\mathrm{R}=$$ Radius of earth, $$\mathrm{g}=10 \mathrm{~m} \mathrm{~s}^{-2}$$)
Correct formula for height of a satellite from earths surface is :
A metal wire of uniform mass density having length $$L$$ and mass $$M$$ is bent to form a semicircular arc and a particle of mass $$\mathrm{m}$$ is placed at the centre of the arc. The gravitational force on the particle by the wire is :
The mass of the moon is $$\frac{1}{144}$$ times the mass of a planet and its diameter is $$\frac{1}{16}$$ times the diameter of a planet. If the escape velocity on the planet is $$v$$, the escape velocity on the moon will be :
Four identical particles of mass $$m$$ are kept at the four corners of a square. If the gravitational force exerted on one of the masses by the other masses is $$\left(\frac{2 \sqrt{2}+1}{32}\right) \frac{\mathrm{Gm}^2}{L^2}$$, the length of the sides of the square is
Escape velocity of a body from earth is $$11.2 \mathrm{~km} / \mathrm{s}$$. If the radius of a planet be onethird the radius of earth and mass be one-sixth that of earth, the escape velocity from the planet is :
The gravitational potential at a point above the surface of earth is $$-5.12 \times 10^7 \mathrm{~J} / \mathrm{kg}$$ and the acceleration due to gravity at that point is $$6.4 \mathrm{~m} / \mathrm{s}^2$$. Assume that the mean radius of earth to be $$6400 \mathrm{~km}$$. The height of this point above the earth's surface is :
A planet takes 200 days to complete one revolution around the Sun. If the distance of the planet from Sun is reduced to one fourth of the original distance, how many days will it take to complete one revolution :
At what distance above and below the surface of the earth a body will have same weight. (take radius of earth as $$R$$.)
Given below are two statements : one is labelled as Assertion (A) and the other is labelled as Reason (R).
Assertion (A) : The angular speed of the moon in its orbit about the earth is more than the angular speed of the earth in its orbit about the sun.
Reason (R) : The moon takes less time to move around the earth than the time taken by the earth to move around the sun.
In the light of the above statements, choose the most appropriate answer from the options given below :
The acceleration due to gravity on the surface of earth is $$\mathrm{g}$$. If the diameter of earth reduces to half of its original value and mass remains constant, then acceleration due to gravity on the surface of earth would be :
(Given $g=$ acceleration due to gravity on the earth.)
Given below are two statements:
Statement I : For a planet, if the ratio of mass of the planet to its radius increases, the escape velocity from the planet also increases.
Statement II : Escape velocity is independent of the radius of the planet.
In the light of above statements, choose the most appropriate answer form the options given below
Two planets A and B of radii $$\mathrm{R}$$ and 1.5 R have densities $$\rho$$ and $$\rho / 2$$ respectively. The ratio of acceleration due to gravity at the surface of $$\mathrm{B}$$ to $$\mathrm{A}$$ is:
A planet having mass $$9 \mathrm{Me}$$ and radius $$4 \mathrm{R}_{\mathrm{e}}$$, where $$\mathrm{Me}$$ and $$\mathrm{Re}$$ are mass and radius of earth respectively, has escape velocity in $$\mathrm{km} / \mathrm{s}$$ given by:
(Given escape velocity on earth $$\mathrm{V}_{\mathrm{e}}=11.2 \times 10^{3} \mathrm{~m} / \mathrm{s}$$ )
The ratio of escape velocity of a planet to the escape velocity of earth will be:-
Given: Mass of the planet is 16 times mass of earth and radius of the planet is 4 times the radius of earth.
Two satellites $$\mathrm{A}$$ and $$\mathrm{B}$$ move round the earth in the same orbit. The mass of $$\mathrm{A}$$ is twice the mass of $$\mathrm{B}$$. The quantity which is same for the two satellites will be
A space ship of mass $$2 \times 10^{4} \mathrm{~kg}$$ is launched into a circular orbit close to the earth surface. The additional velocity to be imparted to the space ship in the orbit to overcome the gravitational pull will be (if $$g=10 \mathrm{~m} / \mathrm{s}^{2}$$ and radius of earth $$=6400 \mathrm{~km}$$ ):
If $$\mathrm{V}$$ is the gravitational potential due to sphere of uniform density on it's surface, then it's value at the center of sphere will be:-
The radii of two planets 'A' and 'B' are 'R' and '4R' and their densities are $$\rho$$ and $$\rho / 3$$ respectively. The ratio of acceleration due to gravity at their surfaces $$\left(g_{A}: g_{B}\right)$$ will be:
The time period of a satellite, revolving above earth's surface at a height equal to $$\mathrm{R}$$ will be
(Given $$g=\pi^{2} \mathrm{~m} / \mathrm{s}^{2}, \mathrm{R}=$$ radius of earth)
Given below are two statements:
Statement I : Rotation of the earth shows effect on the value of acceleration due to gravity (g)
Statement II : The effect of rotation of the earth on the value of 'g' at the equator is minimum and that at the pole is maximum.
In the light of the above statements, choose the correct answer from the options given below
Two satellites of masses m and 3m revolve around the earth in circular orbits of radii r & 3r respectively. The ratio of orbital speeds of the satellites respectively is
Assuming the earth to be a sphere of uniform mass density, the weight of a body at a depth $$d=\frac{R}{2}$$ from the surface of earth, if its weight on the surface of earth is 200 N, will be:
(Given R = radius of earth)
The acceleration due to gravity at height $$h$$ above the earth if $$h << \mathrm{R}$$ (Radius of earth) is given by
The orbital angular momentum of a satellite is L, when it is revolving in a circular orbit at height h from earth surface. If the distance of satellite from the earth centre is increased by eight times to its initial value, then the new angular momentum will be -
Given below are two statements:
Statement I: If $$\mathrm{E}$$ be the total energy of a satellite moving around the earth, then its potential energy will be $$\frac{E}{2}$$.
Statement II: The kinetic energy of a satellite revolving in an orbit is equal to the half the magnitude of total energy $$\mathrm{E}$$.
In the light of the above statements, choose the most appropriate answer from the options given below
The weight of a body on the earth is $$400 \mathrm{~N}$$. Then weight of the body when taken to a depth half of the radius of the earth will be:
The weight of a body on the surface of the earth is $$100 \mathrm{~N}$$. The gravitational force on it when taken at a height, from the surface of earth, equal to one-fourth the radius of the earth is:
Choose the incorrect statement from the following:
Given below are two statements : one is labelled as Assertion $$\mathbf{A}$$ and the other is labelled as Reason $$\mathbf{R}$$.
Assertion A : Earth has atmosphere whereas moon doesn't have any atmosphere.
Reason R : The escape velocity on moon is very small as compared to that on earth.
In the light of the above statements, choose the correct answer from the options given below:
A planet has double the mass of the earth. Its average density is equal to that of the earth. An object weighing $$\mathrm{W}$$ on earth will weigh on that planet:
The escape velocities of two planets $$\mathrm{A}$$ and $$\mathrm{B}$$ are in the ratio $$1: 2$$. If the ratio of their radii respectively is $$1: 3$$, then the ratio of acceleration due to gravity of planet A to the acceleration of gravity of planet B will be :
For a body projected at an angle with the horizontal from the ground, choose the correct statement.
If earth has a mass nine times and radius twice to that of a planet P. Then $$\frac{v_{e}}{3} \sqrt{x} \mathrm{~ms}^{-1}$$ will be the minimum velocity required by a rocket to pull out of gravitational force of $$\mathrm{P}$$, where $$v_{e}$$ is escape velocity on earth. The value of $$x$$ is
Given below are two statements:
Statement I: Acceleration due to gravity is different at different places on the surface of earth.
Statement II: Acceleration due to gravity increases as we go down below the earth's surface.
In the light of the above statements, choose the correct answer from the options given below
At a certain depth "d " below surface of earth, value of acceleration due to gravity becomes four times that of its value at a height $$\mathrm{3 R}$$ above earth surface. Where $$\mathrm{R}$$ is Radius of earth (Take $$\mathrm{R}=6400 \mathrm{~km}$$ ). The depth $$\mathrm{d}$$ is equal to
If the gravitational field in the space is given as $$\left(-\frac{K}{r^{2}}\right)$$. Taking the reference point to be at $$\mathrm{r}=2 \mathrm{~cm}$$ with gravitational potential $$\mathrm{V}=10 \mathrm{~J} / \mathrm{kg}$$. Find the gravitational potential at $$\mathrm{r}=3 \mathrm{~cm}$$ in SI unit (Given, that $$\mathrm{K}=6 \mathrm{~Jcm} / \mathrm{kg}$$)
The time period of a satellite of earth is 24 hours. If the separation between the earth and the satellite is decreased to one fourth of the previous value, then its new time period will become.
Two particles of equal mass '$$m$$' move in a circle of radius '$$r$$' under the action of their mutual gravitational attraction. The speed of each particle will be :
Every planet revolves around the sun in an elliptical orbit :-
A. The force acting on a planet is inversely proportional to square of distance from sun.
B. Force acting on planet is inversely proportional to product of the masses of the planet and the sun.
C. The Centripetal force acting on the planet is directed away from the sun.
D. The square of time period of revolution of planet around sun is directly proportional to cube of semi-major axis of elliptical orbit.
Choose the correct answer from the options given below :
A body of mass is taken from earth surface to the height h equal to twice the radius of earth (R$$_e$$), the increase in potential energy will be :
(g = acceleration due to gravity on the surface of Earth)
Assume that the earth is a solid sphere of uniform density and a tunnel is dug along its diameter throughout the earth. It is found that when a particle is released in this tunnel, it executes a simple harmonic motion. The mass of the particle is 100 g. The time period of the motion of the particle will be (approximately)
(Take g = 10 m s$$^{-2}$$ , radius of earth = 6400 km)
Given below are two statements: one is labelled as Assertion A and the other is labelled as Reason R.
Assertion A : A pendulum clock when taken to Mount Everest becomes fast.
Reason R : The value of g (acceleration due to gravity) is less at Mount Everest than its value on the surface of earth.
In the light of the above statements, choose the most appropriate answer from the options given below
If the distance of the earth from Sun is 1.5 $$\times$$ 10$$^6$$ km. Then the distance of an imaginary planet from Sun, if its period of revolution is 2.83 years is :
Given below are two statements:
Statement I : Acceleration due to earth's gravity decreases as you go 'up' or 'down' from earth's surface.
Statement II : Acceleration due to earth's gravity is same at a height 'h' and depth 'd' from earth's surface, if h = d.
In the light of above statements, choose the most appropriate answer from the options given below
The weight of a body at the surface of earth is 18 N. The weight of the body at an altitude of 3200 km above the earth's surface is (given, radius of earth $$\mathrm{R_e=6400~km}$$) :
An object of mass $$1 \mathrm{~kg}$$ is taken to a height from the surface of earth which is equal to three times the radius of earth. The gain in potential energy of the object will be [If, $$\mathrm{g}=10 \mathrm{~ms}^{-2}$$ and radius of earth $$=6400 \mathrm{~km}$$ ]
If the radius of earth shrinks by $$2 \%$$ while its mass remains same. The acceleration due to gravity on the earth's surface will approximately :
A body of mass $$\mathrm{m}$$ is projected with velocity $$\lambda \,v_{\mathrm{e}}$$ in vertically upward direction from the surface of the earth into space. It is given that $$v_{\mathrm{e}}$$ is escape velocity and $$\lambda<1$$. If air resistance is considered to be negligible, then the maximum height from the centre of earth, to which the body can go, will be :
(R : radius of earth)
Two satellites $$\mathrm{A}$$ and $$\mathrm{B}$$, having masses in the ratio $$4: 3$$, are revolving in circular orbits of radii $$3 \mathrm{r}$$ and $$4 \mathrm{r}$$ respectively around the earth. The ratio of total mechanical energy of $$\mathrm{A}$$ to $$\mathrm{B}$$ is :
A body is projected vertically upwards from the surface of earth with a velocity equal to one third of escape velocity. The maximum height attained by the body will be :
(Take radius of earth $$=6400 \mathrm{~km}$$ and $$\mathrm{g}=10 \mathrm{~ms}^{-2}$$ )
The percentage decrease in the weight of a rocket, when taken to a height of $$32 \mathrm{~km}$$ above the surface of earth will, be :
$$($$ Radius of earth $$=6400 \mathrm{~km})$$
The length of a seconds pendulum at a height h = 2R from earth surface will be:
(Given R = Radius of earth and acceleration due to gravity at the surface of earth, g = $$\pi$$2 ms$$-$$2)
An object is taken to a height above the surface of earth at a distance $${5 \over 4}$$ R from the centre of the earth. Where radius of earth, R = 6400 km. The percentage decrease in the weight of the object will be :
Three identical particles $$\mathrm{A}, \mathrm{B}$$ and $$\mathrm{C}$$ of mass $$100 \mathrm{~kg}$$ each are placed in a straight line with $$\mathrm{AB}=\mathrm{BC}=13 \mathrm{~m}$$. The gravitational force on a fourth particle $$\mathrm{P}$$ of the same mass is $$\mathrm{F}$$, when placed at a distance $$13 \mathrm{~m}$$ from the particle $$\mathrm{B}$$ on the perpendicular bisector of the line $$\mathrm{AC}$$. The value of $$\mathrm{F}$$ will be approximately :
The radii of two planets A and B are in the ratio 2 : 3. Their densities are 3$$\rho$$ and 5$$\rho$$ respectively. The ratio of their acceleration due to gravity is :
The time period of a satellite revolving around earth in a given orbit is 7 hours. If the radius of orbit is increased to three times its previous value, then approximate new time period of the satellite will be
The escape velocity of a body on a planet 'A' is 12 kms$$-$$1. The escape velocity of the body on another planet 'B', whose density is four times and radius is half of the planet 'A', is :
Water falls from a 40 m high dam at the rate of 9 $$\times$$ 104 kg per hour. Fifty percentage of gravitational potential energy can be converted into electrical energy. Using this hydroelectric energy number of 100 W lamps, that can be lit, is :
(Take g = 10 ms$$-$$2)
Two objects of equal masses placed at certain distance from each other attracts each other with a force of F. If one-third mass of one object is transferred to the other object, then the new force will be :
Two planets A and B of equal mass are having their period of revolutions TA and TB such that TA = 2TB. These planets are revolving in the circular orbits of radii rA and rB respectively. Which out of the following would be the correct relationship of their orbits?
The distance of the Sun from earth is 1.5 $$\times$$ 1011 m and its angular diameter is (2000) s when observed from the earth. The diameter of the Sun will be :
Four spheres each of mass m from a square of side d (as shown in figure). A fifth sphere of mass M is situated at the centre of square. The total gravitational potential energy of the system is :

Given below are two statements :
Statement I : The law of gravitation holds good for any pair of bodies in the universe.
Statement II : The weight of any person becomes zero when the person is at the centre of the earth.
In the light of the above statements, choose the correct answer from the options given below.
Given below are two statements : One is labelled as Assertion A and the other is labelled as Reason R.
Assertion A : If we move from poles to equator, the direction of acceleration due to gravity of earth always points towards the center of earth without any variation in its magnitude.
Reason R : At equator, the direction of acceleration due to the gravity is towards the center of earth.
In the light of above statements, choose the correct answer from the options given below:
The variation of acceleration due to gravity (g) with distance (r) from the center of the earth is correctly represented by :
(Given R = radius of earth)
The height of any point P above the surface of earth is equal to diameter of earth. The value of acceleration due to gravity at point P will be : (Given g = acceleration due to gravity at the surface of earth).
The distance between Sun and Earth is R. The duration of year if the distance between Sun and Earth becomes 3R will be :
The approximate height from the surface of earth at which the weight of the body becomes $${1 \over 3}$$ of its weight on the surface of earth is :
[Radius of earth R = 6400 km and $$\sqrt 3 $$ = 1.732]

(1) the gravitational field is zero
(2) the gravitational potential is zero
(3) the gravitational field is same everywhere
(4) the gravitational potential is same everywhere
(5) all of the above
Choose the most appropriate answer from the options given below :
$$\left\{ {Given\,{{4{\pi ^2}} \over G} = 6 \times {{10}^{11}}{N^{ - 1}}{m^{ - 2}}k{g^2}} \right\}$$
Then :
A. a constant velocity of revolution.
B. has the least velocity when it is nearest to the sun.
C. its areal velocity is directly proportional to its velocity.
D. areal velocity is inversely proportional to its velocity.
E. to follow a trajectory such that the areal velocity is constant.
Choose the correct answer from the options given below :


If TA and TB are the time periods of A and B respectively then the value of TB $$-$$ TA :

[Given : radius of earth = 6400 km, mass of earth = 6 $$\times$$ 1024 kg]
Assertion A : The escape velocities of planet A and B are same. But A and B are of unequal mass.
Reason R : The product of their mass and radius must be same. M1R1 = M2R2
In the light of the above statements, choose the most appropriate answer from the options given below :
[Use $$g = {{GM} \over {{R^2}}}$$ = 9.8 ms$$-$$2 and radius of earth, R = 6400 km.]
$$\rho $$(r) = $${\rho _0}\left( {1 - {{{r^2}} \over {{R^2}}}} \right)$$.
Then the gravitational field is maximum at :

[Given ; Mass of planet = 8 × 1022 kg, Radius of planet = 2 × 106 m, Gravitational constant G = 6.67 × 10–11 Nm2 /kg2]

$$\rho $$ (r) = $${k \over r}$$ for r $$ \le $$ R and $$\rho $$ (r) = 0 for r > R,
where r is the distance from the centre.
The correct graph that describes qualitatively the acceleration, a, of a test particle as a function of r is :

($$G=gravitational $$ $$constant$$)
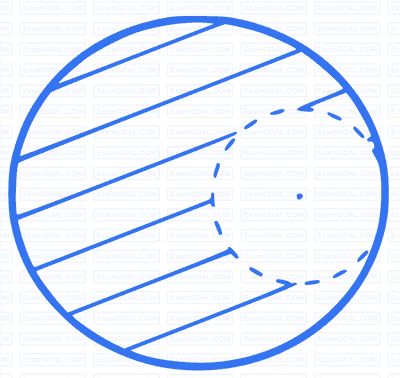
Statement - $$1$$:
For a mass $$M$$ kept at the center of a cube of side $$'a'$$, the flux of gravitational field passing through its sides $$4\,\pi \,GM.$$
Statement - 2:
If the direction of a field due to a point source is radial and its dependence on the distance $$'r'$$ from the source is given as $${1 \over {{r^2}}},$$ its flux through a closed surface depends only on the strength of the source enclosed by the surface and not on the size or shape of the surface.
$${{electro\,\,ch\arg e\,\,on\,\,the\,\,moon} \over {electronic\,\,ch\arg e\,\,on\,\,the\,\,earth}}\,\,to\,be$$
Numerical
A satellite of mass 1000 kg is launched to revolve around the earth in an orbit at a height of 270 km from the earth's surface. Kinetic energy of the satellite in this orbit is____________ $\times 10^{10} \mathrm{~J}$.
(Mass of earth $=6 \times 10^{24} \mathrm{~kg}$, Radius of earth $=6.4 \times 10^6 \mathrm{~m}$, Gravitational constant $=6.67 \times 10^{-11} \mathrm{Nm}^2 \mathrm{~kg}^{-2}$ )
Two planets, $A$ and $B$ are orbiting a common star in circular orbits of radii $R_A$ and $R_B$, respectively, with $R_B=2 R_A$. The planet $B$ is $4 \sqrt{2}$ times more massive than planet $A$. The ratio $\left(\frac{\mathrm{L}_{\mathrm{B}}}{\mathrm{L}_{\mathrm{A}}}\right)$ of angular momentum $\left(L_B\right)$ of planet $B$ to that of planet $A\left(L_A\right)$ is closest to integer ________.
Acceleration due to gravity on the surface of earth is ' $g$ '. If the diameter of earth is reduced to one third of its original value and mass remains unchanged, then the acceleration due to gravity on the surface of the earth is ________ g.
A satellite of mass $\frac{M}{2}$ is revolving around earth in a circular orbit at a height of $\frac{R}{3}$ from earth surface. The angular momentum of the satellite is $\mathrm{M} \sqrt{\frac{\mathrm{GMR}}{x}}$. The value of $x$ is _________ , where M and R are the mass and radius of earth, respectively. ( G is the gravitational constant)
If the radius of earth is reduced to three-fourth of its present value without change in its mass then value of duration of the day of earth will be ________ hours 30 minutes.
A simple pendulum is placed at a place where its distance from the earth's surface is equal to the radius of the earth. If the length of the string is $$4 m$$, then the time period of small oscillations will be __________ s. [take $$g=\pi^2 m s^{-2}$$]
If the earth suddenly shrinks to $$\frac{1}{64}$$th of its original volume with its mass remaining the same, the period of rotation of earth becomes $$\frac{24}{x}$$h. The value of x is __________.
If the acceleration due to gravity experienced by a point mass at a height h above the surface of earth is same as that of the acceleration due to gravity at a depth $$\alpha \mathrm{h}\left(\mathrm{h}<<\mathrm{R}_{\mathrm{e}}\right)$$ from the earth surface. The value of $$\alpha$$ will be _________.
(use $$\left.\mathrm{R}_{\mathrm{e}}=6400 \mathrm{~km}\right)$$
Two satellites S1 and S2 are revolving in circular orbits around a planet with radius R1 = 3200 km and R2 = 800 km respectively. The ratio of speed of satellite S1 to be speed of satellite S2 in their respective orbits would be $${1 \over x}$$ where x = ___________.

[Given : The two planets are fixed in their position]
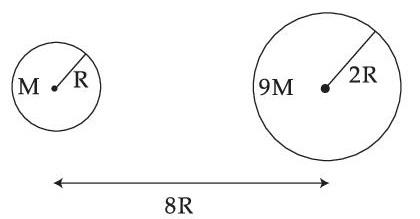
The amount of energy that needs to be supplied will be $${x \over 5}{{G{M^2}} \over R}$$ where x is __________ (Round off to the Nearest Integer) (M is the mass of earth, R is the radius of earth, G is the gravitational constant)
