$$0.3 \mathrm{~g}$$ of ethane undergoes combustion at $$27^{\circ} \mathrm{C}$$ in a bomb calorimeter. The temperature of calorimeter system (includin...

At $$25^{\circ} \mathrm{C}$$, the enthalpy of the following processes are given : .tg {border-collapse:collapse;border-spacing:0;} .tg td{border-col...

Enthalpies of formation of $\mathrm{CCl}_{4}(\mathrm{~g}), \mathrm{H}_{2} \mathrm{O}(\mathrm{g}), \mathrm{CO}_{2}(\mathrm{~g})$ and $\mathrm{HCl}(\mat...

The enthalpy change for the conversion of $$\frac{1}{2} \mathrm{Cl}_{2}(\mathrm{~g})$$ to $$\mathrm{Cl}^{-}$$(aq) is ($$-$$) ___________ $$\mathrm{kJ...

1 mole of ideal gas is allowed to expand reversibly and adiabatically from a temperature of $27^{\circ} \mathrm{C}$. The work done is $3 \mathrm{~kJ} ...

When 2 litre of ideal gas expands isothermally into vacuum to a total volume of 6 litre, the change in internal energy is ____________ J. (Nearest int...

28.0 L of CO$$_2$$ is produced on complete combustion of 16.8 L gaseous mixture of ethene and methane at 25$$^\circ$$C and 1 atm. Heat evolved during ...

An athlete is given 100 g of glucose (C$$_6$$H$$_{12}$$O$$_6$$) for energy. This is equivalent to 1800kJ of energy. The 50% of this energy gained is u...

One mole of an ideal monoatomic gas is subjected to changes as shown in the graph. The magnitude of the work done (by the system or on the system) is ...

Following figure shows spectrum of an ideal black body at four different temperatures. The number of correct statement/s from the following is _______...

For independent process at 300 K .tg {border-collapse:collapse;border-spacing:0;} .tg td{border-color:black;border-style:solid;border-width:1px;font...

When 600 mL of 0.2 M HNO3 is mixed with $$400 \mathrm{~mL}$$ of 0.1 M NaOH solution in a flask, the rise in temperature of the flask is ___________ $$...

Among the following the number of state variables is __________. Internal energy (U) Volume (V) Heat (q) Enthalpy (H)...

A gas (Molar mass = 280 $$\mathrm{~g} \mathrm{~mol}^{-1}$$) was burnt in excess $$\mathrm{O}_{2}$$ in a constant volume calorimeter and during combust...

For the reaction $$\mathrm{H}_{2} \mathrm{F}_{2}(\mathrm{~g}) \rightarrow \mathrm{H}_{2}(\mathrm{~g})+\mathrm{F}_{2}(\mathrm{~g})$$ $$\Delta U=-59.6 \...

$$2.4 \mathrm{~g}$$ coal is burnt in a bomb calorimeter in excess of oxygen at $$298 \mathrm{~K}$$ and $$1 \mathrm{~atm}$$ pressure. The temperature o...

While performing a thermodynamics experiment, a student made the following observations. HCl + NaOH $$\to$$ NaCl + H2O $$\Delta$$H = $$-$$57.3 kJ mol$...

A box contains 0.90 g of liquid water in equilibrium with water vapour at 27$$^\circ$$C. The equilibrium vapour pressure of water at 27$$^\circ$$C is ...

2.2 g of nitrous oxide (N2O) gas is cooled at a constant pressure of 1 atm from 310 K to 270 K causing the compression of the gas from 217.1 mL to 167...

17.0 g of NH3 completely vapourises at $$-$$33.42$$^\circ$$C and 1 bar pressure and the enthalpy change in the process is 23.4 kJ mol$$-$$1. The entha...

For combustion of one mole of magnesium in an open container at 300 K and 1 bar pressure, $$\Delta$$CH$$\Theta $$ = $$-$$601.70 kJ mol$$-$$1, the magn...

4.0 L of an ideal gas is allowed to expand isothermally into vacuum until the total volume is 2.0 L. The amount of heat absorbed in this expansion is ...

When 5 moles of He gas expand isothermally and reversibly at 300 K from 10 litre to 20 litre, the magnitude of the maximum work obtained is __________...

A fish swimming in water body when taken out from the water body is covered with a film of water of weight 36 g. When it is subjected to cooking at 10...

For complete combustion of methanol CH3OH(I) + $${3 \over 2}$$O2(g) $$\to$$ CO2(g) + 2H2O(I) the amount of heat produced as measured by bomb calorimet...

2.0 g of H2 gas is adsorbed on 2.5 g of platinum powder at 300 K and 1 bar pressure. The volume of the gas adsorbed per gram of the adsorbent is _____...

The standard entropy change for the reaction 4Fe(s) + 3O2(g) $$\to$$ 2Fe2O3(s) is $$-$$550 J K$$-$$1 at 298 K. [Given : The standard enthalpy change ...

The standard free energy change ($$\Delta$$G$$^\circ$$) for 50% dissociation of N2O4 into NO2 at 27$$^\circ$$C and 1 atm pressure is $$-$$ x J mol$$-$...

For the reaction, 2NO2(g) $$\rightleftharpoons$$ N2O4(g), when $$\Delta$$S = $$-$$176.0 JK$$-$$1 and $$\Delta$$H = $$-$$57.8 kJ mol$$-$$1, the magnitu...

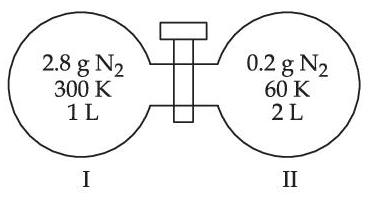
Two flasks I and II shown below are connected by a valve of negligible volume.When the valve is opened, the final pressure of the system in bar is x $...

Data given for the following reaction is as follows :FeO(s) + C(graphite) $$\to$$ Fe(s) + CO(g) .tg {border-collapse:collapse;border-spacing:0;} .tg ...

200 mL of 0.2 M HCl is mixed with 300 mL of 0.1 M NaOH. The molar heat of neutralization of this reaction is $$-$$57.1 kJ. The increase in temperature...

For water $$\Delta$$vap H = 41 kJ mol$$-$$1 at 373 K and 1 bar pressure. Assuming that water vapour is an ideal gas that occupies a much larger volume...

The Born-Haber cycle for KCl is evaluated with the following data :$${\Delta _f}{H^\Theta }$$ for KCl = $$-$$436.7 kJ mol$$-$$1 ;$${\Delta _{sub}}{H^\...

When 400 mL of 0.2 M H2SO4 solution is mixed with 600 mL of 0.1 M NaOH solution, the increase in temperature of the final solution is __________ $$\ti...

For water at 100$$^\circ$$ C and 1 bar,$$\Delta$$vap H $$-$$ $$\Delta$$vap U = _____________ $$\times$$ 102 J mol$$-$$1. (Round off to the Nearest Int...

A system does 200 J of work and at the same time absorbs 150 J of heat. The magnitude of the change in internal energy is ____________ J. (Nearest int...

At 298 K, the enthalpy of fusion of a solid (X) is 2.8 kJ mol$$-$$1 and the enthalpy of vaporisation of the liquid (X) is 98.2 kJ mol$$-$$1. The entha...

If the standard molar enthalpy change for combustion of graphite powder is $$-$$2.48 $$\times$$ 102 kJ mol$$-$$1, the amount of heat generated on comb...

For a given chemical reaction A $$\to$$ B at 300 K the free energy change is $$-$$49.4 kJ mol$$-$$1 and the enthalpy of reaction is 51.4 kJ mol$$-$$1....

For the reaction C2H6 $$ \to $$ C2H4 + H2the reaction enthalpy $$\Delta$$rH = __________ kJ mol$$-$$1. (Round off to the Nearest Integer).[ Given : Bo...

The pressure exerted by a non-reactive gaseous mixture of 6.4g of methane and 8.8 g of carbon dioxide in a 10L vessel at 27$$^\circ$$C is __________ k...

The standard enthalpies of formation of Al2O3 and CaO are $$-$$1675 kJ mol-1 and $$-$$635 kJ mol$$-$$1 respectively.For the reaction3CaO + 2Al $$ \to ...

At 25$$^\circ$$C, 50 g of iron reacts with HCl to form FeCl2. The evolved hydrogen gas expands against a constant pressure of 1 bar. The work done by ...

The average S-F bond energy in kJ mol$$-$$1 of SF6 is __________. (Rounded off to the nearest integer)[Given : The values of standard enthalpy of form...

For a chemical reaction A + B ⇌ C + D($${\Delta _r}{H^\Theta }$$ = 80 kJ mol$$-$$1) the entropy change $${\Delta _r}{S^\Theta }$$ depends on the tempe...

An exothermic reaction X $$ \to $$ Y has an activation energy 30 kJ mol$$-$$1. If energy change $$\Delta$$E during the reaction is $$-$$20 kJ, then th...

Five moles of an ideal gas at 293 K is expanded isothermally from an initial pressure of 2.1 MPa to 1.3 MPa against at constant external pressure 4.3 ...

The reaction of cyanamide, NH2CN(s) with oxygen was run in a bomb calorimeter and $$\Delta$$U was found to be $$-$$742.24 kJ mol$$-$$1. The magnitude ...

The ionization enthalpy of Na+ formation from Na(g) is 495.8 kJ mol$$-$$1, while the electron gain enthalpy of Br is $$-$$325.0 kJ mol$$-$$1. Given th...

For a dimerization reaction, 2A(g) $$ \to $$ A2(g) at 298 K, $$\Delta $$Uo = –20 kJ mol–1, $$\Delta $$So = –30 JK–1 mol–1, then the $$\Delta $$Go w...

The heat of combustion of ethanol into carbon dioxide and water is – 327 kcal at constant pressure. The heat evolved (in cal) at constant volume and 2...

The internal energy change (in J) When 90 g of water undergoes complete evaporation at 100oC is ______. (Given : $$\Delta $$Hvap for water at 373 K = ...

A cylinder containing an ideal gas (0.1 mol of 1.0 dm3) is in thermal equilibrium with a large volume of 0.5 molal aqueous solution of ethylene glycol...

A sample of milk splits after 60 min. at 300 K and after 40 min. at 400 K when the population of lactobacillus acidophilus in it doubles. The activa t...

NaClO3 is used, even in spacecrafts, to produce O2. The daily consumption of pure O2 by a person is 492L at 1 atm, 300K. How much amount of NaClO3, in...

At constant volume, 4 mol of an ideal gas when heated from 300 K to 500K changes its internal energy by 5000 J. The molar heat capacity at constant vo...

The magnitude of work done by a gas that undergoes a reversible expansion along the path ABC shown in the figure is _______. ...

The standard heat of formation $$\left( {{\Delta _f}H_{298}^0} \right)$$ of ethane (in kj/mol), if the heat of combustion of ethane, hydrogen and grap...

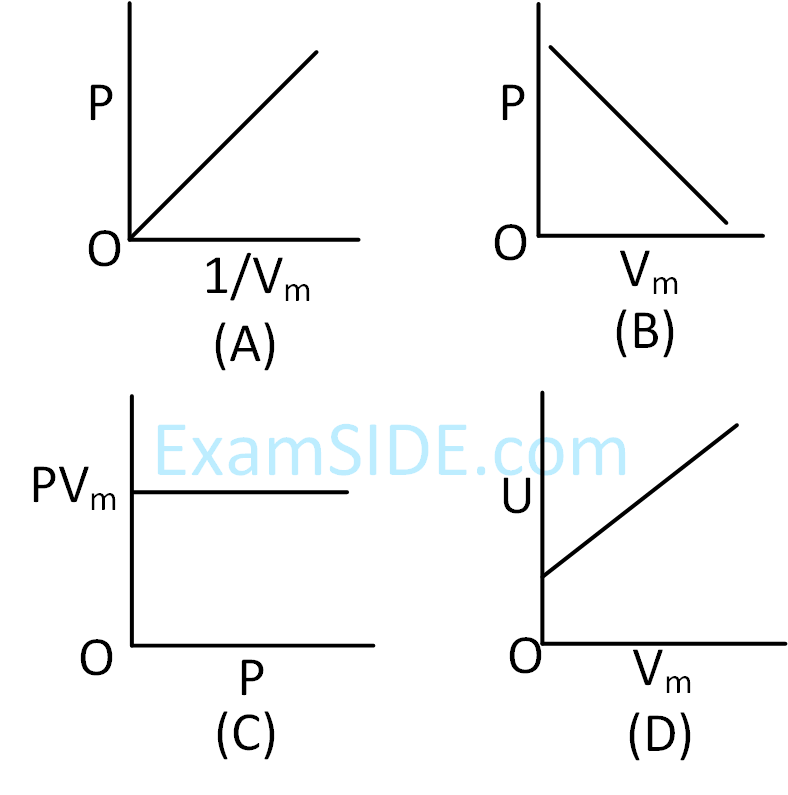
Which of the following relations are correct? (A) $$\mathrm{\Delta U=q+p\Delta V}$$ (B) $$\mathrm{\Delta G=\Delta H-T\Delta S}$$ (C) $$\Delta \mathrm{...

$$ \begin{aligned} &\mathrm{C}(\mathrm{s})+\mathrm{O}_{2}(\mathrm{~g}) \rightarrow \mathrm{CO}_{2}(\mathrm{~g})+400 \mathrm{~kJ} \\ &\mathrm{C}(\mathr...

Given below are two statements: One is labelled as Assertion $$\mathbf{A}$$ and the other is labelled as Reason $$\mathbf{R}$$ Assertion A: The reduct...

Which of the following relation is not correct?

Match List-I with List-II. .tg {border-collapse:collapse;border-spacing:0;} .tg td{border-color:black;border-style:solid;border-width:1px;font-famil...

At 25$$^\circ$$C and 1 atm pressure, the enthalpy of combustion of benzene (I) and acetylene (g) are $$-$$ 3268 kJ mol$$-$$1 and $$-$$1300 kJ mol$$-$$...

At 25$$^\circ$$C and 1 atm pressure, the enthalpies of combustion are as given below : .tg {border-collapse:collapse;border-spacing:0;} .tg td{borde...

The incorrect expression among the following is :

During which of the following processes, does entropy decrease?(A) Freezing of water to ice at 0$$^\circ$$C(B) Freezing of water to ice at $$-$$10$$^\...

For a reaction, 4M(s) + nO2(g) $$ \to $$ 2M2On(s) the free energy change is plotted as a function of temperature. The temperature below which the oxid...

Lattice enthalpy and enthalpy of solution of NaCl are 788 kJ mol–1, and 4 kJ mol–1, respectively. The hydration enthalpy of NaCl is :...

The process that is NOT endothermic in nature is :

Five moles of an ideal gas at 1 bar and 298 K is expanded into vacuum to double the volume. The work done is :

For one mole of an ideal gas, which of these statements must be true? (a) U and H each depends only on temperature (b) Compressibility factor z is not...

The true statement amongst the following is:

According to the following diagram, A reduces BO2 when the temperature is : ...

If enthalpy of atomisation for Br2(1) is x kJ/mol and bond enthalpy for Br2 is y kJ/mol, the relation between them :

A graph of vapour pressure and temperature for three different liquids X, Y, and Z is shown below : The following inferences are made : (A) X has hig...

Indentify the correct labels of A,B and C in the following graph from the options given below : Root mean square speed (Vrms); most probable speed (V...

The INCORRECT match in the following is :

Enthalpy of sublimation of iodine is 24 cal g–1 at 200 oC. If specific heat of I2(s) and l2 (vap) are 0.055 and 0.031 cal g–1K –1 respectively, then ...

An ideal gas is allowed to expand form 1 L to 10 L against a constant external pressure of I bar. The work done in kJ is:

The difference between $$\Delta $$H and $$\Delta $$U ($$\Delta $$H – $$\Delta $$U), when the combustion of one mole of heptane(l) is carried out at a ...

A process will be spontaneous at all temperatures if :

During compression of a spring the work done is 10kJ and 2kJ escaped to the surroundings as heat. The change in internal energy, $$\Delta $$U(inkJ) is...

Consider the given plot of enthalpy of the following reaction between A and B. A+ B $$ \to $$ C + D Identify the incorrect statement. ...

Among the following, the set of parameters that represents path function, is : (A) q + w (B) q (C) w (D) H–TS

5 moles of an ideal gas at 100 K are allowed to undergo reversible compression till its temperature becomes 200 K. If CV = 28 JK–1mol–1, calculate $$\...

For silver, Cp(J K–1 mol–1) = 23 +0.01 T. If the temperature (T) of 3 moles of silver is raised from 300 K to 1000 K at 1 atm pressure, the value of $...

Which one of the following equations does not correctly represent the first law of thermodynamics for the given processes involving an ideal gas ? (As...

The combination of plots which does not represent isothermal expansion of an ideal gas is – ...

For diatomic ideal gas in a closed system, which of the following plots does not correctly describe the relation between various thermodynamic quantit...

The reaction, MgO(s) + C(s) $$ \to $$ Mg(s) + CO(g), for which $$\Delta $$rHo + 491.1 kJ mol–1 and $$\Delta $$rSo = 198.0 JK–1 mol–1, is not feasible...

The standard reaction Gibbs energy for a chemical reaction at an absolute temperature T is given by $$\Delta $$rGo = A – BT Where A and B are non-zero...

For the chemical reaction X $$\rightleftharpoons$$ Y, the standard reaction Gibbs energy depends on temperature T (in K) as $$\Delta $$rGo (in kJ mo...

Two blocks of the same metal having same mass and at temperature T1 and T2, respectively, are brought in contact with each other and allowed to attain...

The process with negative entropy change is

An ideal gas undergoes isothermal compression from 5m3 to 1 m3 against a constant external pressure of 4 Nm–2. Heat released in this process is used ...

A process has $$\Delta $$H = 200 J mol–1 and $$\Delta $$S = 40 JK–1 mol–1. Out of the values given below, choose the minimum temperature above which t...

The values of Kp/Kc for the following reactions at 300 K are, respectively : (At 300 K, RT = 24.62 dm3 atm mol–1) N2(g) + O2(g)  $$\rightle...

The entropy change associated with the conversion of 1 kg of ice at 273 K to water vapours at 383 K is : (Specific heat of water liquid and water vap...

Consider the reversible isothermal expansion of an ideal gas in a closed system at two different temperatures T1 and T2 (T1 < T2). The correct grap...

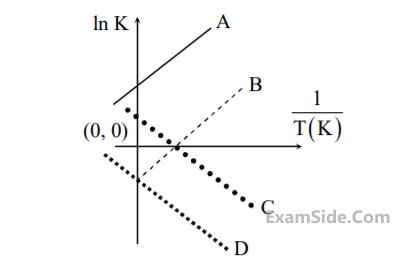
At 320 K, a gas A2 is 20% dissociated to A(g). The standard free energy change at 320 K and 1 atm in J mol-1 is approximately : (R = 8.314 JK-1 mol-1;...

For which of the following processes, $$\Delta $$S is negative ?

The combustion of benzene(l) gives CO2(g) and H2O(l). Given that heat of combustion of benzene at constant volume is –3263.9 kJ mol–1 at 25oC; heat of...

Which of the following lines correctly show the temperature dependence of equilibrium constant K, for an exothermic reaction? ...

Given (i)   2Fe2O3(s) $$ \to $$ 4Fe(s) + 3O2(g); $$\Delta $$rGo = + 1487.0 kJ mol-1 (ii)   2CO(g) + O2(g) $$ \to $$ 2CO2(g); $$\...

$$\Delta $$fGo at 500 K for substance 'S' in liquid state and gaseous state are +100.7 kcl mol-1 and +103 kcal mol-1, respectively. Vapour pressure of...

For which of the following reactions, $$\Delta $$H is equal to $$\Delta $$U?

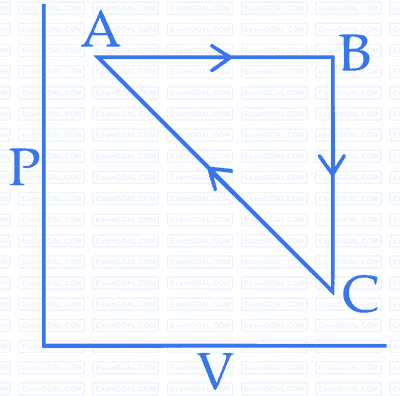
An ideal gas undergoes a cyclic process as shown in Figure. $$\Delta $$UBC = $$-$$5 kJ mol-1, qAB = $$2$$ kJ mol-1, WAB = $$-$$5 kJ mol-1, WCA = 3 kJ ...

An ideal gas undergoes isothermal expansion at constant pressure. During the process :

A gas undergoes change from state A to state B. In this process, the heat absorbed and work done by the gas is 5 J and 8 J, respectively. Now gas is b...

For a reaction, A(g) $$ \to $$ A($$\ell $$); $$\Delta $$H= $$-$$ 3RT. The correct statement for the reaction is :

The enthalpy change on freezing of 1 mol of water at 5oC to ice at −5oC is : (Given $$\Delta $$fusH = 6 kJ mol$$-$$1 at 0oC, Cp(H2O, $$\ell $$ = 75.3...

$$\Delta $$U is equal to

Given, $${C_{(graphite)}} + {O_2} \to C{O_2}(g)$$; $${\Delta _r}{H^o}$$ = - 393.5 kJ mol-1 $${{\rm H}_2}(g)$$ + $${1 \over 2}{O_2}(g)$$$$\to {{\rm H}_...

If 100 mole of H2O2 decompose at 1 bar and 300 K, the work done (kJ) by one mole of O2(g) as it expands against 1 bar pressure is : 2H2O2(l)  &nb...

A reaction at 1 bar is non-spontaneous at low temperature but becomes spontaneous at high temperature. Identify the correct statement about the reacti...

The heats of combustion of carbon and carbon monoxide are –393.5 and –283.5 kJ mol–1, respectively. The heat of formation (in kJ) of carbon monoxide p...

For complete combustion of ethanol, C2H5OH(l) + 3O2(g) $$\to$$ 2CO2(g) + 3H2O(l) the amount of heat produced as measured in bomb calorimeter, is 1364...

A piston filled with 0.04 mol of an ideal gas expands reversibly from 50.0 mL to 375 mL at a constant temperature of 37.0oC. As it does so, it absorbs...

The incorrect expression among the following is :

The entropy change involved in the isothermal reversible expansion of 2 moles of an ideal gas from a volume of 10 dm3 to a volume of 100 dm3 at 27oC i...

The standard enthalpy of formation of NH3 is –46.0 kJ mol–1. If the enthalpy of formation of H2 from its atoms is –436 kJ mol–1 and that of N2 is –712...

For a particular reversible reaction at temperature T, ∆H and ∆S were found to be both +ve. If Te is the temperature at equilibrium, the reaction woul...

On the basis of the following thermochemical data : ($$\Delta _fG^oH^+_{(aq)}$$ = 0) H2O(l) $$\to$$ H+(aq) + OH-(aq); $$\Delta H$$ = 57.32 kJ H2(g) +...

Oxidising power of chlorine in aqueous solution can be determined by the parameters indicated below: $${1 \over 2}C{l_2}(g)$$ $$\buildrel {{1 \over 2...

Standard entropy of X2, Y2 and XY3 are 60, 40 and 50 JK−1 mol−1 , respectively. For the reaction, $${1 \over 2} X_2$$ + $${3 \over 2} Y_2 \to$$ XY3,...

In conversion of lime-stone to lime, CaCO3(s) $$\to$$ CaO(s) + CO2 (g) the vales of ∆H° and ∆S° are +179.1 kJ mol−1 and 160.2 J/K respectively at 29...

Identify the correct statement regarding a spontaneous process

Assuming that water vapour is an ideal gas, the internal energy change $$\left( {\Delta U} \right)$$ when $$1$$ mol of water is vapourised at $$1$$ ba...

An ideal gas is allowed to expand both reversibly and irreversibly in an isolated system. If Ti is the initial temperature and Tf is the final tempera...

The enthalpy changes for the following processes are listed below: Cl2(g) = 2Cl(g), 242.3 kJ mol–1 I2(g) = 2I(g), 151.0 kJ mol–1 ICl(g) = I(g) + Cl...

The standard enthalpy of formation $$\Delta _fH^o$$ at 298 K for methane, CH4(g), is –74.8 kJ mol–1. The additional information required to determine ...

($$\Delta H - \Delta U$$) for the formation of carbon monoxide (CO) from its elements at 298 K is (R = 8.314 J K–1 mol–1)

Consider the reaction: N2 + 3H2 $$\to$$ 2NH3 carried out at constant temperature and pressure. If $$\Delta H$$ and $$\Delta U$$ are the enthalpy and i...

If the bond dissociation energies of XY, X2 and Y2 (all diatomic molecules) are in the ratio of 1:1:0.5 and $$\Delta H_f$$ for the formation of XY is ...

Consider an endothermic reaction, X $$\to$$ Y with the activation energies Eb and Ef for the backward and forward reactions, respectively. In general ...

An ideal gas expands in volume from 1$$\times$$10-3 m3 to 1 $$\times$$ 10-2 m3 at 300 K against a constant pressure of 1$$\times$$105 Nm-2. The work d...

The enthalpies of combustion of carbon and carbon monoxide are -393.5 and -283 kJ mol-1 respectively. The enthalpy of formation of carbon monoxide per...

The internal energy change when a system goes from state A to B is 40 kJ/mole. If the system goes from A to B by a reversible path and returns to sta...

In an irreversible process taking place at constant T and P and in which only pressure-volume work is being done, the change in Gibbs free energy (dG)...

The enthalpy change for a reaction does not depend upon

The correct relationship between free energy change in a reaction and the corresponding equilibrium constant Kc is

If at 298 K the bond energies of C - H, C - C, C = C and H - H bonds are respectively 414, 347, 615 and 435 kJ/mol, the value of enthalpy change for t...

If an endothermic reaction is non-spontaneous at freezing point of water and becomes feasible at its boiling point, then

For the reactions 2C + O2 $$\to$$ 2CO2; $$\Delta H$$ = -393 J 2Zn + O2 $$\to$$ 2ZnO; $$\Delta H$$ = -412 J

The heat required to raise the temperature of body by 1 K is called

A heat engine absorbs heat Q1 at temperature T1 and heat Q2 at temperature T2. Work done by the engine is J (Q1 + Q2). This data...