Probability · Mathematics · JEE Main
MCQ (Single Correct Answer)
If A and B are two events such that $P(A) = 0.7$, $P(B) = 0.4$ and $P(A \cap \overline{B}) = 0.5$, where $\overline{B}$ denotes the complement of B, then $P\left(B \mid (A \cup \overline{B})\right)$ is equal to
A bag contains 19 unbiased coins and one coin with head on both sides. One coin drawn at random is tossed and head turns up. If the probability that the drawn coin was unbiased, is $\frac{m}{n}$, $\gcd(m, n) = 1$, then $n^2 - m^2$ is equal to :
Let a random variable X take values 0, 1, 2, 3 with P(X=0)=P(X=1)=p, P(X=2)=P(X=3) and E(X2)=2E(X). Then the value of 8p−1 is :
The probability, of forming a 12 persons committee from 4 engineers, 2 doctors and 10 professors containing at least 3 engineers and at least 1 doctor, is
A box contains 10 pens of which 3 are defective. A sample of 2 pens is drawn at random and let $X$ denote the number of defective pens. Then the variance of $X$ is
If the probability that the random variable $X$ takes the value $x$ is given by
$P(X=x)=k(x+1) 3^{-x}, x=0,1,2,3 \ldots$, where $k$ is a constant, then $P(X \geq 3)$ is equal to
$$ \text { Given three indentical bags each containing } 10 \text { balls, whose colours are as follows : } $$
$$ \begin{array}{lccc} & \text { Red } & \text { Blue } & \text { Green } \\ \text { Bag I } & 3 & 2 & 5 \\ \text { Bag II } & 4 & 3 & 3 \\ \text { Bag III } & 5 & 1 & 4 \end{array} $$
A person chooses a bag at random and takes out a ball. If the ball is Red, the probability that it is from bag I is p and if the ball is Green, the probability that it is from bag III is $q$, then the value of $\left(\frac{1}{p}+\frac{1}{q}\right)$ is:Bag 1 contains 4 white balls and 5 black balls, and Bag 2 contains n white balls and 3 black balls. One ball is drawn randomly from Bag 1 and transferred to Bag 2. A ball is then drawn randomly from Bag 2. If the probability, that the ball drawn is white, is $ \frac{29}{45} $, then n is equal to:
Bag $B_1$ contains 6 white and 4 blue balls, Bag $B_2$ contains 4 white and 6 blue balls, and Bag $B_3$ contains 5 white and 5 blue balls. One of the bags is selected at random and a ball is drawn from it. If the ball is white, then the probability that the ball is drawn from Bag $B_2$ is:
Let S be the set of all the words that can be formed by arranging all the letters of the word GARDEN. From the set S, one word is selected at random. The probability that the selected word will NOT have vowels in alphabetical order is:
Two number $\mathrm{k}_1$ and $\mathrm{k}_2$ are randomly chosen from the set of natural numbers. Then, the probability that the value of $\mathrm{i}^{\mathrm{k}_1}+\mathrm{i}^{\mathrm{k}_2},(\mathrm{i}=\sqrt{-1})$ is non-zero, equals
Three defective oranges are accidently mixed with seven good ones and on looking at them, it is not possible to differentiate between them. Two oranges are drawn at random from the lot. If $x$ denote the number of defective oranges, then the variance of $x$ is
Let $\mathrm{A}=\left[\mathrm{a}_{\mathrm{ij}}\right]$ be a square matrix of order 2 with entries either 0 or 1 . Let E be the event that A is an invertible matrix. Then the probability $\mathrm{P}(\mathrm{E})$ is :
$A$ and $B$ alternately throw a pair of dice. A wins if he throws a sum of 5 before $B$ throws a sum of 8 , and $B$ wins if he throws a sum of 8 before $A$ throws a sum of 5 . The probability, that A wins if A makes the first throw, is
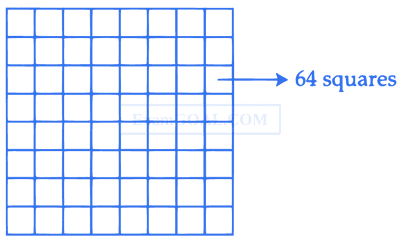
A board has 16 squares as shown in the figure :

Out of these 16 squares, two squares are chosen at random. The probability that they have no side in common is :
One die has two faces marked 1 , two faces marked 2 , one face marked 3 and one face marked 4 . Another die has one face marked 1 , two faces marked 2 , two faces marked 3 and one face marked 4. The probability of getting the sum of numbers to be 4 or 5 , when both the dice are thrown together, is
If $A$ and $B$ are two events such that $P(A \cap B)=0.1$, and $P(A \mid B)$ and $P(B \mid A)$ are the roots of the equation $12 x^2-7 x+1=0$, then the value of $\frac{P(\bar{A} \cup \bar{B})}{P(\bar{A} \cap \bar{B})}$ is :
A coin is tossed three times. Let $X$ denote the number of times a tail follows a head. If $\mu$ and $\sigma^2$ denote the mean and variance of $X$, then the value of $64\left(\mu+\sigma^2\right)$ is:
Two balls are selected at random one by one without replacement from a bag containing 4 white and 6 black balls. If the probability that the first selected ball is black, given that the second selected ball is also black, is $\frac{m}{n}$, where $\operatorname{gcd}(m, n)=1$, then $m+n$ is equal to :
If an unbiased dice is rolled thrice, then the probability of getting a greater number in the $$i^{\text {th }}$$ roll than the number obtained in the $$(i-1)^{\text {th }}$$ roll, $$i=2,3$$, is equal to
There are three bags $$X, Y$$ and $$Z$$. Bag $$X$$ contains 5 one-rupee coins and 4 five-rupee coins; Bag $$Y$$ contains 4 one-rupee coins and 5 five-rupee coins and Bag $$Z$$ contains 3 one-rupee coins and 6 five-rupee coins. A bag is selected at random and a coin drawn from it at random is found to be a one-rupee coin. Then the probability, that it came from bag $$\mathrm{Y}$$, is :
Let the sum of two positive integers be 24 . If the probability, that their product is not less than $$\frac{3}{4}$$ times their greatest possible product, is $$\frac{m}{n}$$, where $$\operatorname{gcd}(m, n)=1$$, then $$n$$-$$m$$ equals
If three letters can be posted to any one of the 5 different addresses, then the probability that the three letters are posted to exactly two addresses is :
A company has two plants $$A$$ and $$B$$ to manufacture motorcycles. $$60 \%$$ motorcycles are manufactured at plant $$A$$ and the remaining are manufactured at plant $$B .80 \%$$ of the motorcycles manufactured at plant $$A$$ are rated of the standard quality, while $$90 \%$$ of the motorcycles manufactured at plant $$B$$ are rated of the standard quality. A motorcycle picked up randomly from the total production is found to be of the standard quality. If $$p$$ is the probability that it was manufactured at plant $$B$$, then $$126 p$$ is
The coefficients $$\mathrm{a}, \mathrm{b}, \mathrm{c}$$ in the quadratic equation $$\mathrm{a} x^2+\mathrm{bx}+\mathrm{c}=0$$ are from the set $$\{1,2,3,4,5,6\}$$. If the probability of this equation having one real root bigger than the other is p, then 216p equals :
The coefficients $$a, b, c$$ in the quadratic equation $$a x^2+b x+c=0$$ are chosen from the set $$\{1,2,3,4,5,6,7,8\}$$. The probability of this equation having repeated roots is :
If the mean of the following probability distribution of a radam variable $$\mathrm{X}$$ :
| $$\mathrm{X}$$ | 0 | 2 | 4 | 6 | 8 |
|---|---|---|---|---|---|
| $$\mathrm{P(X)}$$ | $$a$$ | $$2a$$ | $$a+b$$ | $$2b$$ | $$3b$$ |
is $$\frac{46}{9}$$, then the variance of the distribution is
Three urns A, B and C contain 7 red, 5 black; 5 red, 7 black and 6 red, 6 black balls, respectively. One of the urn is selected at random and a ball is drawn from it. If the ball drawn is black, then the probability that it is drawn from urn $$\mathrm{A}$$ is :
A coin is biased so that a head is twice as likely to occur as a tail. If the coin is tossed 3 times, then the probability of getting two tails and one head is
Three rotten apples are accidently mixed with fifteen good apples. Assuming the random variable $$x$$ to be the number of rotten apples in a draw of two apples, the variance of $$x$$ is
Two marbles are drawn in succession from a box containing 10 red, 30 white, 20 blue and 15 orange marbles, with replacement being made after each drawing. Then the probability, that first drawn marble is red and second drawn marble is white, is
Bag A contains 3 white, 7 red balls and Bag B contains 3 white, 2 red balls. One bag is selected at random and a ball is drawn from it. The probability of drawing the ball from the bag A, if the ball drawn is white, is
Two integers $$x$$ and $$y$$ are chosen with replacement from the set $$\{0,1,2,3, \ldots, 10\}$$. Then the probability that $$|x-y|>5$$, is :
An integer is chosen at random from the integers $$1,2,3, \ldots, 50$$. The probability that the chosen integer is a multiple of atleast one of 4, 6 and 7 is
A fair die is thrown until 2 appears. Then the probability, that 2 appears in even number of throws, is
An urn contains 6 white and 9 black balls. Two successive draws of 4 balls are made without replacement. The probability, that the first draw gives all white balls and the second draw gives all black balls, is :
The random variable $$\mathrm{X}$$ follows binomial distribution $$\mathrm{B}(\mathrm{n}, \mathrm{p})$$, for which the difference of the mean and the variance is 1 . If $$2 \mathrm{P}(\mathrm{X}=2)=3 \mathrm{P}(\mathrm{X}=1)$$, then $$n^{2} \mathrm{P}(\mathrm{X}>1)$$ is equal to :
A coin is biased so that the head is 3 times as likely to occur as tail. This coin is tossed until a head or three tails occur. If $$\mathrm{X}$$ denotes the number of tosses of the coin, then the mean of $$\mathrm{X}$$ is :
Two dice A and B are rolled. Let the numbers obtained on A and B be $$\alpha$$ and $$\beta$$ respectively. If the variance of $$\alpha-\beta$$ is $$\frac{p}{q}$$, where $$p$$ and $$q$$ are co-prime, then the sum of the positive divisors of $$p$$ is equal to :
Let $$S=\left\{M=\left[a_{i j}\right], a_{i j} \in\{0,1,2\}, 1 \leq i, j \leq 2\right\}$$ be a sample space and $$A=\{M \in S: M$$ is invertible $$\}$$ be an event. Then $$P(A)$$ is equal to :
Let a die be rolled $$n$$ times. Let the probability of getting odd numbers seven times be equal to the probability of getting odd numbers nine times. If the probability of getting even numbers twice is $$\frac{k}{2^{15}}$$, then $$\mathrm{k}$$ is equal to :
Let N denote the sum of the numbers obtained when two dice are rolled. If the probability that $${2^N} < N!$$ is $${m \over n}$$, where m and n are coprime, then $$4m-3n$$ is equal to :
If the probability that the random variable $$\mathrm{X}$$ takes values $$x$$ is given by $$\mathrm{P}(\mathrm{X}=x)=\mathrm{k}(x+1) 3^{-x}, x=0,1,2,3, \ldots$$, where $$\mathrm{k}$$ is a constant, then $$\mathrm{P}(\mathrm{X} \geq 2)$$ is equal to :
In a bolt factory, machines $$A, B$$ and $$C$$ manufacture respectively $$20 \%, 30 \%$$ and $$50 \%$$ of the total bolts. Of their output 3, 4 and 2 percent are respectively defective bolts. A bolt is drawn at random from the product. If the bolt drawn is found the defective, then the probability that it is manufactured by the machine $$C$$ is :
Three dice are rolled. If the probability of getting different numbers on the three dice is $$\frac{p}{q}$$, where $$p$$ and $$q$$ are co-prime, then $$q-p$$ is equal to :
A pair of dice is thrown 5 times. For each throw, a total of 5 is considered a success. If the probability of at least 4 successes is $$\frac{k}{3^{11}}$$, then $$k$$ is equal to :
Two dice are thrown independently. Let $$\mathrm{A}$$ be the event that the number appeared on the $$1^{\text {st }}$$ die is less than the number appeared on the $$2^{\text {nd }}$$ die, $$\mathrm{B}$$ be the event that the number appeared on the $$1^{\text {st }}$$ die is even and that on the second die is odd, and $$\mathrm{C}$$ be the event that the number appeared on the $$1^{\text {st }}$$ die is odd and that on the $$2^{\text {nd }}$$ is even. Then :
In a binomial distribution $$B(n,p)$$, the sum and the product of the mean and the variance are 5 and 6 respectively, then $$6(n+p-q)$$ is equal to :
A bag contains 6 balls. Two balls are drawn from it at random and both are found to be black. The probability that the bag contains at least 5 black balls is :
If an unbiased die, marked with $$-2,-1,0,1,2,3$$ on its faces, is thrown five times, then the probability that the product of the outcomes is positive, is :
Let $$\mathrm{S} = \{ {w_1},{w_2},......\} $$ be the sample space associated to a random experiment. Let $$P({w_n}) = {{P({w_{n - 1}})} \over 2},n \ge 2$$. Let $$A = \{ 2k + 3l:k,l \in N\} $$ and $$B = \{ {w_n}:n \in A\} $$. Then P(B) is equal to :
Fifteen football players of a club-team are given 15 T-shirts with their names written on the backside. If the players pick up the T-shirts randomly, then the probability that at least 3 players pick the correct T-shirt is :
Let N be the sum of the numbers appeared when two fair dice are rolled and let the probability that $$N-2,\sqrt{3N},N+2$$ are in geometric progression be $$\frac{k}{48}$$. Then the value of k is :
Let M be the maximum value of the product of two positive integers when their sum is 66. Let the sample space $$S = \left\{ {x \in \mathbb{Z}:x(66 - x) \ge {5 \over 9}M} \right\}$$ and the event $$\mathrm{A = \{ x \in S:x\,is\,a\,multiple\,of\,3\}}$$. Then P(A) is equal to :
Let N denote the number that turns up when a fair die is rolled. If the probability that the system of equations
$$x + y + z = 1$$
$$2x + \mathrm{N}y + 2z = 2$$
$$3x + 3y + \mathrm{N}z = 3$$
has unique solution is $${k \over 6}$$, then the sum of value of k and all possible values of N is :
Let $$\Omega$$ be the sample space and $$\mathrm{A \subseteq \Omega}$$ be an event.
Given below are two statements :
(S1) : If P(A) = 0, then A = $$\phi$$
(S2) : If P(A) = 1, then A = $$\Omega$$
Then :
Bag I contains 3 red, 4 black and 3 white balls and Bag II contains 2 red, 5 black and 2 white balls. One ball is transferred from Bag I to Bag II and then a ball is drawn from Bag II. The ball so drawn is found to be black in colour. Then the probability, that the transferred ball is red, is :
Let $$S=\{1,2,3, \ldots, 2022\}$$. Then the probability, that a randomly chosen number n from the set S such that $$\mathrm{HCF}\,(\mathrm{n}, 2022)=1$$, is :
Let $$\mathrm{A}$$ and $$\mathrm{B}$$ be two events such that $$P(B \mid A)=\frac{2}{5}, P(A \mid B)=\frac{1}{7}$$ and $$P(A \cap B)=\frac{1}{9} \cdot$$ Consider
(S1) $$P\left(A^{\prime} \cup B\right)=\frac{5}{6}$$,
(S2) $$P\left(A^{\prime} \cap B^{\prime}\right)=\frac{1}{18}$$
Then :
Out of $$60 \%$$ female and $$40 \%$$ male candidates appearing in an exam, $$60 \%$$ candidates qualify it. The number of females qualifying the exam is twice the number of males qualifying it. A candidate is randomly chosen from the qualified candidates. The probability, that the chosen candidate is a female, is :
Let X have a binomial distribution B(n, p) such that the sum and the product of the mean and variance of X are 24 and 128 respectively. If $$P(X>n-3)=\frac{k}{2^{n}}$$, then k is equal to :
A six faced die is biased such that
$$3 \times \mathrm{P}($$a prime number$$)\,=6 \times \mathrm{P}($$a composite number$$)\,=2 \times \mathrm{P}(1)$$.
Let X be a random variable that counts the number of times one gets a perfect square on some throws of this die. If the die is thrown twice, then the mean of X is :
Let $$S$$ be the sample space of all five digit numbers. It $$p$$ is the probability that a randomly selected number from $$S$$, is a multiple of 7 but not divisible by 5 , then $$9 p$$ is equal to :
Let $$X$$ be a binomially distributed random variable with mean 4 and variance $$\frac{4}{3}$$. Then, $$54 \,P(X \leq 2)$$ is equal to :
The mean and variance of a binomial distribution are $$\alpha$$ and $$\frac{\alpha}{3}$$ respectively. If $$\mathrm{P}(X=1)=\frac{4}{243}$$, then $$\mathrm{P}(X=4$$ or 5$$)$$ is equal to :
Let $$\mathrm{E}_{1}, \mathrm{E}_{2}, \mathrm{E}_{3}$$ be three mutually exclusive events such that $$\mathrm{P}\left(\mathrm{E}_{1}\right)=\frac{2+3 \mathrm{p}}{6}, \mathrm{P}\left(\mathrm{E}_{2}\right)=\frac{2-\mathrm{p}}{8}$$ and $$\mathrm{P}\left(\mathrm{E}_{3}\right)=\frac{1-\mathrm{p}}{2}$$. If the maximum and minimum values of $$\mathrm{p}$$ are $$\mathrm{p}_{1}$$ and $$\mathrm{p}_{2}$$, then $$\left(\mathrm{p}_{1}+\mathrm{p}_{2}\right)$$ is equal to :
If $$A$$ and $$B$$ are two events such that $$P(A)=\frac{1}{3}, P(B)=\frac{1}{5}$$ and $$P(A \cup B)=\frac{1}{2}$$, then $$P\left(A \mid B^{\prime}\right)+P\left(B \mid A^{\prime}\right)$$ is equal to :
If the sum and the product of mean and variance of a binomial distribution are 24 and 128 respectively, then the probability of one or two successes is :
If the numbers appeared on the two throws of a fair six faced die are $$\alpha$$ and $$\beta$$, then the probability that $$x^{2}+\alpha x+\beta>0$$, for all $$x \in \mathbf{R}$$, is :
If a random variable X follows the Binomial distribution B(5, p) such that P(X = 0) = P(X = 1), then $${{P(X = 2)} \over {P(X = 3)}}$$ is equal to :
The probability that a relation R from {x, y} to {x, y} is both symmetric and transitive, is equal to :
The probability that a randomly chosen 2 $$\times$$ 2 matrix with all the entries from the set of first 10 primes, is singular, is equal to :
The probability that a randomly chosen one-one function from the set {a, b, c, d} to the set {1, 2, 3, 4, 5} satisfies f(a) + 2f(b) $$-$$ f(c) = f(d) is :
The probability, that in a randomly selected 3-digit number at least two digits are odd, is :
If a point A(x, y) lies in the region bounded by the y-axis, straight lines 2y + x = 6 and 5x $$-$$ 6y = 30, then the probability that y < 1 is :
Five numbers $${x_1},{x_2},{x_3},{x_4},{x_5}$$ are randomly selected from the numbers 1, 2, 3, ......., 18 and are arranged in the increasing order $$({x_1} < {x_2} < {x_3} < {x_4} < {x_5})$$. The probability that $${x_2} = 7$$ and $${x_4} = 11$$ is :
Let X be a random variable having binomial distribution B(7, p). If P(X = 3) = 5P(x = 4), then the sum of the mean and the variance of X is :
Let a biased coin be tossed 5 times. If the probability of getting 4 heads is equal to the probability of getting 5 heads, then the probability of getting atmost two heads is :
A biased die is marked with numbers 2, 4, 8, 16, 32, 32 on its faces and the probability of getting a face with mark n is $${1 \over n}$$. If the die is thrown thrice, then the probability, that the sum of the numbers obtained is 48, is :
Let E1 and E2 be two events such that the conditional probabilities $$P({E_1}|{E_2}) = {1 \over 2}$$, $$P({E_2}|{E_1}) = {3 \over 4}$$ and $$P({E_1} \cap {E_2}) = {1 \over 8}$$. Then :
A random variable X has the following probability distribution :
| X | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| P(X) | k | 2k | 4k | 6k | 8k |
The value of P(1 < X < 4 | X $$\le$$ 2) is equal to :
Bag A contains 2 white, 1 black and 3 red balls and bag B contains 3 black, 2 red and n white balls. One bag is chosen at random and 2 balls drawn from it at random, are found to be 1 red and 1 black. If the probability that both balls come from Bag A is $${6 \over {11}}$$, then n is equal to __________.
If a random variable X follows the Binomial distribution B(33, p) such that
$$3P(X = 0) = P(X = 1)$$, then the value of $${{P(X = 15)} \over {P(X = 18)}} - {{P(X = 16)} \over {P(X = 17)}}$$ is equal to :
x + y + z = 5
x + 2y + 3z = $$\mu$$
x + 3y + $$\lambda$$z = 1
is constructed. If p is the probability that the system has a unique solution and q is the probability that the system has no solution, then :
P(A) = 0.6, P(B) = 0.4 and P(C) = 0.5.
If P(A$$ \cup $$B) = 0.8, P(A$$ \cap $$C) = 0.3, P(A$$ \cap $$B$$ \cap $$C) = 0.2, P(B$$ \cap $$C) = $$\beta $$
and P(A$$ \cup $$B$$ \cup $$C) = $$\alpha $$, where 0.85 $$ \le \alpha \le $$ 0.95, then $$\beta $$ lies in the interval :
and P(E1 $$ \cap $$ E2 $$ \cap $$ E3) = 0.
Then P($$E_2^C \cap E_3^C/{E_1}$$) is equal to :
| X: | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| P(X): | K2 | 2K | K | 2K | 5K2 |
Then P(X > 2) is equal to :
P(A) = $${1 \over 3}$$ and P(B) = $${1 \over 6}$$.
Then, which of the following is TRUE?
P(Exactly one of A or B occurs)
= P(Exactly one of B or C occurs)
= P (Exactly one of C or A occurs) = $${1 \over 4}$$
and P(All the three events occur simultaneously) = $${1 \over {16}}$$.
Then the probability that at least one of the events occurs, is :
Statement - 1: The probability that the chosen numbers when arranged in some order will form an AP is $${1 \over {85}}.$$
Statement - 2: If the four chosen numbers form an AP, then the set of all possible values of common difference is $$\left( { \pm 1, \pm 2, \pm 3, \pm 4, \pm 5} \right).$$
$$P\left( A \right) = {1 \over 4},P\left( {A|B} \right) = {1 \over 2}$$ and $$P\left( {B|A} \right) = {2 \over 3}.$$ Then $$P(B)$$ is :
Then $$P\left( {X > 1.5} \right)$$ equals :
$$P\left( {\overline A } \right) = 2/3$$ then $$P\left( {\overline A \cap B} \right)$$ is :
Numerical
A card from a pack of 52 cards is lost. From the remaining 51 cards, n cards are drawn and are found to be spades. If the probability of the lost card to be a spade is $\frac{11}{50}$, then n is equal to ________ .
Three distinct numbers are selected randomly from the set $\{1,2,3, \ldots, 40\}$. If the probability, that the selected numbers are in an increasing G.P., is $\frac{m}{n}, \operatorname{gcd}(m, n)=1$, then $m+n$ is equal to __________ .
Let $$\mathrm{a}, \mathrm{b}$$ and $$\mathrm{c}$$ denote the outcome of three independent rolls of a fair tetrahedral die, whose four faces are marked $$1,2,3,4$$. If the probability that $$a x^2+b x+c=0$$ has all real roots is $$\frac{m}{n}, \operatorname{gcd}(\mathrm{m}, \mathrm{n})=1$$, then $$\mathrm{m}+\mathrm{n}$$ is equal to _________.
Three balls are drawn at random from a bag containing 5 blue and 4 yellow balls. Let the random variables $$X$$ and $$Y$$ respectively denote the number of blue and yellow balls. If $$\bar{X}$$ and $$\bar{Y}$$ are the means of $$X$$ and $$Y$$ respectively, then $$7 \bar{X}+4 \bar{Y}$$ is equal to ___________.
From a lot of 12 items containing 3 defectives, a sample of 5 items is drawn at random. Let the random variable $$X$$ denote the number of defective items in the sample. Let items in the sample be drawn one by one without replacement. If variance of $$X$$ is $$\frac{m}{n}$$, where $$\operatorname{gcd}(m, n)=1$$, then $$n-m$$ is equal to _________.
From a lot of 10 items, which include 3 defective items, a sample of 5 items is drawn at random. Let the random variable $$X$$ denote the number of defective items in the sample. If the variance of $$X$$ is $$\sigma^2$$, then $$96 \sigma^2$$ is equal to __________.
In a tournament, a team plays 10 matches with probabilities of winning and losing each match as $$\frac{1}{3}$$ and $$\frac{2}{3}$$ respectively. Let $$x$$ be the number of matches that the team wins, and $y$ be the number of matches that team loses. If the probability $$\mathrm{P}(|x-y| \leq 2)$$ is $$p$$, then $$3^9 p$$ equals _________.
A group of 40 students appeared in an examination of 3 subjects - Mathematics, Physics and Chemistry. It was found that all students passed in atleast one of the subjects, 20 students passed in Mathematics, 25 students passed in Physics, 16 students passed in Chemistry, atmost 11 students passed in both Mathematics and Physics, atmost 15 students passed in both Physics and Chemistry, atmost 15 students passed in both Mathematics and Chemistry. The maximum number of students passed in all the three subjects is _________.
$a=P(X=3), b=P(X \geqslant 3)$ and $c=P(X \geqslant 6 \mid X>3)$. Then $\frac{b+c}{a}$ is equal to __________.
A fair $$n(n > 1)$$ faces die is rolled repeatedly until a number less than $$n$$ appears. If the mean of the number of tosses required is $$\frac{n}{9}$$, then $$n$$ is equal to ____________.
Let the probability of getting head for a biased coin be $$\frac{1}{4}$$. It is tossed repeatedly until a head appears. Let $$\mathrm{N}$$ be the number of tosses required. If the probability that the equation $$64 \mathrm{x}^{2}+5 \mathrm{Nx}+1=0$$ has no real root is $$\frac{\mathrm{p}}{\mathrm{q}}$$, where $$\mathrm{p}$$ and $$\mathrm{q}$$ are coprime, then $$q-p$$ is equal to ________.
25% of the population are smokers. A smoker has 27 times more chances to develop lung cancer than a non smoker. A person is diagnosed with lung cancer and the probability that this person is a smoker is $$\frac{k}{10}%$$. Then the value of k is __________.
Three urns A, B and C contain 4 red, 6 black; 5 red, 5 black; and $$\lambda$$ red, 4 black balls respectively. One of the urns is selected at random and a ball is drawn. If the ball drawn is red and the probability that it is drawn from urn C is 0.4 then the square of the length of the side of the largest equilateral triangle, inscribed in the parabola $$y^2=\lambda x$$ with one vertex at the vertex of the parabola, is :
The sum and product of the mean and variance of a binomial distribution are 82.5 and 1350 respectively. Then the number of trials in the binomial distribution is ____________.
A bag contains 4 white and 6 black balls. Three balls are drawn at random from the bag. Let $$\mathrm{X}$$ be the number of white balls, among the drawn balls. If $$\sigma^{2}$$ is the variance of $$\mathrm{X}$$, then $$100 \sigma^{2}$$ is equal to ________.
The probability distribution of X is :
| X | 0 | 1 | 2 | 3 |
|---|---|---|---|---|
| P(X) | $${{1 - d} \over 4}$$ | $${{1 + 2d} \over 4}$$ | $${{1 - 4d} \over 4}$$ | $${{1 + 3d} \over 4}$$ |
For the minimum possible value of d, sixty times the mean of X is equal to _______________.
Let S = {E1, E2, ........., E8} be a sample space of a random experiment such that $$P({E_n}) = {n \over {36}}$$ for every n = 1, 2, ........, 8. Then the number of elements in the set $$\left\{ {A \subseteq S:P(A) \ge {4 \over 5}} \right\}$$ is ___________.
If the probability that a randomly chosen 6-digit number formed by using digits 1 and 8 only is a multiple of 21 is p, then 96 p is equal to _______________.
In an examination, there are 10 true-false type questions. Out of 10, a student can guess the answer of 4 questions correctly with probability $${3 \over 4}$$ and the remaining 6 questions correctly with probability $${1 \over 4}$$. If the probability that the student guesses the answers of exactly 8 questions correctly out of 10 is $${{{{27}k}} \over {{4^{10}}}}$$, then k is equal to ___________.
| x | $$ - $$2 | $$ - $$1 | 3 | 4 | 6 |
|---|---|---|---|---|---|
| P(X = x) | $${1 \over 5}$$ | a | $${1 \over 3}$$ | $${1 \over 5}$$ | b |
If the mean of X is 2.3 and variance of X is $$\sigma$$2, then 100 $$\sigma$$2 is equal to :
| X | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| P(X) | K | 2K | 2K | 3K | K |
Let p = P(1 < X < 4 | X < 3). If 5p = $$\lambda$$K, then $$\lambda$$ equal to ___________.
($$\alpha$$ $$-$$ 2$$\beta$$)p = $$\alpha$$$$\beta$$ and ($$\beta$$ $$-$$ 3$$\gamma$$)p = 2$$\beta$$$$\gamma$$. All the given probabilities are assumed to lie in the interval (0, 1).
Then, $$\frac{Probability\ of\ occurrence\ of\ E_{1}}{Probability\ of\ occurrence\ of\ E_{3}} $$ is equal to _____________.
Then $${{P\left( {{B_1}} \right)} \over {P\left( {{B_3}} \right)}}$$ is equal to ________.