Given below are two statements. One is labelled as Assertion (A) and the other is labelled as Reason (R).

Assertion (A): Three identical spheres of same mass undergo one dimensional motion as shown in figure with initial velocities $v_{\mathrm{A}}=5 \mathrm{~m} / \mathrm{s}, v_{\mathrm{B}}=2 \mathrm{~m} / \mathrm{s}, v_{\mathrm{C}}=4 \mathrm{~m} / \mathrm{s}$. If we wait sufficiently long for elastic collision to happen, then $v_{\mathrm{A}}=4 \mathrm{~m} / \mathrm{s}, v_{\mathrm{B}}=2 \mathrm{~m} / \mathrm{s}$, $v_{\mathrm{C}}=5 \mathrm{~m} / \mathrm{s}$ will be the final velocities.

Reason (R): In an elastic collision between identical masses, two objects exchange their velocities.

In the light of the above statements, choose the correct answer from the options given below:

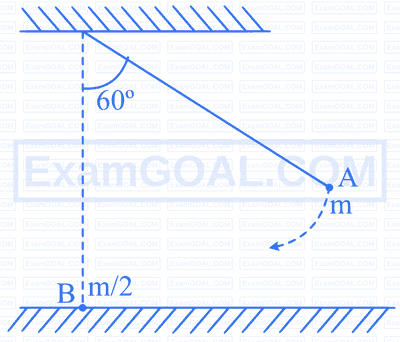
As shown below, bob A of a pendulum having massless string of length 'R' is released from 60° to the vertical. It hits another bob B of half the mass that is at rest on a frictionless table in the center. Assuming elastic collision, the magnitude of the velocity of bob A after the collision will be (take g as acceleration due to gravity.)

The center of mass of a thin rectangular plate (fig - x ) with sides of length $a$ and $b$, whose mass per unit area $(\sigma)$ varies as $\sigma=\frac{\sigma_0 x}{a b}$ (where $\sigma_0$ is a constant), would be __________.

Consider a circular disc of radius 20 cm with centre located at the origin. A circular hole of radius 5 cm is cut from this disc in such a way that the edge of the hole touches the edge of the disc. The distance of centre of mass of residual or remaining disc from the origin will be