Eight players $${P_1},{P_2},.....{P_8}$$ play a knock-out tournament. It is known that whenever the players $${P_i}$$ and $${P_j}$$ play, the player $${P_i}$$ will win if $$i < j.$$ Assuming that the players are paired at random in each round, what is the probability that the player $${P_4}$$ reaches the final?

Three players, $$A,B$$ and $$C,$$ toss a coin cyclically in that order (that is $$A, B, C, A, B, C, A, B,...$$) till a head shows. Let $$p$$ be the probability that the coin shows a head. Let $$\alpha ,\,\,\,\beta $$ and $$\gamma $$ be, respectively, the probabilities that $$A, B$$ and $$C$$ gets the first head. Prove that $$\beta = \left( {1 - p} \right)\alpha $$ Determine $$\alpha ,\beta $$ and $$\gamma $$ (in terms of $$p$$).

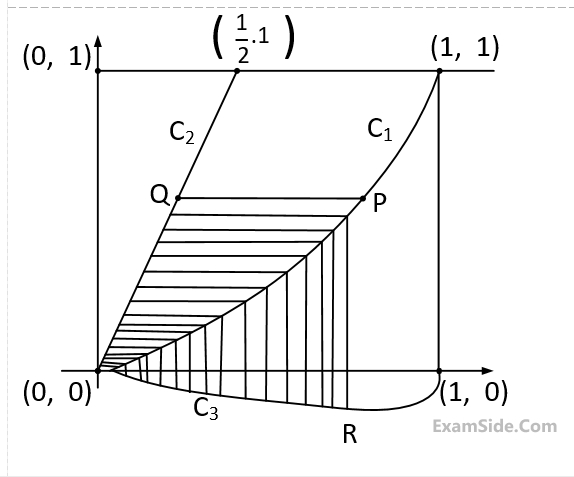
Let $${C_1}$$ and $${C_2}$$ be the graphs of the functions $$y = {x^2}$$ and $$y = 2x,$$ $$0 \le x \le 1$$ respectively. Let $${C_3}$$ be the graph of a function $$y=f(x),$$ $$0 \le x \le 1,$$ $$f(0)=0.$$ For a point $$P$$ on $${C_1},$$ let the lines through $$P,$$ parallel to the axes, meet $${C_2}$$ and $${C_3}$$ at $$Q$$ and $$R$$ respectively (see figure.) If for every position of $$P$$ (on $${C_1}$$ ), the areas of the shaded regions $$OPQ$$ and $$ORP$$ are equal, determine the function$$f(x).$$

If $$p$$ and $$q$$ are chosen randomly from the set $$\left\{ {1,2,3,4,5,6,7,8,9,10} \right\},$$ with replacement, determine the probability that the roots of the equation $${x^2} + px + q = 0$$ are real.