Functions · Mathematics · JEE Advanced
Numerical
Let the function $f: \mathbb{R} \rightarrow \mathbb{R}$ be defined by
$$ f(x)=\frac{\sin x}{e^{\pi x}} \frac{\left(x^{2023}+2024 x+2025\right)}{\left(x^2-x+3\right)}+\frac{2}{e^{\pi x}} \frac{\left(x^{2023}+2024 x+2025\right)}{\left(x^2-x+3\right)} . $$
Then the number of solutions of $f(x)=0$ in $\mathbb{R}$ is _________.
$$f(x) = {{{4^x}} \over {{4^x} + 2}}$$
Then the value of $$f\left( {{1 \over {40}}} \right) + f\left( {{2 \over {40}}} \right) + f\left( {{3 \over {40}}} \right) + ... + f\left( {{{39} \over {40}}} \right) - f\left( {{1 \over 2}} \right)$$ is ..........
Suppose the function f has a local minimum at $$\theta $$ precisely when $$\theta \in \{ {\lambda _1}\pi ,....,{\lambda _r}\pi \} $$, where $$0 < {\lambda _1} < ...{\lambda _r} < 1$$. Then the value of $${\lambda _1} + ... + {\lambda _r}$$ is .............
$$f(x) = (3 - \sin (2\pi x))\sin \left( {\pi x - {\pi \over 4}} \right) - \sin \left( {3\pi x + {\pi \over 4}} \right)$$
If $$\alpha ,\,\beta \in [0,2]$$ are such that $$\{ x \in [0,2]:f(x) \ge 0\} = [\alpha ,\beta ]$$, then the value of $$\beta - \alpha $$ is ..........
$$S = \{ {({x^2} - 1)^2}({a_0} + {a_1}x + {a_2}{x^2} + {a_3}{x^3}):{a_0},{a_1},{a_2},{a_3} \in R\} $$;
For a polynomial f, let f' and f'' denote its first and second order derivatives, respectively. Then the minimum possible value of (mf' + mf''), where f $$ \in $$ S, is ..............
If the function $$f(x) = {x^3} + {e^{x/2}}$$ and $$g(x) = {f^{ - 1}}(x)$$, then the value of $$g'(1)$$ is _________.
MCQ (More than One Correct Answer)
Let $$|M|$$ denote the determinant of a square matrix $$M$$. Let $$g:\left[0, \frac{\pi}{2}\right] \rightarrow \mathbb{R}$$ be the function defined by
$$ g(\theta)=\sqrt{f(\theta)-1}+\sqrt{f\left(\frac{\pi}{2}-\theta\right)-1} $$
where
$$ f(\theta)=\frac{1}{2}\left|\begin{array}{ccc} 1 & \sin \theta & 1 \\ -\sin \theta & 1 & \sin \theta \\ -1 & -\sin \theta & 1 \end{array}\right|+\left|\begin{array}{ccc} \sin \pi & \cos \left(\theta+\frac{\pi}{4}\right) & \tan \left(\theta-\frac{\pi}{4}\right) \\ \sin \left(\theta-\frac{\pi}{4}\right) & -\cos \frac{\pi}{2} & \log _{e}\left(\frac{4}{\pi}\right) \\ \cot \left(\theta+\frac{\pi}{4}\right) & \log _{e}\left(\frac{\pi}{4}\right) & \tan \pi \end{array}\right| . $$
Let $$p(x)$$ be a quadratic polynomial whose roots are the maximum and minimum values of the function $$g(\theta)$$, and $$p(2)=2-\sqrt{2}$$. Then, which of the following is/are TRUE ?
Let $$f(x) = \sin \left( {{\pi \over 6}\sin \left( {{\pi \over 2}\sin x} \right)} \right)$$ for all $$x \in R$$ and g(x) = $${{\pi \over 2}\sin x}$$ for all x$$\in$$R. Let $$(f \circ g)(x)$$ denote f(g(x)) and $$(g \circ f)(x)$$ denote g(f(x)). Then which of the following is/are true?
Let $$f:( - 1,1) \to R$$ be such that $$f(\cos 4\theta ) = {2 \over {2 - {{\sec }^2}\theta }}$$ for $$\theta \in \left( {0,{\pi \over 4}} \right) \cup \left( {{\pi \over 4},{\pi \over 2}} \right)$$. Then the value(s) of $$f\left( {{1 \over 3}} \right)$$ is(are)
Let $$f:(0,1) \to R$$ be defined by $$f(x) = {{b - x} \over {1 - bx}}$$, where b is a constant such that $$0 < b < 1$$. Then
MCQ (Single Correct Answer)
$${E_2} = \left\{ \matrix{ x \in {E_1}:{\sin ^{ - 1}}\left( {{{\log }_e}\left( {{x \over {x - 1}}} \right)} \right) \hfill \cr is\,a\,real\,number \hfill \cr} \right\}$$
(Here, the inverse trigonometric function $${\sin ^{ - 1}}$$ x assumes values in $$\left[ { - {\pi \over 2},{\pi \over 2}} \right]$$.).
Let f : E1 $$ \to $$ R be the function defined by f(x) = $${{{\log }_e}\left( {{x \over {x - 1}}} \right)}$$ and g : E2 $$ \to $$ R be the function defined by g(x) = $${\sin ^{ - 1}}\left( {{{\log }_e}\left( {{x \over {x - 1}}} \right)} \right)$$.
| LIST-I | LIST-II |
|---|---|
| P. The range of $f$ is | 1. $\left( -\infty, \frac{1}{1-e} \right] \cup \left[ \frac{e}{e-1}, \infty \right)$ |
| Q. The range of $g$ contains | 2. $(0, 1)$ |
| R. The domain of $f$ contains | 3. $\left[ -\frac{1}{2}, \frac{1}{2} \right]$ |
| S. The domain of $g$ is | 4. $(-\infty, 0) \cup (0, \infty)$ |
| 5. $\left( -\infty, \frac{e}{e-1} \right)$ | |
| 6. $(-\infty, 0) \cup \left( \frac{1}{2}, \frac{e}{e-1} \right]$ |
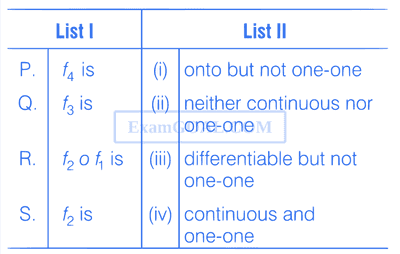
$${f_1}\left( x \right) = \left\{ {\matrix{ {\left| x \right|} & {if\,x < 0,} \cr {{e^x}} & {if\,x \ge 0;} \cr } } \right.$$
f2(x) = x2 ;
$${f_3}\left( x \right) = \left\{ {\matrix{ {\sin x} & {if\,x < 0,} \cr x & {if\,x \ge 0;} \cr } } \right.$$and
$${f_4}\left( x \right) = \left\{ {\matrix{ {{f_2}\left( {{f_1}\left( x \right)} \right)} & {if\,x < 0,} \cr {{f_2}\left( {{f_1}\left( x \right)} \right) - 1} & {if\,x \ge 0;} \cr } } \right.$$
The function $$f:[0,3] \to [1,29]$$, defined by $$f(x) = 2{x^3} - 15{x^2} + 36x + 1$$, is
Let f(x) = x2 and g(x) = sin x for all x $$\in$$ R. Then the set of all x satisfying $$(f \circ g \circ g \circ f)(x) = (g \circ g \circ f)(x)$$, where $$(f \circ g)(x) = f(g(x))$$, is
Match the statements given in Column I with the intervals/union of intervals given in Column II :
Let $f, g$ and $h$ be real valued functions defined on the interval $[0,1]$ by
$f(x)=e^{x^2}+e^{-x^2}$,
$g(x)=x e^{x^2}+e^{-x^2}$
and $h(x)=x^2 e^{x^2}+e^{-x^2}$.
If $a, b$ and $c$ denote, respectively, the absolute maximum of $f, g$ and $h$ on $[0,1]$, then :
Consider the polynomial
$$f\left( x \right) = 1 + 2x + 3{x^2} + 4{x^3}.$$
Let $$s$$ be the sum of all distinct real roots of $$f(x)$$ and let $$t = \left| s \right|.$$
The real numbers lies in the interval
Consider the polynomial
$$f\left( x \right) = 1 + 2x + 3{x^2} + 4{x^3}.$$
Let $$s$$ be the sum of all distinct real roots of $$f(x)$$ and let $$t = \left| s \right|.$$
The function$$f'(x)$$ is