Simple Harmonic Motion · Physics · JEE Advanced

Numerical

If the collision occurs at time $t_0=0$, the value of $v_{\mathrm{cm}} /(a \omega)$ will be ______.

JEE Advanced 2024 Paper 2 Online

If the collision occurs at time $t_0=\pi /(2 \omega)$, then the value of $4 b^2 / a^2$ will be ______.

JEE Advanced 2024 Paper 2 Online

A particle of mass $1 \mathrm{~kg}$ is subjected to a force which depends on the position as $\vec{F}=$ $-k(x \hat{\imath}+y \hat{\jmath}) \mathrm{kg}\, \mathrm{m} \mathrm{s}^{-2}$ with $k=1 \mathrm{~kg} \mathrm{~s}^{-2}$. At time $t=0$, the particle's position $\vec{r}=$ $\left(\frac{1}{\sqrt{2}} \hat{\imath}+\sqrt{2} \hat{\jmath}\right) m$ and its velocity $\vec{v}=\left(-\sqrt{2} \hat{\imath}+\sqrt{2} \hat{\jmath}+\frac{2}{\pi} \hat{k}\right) m s^{-1}$. Let $v_{x}$ and $v_{y}$ denote the $x$ and the $y$ components of the particle's velocity, respectively. Ignore gravity. When $z=0.5 \mathrm{~m}$, the value of $\left(x v_{y}-y v_{x}\right)$ is __________ $m^{2} s^{-1}$.

JEE Advanced 2022 Paper 2 Online

On a frictionless horizontal plane, a bob of mass $m=0.1 \mathrm{~kg}$ is attached to a spring with natural length $l_{0}=0.1 \mathrm{~m}$. The spring constant is $k_{1}=0.009 \,\mathrm{Nm}^{-1}$ when the length of the spring $l>l_{0}$ and is $k_{2}=0.016 \,\mathrm{Nm}^{-1}$ when $l < l_{0}$. Initially the bob is released from $l=$ $0.15 \mathrm{~m}$. Assume that Hooke's law remains valid throughout the motion. If the time period of the full oscillation is $T=(n \pi) s$, then the integer closest to $n$ is __________.

JEE Advanced 2022 Paper 2 Online

A 0.1 kg mass is suspended from a wire of negligible mass. The length of the wire is 1 m and its crosssectional area is 4.9 $$ \times $$ 10^-7 m². If the mass is pulled a little in the vertically downward direction and released, it performs simple harmonic motion of angular frequency 140 rad s⁻¹. If the Young’s modulus of the material of the wire is n $$ \times $$ 10⁹ Nm^-2, the value of n is

IIT-JEE 2010 Paper 1 Offline

An object of mass 0.2 kg executes simple harmonic oscillation along the x-axis with a frequency of $$\left( {{{25} \over \pi }} \right)$$ Hz. At the position x = 0.04, the object has kinetic energy of 0.5 J and potential energy 0.4 J. The amplitude of oscillations is ................ m.

IIT-JEE 1994

MCQ (More than One Correct Answer)

A block with mass M is connected by a massless spring with stiffness constant k to a rigid wall and moves without friction on a horizontal surface. The block oscillates with small amplitude A about an equilibrium position x₀. Consider two cases:
(i) when the block is at x₀; and
(ii) when the block is at x = x₀ + A.
In both cases, a particle with mass m( < M) is softly placed on the block after which they stick on each other. Which of the following statement(s) is(are) true about the motion after the mass m is placed on the mass M?

JEE Advanced 2016 Paper 2 Offline

Two independent harmonic oscillators of equal masses are oscillating about the origin with angular frequencies $$\omega$$₁ and $$\omega$$₂ and have total energies E₁ and E₂, respectively. The variations of their momenta p with positions x are shown in the figures. If $${a \over b} = {n^2}$$ and $${a \over R} = n$$, then the correct equations is/are

JEE Advanced 2015 Paper 1 Offline

A student performed the experiment to measure the speed of sound in air using resonance air-column method. Two resonances in the air-column were obtained by lowering the water level. The resonance with the shorter air-column is the first resonance and that with the longer air-column is the second resonance. Then,

IIT-JEE 2009 Paper 2 Offline

MCQ (Single Correct Answer)

A small block is connected to one end of a massless spring of un-stretched length 4.9 m. The other end of the spring (see the figure) is fixed. The system lies on a horizontal frictionless surface. The block is stretched by 0.2 m and released from rest at t = 0. It then executes simple harmonic motion with angular frequency $$\omega$$ = ($$\pi$$/3) rad/s. Simultaneously, at t = 0, a small pebble is projected with speed v from point P at an angle of 45$$^\circ$$ as shown in the figure. Point O is at a horizontal distance of 10 m from O. If the pebble hits the block at t = 1 s, the value of v is (take g = 10 m/s²)

IIT-JEE 2012 Paper 1 Offline

The phase space diagram for a ball thrown vertically up from ground is

IIT-JEE 2011 Paper 1 Offline

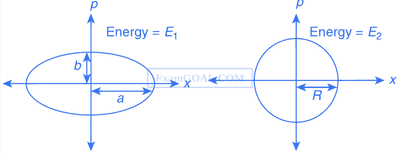
The phase space diagram for simple harmonic motion is a circle centred at the origin. In the figure, the two circles represent the same oscillator but for different initial conditions, and E₁ and E₂ are the total mechanical energies respectively. Then

IIT-JEE 2011 Paper 1 Offline

Consider the spring-mass system, with the mass submerged in water, as shown in the figure. The phase space diagram for one cycle of this system is

IIT-JEE 2011 Paper 1 Offline

A point mass is subjected to two simultaneous sinusoidal displacements in x-direction, $${x_1}\left( t \right) = A\sin \omega t$$ and $${x_2}\left( t \right) = A\sin \left( {\omega t + {{2\pi } \over 3}} \right)$$. Adding a third sinusoidal displacement $${x_3}\left( t \right) = B\sin \left( {\omega t + \phi } \right)$$ brings the mass to a complete rest. The values of B and $$\phi $$ are

IIT-JEE 2011 Paper 2 Offline

A wooden block performs $$SHM$$ on a frictionless surface with frequency, $${v_0}.$$ The block carries a charge $$+Q$$ on its surface . If now a uniform electric field $$\overrightarrow E $$ is switched- on as shown, then the $$SHM$$ of the block will be

IIT-JEE 2011 Paper 2 Offline

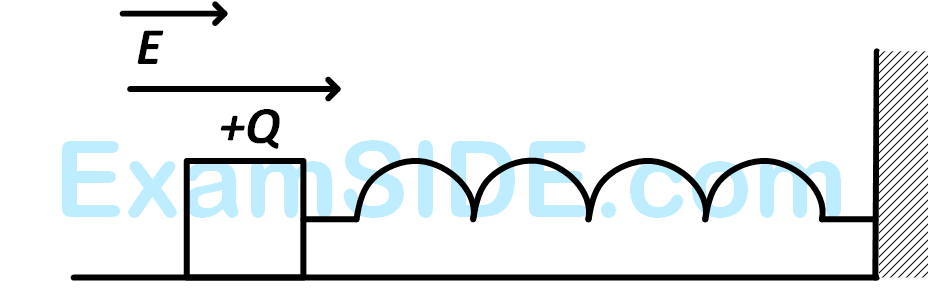
If the total energy of the particle is E, it will perform periodic motion only if

IIT-JEE 2010 Paper 1 Offline

For periodic motion of small amplitude A, the time period T of this particle is proportional to

IIT-JEE 2010 Paper 1 Offline

The acceleration of this particle for $$|x| > {X_0}$$ is

IIT-JEE 2010 Paper 1 Offline

The mass M shown in the figure below oscillates in simple harmonic motion with amplitude A. The amplitude of the point P is

IIT-JEE 2009 Paper 2 Offline

A uniform rod of length L and mass M is pivoted at the centre. Its two ends are attached to two springs of equal spring constants $$k$$. The springs are fixed to rigid supports as shown in the figure, and the rod is free to oscillate in the horizontal plane. The rod is gently pushed through a small angle $$\theta$$ in one direction and released. The frequency of oscillation is

IIT-JEE 2009 Paper 2 Offline

The $$x$$-$$t$$ graph of a particle undergoing simple harmonic motion is shown in the figure. The acceleration of the particle at $$t=4/3$$ s is

IIT-JEE 2009 Paper 1 Offline

Column I gives a list of possible set of parameters measured in some experiments. The variations of the parameters in the form of graphs are shown in Column II. Match the set of parameters given in Column I with the graphs given in Column II. Indicate your answer by darkening the appropriate bubbles of the 4 $$\times$$ 4 matrix given in the ORS.

	Column I
(A)	Potential energy of a simple pendulum (y-axis) as a function of displacement (x) axis	(P)
(B)	Displacement (y-axis) as a function of time (x-axis) for a one dimensional motion at zero or constant acceleration when the body is moving along the positive x-direction	(Q)
(C)	Range of a projectile (y-axis) as a function of its velocity (x-axis) when projected at a fixed angle	(R)
(D)	The square of the time period (y-axis) of a simple pendulum as a function of its length (x-axis)	(S)