Trigonometric Functions & Equations · Mathematics · JEE Advanced
MCQ (Single Correct Answer)
1
Let $\frac{\pi}{2} < x < \pi$ be such that $\cot x=\frac{-5}{\sqrt{11}}$. Then
$$ \left(\sin \frac{11 x}{2}\right)(\sin 6 x-\cos 6 x)+\left(\cos \frac{11 x}{2}\right)(\sin 6 x+\cos 6 x) $$
is equal to :
JEE Advanced 2024 Paper 1 Online
2
Consider the following lists :
| List-I | List-II |
|---|---|
| (I) $$\left\{x \in\left[-\frac{2 \pi}{3}, \frac{2 \pi}{3}\right]: \cos x+\sin x=1\right\}$$ | (P) has two elements |
| (II) $$\left\{x \in\left[-\frac{5 \pi}{18}, \frac{5 \pi}{18}\right]: \sqrt{3} \tan 3 x=1\right\}$$ | (Q) has three elements |
| (III) $$\left\{x \in\left[-\frac{6 \pi}{5}, \frac{6 \pi}{5}\right]: 2 \cos (2 x)=\sqrt{3}\right\}$$ | (R) has four elements |
| (IV) $$\left\{x \in\left[-\frac{7 \pi}{4}, \frac{7 \pi}{4}\right]: \sin x-\cos x=1\right\}$$ | (S) has five elements |
| (T) has six elements |
The correct option is:
JEE Advanced 2022 Paper 1 Online
3
Let f(x) = sin($$\pi $$ cos x) and g(x) = cos(2$$\pi $$ sin x) be two functions defined for x > 0. Define the following sets whose elements are written in the increasing order:
X = {x : f(x) = 0}, Y = {x : f'(x) = 0}
Z = {x : g(x) = 0}, W = {x : g'(x) = 0}
List - I contains the sets X, Y, Z and W. List - II contains some information regarding these sets.
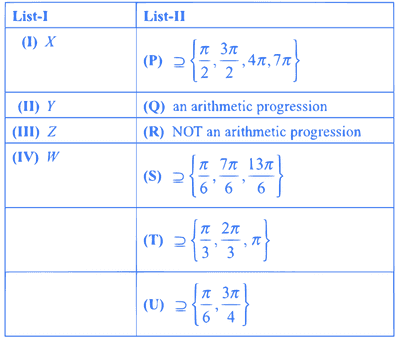
Which of the following is the only CORRECT combination?
X = {x : f(x) = 0}, Y = {x : f'(x) = 0}
Z = {x : g(x) = 0}, W = {x : g'(x) = 0}
List - I contains the sets X, Y, Z and W. List - II contains some information regarding these sets.
Which of the following is the only CORRECT combination?
JEE Advanced 2019 Paper 2 Offline
4
Let f(x) = sin($$\pi $$ cos x) and g(x) = cos(2$$\pi $$ sin x) be two functions defined for x > 0. Define the following sets whose elements are written in the increasing order :
X = {x : f(x) = 0}, Y = {x : f'(x) = 0}
Z = {x : g(x) = 0}, W = {x : g'(x) = 0}
List - I contains the sets X, Y, Z and W. List - II contains some information regarding these sets.
Which of the following combinations is correct?
X = {x : f(x) = 0}, Y = {x : f'(x) = 0}
Z = {x : g(x) = 0}, W = {x : g'(x) = 0}
List - I contains the sets X, Y, Z and W. List - II contains some information regarding these sets.
Which of the following combinations is correct?
JEE Advanced 2019 Paper 2 Offline
5
If the triangle PQR varies, then the minimum value of cos(P + Q) + cos(Q + R) + cos(R + P) is
JEE Advanced 2017 Paper 2 Offline
6
The value of
$$\sum\limits_{k = 1}^{13} {{1 \over {\sin \left( {{\pi \over 4} + {{\left( {k - 1} \right)\pi } \over 6}} \right)\sin \left( {{\pi \over 4} + {{k\pi } \over 6}} \right)}}} $$ is equal to
$$\sum\limits_{k = 1}^{13} {{1 \over {\sin \left( {{\pi \over 4} + {{\left( {k - 1} \right)\pi } \over 6}} \right)\sin \left( {{\pi \over 4} + {{k\pi } \over 6}} \right)}}} $$ is equal to
JEE Advanced 2016 Paper 2 Offline
7
Let $$S = \left\{ {x \in \left( { - \pi ,\pi } \right):x \ne 0, \pm {\pi \over 2}} \right\}.$$ The sum of all distinct solutions of the equation $$\sqrt 3 \,\sec x + \cos ec\,x + 2\left( {\tan x - \cot x} \right) = 0$$ in the set S is equal to
JEE Advanced 2016 Paper 1 Offline
8
For $$x \in \left( {0,\pi } \right),$$ the equation $$\sin x + 2\sin 2x - \sin 3x = 3$$ has
JEE Advanced 2014 Paper 2 Offline
9
The number of points in $$\left( { - \infty \,\infty } \right),$$ for which $${x^2} - x\sin x - \cos x = 0,$$ is
JEE Advanced 2013 Paper 1 Offline
10
Let $$P = \{ \theta :\sin \theta - \cos \theta = \sqrt 2 \cos \theta \} $$ and $$Q = \{ \theta :\sin \theta + \cos \theta = \sqrt 2 \sin \theta \} $$ be two sets. Then
IIT-JEE 2011 Paper 1 Offline
11
Match the statements/expressions in Column I with the values given in Column II:
| Column I | Column II | ||
|---|---|---|---|
| (A) | Root(s) of the expression $$2{\sin ^2}\theta + {\sin ^2}2\theta = 2$$ | (P) | $${\pi \over 6}$$ |
| (B) | Points of discontinuity of the function $$f(x) = \left[ {{{6x} \over \pi }} \right]\cos \left[ {{{3x} \over \pi }} \right]$$, where $$[y]$$ denotes the largest integer less than or equal to y | (Q) | $${\pi \over 4}$$ |
| (C) | Volume of the parallelopiped with its edges represented by the vectors $$\widehat i + \widehat j + \widehat i + 2\widehat j$$ and $$\widehat i + \widehat j + \pi \widehat k$$ | (R) | $${\pi \over 3}$$ |
| (D) | Angle between vectors $$\overrightarrow a $$ and $$\overrightarrow b $$ where $$\overrightarrow a $$, $$\overrightarrow b $$ and $$\overrightarrow c $$ are unit vectors satisfying $$\overrightarrow a + \overrightarrow b + \sqrt 3 \overrightarrow c = \overrightarrow 0 $$ | (S) | $${\pi \over 2}$$ |
| (T) | $$\pi $$ |
IIT-JEE 2009 Paper 2 Offline
12
Match the Statements/Expressions in Column I with the Statements/Expressions in Column II.
| Column I | Column II | ||
|---|---|---|---|
| (A) | The minimum value of $${{{x^2} + 2x + 4} \over {x + 2}}$$ is | (P) | 0 |
| (B) | Let A and B be 3 $$\times$$ 3 matrices of real numbers, where A is symmetric, B is skew-symmetric and (A + B) (A $$-$$ B) = (A $$-$$ B) (A + B). If (AB)$$^t$$ = ($$-1$$)$$^k$$ AB, where (AB)$$^t$$ is the transpose of the matrix AB, then the possible values of k are | (Q) | 1 |
| (C) | Let $$a=\log_3\log_3 2$$. An integer k satisfying $$1 < {2^{( - k + 3 - a)}} < 2$$, must be less than | (R) | 2 |
| (D) | If $$\sin \theta = \cos \varphi $$, then the possible values of $${1 \over \pi }\left( {\theta + \varphi - {\pi \over 2}} \right)$$ are | (S) | 3 |
IIT-JEE 2008 Paper 2 Offline
13
The number of solutions of the pair of equations
$$$\,2{\sin ^2}\theta - \cos 2\theta = 0$$$
$$$2co{s^2}\theta - 3\sin \theta = 0$$$
in the interval $$\left[ {0,2\pi } \right]$$
IIT-JEE 2007
14
Let $$\theta \in \left( {0,{\pi \over 4}} \right)$$ and $${t_1} = {\left( {\tan \theta } \right)^{\tan \theta }},\,\,\,\,{t_2} = \,\,{\left( {\tan \theta } \right)^{\cot \theta }}$$, $${t_3}\, = \,\,{\left( {\cot \theta } \right)^{\tan \theta }}$$ and $${t_4}\, = \,\,{\left( {\cot \theta } \right)^{\cot \theta }},$$then
IIT-JEE 2006
15
The values of $$\theta \in \left( {0,2\pi } \right)$$ for which $$2\,{\sin ^2}\theta - 5\,\sin \theta + 2 > 0,$$ are
IIT-JEE 2006 Screening
16
$$\cos \left( {\alpha - \beta } \right) = 1$$ and $$\,\cos \left( {\alpha + \beta } \right) = 1/e$$ where $$\alpha ,\,\beta \in \left[ { - \pi ,\pi } \right].$$
Paris of $$\alpha ,\,\beta $$ which satisfy both the equations is/are
Paris of $$\alpha ,\,\beta $$ which satisfy both the equations is/are
IIT-JEE 2005 Screening
17
Given both $$\theta $$ and $$\phi $$ are acute angles and $$\sin \,\theta = {1 \over 2},\,$$ $$\cos \,\phi = {1 \over 3},$$ then the value of $$\theta + \phi $$ belongs to
IIT-JEE 2004 Screening
18
The number of integral values of $$k$$ for which the equation $$7\cos x + 5\sin x = 2k + 1$$ has a solution is
IIT-JEE 2002 Screening
19
The maximum value of $$\left( {\cos {\alpha _1}} \right).\left( {\cos {\alpha _2}} \right).....\left( {\cos {\alpha _n}} \right),$$ under the restrictions
$$0 \le {\alpha _1},{\alpha _2},....,{\alpha _n} \le {\pi \over 2}$$ vand $$\left( {\cot {\alpha _1}} \right).\left( {\cot {\alpha _2}} \right)....\left( {\cot {\alpha _n}} \right) = 1$$ is
$$0 \le {\alpha _1},{\alpha _2},....,{\alpha _n} \le {\pi \over 2}$$ vand $$\left( {\cot {\alpha _1}} \right).\left( {\cot {\alpha _2}} \right)....\left( {\cot {\alpha _n}} \right) = 1$$ is
IIT-JEE 2001 Screening
20
The number of distinct real roots of $$\left| {\matrix{
{\sin x} & {\cos x} & {\cos x} \cr
{\cos x} & {\sin x} & {\cos x} \cr
{\cos x} & {\cos x} & {\sin x} \cr
} } \right|\,$$
$$\, = 0$$ in the interval $$ - {\pi \over 4} \le x \le {\pi \over 4}$$ is
$$\, = 0$$ in the interval $$ - {\pi \over 4} \le x \le {\pi \over 4}$$ is
IIT-JEE 2001 Screening
21
If $$\alpha + \beta = \pi /2$$ and $$\beta + \gamma = \alpha ,$$ then $$\tan \,\alpha \,$$ equals
IIT-JEE 2001 Screening
22
Let $$f\left( \theta \right) = \sin \theta \left( {\sin \theta + \sin 3\theta } \right)$$. Then $$f\left( \theta \right)$$ is
IIT-JEE 2000 Screening
23
In a triangle $$PQR,\angle R = \pi /2$$. If $$\,\,\tan \left( {P/2} \right)$$ and $$\tan \left( {Q/2} \right)$$ are the roots of the equation $$a{x^2} + bx + c = 0\left( {a \ne 0} \right)$$ then.
IIT-JEE 1999
24
Which of the following number(s) is /are rational?
IIT-JEE 1998
25
The number of values of $$x\,\,$$ in the interval $$\left[ {0,\,5\pi } \right]$$ satisfying the equation $$3\,{\sin ^2}x - 7\,\sin \,x + 2 = 0$$ is
IIT-JEE 1998
26
$${\sec ^2}\theta = {{4xy} \over {{{\left( {x + y} \right)}^2}}}\,$$ is true if and only if
IIT-JEE 1996
27
$$\,3{\left( {\sin x - \cos x} \right)^4} + 6{\left( {\sin x + \cos x} \right)^2} + 4\left( {{{\sin }^6}x + {{\cos }^6}x} \right) = $$
IIT-JEE 1995 Screening
28
The general values of $$\theta $$ satisfying the equation $$2{\sin ^2}\theta - 3\sin \theta - 2 = 0$$ is
IIT-JEE 1995 Screening
29
The minimum value of the expression $$\sin \,\alpha + \sin \,\beta \, + \sin \,\gamma ,\,$$ where $$\alpha ,\,\beta ,\,\gamma $$ are real numbers satisfying $$\alpha + \beta + \gamma = \pi $$ is
IIT-JEE 1995
30
If $$\omega \,$$ is an imaginary cube root of unity then the value of $$\sin \left\{ {\left( {{\omega ^{10}} + {\omega ^{23}}} \right)\pi - {\pi \over 4}} \right\}$$ is
IIT-JEE 1994
31
Let $$2{\sin ^2}x + 3\sin x - 2 > 0$$ and $${x^2} - x - 2 < 0$$ ($$x$$ is measured in radians). Then $$x$$ lies in the interval
IIT-JEE 1994
32
Let $$0 < x < {\pi \over 4}$$ then $$\left( {\sec 2x - \tan 2x} \right)$$ equals
IIT-JEE 1994
33
Let $$n$$ be a positive integer such that $$\sin {\pi \over {2n}} + \cos {\pi \over {2n}} = {{\sqrt n } \over 2}.$$ Then
IIT-JEE 1994
34
Number of solutions of the equation $$\tan x + \sec x = 2\cos x\,$$ lying in the interval $$\left[ {0,2\pi } \right]$$ is:
IIT-JEE 1993
35
In this questions there are entries in columns 1 and 2. Each entry in column 1 is related to exactly one entry in column 2. Write the correct letter from column 2 against the entry number in column 1 in your answer book.
$${{\sin \,3\alpha } \over {\cos 2\alpha }}$$ is
Column $${\rm I}$$
(A) positive
(B) negative
Column $${\rm I}$$$${\rm I}$$
(p) $$\left( {{{13\pi } \over {48}},{{14\pi } \over {48}}} \right)$$
(q) $$\left( {{{14\pi } \over {48}},\,{{18\pi } \over {48}}} \right)$$
(r) $$\left( {{{18\pi } \over {48}},\,{{23\pi } \over {48}}} \right)$$
(s) $$\left( {0,\,{\pi \over 2}} \right)$$
Options:-
IIT-JEE 1992
36
The equation $$\left( {\cos p - 1} \right){x^2} + \left( {\cos p} \right)x + \sin p = 0\,$$
In the variable x, has real roots. Then p can take any value in the interval
IIT-JEE 1990
37
The general solutions of $$\,\sin x - 3\sin 2x + \sin 3x = \cos x - 3\cos 2x + \cos 3x$$ is
IIT-JEE 1989
38
The value of the expression $$\sqrt 3 \,\cos \,ec\,{20^0} - \sec \,{20^0}$$ is equal to
IIT-JEE 1988
39
The number of all possible triplets $$\left( {{a_1},\,{a_2},\,{a_3}} \right)$$ such that $${a_1} + {a_2}\,\,\cos \left( {2x} \right) + {a_3}{\sin ^2}\left( x \right) = 0\,$$ for all $$x$$ is
IIT-JEE 1987
40
The expression $$2\left[ {{{\sin }^6}\left( {{\pi \over 2} + \alpha } \right) + {{\sin }^6}\left( {5\pi - \alpha } \right)} \right]$$ is equal to
IIT-JEE 1986
41
$$\left( {1 + \cos {\pi \over 8}} \right)\left( {1 + \cos {{3\pi } \over 8}} \right)\left( {1 + \cos {{5\pi } \over 8}} \right)\left( {1 + \cos {{7\pi } \over 8}} \right)$$ is equal to
IIT-JEE 1984
42
The general solution of the trigonometric equation sin x+cos x=1 is given by:
IIT-JEE 1981
43
The equation $$\,2{\cos ^2}{x \over 2}{\sin ^2}x = {x^2} + {x^{ - 2}};\,0 < x \le {\pi \over 2}$$ has
IIT-JEE 1980
44
Given $$A = {\sin ^2}\theta + {\cos ^4}\theta $$ then for all real values of $$\theta $$
IIT-JEE 1980
45
If $$\alpha + \beta + \gamma = 2\pi ,$$ then
IIT-JEE 1979
46
If $$\tan \theta = - {4 \over 3},then\sin \theta \,is\,$$
IIT-JEE 1979
Numerical
1
$$
\text { Then the inradius of the triangle } A B C \text { is }
$$ :
JEE Advanced 2023 Paper 2 Online
2
Let $\alpha$ and $\beta$ be real numbers such that $-\frac{\pi}{4}<\beta<0<\alpha<\frac{\pi}{4}$.
If $\sin (\alpha+\beta)=\frac{1}{3}$ and $\cos (\alpha-\beta)=\frac{2}{3}$, then the greatest integer less than or equal to
$$ \left(\frac{\sin \alpha}{\cos \beta}+\frac{\cos \beta}{\sin \alpha}+\frac{\cos \alpha}{\sin \beta}+\frac{\sin \beta}{\cos \alpha}\right)^{2} $$ is
If $\sin (\alpha+\beta)=\frac{1}{3}$ and $\cos (\alpha-\beta)=\frac{2}{3}$, then the greatest integer less than or equal to
$$ \left(\frac{\sin \alpha}{\cos \beta}+\frac{\cos \beta}{\sin \alpha}+\frac{\cos \alpha}{\sin \beta}+\frac{\sin \beta}{\cos \alpha}\right)^{2} $$ is
JEE Advanced 2022 Paper 2 Online
3
Let f : R $$ \to $$ R be a differentiable function with f(0) = 1 and satisfying the equation f(x + y) = f(x) f'(y) + f'(x) f(y) for all x, y$$ \in $$ R.
Then, the value of loge(f(4)) is ...........
Then, the value of loge(f(4)) is ...........
JEE Advanced 2018 Paper 2 Offline
4
The number of distinct solutions of the equation
$${5 \over 4}{\cos ^2}\,2x + {\cos ^4}\,x + {\sin ^4}\,x + {\cos ^6}\,x + {\sin ^6}\,x\, = \,2$$
in the interval $$\left[ {0,\,2\pi } \right]$$ is
$${5 \over 4}{\cos ^2}\,2x + {\cos ^4}\,x + {\sin ^4}\,x + {\cos ^6}\,x + {\sin ^6}\,x\, = \,2$$
in the interval $$\left[ {0,\,2\pi } \right]$$ is
JEE Advanced 2015 Paper 1 Offline
5
The positive integer value of $$n\, > \,3$$ satisfying the equation $${1 \over {\sin \left( {{\pi \over n}} \right)}} = {1 \over {\sin \left( {{{2\pi } \over n}} \right)}} + {1 \over {\sin \left( {{{3\pi } \over n}} \right)}}$$ is
IIT-JEE 2011 Paper 1 Offline
6
The number of values of $$\theta $$ in the interval, $$\left( { - {\pi \over 2},\,{\pi \over 2}} \right)$$ such
that$$\,\theta \ne {{n\pi } \over 5}$$ for $$n = 0,\, \pm 1,\, \pm 2$$ and $$\tan \,\theta = \cot \,5\theta \,$$ as well as $$\sin \,2\theta = \cos \,4 \theta $$ is
IIT-JEE 2010 Paper 1 Offline
7
The number of all possible values of $$\theta $$ where $$0 < \theta < \pi ,$$ for which the system of equations
$$$\left( {y + z} \right)\cos {\mkern 1mu} 3\theta = \left( {xyz} \right){\mkern 1mu} \sin 3\theta $$$
$$$x\sin 3\theta = {{2\cos 3\theta } \over y} + {{2\sin 3\theta } \over z}$$$
$$$\left( {xyz} \right){\mkern 1mu} \sin 3\theta = \left( {y + 2z} \right){\mkern 1mu} \cos 3\theta + y{\mkern 1mu} sin3\theta $$$
have a solution $$\left( {{x_0},{y_0},{z_0}} \right)$$ with $${y_0}{z_0}{\mkern 1mu} \ne {\mkern 1mu} 0,$$ is
IIT-JEE 2010 Paper 1 Offline
8
The maximum value of the expression $${1 \over {{{\sin }^2}\theta + 3\sin \theta \cos \theta + 5{{\cos }^2}\theta }}$$ is
IIT-JEE 2010 Paper 1 Offline
9
Two parallel chords of a circle of radius 2 are at a distance $$\sqrt 3 + 1$$ apart. If the chords subtend at the center , angles of $${\pi \over k}$$ and $${{2\pi } \over k},$$ where$$k > 0,$$ then the value of $$\left[ k \right]$$ is
[Note :[k] denotes the largest integer less than or equal to k ]
IIT-JEE 2010 Paper 2 Offline
MCQ (More than One Correct Answer)
1
Let $P Q R S$ be a quadrilateral in a plane, where
$Q R=1, \angle P Q R=\angle Q R S=70^{\circ}, \angle P Q S=15^{\circ}$ and $\angle P R S=40^{\circ}$.
If $\angle R P S=\theta^{\circ}, P Q=\alpha$ and $P S=\beta$, then the interval(s) that contain(s) the value of
$4 \alpha \beta \sin \theta^{\circ}$ is/are
$Q R=1, \angle P Q R=\angle Q R S=70^{\circ}, \angle P Q S=15^{\circ}$ and $\angle P R S=40^{\circ}$.
If $\angle R P S=\theta^{\circ}, P Q=\alpha$ and $P S=\beta$, then the interval(s) that contain(s) the value of
$4 \alpha \beta \sin \theta^{\circ}$ is/are
JEE Advanced 2022 Paper 2 Online
2
For non-negative integers n, let
$$f(n) = {{\sum\limits_{k = 0}^n {\sin \left( {{{k + 1} \over {n + 2}}\pi } \right)} \sin \left( {{{k + 2} \over {n + 2}}\pi } \right)} \over {\sum\limits_{k = 0}^n {{{\sin }^2}\left( {{{k + 1} \over {n + 2}}\pi } \right)} }}$$
Assuming cos$$-1$$ x takes values in [0, $$\pi $$], which of the following options is/are correct?
$$f(n) = {{\sum\limits_{k = 0}^n {\sin \left( {{{k + 1} \over {n + 2}}\pi } \right)} \sin \left( {{{k + 2} \over {n + 2}}\pi } \right)} \over {\sum\limits_{k = 0}^n {{{\sin }^2}\left( {{{k + 1} \over {n + 2}}\pi } \right)} }}$$
Assuming cos$$-1$$ x takes values in [0, $$\pi $$], which of the following options is/are correct?
JEE Advanced 2019 Paper 2 Offline
3
In a $$\Delta $$PQR = 30$$^\circ $$ and the sides PQ and QR have lengths 10$$\sqrt 3 $$ and 10, respectively. Then, which of the following statement(s) is(are) TRUE?
JEE Advanced 2018 Paper 1 Offline
4
Let $$\alpha $$ and $$\beta $$ be non zero real numbers such that $$2(\cos \beta - \cos \alpha ) + \cos \alpha \cos \beta = 1$$. Then which of the following is/are true?
JEE Advanced 2017 Paper 2 Offline
5
Let $$f\left( x \right) = x\sin \,\pi x,\,x > 0.$$ Then for all natural numbers $$n,\,f'\left( x \right)$$ vanishes at
JEE Advanced 2013 Paper 1 Offline
6
Let $$\theta ,\,\varphi \, \in \,\left[ {0,2\pi } \right]$$ be such that
$$2\cos \theta \left( {1 - \sin \,\varphi } \right) = {\sin ^2}\theta \,\,\left( {\tan {\theta \over 2} + \cot {\theta \over 2}} \right)\cos \varphi - 1,\,\tan \left( {2\pi - \theta } \right) > 0$$ and $$ - 1 < \sin \theta \, < - {{\sqrt 3 } \over 2},$$
$$2\cos \theta \left( {1 - \sin \,\varphi } \right) = {\sin ^2}\theta \,\,\left( {\tan {\theta \over 2} + \cot {\theta \over 2}} \right)\cos \varphi - 1,\,\tan \left( {2\pi - \theta } \right) > 0$$ and $$ - 1 < \sin \theta \, < - {{\sqrt 3 } \over 2},$$
then $$\varphi $$ cannot satisfy
IIT-JEE 2012 Paper 1 Offline
7
For $$0 < \theta < {\pi \over 2},$$ the solution (s) of
$$$\sum\limits_{m = 1}^6 {\cos ec\,\left( {\theta + {{\left( {m - 1} \right)\pi } \over 4}} \right)\,\cos ec\,\left( {\theta + {{m\pi } \over 4}} \right) = 4\sqrt 2 } $$$ is (are)
IIT-JEE 2009 Paper 2 Offline
8
If $${{{{\sin }^4}x} \over 2} + {{{{\cos }^4}x} \over 3} = {1 \over 5},$$ then
IIT-JEE 2009 Paper 1 Offline
9
For a positive integer $$\,n$$, let
$${f_n}\left( \theta \right) = \left( {\tan {\theta \over 2}} \right)\,\left( {1 + \sec \theta } \right)\,\left( {1 + \sec 2\theta } \right)\,\left( {1 + \sec 4\theta } \right).....\left( {1 + \sec {2^n}\theta } \right).$$ Then
$${f_n}\left( \theta \right) = \left( {\tan {\theta \over 2}} \right)\,\left( {1 + \sec \theta } \right)\,\left( {1 + \sec 2\theta } \right)\,\left( {1 + \sec 4\theta } \right).....\left( {1 + \sec {2^n}\theta } \right).$$ Then
IIT-JEE 1999
10
The values of $$\theta $$ lying between $$\theta = \theta $$ and $$\theta = \pi /2$$ and satisfying the equation
$$\left| {\matrix{ {1 + {{\sin }^2}\theta } & {{{\cos }^2}\theta } & {4\sin 4\theta } \cr {{{\sin }^2}\theta } & {1 + {{\cos }^2}\theta } & {4\sin 4\theta } \cr {{{\sin }^2}\theta } & {{{\cos }^2}\theta } & {1 + 4\sin 4\theta } \cr } } \right| = 0$$ are
IIT-JEE 1988
Subjective
1
Find the range of values of $$\,t$$ for which $$$2\,\sin \,t = {{1 - 2x + 5{x^2}} \over {3{x^2} - 2x - 1}},\,\,\,\,\,t\, \in \,\left[ { - {\pi \over 2},\,{\pi \over 2}} \right].$$$
IIT-JEE 2005
2
In any triangle $$ABC,$$ prove that
$$$\cot {A \over 2} + \cot {B \over 2} + \cot {C \over 2} = \cot {A \over 2}\cot {B \over 2}\cot {C \over 2}.$$$
IIT-JEE 2000
3
Prove that $$\tan \,\alpha + 2\tan 2\alpha + 4\tan 4\alpha + 8\cot 8\alpha = \cot \alpha $$
IIT-JEE 1998
4
Prove that the values of the function $${{\sin x\cos 3x} \over {\sin 3x\cos x}}$$ do not lie between $${1 \over 3}$$ and 3 for any real $$x.$$
IIT-JEE 1997
5
Prove that $$\sum\limits_{k = 1}^{n - 1} {\left( {n - k} \right)\,\cos \,{{2k\pi } \over n} = - {n \over 2},} $$ where $$n \ge 3$$ is an integer.
IIT-JEE 1997
6
Find all values of $$\theta $$ in the interval $$\left( { - {\pi \over 2},{\pi \over 2}} \right)$$ satisfying the equation $$\left( {1 - \tan \,\theta } \right)\left( {1 + \tan \,\theta } \right)\,\,{\sec ^2}\theta + \,\,{2^{{{\tan }^2}\theta }} = 0.$$
IIT-JEE 1996
7
Find the smallest positive number $$p$$ for which the equation $$\cos \left( {p\,\sin x} \right) = \sin \left( {p\cos x} \right)$$ has a solution $$x\, \in \,\left[ {0,2\pi } \right]$$.
IIT-JEE 1995
8
Determine the smallest positive value of number $$x$$ (in degrees) for which
$$$\tan \left( {x + {{100}^ \circ }} \right) = \tan \left( {x + {{50}^ \circ }} \right)\,\tan \left( x \right)\tan \left( {x - {{50}^ \circ }} \right).$$$
IIT-JEE 1993
9
Show that the value of $${{\tan x} \over {\tan 3x}},$$ wherever defined never lies between $${1 \over 3}$$ and 3.
IIT-JEE 1992
10
If $$\exp \,\,\,\left\{ {\left( {\left( {{{\sin }^2}x + {{\sin }^4}x + {{\sin }^6}x + \,\,\,..............\infty } \right)\,In\,\,2} \right)} \right\}$$ satiesfies the equation $${x^2} - 9x + 8 = 0,$$ find the value of $${{\cos x} \over {\cos x + \sin x}},\,0 < x < {\pi \over 2}.$$
IIT-JEE 1991
11
$$ABC$$ is a triangle such that
$$$\sin \left( {2A + B} \right) = \sin \left( {C - A} \right) = \, - \sin \left( {B + 2C} \right) = {1 \over 2}.$$$
If $$A,\,B$$ and $$C$$ are in arithmetic progression, determine the values of $$A,\,B$$ and $$C$$.
IIT-JEE 1990
12
Find the values of $$x \in \left( { - \pi , + \pi } \right)$$ which satisfy the equation $${g^{(1 + \left| {\cos x} \right| + \left| {{{\cos }^2}x} \right| + \left| {{{\cos }^3}x} \right| + ...)}} = {4^3}$$
IIT-JEE 1984
13
Find all solutions of $$4{\cos ^2}\,x\sin x - 2{\sin ^2}x = 3\sin x$$
IIT-JEE 1983
14
Show that $$$16\cos \left( {{{2\pi } \over {15}}} \right)\cos \left( {{{4\pi } \over {15}}} \right)\cos \left( {{{8\pi } \over {15}}} \right)\cos \left( {{{16\pi } \over {15}}} \right) = 1$$$
IIT-JEE 1983
15
Without using tables, prove that $$\left( {\sin \,{{12}^ \circ }} \right)\left( {\sin \,{{48}^ \circ }} \right)\left( {\sin \,{{54}^ \circ }} \right) = {1 \over 8}.$$
IIT-JEE 1982
16
Given $$A = \left\{ {x:{\pi \over 6} \le x \le {\pi \over 3}} \right\}$$ and
$$f\left( x \right) = \cos x - x\left( {1 + x} \right);$$ find $$f\left( A \right).$$
$$f\left( x \right) = \cos x - x\left( {1 + x} \right);$$ find $$f\left( A \right).$$
IIT-JEE 1980
17
Given $$\alpha + \beta - \gamma = \pi ,$$ prove that
$$\,{\sin ^2}\alpha + {\sin ^2}\beta - {\sin ^2}\gamma = 2\sin \alpha {\mkern 1mu} \sin \beta {\mkern 1mu} \cos y$$
$$\,{\sin ^2}\alpha + {\sin ^2}\beta - {\sin ^2}\gamma = 2\sin \alpha {\mkern 1mu} \sin \beta {\mkern 1mu} \cos y$$
IIT-JEE 1980
18
For all $$\theta $$ in $$\left[ {0,\,\pi /2} \right]$$ show that, $$\cos \left( {\sin \theta } \right) \ge \,\sin \,\left( {\cos \theta } \right).$$
IIT-JEE 1980
19
(a) Draw the graph of $$y = {1 \over {\sqrt 2 }}\left( {cinx + \cos x} \right)$$ from $$x = - {\pi \over 2}$$ to $$x = {\pi \over 2}$$.
(b) If $$\cos \left( {\alpha + \beta } \right) = {4 \over 5},\,\,\sin \,\left( {\alpha - \beta } \right) = \,{5 \over {13}},$$ and $$\alpha ,\,\beta $$ lies between 0 and $${\pi \over 4}$$, find tan2$$\alpha $$.
IIT-JEE 1979
20
If $$\tan \alpha = {m \over {m + 1}}\,$$ and $$\tan \beta = {2 \over {2m + 1}},$$ find the possible values of $$\left( {\alpha + \beta } \right).$$
IIT-JEE 1978
Fill in the Blanks
1
The real roots of the equation $$\,{\cos ^7}x + {\sin ^4}x = 1$$ in the interval $$\left( { - \pi ,\pi } \right)$$ are ...., ...., and ______.
IIT-JEE 1997
2
General value of $$\theta $$ satisfying the equation $${\tan ^2}\theta + \sec \,2\,\theta = 1$$ is _________.
IIT-JEE 1996
3
If $$A > 0,B > 0\,$$ and $$A + B = \pi /3,$$ then the maximum value of tan A tan B is _______.
IIT-JEE 1993
4
If $$K = \sin \left( {\pi /18} \right)\sin \left( {5\pi /18} \right)\sin \left( {7\pi /18} \right),$$ then the numerical value of K is ______.
IIT-JEE 1993
5
The value of
$$\sin {\pi \over {14}}\sin {{3\pi } \over {14}}\sin {{5\pi } \over {14}}\sin {{7\pi } \over {14}}\sin {{9\pi } \over {14}}\sin {{11\pi } \over {14}}\sin {{13\pi } \over {14}}$$ is equal to ______.
$$\sin {\pi \over {14}}\sin {{3\pi } \over {14}}\sin {{5\pi } \over {14}}\sin {{7\pi } \over {14}}\sin {{9\pi } \over {14}}\sin {{11\pi } \over {14}}\sin {{13\pi } \over {14}}$$ is equal to ______.
IIT-JEE 1991
6
The solution set of the system of equations $$X + Y = {{2\pi } \over 3},$$ $$cox\,x + cos\,y = {3 \over 2},$$ where x and y are real, is _____.
IIT-JEE 1987
7
The set of all $$x$$ in the interval $$\left[ {0,\,\pi } \right]$$ for which $$2\,{\sin ^2}x - 3$$ $$\sin x + 1 \ge 0,$$ is _____.
IIT-JEE 1987
8
The sides of a triangle inscribed in a given circle subtend angles $$\alpha $$, $$\beta $$ and $$\gamma $$ at the centre. The minimum value of the arithmetic mean of $$cos\left[ {\alpha + {\pi \over 2}} \right],\,\cos \left[ {\beta + {\pi \over 2}} \right]$$ and $$cos\left[ {\gamma + {\pi \over 2}} \right]$$ is equal to _______.
IIT-JEE 1987
9
Suppose $${\sin ^3}\,x\sin 3x = \sum\limits_{m = 0}^n {{C_m}\cos \,mx} $$ is an identity in x, where C0, C1 ,....Cn are constants, and $${C_n} \ne 0$$ , then the value of n is _____.
IIT-JEE 1981