If the measurement errors in all the independent quantities are known, then it is possible to determine the error in any dependent quantity. This is done by the use of series expansion and truncating the expansion at the first power of the error. For example, consider the relation $$z = x/y.$$ If the errors in $$x,y$$ and $$z$$ are $$\Delta x,\Delta y$$ and $$\Delta z,$$ respectively, then
$$$z \pm \Delta z = {{x \pm \Delta x} \over {y \pm \Delta y}} = {x \over y}\left( {1 \pm {{\Delta x} \over x}} \right){\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}}.$$$

The series expansion for $${\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}},$$ to first power in $$\Delta y/y.$$ is $$1 \pm \left( {\Delta y/y} \right).$$ The relative errors in independent variables are always added. So the error in $$z$$ will be
$$$\Delta z = z\left( {{{\Delta x} \over x} + {{\Delta y} \over y}} \right).$$$

The above derivation makes the assumption that $$\Delta x/x < < 1,$$ $$\Delta y/y < < 1.$$ Therefore, the higher powers of these quantities are neglected.

Consider the ratio $$r = {{\left( {1 - a} \right)} \over {1 + a}}$$ to be determined by measuring a dimensionless quantity $$a.$$ If the error in the measurement of $$a$$ is $$\Delta a\left( {\Delta a/a < < 1.} \right.$$ then what is the error $$\Delta r$$ in determining $$r$$?

A person measures the depth of a well by measuring the time interval between dropping a stone and receiving the sound of impact with the bottom of the well. The error in his measurement of time is $$\delta T = 0.01$$ seconds and he measures the depth of the well to be $$L=20$$ meters. Take the acceleration due to gravity $$g = 10m{s^{ - 2}}$$ and the velocity of sound is $$300$$ $$m{s^{ - 1}}$$. Then the fractional error in the measurement, $$\delta L/L,$$ is closest to

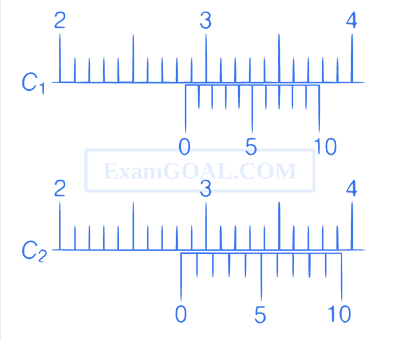
There are two Vernier calipers both of which have 1 cm divided into 10 equal divisions on the main scale. The Vernier scale of one of the calipers (C₁) has 10 equal divisions that correspond to 9 main scale divisions. The Vernier scale of the other caliper (C₂) has 10 equal divisions that correspond to 11 main scale divisions. The readings of the two calipers are shown in the figure. The measured values (in cm) by calipers C₁ and C₂ respectively, are

Match List I with List II and select the correct answer using the codes given below the lists:

List I

P. Boltzmann Constant
Q. Coefficient of viscosity
R. Plank Constant
S. Thermal conductivity

List II

1. [ML²T^-1]
2. [ML^-1T^-1]
3. [MLT^-3K^-1]
4. [ML²T^-2K^-1]