JEE Advanced 2018 Paper 2 Offline
Paper was held on
Sun, May 20, 2018 2:00 AM
Chemistry
1
Galena (an ore) is partially oxidized by passing air through it at high temperature. After some time, the passage of air is stopped, but the heating is continued in a closed furnace such that the contents undergo self-reduction. The weight (in kg) of $$Pb$$ produced per kg of $${O_2}$$ consumed is ___________.
(Atomic weights in $$g\,mo{l^{ - 1}}:O = 16,S = 32,Pb = 207$$)
(Atomic weights in $$g\,mo{l^{ - 1}}:O = 16,S = 32,Pb = 207$$)
2
The Fischer presentation of $$D$$-glucose is given below.

The correct structure(s) of $$\beta $$-$$L$$-glucopyranose is (are) :

The correct structure(s) of $$\beta $$-$$L$$-glucopyranose is (are) :
3
The correct option(s) to distinguish nitrate salts of $$M{n^{2 + }}$$ and $$C{u^{2 + }}$$ taken separately is (are)
4
The total number of compounds having at least one bridging oxo group among the molecules given below is _________.
$${N_2}{O_3},{N_2}{O_5},$$ $${P_4}{O_6},{P_4}{O_7},$$ $${H_4}{P_2}{O_5},{H_5}{P_3}{O_{10}},$$ $${H_2}{S_2}{O_3},{H_2}{S_2}{O_5}$$
$${N_2}{O_3},{N_2}{O_5},$$ $${P_4}{O_6},{P_4}{O_7},$$ $${H_4}{P_2}{O_5},{H_5}{P_3}{O_{10}},$$ $${H_2}{S_2}{O_3},{H_2}{S_2}{O_5}$$
5
The correct option(s) regarding the complex
$${\left[ {Co\left( {en} \right){{\left( {N{H_3}} \right)}_3}\left( {{H_2}O} \right)} \right]^{3 + }}\,\,$$ $$\left( {en = {H_2}NC{H_2}C{H_2}N{H_2}} \right)$$ is (are)
$${\left[ {Co\left( {en} \right){{\left( {N{H_3}} \right)}_3}\left( {{H_2}O} \right)} \right]^{3 + }}\,\,$$ $$\left( {en = {H_2}NC{H_2}C{H_2}N{H_2}} \right)$$ is (are)
6
To measure the quantity of $$MnC{l_2}$$ dissolved in an aqueous solution, it was completely converted to $$KMn{O_4}$$ using the reaction,
$$MnC{l_2} + {K_2}{S_2}{O_8} + {H_2}O \to KMn{O_4} + {H_2}S{O_4} + HCl$$ (equation not balanced).
Few drops of concentrated $$HCl$$ were added to this solution and gently warmed. Further, oxalic acid ($$225$$ $$mg$$) was added in portions till the colour of the permanganate ion disappeared. The quantity of $$MnC{l_2}$$ (in mg) present in the initial solution is ____________.
(Atomic weights in $$g\,\,mo{l^{ - 1}}:Mn = 55,Cl = 35.5$$ )
$$MnC{l_2} + {K_2}{S_2}{O_8} + {H_2}O \to KMn{O_4} + {H_2}S{O_4} + HCl$$ (equation not balanced).
Few drops of concentrated $$HCl$$ were added to this solution and gently warmed. Further, oxalic acid ($$225$$ $$mg$$) was added in portions till the colour of the permanganate ion disappeared. The quantity of $$MnC{l_2}$$ (in mg) present in the initial solution is ____________.
(Atomic weights in $$g\,\,mo{l^{ - 1}}:Mn = 55,Cl = 35.5$$ )
7
Aniline reacts with mixed acid (conc. $$HN{O_3}$$ and conc. $${H_2}S{O_4}$$) at $$288$$ $$K$$ to give P $$\left( {51\% } \right),$$ Q $$\left( {47\% } \right)$$ and R $$\left( {2\% } \right).$$ The major product(s) of the following reaction sequence is (are)


8
The surface of copper gets tarnished by the formation of copper oxide. $${N_2}$$ gas was passed to prevent the oxide formation during heating of copper at $$1250$$ $$K.$$ However, the $${N_2}$$ gas contains $$1$$ mole % of water vapor as impurity. The water vapor oxidises copper as per the reaction given below : $$2Cu\left( s \right) + {H_2}O\left( g \right) \to C{u_2}O\left( s \right) + {H_2}\left( g \right)$$
$${P_{H2}}$$ is the minimum partial pressure of $${H_2}$$ (in bar) needed to prevent the oxidation at $$1250$$ $$K.$$ The value of $$\ln \left( {{P_{H2}}} \right)$$ is ________.
Given: total pressure $$=1$$ bar, $$R$$ (universal gas constant ) $$=$$ $$8J{K^{ - 1}}\,\,mo{l^{ - 1}},$$ $$\ln \left( {10} \right) = 2.3.\,$$ $$Cu(s)$$ and $$C{u_2}O\left( s \right)$$ are naturally immiscible.
At $$1250$$ $$K:2Cu(s)$$ $$ + {\raise0.5ex\hbox{$\scriptstyle 1$} \kern-0.1em/\kern-0.15em \lower0.25ex\hbox{$\scriptstyle 2$}}{O_2}\left( g \right) \to C{u_2}O\left( s \right);$$ $$\Delta {G^ \circ } = - 78,000J\,mo{l^{ - 1}}$$
$${H_2}\left( g \right) + {\raise0.5ex\hbox{$\scriptstyle 1$} \kern-0.1em/\kern-0.15em \lower0.25ex\hbox{$\scriptstyle 2$}}{O_2}\left( g \right) \to {H_2}O\left( g \right);$$
$$\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,$$ $$\Delta {G^ \circ } = - 1,78,000J\,mo{l^{ - 1}};$$ ($$G$$ is the Gibbs energy)
$${P_{H2}}$$ is the minimum partial pressure of $${H_2}$$ (in bar) needed to prevent the oxidation at $$1250$$ $$K.$$ The value of $$\ln \left( {{P_{H2}}} \right)$$ is ________.
Given: total pressure $$=1$$ bar, $$R$$ (universal gas constant ) $$=$$ $$8J{K^{ - 1}}\,\,mo{l^{ - 1}},$$ $$\ln \left( {10} \right) = 2.3.\,$$ $$Cu(s)$$ and $$C{u_2}O\left( s \right)$$ are naturally immiscible.
At $$1250$$ $$K:2Cu(s)$$ $$ + {\raise0.5ex\hbox{$\scriptstyle 1$} \kern-0.1em/\kern-0.15em \lower0.25ex\hbox{$\scriptstyle 2$}}{O_2}\left( g \right) \to C{u_2}O\left( s \right);$$ $$\Delta {G^ \circ } = - 78,000J\,mo{l^{ - 1}}$$
$${H_2}\left( g \right) + {\raise0.5ex\hbox{$\scriptstyle 1$} \kern-0.1em/\kern-0.15em \lower0.25ex\hbox{$\scriptstyle 2$}}{O_2}\left( g \right) \to {H_2}O\left( g \right);$$
$$\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,$$ $$\Delta {G^ \circ } = - 1,78,000J\,mo{l^{ - 1}};$$ ($$G$$ is the Gibbs energy)
9
For the given compound $$X,$$ the total number of optically active stereoisomers is ____________.
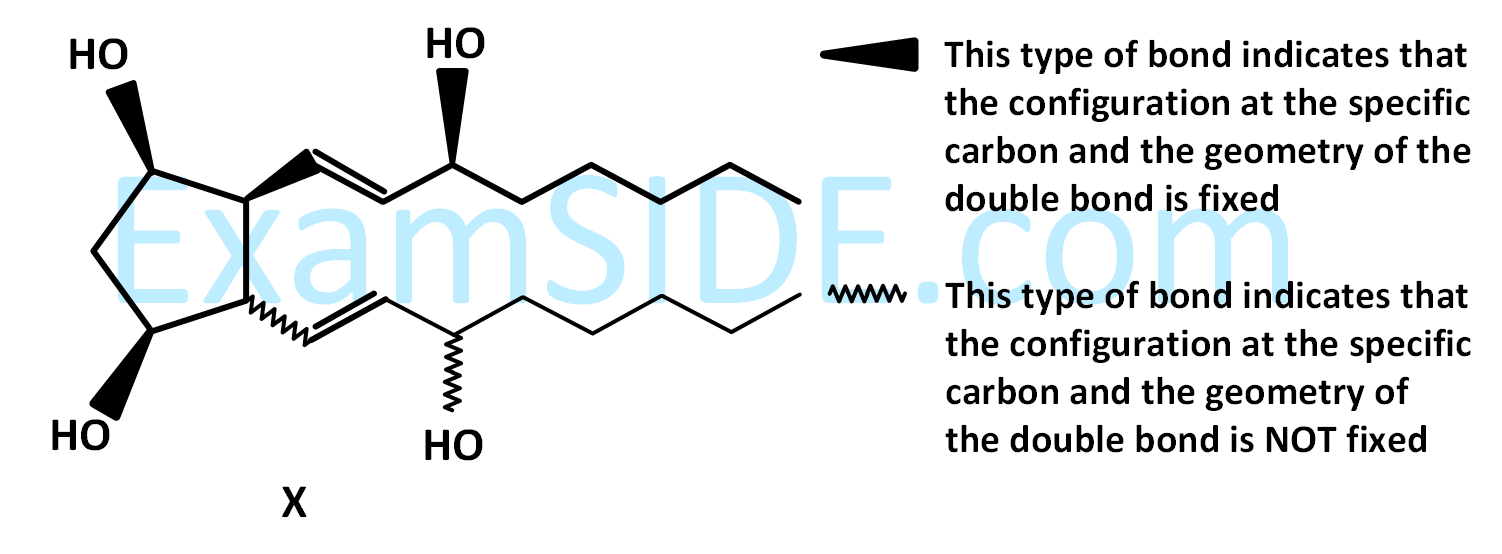



10
In the following reaction sequence, the amount of $$D$$ (in g) formed from $$10$$ moles of acetophenone is ___________.
(Atomic weights in $$g\,mo{l^{ - 1}}:H = 1,C = 12,$$ $$N = 14,O = 16,$$ $$Br = 80.$$. The yield (%) corresponding to the product in each step is given in the parenthesis)
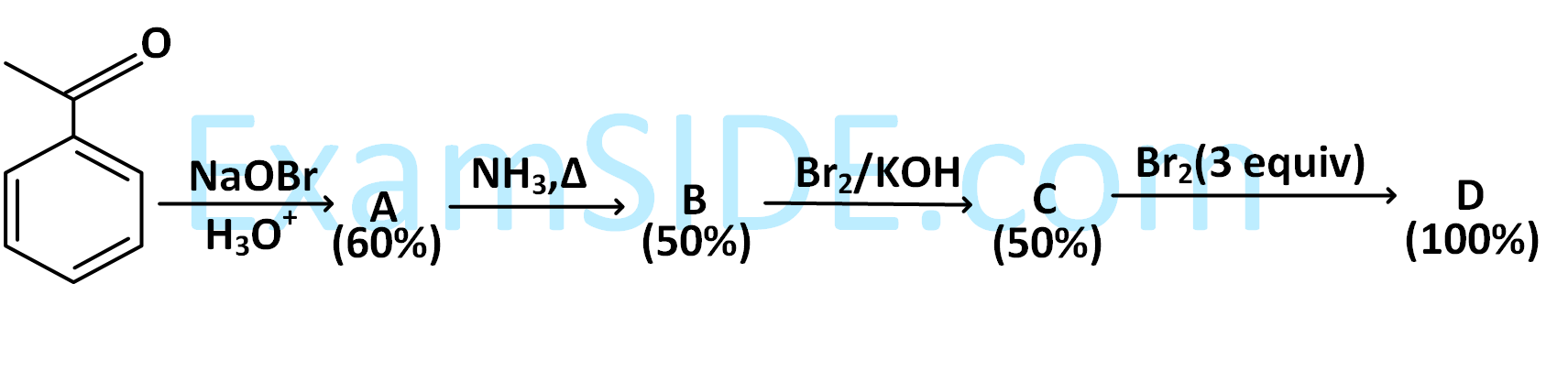
(Atomic weights in $$g\,mo{l^{ - 1}}:H = 1,C = 12,$$ $$N = 14,O = 16,$$ $$Br = 80.$$. The yield (%) corresponding to the product in each step is given in the parenthesis)

11
For a first order reaction $$A\left( g \right) \to 2B\left( g \right) + C\left( g \right)$$ at constant volume and $$300K,$$ the total pressure at the beginning $$(t=0)$$ and at time $$t$$ are $${P_0}$$ and $${P_1},$$ respectively. Initially, only $$A$$ is present with concentration $${\left[ A \right]_0},$$ and $${t_{1/3}}$$ is the time required for the partial pressure of $$A$$ to reach $$1/{3^{rd}}$$ of its initial value. The correct option(s) is (are) (Assume that all these gases behave as ideal gases)
12
Consider an electrochemical cell :
$$A\left( s \right)\left| {{A^{n + }}\left( {aq,2M} \right)} \right|{B^{2n + }}\left( {aq,1M} \right)\left| {B\left( s \right).} \right.$$
The value of $$\Delta {H^ \circ }$$ for the cell reaction is twice that of $$\Delta {G^ \circ }$$ at $$300$$ $$K.$$ If the $$emf$$ of the cell is zero, the $$\Delta {S^ \circ }$$ (in $$J\,{K^{ - 1}}mo{l^{ - 1}}$$) of the cell reaction per mole of $$B$$ formed at $$300$$ $$K$$ is ___________.
(Given: $$\ln \left( 2 \right) = 0.7,R$$ (universal gas constant) $$ = 8.3J\,{K^{ - 1}}\,mo{l^{ - 1}}.$$ $$H,S$$ and $$G$$ are enthalpy, entropy and Gibbs energy, respectively.)
$$A\left( s \right)\left| {{A^{n + }}\left( {aq,2M} \right)} \right|{B^{2n + }}\left( {aq,1M} \right)\left| {B\left( s \right).} \right.$$
The value of $$\Delta {H^ \circ }$$ for the cell reaction is twice that of $$\Delta {G^ \circ }$$ at $$300$$ $$K.$$ If the $$emf$$ of the cell is zero, the $$\Delta {S^ \circ }$$ (in $$J\,{K^{ - 1}}mo{l^{ - 1}}$$) of the cell reaction per mole of $$B$$ formed at $$300$$ $$K$$ is ___________.
(Given: $$\ln \left( 2 \right) = 0.7,R$$ (universal gas constant) $$ = 8.3J\,{K^{ - 1}}\,mo{l^{ - 1}}.$$ $$H,S$$ and $$G$$ are enthalpy, entropy and Gibbs energy, respectively.)
13
Consider the following reversible reaction, $$A\left( g \right) + B\left( g \right) \to AB\left( g \right).$$
The activation energy of the backward reaction exceeds that of the forward reaction by $$2RT$$ (in $$J\,mo{l^{ - 1}}$$). If the pre-exponential factor of the forward reaction is $$4$$ times that of the reverse reaction, the absolute value of $$\Delta {G^ \circ }$$ (in $$J\,mo{l^{ - 1}}$$ ) for the reaction at $$300$$ $$K$$ is ____________.
(Given; $$\ln \left( 2 \right) = 0.7,RT = 2500$$ $$J\,mo{l^{ - 1}}$$ at $$300$$ $$K$$ and $$G$$ is the Gibbs energy)
The activation energy of the backward reaction exceeds that of the forward reaction by $$2RT$$ (in $$J\,mo{l^{ - 1}}$$). If the pre-exponential factor of the forward reaction is $$4$$ times that of the reverse reaction, the absolute value of $$\Delta {G^ \circ }$$ (in $$J\,mo{l^{ - 1}}$$ ) for the reaction at $$300$$ $$K$$ is ____________.
(Given; $$\ln \left( 2 \right) = 0.7,RT = 2500$$ $$J\,mo{l^{ - 1}}$$ at $$300$$ $$K$$ and $$G$$ is the Gibbs energy)
14
Match each set of hybrid orbitals from LIST - A with complex(es) given in LIST - B
The correct option is
| List - A | List - B | |||
|---|---|---|---|---|
| P. | dsp2 | 1. | [FeF6]4- | |
| Q. | sp3 | 2. | [Ti(H2O)3Cl3] | |
| R. | sp3d2 | 3. | [Cr(NH3)6]3+ | |
| S. | d2sp3 | 4. | [FeCl4]2- | |
| 5. | Ni(CO)4 | |||
| 6. | [Ni(CN)4]2- |
The correct option is
15
Dilution processes of different aqueous solutions, with water, are given in LIST - I. The effects of dilution of the solutions on $$\left[ {{H^ + }} \right]$$ are given in LIST - II
(Note: Degree of dissociation (a) of weak acid and weak base is $$<<1;$$ degree of hydrolysis of salt $$<<1;$$ $$\left[ {{H^ + }} \right]$$ represents the concentration of $${H^ + }$$ ions)
Match each process given in LIST-I with one or more effect(s) in LIST-II. The correct option is :
(Note: Degree of dissociation (a) of weak acid and weak base is $$<<1;$$ degree of hydrolysis of salt $$<<1;$$ $$\left[ {{H^ + }} \right]$$ represents the concentration of $${H^ + }$$ ions)
| LIST-I | LIST-II | ||
|---|---|---|---|
| P. | (10 mL of 0.1 M NaOH + 20 mL of 0.1 M acetic acid) diluted to 60 mL |
1. | the value of [H+] does not change on dilution |
| Q. | (20 mL of 0.1 M NaOH + 20 mL of 0.1 M acetic acid) diluted to 80 mL |
2. | the value of [H+] changes to half of its initial value on dilution |
| R. | (20 mL of 0.1 M HCL + 20 mL of 0.1 M ammonia solution) diluted to 80 mL |
3. | the value of [H+] changes to two times of its initial value on dilution |
| S. | 10 mL saturated solution of Ni(OH)2 in equilibrium with excess solid Ni(OH)2 is diluted to 20 mL (solid Ni(OH)2 is still present after dilution). |
4. | the value of [H+] changes to $${1 \over {\sqrt 2 }}$$ times of its initial value on dilution |
| 5. | the value of [H+] changes to $$\sqrt 2 $$ times of its initial value on dilution |
Match each process given in LIST-I with one or more effect(s) in LIST-II. The correct option is :
16
The desired product $$X$$ can be prepared by reacting the major product of the reactions in LIST-I with one or more appropriate reagents in LIST-II (given, order of migratory aptitude: aryl > alkyl > hydrogen)
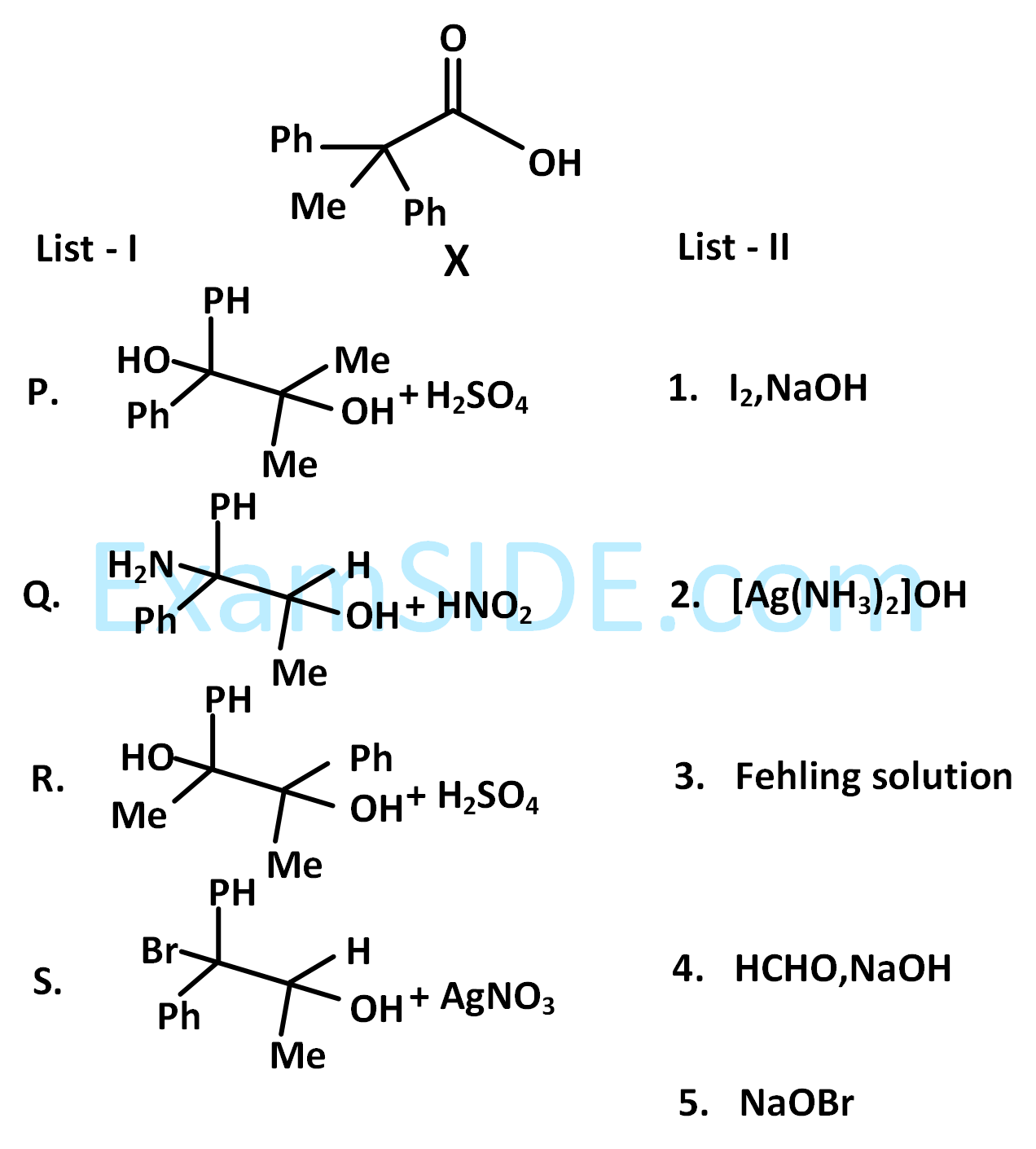
The correct option is

The correct option is
17
LIST-I contains reactions and LIST-II contains major products.
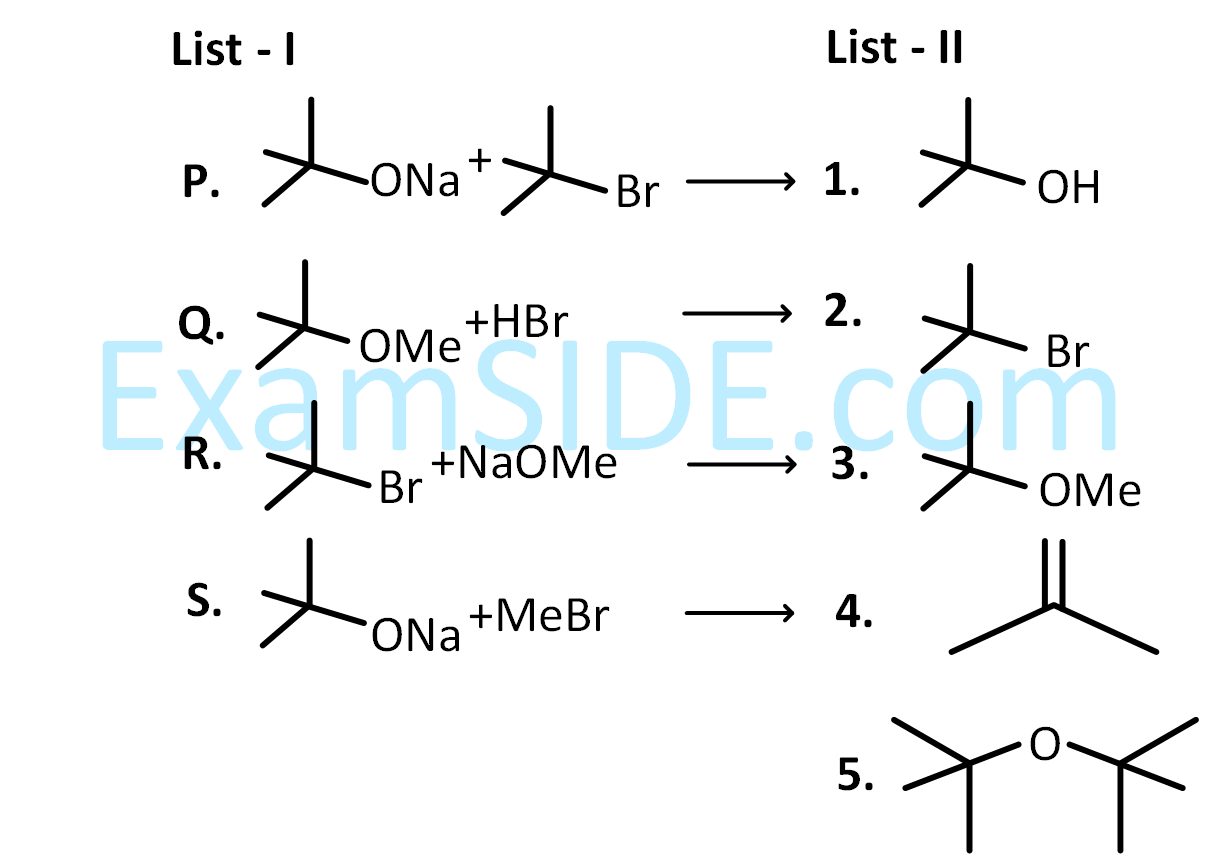
Match the reaction in LIST-I with one or more products in LIST-II and choose the correct option.

Match the reaction in LIST-I with one or more products in LIST-II and choose the correct option.
18
For a reaction, $$A\,\,\rightleftharpoons\,\,P,$$ the plots of $$\left[ A \right]$$ and $$\left[ P \right]$$ with time at temperature $${T_1}$$ and $${T_2}$$ are given below.

If $${T_2} > {T_1},$$ the correct statement(s) is (are) (Assume $$\Delta {H^ \circ }$$ and $$\Delta {S^ \circ }$$ are independent of temperature and ratio of $$lnK$$ at $${T_1}$$ to $$lnK$$ at $${T_2}$$ is greater than $${{{T_2}} \over {{T_1}}}.$$ Here $$H,$$ $$S,G$$ and $$K$$ are enthalpy, entropy, Gibbs energy and equilibrium constant, respectively.)

If $${T_2} > {T_1},$$ the correct statement(s) is (are) (Assume $$\Delta {H^ \circ }$$ and $$\Delta {S^ \circ }$$ are independent of temperature and ratio of $$lnK$$ at $${T_1}$$ to $$lnK$$ at $${T_2}$$ is greater than $${{{T_2}} \over {{T_1}}}.$$ Here $$H,$$ $$S,G$$ and $$K$$ are enthalpy, entropy, Gibbs energy and equilibrium constant, respectively.)
Mathematics
1
For any positive integer n, define
$${f_n}:(0,\infty ) \to R$$ as
$${f_n} = \sum\limits_{j = 1}^n {{{\tan }^{ - 1}}} \left( {{1 \over {1 + (x + j)(x + j - 1)}}} \right)$$
for all x$$ \in $$(0, $$\infty $$). (Here, the inverse trigonometric function tan$$-$$1 x assumes values in $$\left( { - {\pi \over 2},{\pi \over 2}} \right)$$). Then, which of the following statement(s) is (are) TRUE?
$${f_n}:(0,\infty ) \to R$$ as
$${f_n} = \sum\limits_{j = 1}^n {{{\tan }^{ - 1}}} \left( {{1 \over {1 + (x + j)(x + j - 1)}}} \right)$$
for all x$$ \in $$(0, $$\infty $$). (Here, the inverse trigonometric function tan$$-$$1 x assumes values in $$\left( { - {\pi \over 2},{\pi \over 2}} \right)$$). Then, which of the following statement(s) is (are) TRUE?
2
Let T be the line passing through the points P($$-$$2, 7) and Q(2, $$-$$5). Let F1 be the set of al pairs of circles (S1, S2) such that T is tangent to S1 at P and tangent to S2 at Q, and also such that S1 and S2 touch each other at a point, say M. Let E1 be the set representing the locus of M as the pair (S1, S2) varies in F1. Let the set of all straight line segments joining a pair of distinct points of E1 and passing through the point R(1, 1) be F2. Let E2 be the set of the mid-points of the line segments in the set F2. Then, which of the following statement(s) is (are) TRUE?
3
Let S be the set of all column matrices $$\left[ {\matrix{
{{b_1}} \cr
{{b_2}} \cr
{{b_3}} \cr
} } \right]$$ such that $${b_1},{b_2},{b_3} \in R$$ and the system of equations (in real variables)
$$\eqalign{ & - x + 2y + 5z = {b_1} \cr & 2x - 4y + 3z = {b_2} \cr & x - 2y + 2z = {b_3} \cr} $$
has at least one solution. Then, which of the following system(s) (in real variables) has (have) at least one solution for each $$\left[ {\matrix{ {{b_1}} \cr {{b_2}} \cr {{b_3}} \cr } } \right]$$$$ \in $$S?
$$\eqalign{ & - x + 2y + 5z = {b_1} \cr & 2x - 4y + 3z = {b_2} \cr & x - 2y + 2z = {b_3} \cr} $$
has at least one solution. Then, which of the following system(s) (in real variables) has (have) at least one solution for each $$\left[ {\matrix{ {{b_1}} \cr {{b_2}} \cr {{b_3}} \cr } } \right]$$$$ \in $$S?
4
Consider two straight lines, each of which is tangent to both the circle x2 + y2 = (1/2) and the parabola y2 = 4x. Let these lines intersect at the point Q. Consider the ellipse whose centre is at the origin O(0, 0) and whose semi-major axis is OQ. If the length of the minor axis of this ellipse is $$\sqrt 2 $$, then which of the following statement(s) is (are) TRUE?
5
Let s, t, r be non-zero complex numbers and L be the set of solutions $$z = x + iy(x,y \in R,\,i = \sqrt { - 1} )$$ of the equation $$sz + t\overline z + r = 0$$ where $$\overline z $$ = x $$-$$ iy. Then, which of the following statement(s) is(are) TRUE?
6
Let f : (0, $$\pi $$) $$ \to $$ R be a twice differentiable function such that $$\mathop {\lim }\limits_{t \to x} {{f(x)\sin t - f(t)\sin x} \over {t - x}} = {\sin ^2}x$$ for all x$$ \in $$ (0, $$\pi $$).
If $$f\left( {{\pi \over 6}} \right) = - {\pi \over {12}}$$, then which of the following statement(s) is (are) TRUE?
If $$f\left( {{\pi \over 6}} \right) = - {\pi \over {12}}$$, then which of the following statement(s) is (are) TRUE?
7
The value of the integral
$$\int_0^{1/2} {{{1 + \sqrt 3 } \over {{{({{(x + 1)}^2}{{(1 - x)}^6})}^{1/4}}}}dx} $$ is ........
$$\int_0^{1/2} {{{1 + \sqrt 3 } \over {{{({{(x + 1)}^2}{{(1 - x)}^6})}^{1/4}}}}dx} $$ is ........
8
Let P be a matrix of order 3 $$ \times $$ 3 such that all the entries in P are from the set {$$-$$1, 0, 1}. Then, the maximum possible value of the determinant of P is ............ .
9
Let X be a set with exactly 5 elements and Y be a set with exactly 7 elements. If $$\alpha $$ is the number of one-one functions from X to Y and $$\beta $$ is the number of onto functions from Y to X, then the value of $${1 \over {5!}}(\beta - \alpha )$$ is ..................
10
Let f : R $$ \to $$ R be a differentiable function with f(0) = 0. If y = f(x) satisfies the differential equation $${{dy} \over {dx}} = (2 + 5y)(5y - 2)$$, then the value of $$\mathop {\lim }\limits_{n \to - \infty } f(x)$$ is ...........
11
Let f : R $$ \to $$ R be a differentiable function with f(0) = 1 and satisfying the equation f(x + y) = f(x) f'(y) + f'(x) f(y) for all x, y$$ \in $$ R.
Then, the value of loge(f(4)) is ...........
Then, the value of loge(f(4)) is ...........
12
Let P be a point in the first octant, whose image Q in the plane x + y = 3 (that is, the line segment PQ is perpendicular to the plane x + y = 3 and the mid-point of PQ lies in the plane x + y = 3) lies on the Z-axis. Let the distance of P from the X-axis be 5. If R is the image of P in the XY-plane, then the length of PR is ...............
13
Consider the cube in the first octant with sides OP, OQ and OR of length 1, along the X-axis, Y-axis and Z-axis, respectively, where O(0, 0, 0) is the origin. Let $$S\left( {{1 \over 2},{1 \over 2},{1 \over 2}} \right)$$ be the centre of the cube and T be the vertex of the cube opposite to the origin O such that S lies on the diagonal OT. If p = SP, q = SQ, r = SR and t = ST, then the value of |(p $$ \times $$ q) $$ \times $$ (r $$ \times $$ t)| is ............
14
Let $$X = {({}^{10}{C_1})^2} + 2{({}^{10}{C_2})^2} + 3{({}^{10}{C_3})^2} + ... + 10{({}^{10}{C_{10}})^2}$$,
where $${}^{10}{C_r}$$, r $$ \in $${1, 2, ..., 10} denote binomial coefficients. Then, the value of $${1 \over {1430}}X$$ is ..........
where $${}^{10}{C_r}$$, r $$ \in $${1, 2, ..., 10} denote binomial coefficients. Then, the value of $${1 \over {1430}}X$$ is ..........
15
Let $${E_1} = \left\{ {x \in R:x \ne 1\,and\,{x \over {x - 1}} > 0} \right\}$$ and
$${E_2} = \left\{ \matrix{ x \in {E_1}:{\sin ^{ - 1}}\left( {{{\log }_e}\left( {{x \over {x - 1}}} \right)} \right) \hfill \cr is\,a\,real\,number \hfill \cr} \right\}$$
(Here, the inverse trigonometric function $${\sin ^{ - 1}}$$ x assumes values in $$\left[ { - {\pi \over 2},{\pi \over 2}} \right]$$.).
Let f : E1 $$ \to $$ R be the function defined by f(x) = $${{{\log }_e}\left( {{x \over {x - 1}}} \right)}$$ and g : E2 $$ \to $$ R be the function defined by g(x) = $${\sin ^{ - 1}}\left( {{{\log }_e}\left( {{x \over {x - 1}}} \right)} \right)$$.
The correct option is :
$${E_2} = \left\{ \matrix{ x \in {E_1}:{\sin ^{ - 1}}\left( {{{\log }_e}\left( {{x \over {x - 1}}} \right)} \right) \hfill \cr is\,a\,real\,number \hfill \cr} \right\}$$
(Here, the inverse trigonometric function $${\sin ^{ - 1}}$$ x assumes values in $$\left[ { - {\pi \over 2},{\pi \over 2}} \right]$$.).
Let f : E1 $$ \to $$ R be the function defined by f(x) = $${{{\log }_e}\left( {{x \over {x - 1}}} \right)}$$ and g : E2 $$ \to $$ R be the function defined by g(x) = $${\sin ^{ - 1}}\left( {{{\log }_e}\left( {{x \over {x - 1}}} \right)} \right)$$.
| LIST-I | LIST-II |
|---|---|
| P. The range of $f$ is | 1. $\left( -\infty, \frac{1}{1-e} \right] \cup \left[ \frac{e}{e-1}, \infty \right)$ |
| Q. The range of $g$ contains | 2. $(0, 1)$ |
| R. The domain of $f$ contains | 3. $\left[ -\frac{1}{2}, \frac{1}{2} \right]$ |
| S. The domain of $g$ is | 4. $(-\infty, 0) \cup (0, \infty)$ |
| 5. $\left( -\infty, \frac{e}{e-1} \right)$ | |
| 6. $(-\infty, 0) \cup \left( \frac{1}{2}, \frac{e}{e-1} \right]$ |
16
In a high school, a committee has to be formed from a group of 6 boys M1, M2, M3, M4, M5, M6 and 5 girls G1, G2, G3, G4, G5.
(i) Let $$\alpha $$1 be the total number of ways in which the committee can be formed such that the committee has 5 members, having exactly 3 boys and 2 girls.
(ii) Let $$\alpha $$2 be the total number of ways in which the committee can be formed such that the committee has at least 2 members, and having an equal number of boys and girls.
i) Let $$\alpha $$3 be the total number of ways in which the committee can be formed such that the committee has 5 members, at least 2 of them being girls.
(iv) Let $$\alpha $$4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls such that both M1 and G1 are NOT in the committee together.
The correct option is
(i) Let $$\alpha $$1 be the total number of ways in which the committee can be formed such that the committee has 5 members, having exactly 3 boys and 2 girls.
(ii) Let $$\alpha $$2 be the total number of ways in which the committee can be formed such that the committee has at least 2 members, and having an equal number of boys and girls.
i) Let $$\alpha $$3 be the total number of ways in which the committee can be formed such that the committee has 5 members, at least 2 of them being girls.
(iv) Let $$\alpha $$4 be the total number of ways in which the committee can be formed such that the committee has 4 members, having at least 2 girls such that both M1 and G1 are NOT in the committee together.
| LIST-I | LIST-II |
|---|---|
| P. The value of $\alpha_1$ is | 1. 136 |
| Q. The value of $\alpha_2$ is | 2. 189 |
| R. The value of $\alpha_3$ is | 3. 192 |
| S. The value of $\alpha_4$ is | 4. 200 |
| 5. 381 | |
| 6. 461 |
17
Let $$H:{{{x^2}} \over {{a^2}}} - {{{y^2}} \over {{b^2}}} = 1$$, where a > b > 0, be a hyperbola in the XY-plane whose conjugate axis LM subtends an angle of 60$$^\circ $$ at one of its vertices N. Let the area of the $$\Delta $$LMN be $$4\sqrt 3 $$.
| List - I | List - II | ||
|---|---|---|---|
| P. | The length of the conjugate axis of H is | 1. | 8 |
| Q. | The eccentricity of H is | 2. | $${4 \over {\sqrt 3 }}$$ |
| R. | The distance between the foci of H is | 3. | $${2 \over {\sqrt 3 }}$$ |
| S. | The length of the latus rectum of H is | 4. | 4 |
18
Let $${f_1}:R \to R,\,{f_2}:\left( { - {\pi \over 2},{\pi \over 2}} \right) \to R,\,{f_3}:( - 1,{e^{\pi /2}} - 2) \to R$$ and $${f_4}:R \to R$$ be functions defined by
(i) $${f_1}(x) = \sin (\sqrt {1 - {e^{ - {x^2}}}} )$$,
(ii) $${f_2}(x) = \left\{ \matrix{ {{|\sin x|} \over {\tan { - ^1}x}}if\,x \ne 0,\,where \hfill \cr 1\,if\,x = 0 \hfill \cr} \right.$$
the inverse trigonometric function tan$$-$$1x assumes values in $$\left( { - {\pi \over 2},{\pi \over 2}} \right)$$,
(iii) $${f_3}(x) = [\sin ({\log _e}(x + 2))]$$, where for $$t \in R,\,[t]$$ denotes the greatest integer less than or equal to t,
(iv) $${f_4}(x) = \left\{ \matrix{ {x^2}\sin \left( {{1 \over x}} \right)\,if\,x \ne 0 \hfill \cr 0\,if\,x = 0 \hfill \cr} \right.$$
(i) $${f_1}(x) = \sin (\sqrt {1 - {e^{ - {x^2}}}} )$$,
(ii) $${f_2}(x) = \left\{ \matrix{ {{|\sin x|} \over {\tan { - ^1}x}}if\,x \ne 0,\,where \hfill \cr 1\,if\,x = 0 \hfill \cr} \right.$$
the inverse trigonometric function tan$$-$$1x assumes values in $$\left( { - {\pi \over 2},{\pi \over 2}} \right)$$,
(iii) $${f_3}(x) = [\sin ({\log _e}(x + 2))]$$, where for $$t \in R,\,[t]$$ denotes the greatest integer less than or equal to t,
(iv) $${f_4}(x) = \left\{ \matrix{ {x^2}\sin \left( {{1 \over x}} \right)\,if\,x \ne 0 \hfill \cr 0\,if\,x = 0 \hfill \cr} \right.$$
| LIST-I | LIST-II |
|---|---|
| P. The function $$ f_1 $$ is | 1. NOT continuous at $$ x = 0 $$ |
| Q. The function $$ f_2 $$ is | 2. continuous at $$ x = 0 $$ and NOT differentiable at $$ x = 0 $$ |
| R. The function $$ f_3 $$ is | 3. differentiable at $$ x = 0 $$ and its derivative is NOT continuous at $$ x = 0 $$ |
| S. The function $$ f_4 $$ is | 4. differentiable at $$ x = 0 $$ and its derivative is continuous at $$ x = 0 $$ |
Physics
1
A particle, of mass $${10^{ - 3}}$$ $$kg$$ and charge $$1.0$$ $$C,$$ is initially at rest. At time $$t=0,$$ the particle comes under the influence of an electric field $$\overrightarrow E \left( t \right) = {E_0}\sin \,\,$$ $$\omega t\widehat i,$$ where $${E_0} = 1.0\,N{C^{ - 1}}$$ and $$\omega = 10{}^3\,rad\,{s^{ - 1}}.$$ Consider the effect of only the electrical force on the particle. Then the maximum speed, in $$m{s^{ - 1}},$$ attained by the particle at subsequent times is _______________.
2
Consider a thin square plate floating on a viscous liquid in a large tank. The height $$h$$ of the liquid in the tank is much less than the width of the tank. The floating place is pulled horizontally with a constant velocity $${\mu _{0.}}$$ Which of the following statements is (are) true?
3
An infinitely long thin non-conducting wire is parallel to the $$z$$-axis and carries a uniform line charge density $$\lambda .$$ It pierces a thin non-conducting spherical shell of radius $$R$$ in such a way that the arc $$PQ$$ subtends an angle $${120^ \circ }$$ at the center $$O$$ of the spherical shell, as shown in the figure. The permittivity of free space is $${ \in _0}.$$ Which of the following statement is (are) true?
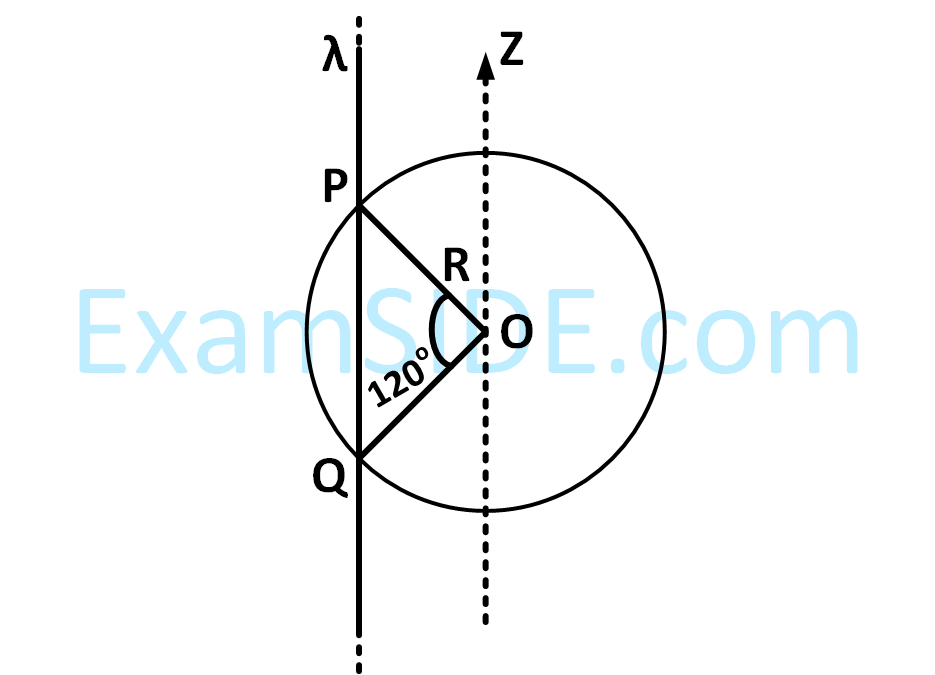

4
In a radioactive decay chain, $${}_{90}^{232}Th$$ nucleus decays to $${}_{82}^{212}Pb$$ nucleus. Let $${N_\alpha }$$ and $${N_\beta }$$ be the number of $$\alpha $$ and $${\beta ^ - }$$ particles, respectively, emitted in this decay process. Which of the following statements is (are) true?
5
A solid horizontal surface is covered with a thin layer of oil. A rectangular block of mass $$m=0.4$$ $$kg$$ is at rest on this surface. An impulse of $$1.0$$ $$Ns$$ is applied to the block at time $$t=0$$ so that it starts moving along the $$x$$-axis with a velocity $$v\left( t \right) = {v_0}{e^{ - t/\tau }},$$ where $${v_0}$$ is a constant and $$\tau = 4s.$$ The displacement of the block, in metres, at $$t = \tau $$ is ______________ Take $${e^{ - 1}} = 0.37.$$
6
In an experiment to measure the speed of sound by a resonating air column, a tuning fork of frequency $$500$$ $$Hz$$ is used. The length of the air column is varied by changing the level of water in the resonance tube. Two successive resonances are heard at air columns of length $$50.7$$ $$cm$$ and $$83.9$$ $$cm.$$ Which of the following statements is (are) true?
7
A wire is bent in the shape of a right angled triangle and is placed in front of a concave mirror of focal length $$f,$$ as shown in the figure. Which of the figures shown in the four options qualitatively represent(s) the shape of the image of the bent wire? (These figures are not to scale.)


8
A moving coil galvanometer has $$50$$ turns and each turn has an area $$2 \times {10^{ - 4}}\,{m^2}.$$ The magnetic field produced by the magnet inside the galvanometer is $$0.02$$ $$T.$$ The torsional constant of the suspension wire is $${10^{ - 4}}\,N\,m\,ra{d^{ - 1}}.$$ When a current flows through the galvanometer, a full scale deflection occurs if the coil rotates by $$0.2$$ $$rad$$. The resistance of the coil of the galvanometer is $$50\Omega .$$ This galvanometer is to be converted into an ammeter capable of measuring current in the range $$0-1.0$$ $$A$$. For this purpose, a shunt resistance is to be added in parallel to the galvanometer. The value of this shunt resistance, in ohms, is _____________.
9
A ball is projected from the ground at an angle of $${45^o}$$ with the horizontal surface. It reaches a maximum height of $$120$$ $$m$$ and returns to the ground. Upon hitting the ground for the first time, it loses half of its kinetic energy. Immediately after the bounce, the velocity of the ball makes an angle of $${30^o}$$ with the horizontal surface. The maximium height it reaches after the bounce, in metres, is ______________.
10
A steel wire of diameter $$0.5$$ $$mm$$ and Young's modulus $$2 \times {10^{11}}\,\,N{m^{ - 2}}$$ carries a load of mass $$M.$$ The length of the wire with the load is $$1.0$$ $$m.A$$ vernier scale with $$10$$ divisions is attached to the end of this wire. Next to the steel wire is a reference wire to which a main scale, of least count $$1.0$$ $$mm$$ , is attached. The $$10$$ divisions of the vernier scale correspond to $$9$$ divisions of the main scale. Initially, the zero of vernier scale coincides with the zero of main scale. If the load on the steel wire is increased by $$1.2$$ $$kg,$$ the vernier scale division which coincides with a main scale division is _____________. Take $$g = 10\,m\,{s^{ - 2}}.$$ and $$\pi = 3.2.$$
11
One mole of a monatomic ideal gas undergoes an adiabatic expansion in which its volume becomes eight times its initial value. If the initial temperature of the gas is $$100\,K$$ and the universal gas constant $$R=8.0$$ $$J\,mo{l^{ - 1}}{K^{ - 1}},$$ the decrease in its internal energy, in Joule, is ____________.
12
In a photoelectric experiment a parallel beam of monochromatic light with power of $$200$$ $$W$$ is incident on a perfectly absorbing cathode of work function $$6.25$$ $$ev.$$ The frequency of light is just above the threshold frequency so that the photoelectrons are emitted with negligible kinetic energy. Assume that the photoelectron emission efficiency is $$100\% $$. A potential difference of $$500$$ $$V$$ is applied between the cathode and the anode. All the emitted electrons are incident normally on the anode and are absorbed. The anode experiences a force $$F = n \times {10^{ - 4}}$$ $$N$$ due to the impact of the electrons. The value of $$n$$ is ______________. Mass of the electron $${M_e} = 9 \times {10^{ - 31}}\,kg$$ and $$1.0eV = 1.6 \times {10^{ - 19}}\,J.$$
13
Consider a hydrogen-like ionized atom with atomic number $$Z$$ with a single electron. In the emission spectrum of this atom, the photon emitted in the $$n=2$$ to $$n=1$$ transition has energy $$74.8eV$$ higher than the photon emitted in the $$n=3$$ to $$n=2$$ transition. The ionization energy of the hydrogen atom is $$13.6$$ $$eV.$$ The value of $$Z$$ is ____________.
14
A planet of mass $$M,$$ has two natural satellites with masses $${m_1}$$ and $${m_2}.$$ The radii of their circular orbits are $${R_1}$$ and $${R_2}$$ respectively, Ignore the gravitational force between the satellites. Define $${v_1},{L_1},{K_1}$$ and $${T_1}$$ to be , respectively, the orbital speed, angular momentum, kinetic energy and time period of revolution of satellite $$1$$; and $${v_2},{L_2},{K_2},$$ and $${T_2}$$ to be the corresponding quantities of satellite $$2.$$ Given $${m_1}/{m_2} = 2$$ and $${R_1}/{R_2} = 1/4,$$ match the ratios in List-$${\rm I}$$ to the numbers in List-$${\rm II}.$$
| LIST - I | LIST - II | ||
|---|---|---|---|
| P. | v1/v2 | 1. | 1/8 |
| Q. | L1/L2 | 2. | 1 |
| R. | K1/K2 | 3. | 2 |
| S. | T1/T2 | 4. | 8 |
15
The electric field $$E$$ is measured at a point $$P(0,0,d)$$ generated due to various charge distributions and the dependence of $$E$$ on $$d$$ is found to be different for different charge distributions. List-$${\rm I}$$ contains different relations between $$E$$ and $$d$$. List-$${\rm II}$$ describes different electric charge distributions, along with their locations. Match the functions in List-$${\rm I}$$ with the related charge distributions in List-$${\rm II}$$.
| LIST - I | LIST - II | ||
|---|---|---|---|
| P. | $$E$$ is independent of $$d$$ | 1. | A point charge Q at the origin |
| Q. | $$E\, \propto \,1/d$$ | 2. | A small dipole with point charges $$Q$$ at $$\left( {0,0,l} \right)$$ and $$-Q$$ at $$\left( {0,0, - l} \right).$$ Take $$2l < < d$$ |
| R. | $$E\, \propto \,1/{d^2}$$ | 3. | An infinite line charge coincident with the x-axis, with uniform linear charge density $$\lambda $$ |
| S. | $$E\, \propto \,1/{d^3}$$ | 4. | Two infinite wires carrying uniform linear charge density parallel to the $$x$$-axis. The one along $$\left( {y = 0,z = l} \right)$$ has a charge density $$ + \lambda $$ and the one along $$\left( {y = 0,z = - l} \right)$$ has a charge density Take |
| 5. | Infinite plane charge coincident
with the $$xy$$-plane with uniform surface charge density |
||
16
One mole of a monatomic ideal gas undergoes four thermodynamic processes as shown schematically in the $$PV$$-diagram below. Among these four processes, one is isobaric, one is isochoric, one is isothermal and one is adiabatic. Match the processes mentioned in List-I with the corresponding statements in List-II.
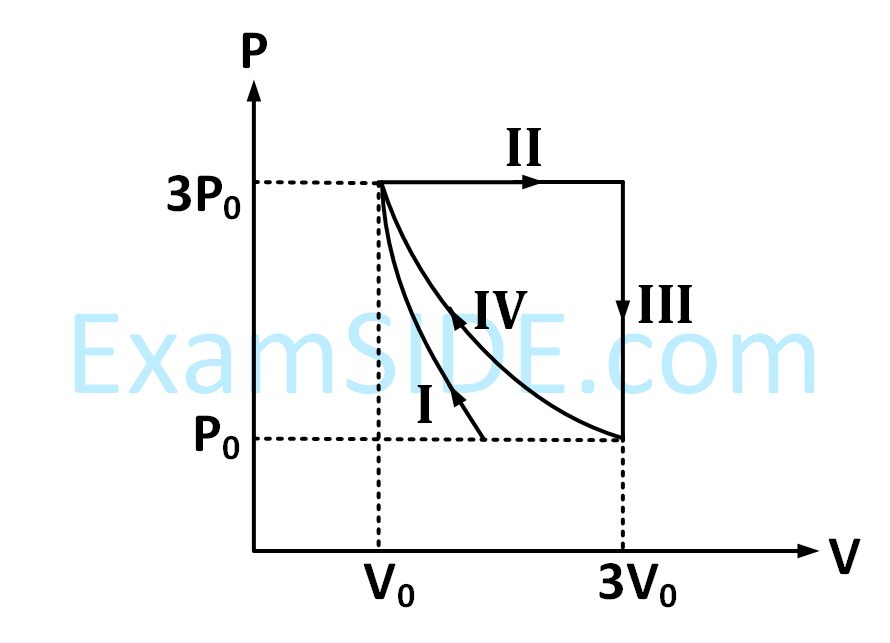

| LIST - I | LIST - II | ||
|---|---|---|---|
| P. | In process I | 1. | Work done by the gas is zero |
| Q. | In process II | 2. | Temperature of the gas remains unchanged |
| R. | In process III | 3. | No heat is exchanged between the gas and its surroundings |
| S. | In process IV | 4. | Work done by the gas is 6P0V0 |
17
In the List-$${\rm I}$$ below, four different paths of a particle are given as functions of time. In these functions, $$\alpha $$ and $$\beta $$ are positive constants of appropriate dimensions and $$\alpha \ne \beta $$ In each case, the force acting on the particle is either zero or conservative. In List-$${\rm I}{\rm I}$$, five physical quantities of the particle are mentioned $$\overrightarrow p $$ is the linear momentum, $$\overrightarrow L $$ is the angular momentum about the origin, $$K$$ is the kinetic energy, $$U$$ is the potential energy and $$E$$ is the total energy. Match each path in List-$${\rm I}$$ with those quantities in List-$${\rm II}$$, which are conserved for that path.
| LIST - I | LIST - II | ||
|---|---|---|---|
| P. | $$\overrightarrow r $$(t)=$$\alpha $$ $$t\,\widehat i + \beta t\widehat j$$ | 1. | $$\overrightarrow p $$ |
| Q. | $$\overrightarrow r \left( t \right) = \alpha \cos \,\omega t\,\widehat i + \beta \sin \omega t\,\widehat j$$ | 2. | $$\overrightarrow L $$ |
| R. | $$\overrightarrow r \left( t \right) = \alpha \left( {\cos \omega t\,\widehat i + \sin \omega t\widehat j} \right)$$ | 3. | K |
| S. | $$\overrightarrow r \left( t \right) = \alpha t\,\widehat i + {\beta \over 2}{t^2}\widehat j$$ | 4. | U |
| 5. | E | ||
18
A particle of mass $$m$$ is initially at rest at the origin. It is subjected to a force and starts moving along the $$x$$-axis. Its kinetic energy $$K$$ changes with time as $$dK/dt = \gamma t,$$ where $$\gamma $$ is a positive constant of appropriate dimensions. Which of a positive constant of appropriate dimensions. Which of the following statement is (are) true?