The dipole moment of CH₃F is greater than of CH₃Cl

Upon mixing 45.0 ml. of 0.25 M lead nitrate solution with 25.0 ml. of 0.10M chromic sulphate solution, precipitation of lead sulphate takes place. How many moles of lead sulphate are formed? Also, calculate the molar concentrations of the species left behind in the final solution. Assume that lead sulphate is completely insoluble.

The light radiations with discrete quantities of energy are called ______.

Wave functions of electrons in atoms and molecules are called ______.

The 2p_x, 2p_y and 2p_z orbitals of atom have identical shapes but differ in their _____.

In a given electric field, $$\beta-particles $$ are deflected more than $$\alpha-particles$$ in spite of $$\alpha-particles$$ having larger charge.

Estimate the difference in energy between 1st and 2nd Bohr orbit for a hydrogen atom. At what minimum atomic number, a transition from n = 2 to n = 1 energy level would result in the emission of X-rays with $$\lambda = 3.0 \times {10^{ - 8}}$$? Which hydrogen atom like species does this atomic number correspond to?

What transition in the hydrogen spectrum would have the same wavelength as the Balmer transition n = 4 to n = 2 of He⁺ spectrum?

The decreasing order of electron affinity of F, Cl, Br is F > Cl > Br

The basic nature of the hydroxides of group 13 (Gr. III B) decreases progressively down the group.

Pick out the isoelectronic structures from the following
I. $$CH_3^+$$
II. $$H_3O^+$$
III. $$NH_3$$
IV. $$CH_3^-$$

The dipole moment of KCl is 3.336 $$\times$$ 10^-29 Coulomb meters which indicates that it is a highly polar molecule. The interatomic distance between K⁺ and Cl^- in this molecule is 2.6 $$\times$$ 10^-10. Calculate the dipole moment of KCl molecule if there were opposite charges of one fundamental unit located at each nucleus. Calculate the percentage ionic character of KCl.

In the van der Waal's equation (P + $${{{n^2}a} \over {{V^2}}}$$)(V - nb) = nRT the constant 'a' reflects the actual volume of the gas molecules.

A gas bulb of 1 litre capacity contains 2.0 $$\times$$ 10²¹ molecules of nitrogen exerting a pressure of 7.57 $$\times$$ 10³ Nm^-2. Calculate the root mean square (r.m.s) speed and the temparature of the gas molecules. If the ratio of the most probable speed to the root mean square speed is 0.82, calculate the most probable speed for these molecules at this temparature.

Ca²⁺ has a small ionic radius than K⁺ because it has ______.

Give briefly the isolation of magnesium from sea water by the Dow process. Give equations for the steps involved.

What weight of the non-volatile solute, urea(NH₂ - CO - NH₂) needs to be dissolved in 100g of water, in order to decrease the vapour pressure of water by 25%? What will be the molality of the solution?

The standard reduction potential for the half-cell
$$NO_3^-$$ + 2H⁺ (aq) + e $$\to$$ NO₂ (g) + H₂O is 0.78 V
(i) Calculate the reduction potential in 8 M H⁺
(ii) What will be the reduction potential of the half-cell in a neutral solution? Assume all the other species to be at unit concentration.

Chromium metal can be plated out from an acidic solution containing CrO₃ according to the following equation
CrO₃ (aq) + 6H⁺ (aq) + 6e^- $$\to$$ Cr(s) + 3H₂O
Calculate (i) how many grams of chromium will be plated out by 24,000 coulombs and (ii) how long will it take to plate out 1.5 g of chromium by using 12.5 amp current.

A first order reaction A $$\to$$ B, requires activation energy of 70 kJ mol^-1. When 20% solution of A was kept at 25^oC for 20 minutes, 25% decomposition tooks place. What will be the percentage decomposition in the same time in a 30% solution maintained at 40^oC? Assume that activation energy remains constant in this range of temperature.

Let $$a, b, c$$ be distinct non-negative numbers. If the vectors $$a\widehat i + a\widehat j + c\widehat k,\widehat i + \widehat k$$ and $$c\widehat i + c\widehat j + b\widehat k$$ lie in a plane, then $$c$$ is

Let $$\vec a = 2\hat i - \hat j + \hat k,\vec b = \hat i + 2\hat j - \hat k$$ and $$\overrightarrow c = \widehat i + \widehat j - 2\widehat k - 2\widehat k$$ be three vectors. A vector in the plane of $${\overrightarrow b }$$ and $${\overrightarrow c }$$, whose projection on $${\overrightarrow a }$$ is of magnitude $$\sqrt {2/3,} $$ is :

In a triangle $$ABC, D$$ and $$E$$ are points on $$BC$$ and $$AC$$ respectively, such that $$BD=2DC$$ and $$AE=3EC.$$ Let $$P$$ be the point of intersection of $$AD$$ and $$BE.$$ Find $$BP/PE$$ using vector methods.

Numbers are selected at random, one at a time, from the two- digit numbers $$00, 01, 02 ......, 99$$ with replacement. An event $$E$$ occurs if only if the product of the two digits of a selected number is $$18$$. If four numbers are selected, find probability that the event $$E$$ occurs at least $$3$$ times.

Find the coordinates of the point at which the circles $${x^2}\, + \,{y^2} - \,4x - \,2y = - 4\,\,and\,\,{x^2}\, + \,{y^2} - \,12x - \,8y = - 36$$ touch each other. Also find equations common tangests touching the circles in the distinct points.

If $$A > 0,B > 0\,$$ and $$A + B = \pi /3,$$ then the maximum value of tan A tan B is _______.

Number of solutions of the equation $$\tan x + \sec x = 2\cos x\,$$ lying in the interval $$\left[ {0,2\pi } \right]$$ is:

$$ABCD$$ is a rhombus. Its diagonals $$AC$$ and $$BD$$ intersect at the point $$M$$ and satisfy $$BD$$ = 2$$AC$$. If the points $$D$$ and $$M$$ represent the complex numbers $$1 + i$$ and $$2 - i$$ respectively, then A represents the comp[lex number ..........or..........

Determine the smallest positive value of number $$x$$ (in degrees) for which $$$\tan \left( {x + {{100}^ \circ }} \right) = \tan \left( {x + {{50}^ \circ }} \right)\,\tan \left( x \right)\tan \left( {x - {{50}^ \circ }} \right).$$$

Using mathematical induction, prove that
$${\tan ^{ - 1}}\left( {1/3} \right) + {\tan ^{ - 1}}\left( {1/7} \right) + ........{\tan ^{ - 1}}\left\{ {1/\left( {{n^2} + n + 1} \right)} \right\} = {\tan ^{ - 1}}\left\{ {n/\left( {n + 2} \right)} \right\}$$

Prove that $$\sum\limits_{r = 1}^k {{{\left( { - 3} \right)}^{r - 1}}\,\,{}^{3n}{C_{2r - 1}} = 0,} $$ where $$k = \left( {3n} \right)/2$$ and $$n$$ is an even positive integer.

For $$0 < \phi < \pi /2,$$ if
$$x = $$$$\sum\limits_{n = 0}^\infty {{{\cos }^{2n}}\phi ,y = \sum\limits_{n = 0}^\infty {{{\sin }^{2n}}\phi ,\,\,\,\,z = \sum\limits_{n = 0}^{} {{{\cos }^{2n}}\phi {{\sin }^{2n}}\phi } } } \infty $$ then

The vertices of a triangle are $$A\left( { - 1, - 7} \right)B\left( {5,\,1} \right)$$ and $$C\left( {1,\,4} \right).$$ The equation of the bisector of the angle $$\angle ABC$$ is ............... .

Tagent at a point $${P_1}$$ {other than $$(0, 0)$$} on the curve $$y = {x^3}$$ meets the curve again at $${P_2}$$. The tangent at $${P_2}$$ meets the curve at $${P_3}$$, and so on. Show that the abscissae of $${P_1},\,{P_2},{P_3}......{P_n},$$ form a G.P. Also find the ratio.

[area $$\left( {\Delta {P_1},{P_2},{P_3}} \right)$$]/[area $$\left( {{P_2},{P_3},{P_4}} \right)$$]

A line through $$A (-5, -4)$$ meets the line $$x + 3y + 2 = 0,$$ $$2x + y + 4 = 0$$ and $$x - y - 5 = 0$$ at the points $$B, C$$ and $$D$$ respectively. If $${\left( {15/AB} \right)^2} + {\left( {10/AC} \right)^2} = {\left( {6/AD} \right)^2},$$ find the equation of the line.

The equation of the locus of the mid-points of the circle $$4{x^2} + 4{y^2} - 12x + 4y + 1 = 0$$ that subtend an angle of $$2\pi /3$$ at its centre is.................................

The locus of the centre of a circle, which touches externally the circle $${x^2} + {y^2} - 6x - 6y + 14 = 0$$ and also touches the y-axis, is given by the equation:

If $$K = \sin \left( {\pi /18} \right)\sin \left( {5\pi /18} \right)\sin \left( {7\pi /18} \right),$$ then the numerical value of K is ______.

Consider a family of circles passing through two fixed points A (3, 7) and B (6, 5). Show that the chords on which the circle $${x^2}\, + \,{y^2} - \,4x - \,6y - 3 = 0$$ cuts the members of the family are concurrent at a point. Find the coordinate of this point.

If in a triangle $$ABC$$, $${{2\cos A} \over a} + {{\cos B} \over b} + {{2\cos C} \over c} = {a \over {bc}} + {b \over {ca}},$$ then the value of the angle $$A$$ is .................... degrees.

An observer at $$O$$ notices that the angle of elevation of the top of a tower is $${30^ \circ }$$. The line joining $$O$$ to the base of the tower makes an angle of $${\tan ^{ - 1}}\left( {1/\sqrt 2 } \right)$$ with the North and is inclined Eastwards. The observer travels a distance of $$300$$ meters towards the North to a point A and finds the tower to his East. The angle of elevation of the top of the tower at $$A$$ is $$\phi $$, Find $$\phi $$ and the height of the tower.

If $$f\left( x \right) = \left\{ {\matrix{ {3{x^2} + 12x - 1,} & { - 1 \le x \le 2} \cr {37 - x} & {2 < x \le 3} \cr } } \right.$$ then:

Find the equation of the normal to the curve
$$y = {\left( {1 + x} \right)^y} + {\sin ^{ - 1}}\left( {{{\sin }^2}x} \right)$$ at $$x=0$$

Let $$f\left( x \right) = \left\{ {\matrix{ { - {x^3} + {{\left( {{b^3} - {b^2} + b - 1} \right)} \over {\left( {{b^2} + 3b + 2} \right)}},} & {0 \le x < 1} \cr {2x - 3} & {1 \le x \le 3} \cr } } \right.$$

Find all possible real values of $$b$$ such that $$f(x)$$ has the smallest value at $$x=1$$.

The value of $$\int\limits_{\pi /4}^{3\pi /4} {{\phi \over {1 + \sin \phi }}d\phi } $$ is ..............

The value of $$\int\limits_0^{\pi /2} {{{dx} \over {1 + {{\tan }^3}\,x}}} $$ is

Evaluate $$\int_2^3 {{{2{x^5} + {x^4} - 2{x^3} + 2{x^2} + 1} \over {\left( {{x^2} + 1} \right)\left( {{x^4} - 1} \right)}}} dx.$$

An unbiased die with faces marked $$1,2,3,4,5$$ and $$6$$ is rolled four times. Out of four face values obtained, the probability that the minimum face value is not less than $$2$$ and the maximum face value is not greater than $$5,$$ is then:

$$E$$ and $$F$$ are two independent events. The probability that both $$E$$ and $$F$$ happen is $$1/12$$ and the probability that neither $$E$$ nor $$F$$ happens is $$1/2.$$ Then,

A particle of mass m moves on the x-axis as follows: it starts from rest at t = 0 from the point x = 0, and come to rest at t = 1 at the point x = 1. NO other information is available about its motion at intermediate times ( 0 < t < 1 ). If $$\alpha $$ denotes the instantaneous acceleration of the particle, then:

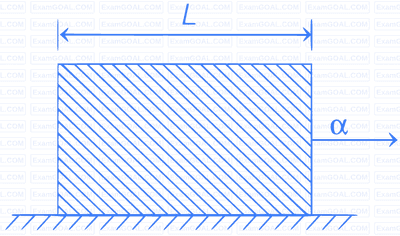
A uniform rod of length L and density $$\rho $$ is being pulled along a smooth floor with a horizontal acceleration $$\alpha $$ (see Fig.). The magnitude of the stress at the transverse cross-secion through the mid-point of the rod is _________.