Chemistry
H2O(l) $$\to$$ H2O(g)
at T = 100oC and 1 atmosphere pressure, the correct choice is
For the identification of $$\beta$$-naphthol using dye test, it is necessary to use
Isomers of hexane, based on their branching, can be divided into three distinct classes as shown in the figure.

The correct order of their boiling point is
The acidic hydrolysis of ether (X) shown below is fastest when

The major product in the following reaction is
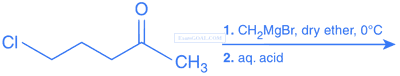
The product formed in the reaction of SOCl2 with white phosphorus is
Under ambient conditions, the total number of gases released as products in the final step of the reaction scheme shown below is

The experimental value of d is found to be smaller than the estimate obtained using Graham's law. This is due to
The correct statement with respect to product Y is
Reagent S is
Match each coordination compound in List I with an appropriate pair of characteristics from List II and select the correct answer using the code given below the lists.
{en = H2NCH2CH2NH2; atomic numbers : Ti = 22, Cr = 24; Co = 27; Pt = 78}
| List I | List II | ||
|---|---|---|---|
| P. | $$[Cr{(N{H_3})_3}C{l_2}]Cl$$ |
1. | Paramagnetic and exhibits ionisation isomerism. |
| Q. | $$[Ti{({H_2}O)_5}Cl]{(N{O_3})_2}$$ |
2. | Diamagnetic and exhibits cis-trans isomerism. |
| R. | $$[Pt(en)(N{H_3})Cl]N{O_3}$$ |
3. | Paramagnetic and exhibits cis-trans isomerism. |
| S. | $$[Co{(N{H_3})_4}{(N{O_3})_2}]N{O_3}$$ |
4. | Diamagnetic and exhibits ionisation isomerism. |
Match the orbital overlap figures shown in List I with the description given in List II and select the correct answer using the code given below the lists.

Different possible thermal decomposition pathways for peroxyesters are shown below. Match each pathway from List I with an appropriate structure from List II and select the correct answer using the code given below the lists.


Match the four starting materials (P, Q, R, S) given in List I with the corresponding reaction schemes (I, II, III, IV) provided in List II and select the correct answer using the code given below the lists.

Mathematics
$$\,\,\,\,$$ $$\,\,\,\,$$ $$\,\,\,\,$$ List-$$I$$
(P.)$$\,\,\,\,$$ Let $$y\left( x \right) = \cos \left( {3{{\cos }^{ - 1}}x} \right),x \in \left[ { - 1,1} \right],x \ne \pm {{\sqrt 3 } \over 2}.$$ Then $${1 \over {y\left( x \right)}}\left\{ {\left( {{x^2} - 1} \right){{{d^2}y\left( x \right)} \over {d{x^2}}} + x{{dy\left( x \right)} \over {dx}}} \right\}$$ equals
(Q.)$$\,\,\,\,$$ Let $${A_1},{A_2},....,{A_n}\left( {n > 2} \right)$$ be the vertices of a regular polygon of $$n$$ sides with its centre at the origin. Let $${\overrightarrow {{a_k}} }$$ be the position vector of the point $${A_k},k = 1,2,......,n.$$
$$$f\left| {\sum\nolimits_{k = 1}^{n - 1} {\left( {\overrightarrow {{a_k}} \times \overrightarrow {{a_{k + 1}}} } \right)} } \right| = \left| {\sum\limits_{k = 1}^{n - 1} {\left( {\overrightarrow {{a_k}} .\,\overrightarrow {{a_{k + 1}}} } \right)} } \right|,$$$
then the minimum value of $$n$$ is
(R.)$$\,\,\,\,$$ If the normal from the point $$P(h, 1)$$ on the ellipse $${{{x^2}} \over 6} + {{{y^2}} \over 3} = 1$$ is perpendicular to the line $$x+y=8,$$ then the value of $$h$$ is
(S.)$$\,\,\,\,$$ Number of positive solutions satisfying the equation $${\tan ^{ - 1}}\left( {{1 \over {2x + 1}}} \right) + {\tan ^{ - 1}}\left( {{1 \over {4x + 1}}} \right) = {\tan ^{ - 1}}\left( {{2 \over {{x^2}}}} \right)$$ is
$$\,\,\,\,$$ $$\,\,\,\,$$ $$\,\,\,\,$$List-$$II$$
(1.)$$\,\,\,\,$$ $$1$$
(2.)$$\,\,\,\,$$ $$2$$
(3.)$$\,\,\,\,$$ $$8$$
(4.)$$\,\,\,\,$$ $$9$$
The probability that $${x_1},$$, $${x_2},$$ $${x_3}$$ are in an arithmetic progression, is
The probability that $${x_1} + {x_2} + {x_3}$$ is odd, is
If $$st=1$$, then the tangent at $$P$$ and the normal at $$S$$ to the parabola meet at a point whose ordinate is
List-I
P. For each $${z_k}$$ = there exits as $${z_j}$$ such that $${z_k}$$.$${z_j}$$ = 1
Q. There exists a $$k \in \left\{ {1,2,....,9} \right\}$$ such that $${z_1}.z = {z_k}$$ has no solution z in the set of complex numbers
R. $${{\left| {1 - {z_1}} \right|\,\left| {1 - {z_2}} \right|\,....\left| {1 - {z_9}} \right|} \over {10}}$$ equals
S. $$1 - \sum\limits_{k = 1}^9 {\cos \left( {{{2k\pi } \over {10}}} \right)} $$ equals
List-II
1. True
2. False
3. 1
4. 2
The value of $$r$$ is
$${{dy} \over {dx}} + {{xy} \over {{x^2} - 1}} = {{{x^4} + 2x} \over {\sqrt {1 - {x^2}} }}\,$$ in $$(-1,1)$$ satisfying $$f(0)=0$$.
Then $$\int\limits_{ - {{\sqrt 3 } \over 2}}^{{{\sqrt 3 } \over 2}} {f\left( x \right)} \,d\left( x \right)$$ is
$$F\left( x \right) = \int\limits_0^{{x^2}} {f\left( {\sqrt t } \right)dt} $$ for $$x \in \left[ {0,2} \right]$$. If $$F'\left( x \right) = f'\left( x \right)$$ for all $$x \in \left[ {0,2} \right]$$, then $$F(2)$$ equals
P.$$\,\,\,\,$$ The number of polynomials $$f(x)$$ with non-negative integer coefficients of degree $$ \le 2$$, satisfying $$f(0)=0$$ and $$\int_0^1 {f\left( x \right)dx = 1,} $$ is
Q.$$\,\,\,\,$$ The number of points in the interval $$\left[ { - \sqrt {13} ,\sqrt {13} } \right]$$
at which $$f\left( x \right) = \sin \left( {{x^2}} \right) + \cos \left( {{x^2}} \right)$$ attains its maximum value, is
R.$$\,\,\,\,$$ $$\int\limits_{ - 2}^2 {{{3{x^2}} \over {\left( {1 + {e^x}} \right)}}dx} $$ equals
S.$$\,\,\,\,$$ $${{\left( {\int\limits_{ - {1 \over 2}}^{{1 \over 2}} {\cos 2x\log \left( {{{1 + x} \over {1 - x}}} \right)dx} } \right)} \over {\left( {\int\limits_0^{{1 \over 2}} {\cos 2x\log \left( {{{1 + x} \over {1 - x}}} \right)dx} } \right)}}$$
List $$II$$
1.$$\,\,\,\,$$ $$8$$
2.$$\,\,\,\,$$ $$2$$
3.$$\,\,\,\,$$ $$4$$
4.$$\,\,\,\,$$ $$0$$
The value of $$g'\left( {{1 \over 2}} \right)$$ is
The value of $$g\left( {{1 \over 2}} \right)$$ is
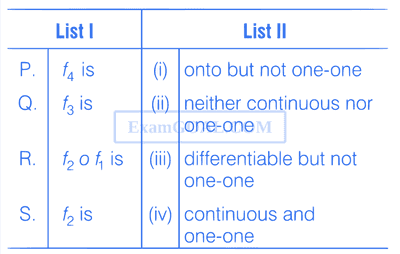
$${f_1}\left( x \right) = \left\{ {\matrix{ {\left| x \right|} & {if\,x < 0,} \cr {{e^x}} & {if\,x \ge 0;} \cr } } \right.$$
f2(x) = x2 ;
$${f_3}\left( x \right) = \left\{ {\matrix{ {\sin x} & {if\,x < 0,} \cr x & {if\,x \ge 0;} \cr } } \right.$$and
$${f_4}\left( x \right) = \left\{ {\matrix{ {{f_2}\left( {{f_1}\left( x \right)} \right)} & {if\,x < 0,} \cr {{f_2}\left( {{f_1}\left( x \right)} \right) - 1} & {if\,x \ge 0;} \cr } } \right.$$
Physics

A person in a lift is holding a water jar, which has a small hole at the lower end of its side. When the lift is at rest, the water jet coming out of the hole hits the floor of the lift at a distance d of 1.2 m from the person. In the following, state of the lift’s motion is given in List I and the distance where the water jet hits the floor of the lift is given in List II. Match the statements from List I with those in List II and select the correct answer using the options given below the lists.
| List - I | List - II |
|---|---|
| P. Lift is accelerating vertically up. | 1. d=1.2 m |
| Q. Lift is accelerating vertically down with an acceleration less than the gravitational acceleration. |
2. d > 1.02 m |
| R. Lift is moving vertically up with constant speed. |
3. d < 1.2 m |
| S. Lift is falling freely. | 4. No water leaks out of the jar |
If $$\lambda$$Cu is the wavelength of K$$\alpha$$ X-ray line of copper (atomic number 29) and $$\lambda$$Mo is the wavelength of the K$$\alpha$$ X-ray line of molybdenum (atomic number 42), then the ratio $$\lambda$$Cu/$$\lambda$$Mo is close to
A metal surface is illuminated by light of two different wavelengths 248 nm and 310 nm. The maximum speeds of the photoelectrons corresponding to these wavelengths are u1 and u2, respectively. If the ratio u1 : u2 = 2 : 1 and hc = 1240 eV nm, the work function of the metal is nearly
During an experiment with a metre bridge, the galvanometer shall a null point when the jockey is pressed at 40.0 cm using a standard resistance of 90$$\Omega$$, as shown in the figure. The least count of the scale used in the meter bridge is 1 mm. The unknown resistance is

A wire, which passes through the hole in a small bead, is bent in the form of quarter of a circle. The wire is fixed vertically on ground as shown in the below figure. The bead is released from near the top of the wire and it slides along the wire without friction. As the bead moves from A to B, the force it applies on the wire is

A glass capillary tube is of the shape of truncated cone with an apex angle $$\alpha$$ so that its two ends have cross sections of different radii. When dipped in water vertically, water rises in it to a height h, where the radius of its cross section is b. If the surface tension of water is S, its density is $$\rho$$, and its contact angle with glass is $$\theta$$, the value of h will be (g is the acceleration due to gravity)

A point source S is placed at the bottom of a transparent block of height 10 mm and refractive index 2.72. It is immersed in a lower refractive index liquid as shown in the below figure. It is found that the light emerging from the block to the liquid forms a circular bright spot of diameter 11.54 mm on the top of the block. The refractive index of the liquid is

When d $$\approx$$ a but wires are not touching the loop, it is found that the net magnetic field on the axis of the loop is zero at a height h above the loop. In that case
Consider d >> a, and the loop is rotated about its diameter parallel to the wires by 30$$^\circ$$ from the position shown in the below figure. If the currents in the wires are in the opposite directions, the torque on the loop at its new position will be (assume that the net field due to the wires is constant over the loop)
Consider the partition to be rigidly fixed so that it does not move. When equilibrium is achieved, the final temperature of the gases will be
Now consider the partition to be free to move without friction so that the pressure of gases in both compartments is the same. Then total work done by the gases till the time they achieve equilibrium will be
If the piston is pushed at a speed of 5 mm s$$-$$1, the air comes out of the nozzle with a speed of
If the density of air is $$\rho$$a and that of the liquid $$\rho$$l, then for a given piston speed the rate (volume per unit time) at which the liquid is sprayed will be proportional to
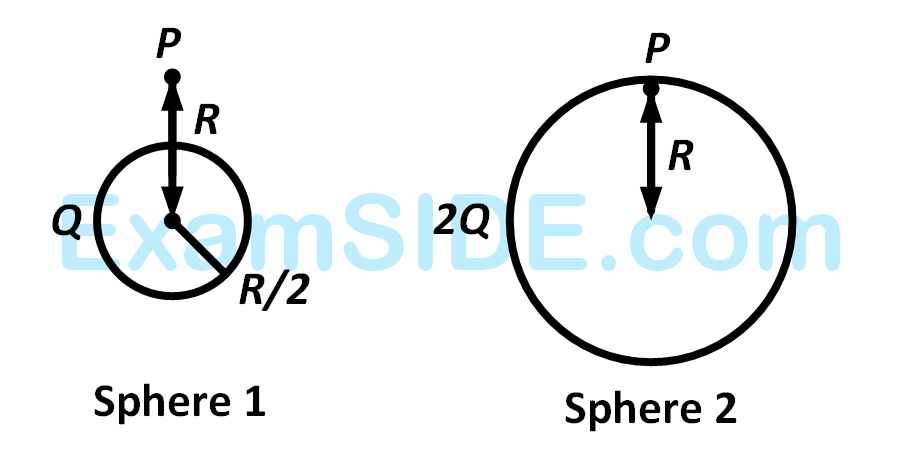
Four charges Q1, Q2, Q3 and Q4 of same magnitude are fixed along the x axis at x = $$-$$2a, $$-$$a, +a and +2a, respectively. A positive charge q is placed on the positive y axis at a distance b > 0. Four options of the signs of these charges are given in List I. The direction of the forces on the charge q is given in List II. Match List I with List II and select the correct answer using the code given below the lists.

| List I | List II | ||
|---|---|---|---|
| P. | Q$$_1$$, Q$$_2$$, Q$$_3$$, Q$$_4$$ all positive | 1. | +x |
| Q. | Q$$_1$$, Q$$_2$$ positive; Q$$_3$$, Q$$_4$$ negative | 2. | $$ - $$x |
| R. | Q$$_1$$, Q$$_4$$ positive; Q$$_2$$, Q$$_3$$ negative | 3. | +y |
| S. | Q$$_1$$, Q$$_3$$ positive; Q$$_2$$, Q$$_4$$ negative | 4. | $$ - $$y |
Four combinations of two thin lenses are given in List I. The radius of curvature of all curved surfaces is r and the refractive index of all the lenses is 1.5. Match lens combinations in List I with their focal length in List II and select the correct answer using the code given below the lists.

A block of mass m1 = 1 kg another mass m2 = 2 kg, are placed together (see figure) on an inclined plane with angle of inclination $$\theta$$. Various values of $$\theta$$ are given in List I. The coefficient of friction between the block m1 and the plane is always zero. The coefficient of static and dynamic friction between the block m2 and the plane are equal to $$\mu$$ = 0.3. In List II expressions for the friction on the block m2 are given. Match the correct expression of the friction in List II with the angles given in List I, and choose the correct option. The acceleration due to gravity is denoted by g.
[Useful information : tan (5.5$$^\circ$$) $$\approx$$ 0.1; tan (11.5$$^\circ$$) $$\approx$$ 0.2; tan (16.5$$^\circ$$) $$\approx$$ 0.3]

| List I | List II | ||
|---|---|---|---|
| P. | $$\theta = 5^\circ $$ |
1. | $${m_2}g\sin \theta $$ |
| Q. | $$\theta = 10^\circ $$ |
2. | $$({m_1} + {m_2})g\sin \theta $$ |
| R. | $$\theta = 15^\circ $$ |
3. | $$\mu {m_2}g\cos \theta $$ |
| S. | $$\theta = 20^\circ $$ |
4. | $$\mu ({m_1} + {m_2})g\cos \theta $$ |