Pure water freezes at $$273$$ $$K$$ and $$1$$ bar. The addition of $$34.5$$ $$g$$ of ethanol to $$500$$ $$g$$ of water changes the freezing point of the solution. Use the freezing point depression constant of water as $$2$$ kg $$mo{l^{ - 1}}.$$ The figures shown below represent plots of vapor pressure $$(V.P.)$$ versus temperature $$(T).$$ [molecular weight of ethanol is $$46$$ $$g$$ $$mo{l^{ - 1}}.$$ ] Among the following, the option representing change in the freezing point is

The product $$S$$ is

The order of basicity among the following compounds is

$$Y$$ and $$Z$$ are, respectively

Which of the following combination will produce $${H_2}$$ gas ?

For the following compounds, the correct statement(s) with respect to nucleophilic substitution reaction is (are)

For the following cell,

$$Zn\left( s \right)\left| {ZnS{O_4}\left( {aq} \right)} \right|\left| {CuS{O_4}\left( {aq} \right)} \right|Cu\left( s \right)$$

when the concentration of $$Z{n^{2 + }}$$ is $$10$$ times the concentration of $$C{u^{2 + }},$$ the expression for $$\Delta G$$ (in $$J\,mo{l^{ - 1}}$$) is [$$F$$ is Faraday constant; $$R$$ is gas constant; $$T$$ is temperature; $${E^0}$$ (cell)$$=1.1$$ $$V$$]

The standard state Gibbs free energies of formation of $$C$$(graphite) and $$C$$(diamond) at $$T=298$$ $$K$$ are

$${\Delta _f}{G^0}$$ [$$C$$(graphite)] $$ = 0kJmo{l^{ - 1}}$$

$${\Delta _f}{G^0}$$ [$$C$$(diamond)] $$ = 2.9kJmo{l^{ - 1}}$$

The standard state means that the pressure should be $$1$$ bar, and substance should be pure at a given temperature. The conversion of graphite [$$C$$(graphite)] to diamond [$$C$$(diamond)] reduces its volume by $$2 \times {10^{ - 6}}\,{m^3}\,mo{l^{ - 1}}$$ If $$C$$(graphite) is converted to $$C$$(diamond) isothermally at $$T=298$$ $$K,$$ the pressure at which $$C$$(graphite) is in equilibrium with $$C$$(diamond), is

[Useful information : $$1$$ $$J=1$$ $$kg\,{m^2}{s^{ - 2}};1\,Pa = 1\,kg\,{m^{ - 1}}{s^{ - 2}};$$ $$1$$ bar $$ = {10^5}$$ $$Pa$$]

The order of the oxidation state of the phosphorous atom in

$${H_3}P{O_2},{H_3}P{O_4},{H_3}P{O_3}$$ and $${H_4}{P_2}{O_6}$$ is

For a reaction taking place in a container in equilibrium with its surroundings, the effect of temperature on its equilibrium constant $$K$$ in terms of change in entropy is described by

The correct statement(s) about surface properties is (are)

In a bimolecular reaction, the steric factor $$P$$ was experimentally determined to be $$4.5.$$ The correct option(s) among the following is (are)

Among the following, the correct statement(s) is (are)

The options(s) with only amphoteric oxides is (are)

Compounds $$P$$ and $$R$$ upon ozonolysis produce $$Q$$ and $$S,$$ respectively. The molecular formula of $$Q$$ and $$S$$ is $${C_8}{H_8}O.Q$$ undergoes Canninzzaro reaction but not haloform reaction, whereas $$S$$ undergoes haloform reaction but not Cannizzaro reaction


The option(s) with suitable combination of $$P$$ and $$R,$$ respectively, is (are)

$$W$$ and $$X$$ are, respectively

The reactions, $$Q$$ to $$R$$ and $$R$$ to $$S,$$ are

The major product of the following reaction is

If f : R $$ \to $$ R is a twice differentiable function such that f"(x) > 0 for all x$$ \in $$R, and $$f\left( {{1 \over 2}} \right) = {1 \over 2}$$, f(1) = 1, then

If y = y(x) satisfies the differential equation

$${8\sqrt x \left( {\sqrt {9 + \sqrt x } } \right)dy = {{\left( {\sqrt {4 + \sqrt {9 + \sqrt x } } } \right)}^{ - 1}}}$$

dx, x > 0 and y(0) = $$\sqrt 7 $$, then y(256) =

How many 3 $$ \times $$ 3 matrices M with entries from {0, 1, 2} are there, for which the sum of the diagonal entries of M^TM is 5?

Three randomly chosen nonnegative integers x, y and z are found to satisfy the equation x + y + z = 10. Then the probability that z is even, is

Let S = {1, 2, 3, .........., 9}. For k = 1, 2, .........., 5, let N_k be the number of subsets of S, each containing five elements out of which exactly k are odd. Then N₁ + N₂ + N₃ + N₄ + N₅ =

Let O be the origin and let PQR be an arbitrary triangle. The point S is such that

$$\overrightarrow{OP}$$ . $$\overrightarrow{OQ}$$ + $$\overrightarrow{OR}$$ . $$\overrightarrow{OS}$$ = $$\overrightarrow{OR}$$ . $$\overrightarrow{OP}$$ + $$\overrightarrow{OQ}$$ . $$\overrightarrow{OS}$$ = $$\overrightarrow{OQ}$$ . $$\overrightarrow{OR}$$ + $$\overrightarrow{OP}$$ . $$\overrightarrow{OS}$$

Then the triangle PQR has S as its

The equation of the plane passing through the point (1, 1, 1) and perpendicular to the planes 2x + y $$-$$ 2z = 5 and 3x $$-$$ 6y $$-$$ 2z = 7 is

f : R $$ \to $$ R is a differentiable function such that f'(x) > 2f(x) for all x$$ \in $$R, and f(0) = 1 then

If $$I = \sum\nolimits_{k = 1}^{98} {\int_k^{k + 1} {{{k + 1} \over {x(x + 1)}}} dx} $$, then

If the line x = $$\alpha $$ divides the area of region R = {(x, y) $$ \in $$R² : x³ $$ \le $$ y $$ \le $$ x, 0 $$ \le $$ x $$ \le $$ 1} into two equal parts, then

Let $$\alpha $$ and $$\beta $$ be non zero real numbers such that $$2(\cos \beta - \cos \alpha ) + \cos \alpha \cos \beta = 1$$. Then which of the following is/are true?

Let $$f(x) = {{1 - x(1 + |1 - x|)} \over {|1 - x|}}\cos \left( {{1 \over {1 - x}}} \right)$$

for x $$ \ne $$ 1. Then

If $$g(x) = \int_{\sin x}^{\sin (2x)} {{{\sin }^{ - 1}}} (t)\,dt$$, then

If $$f(x) = \left| {\matrix{ {\cos 2x} & {\cos 2x} & {\sin 2x} \cr { - \cos x} & {\cos x} & { - \sin x} \cr {\sin x} & {\sin x} & {\cos x} \cr } } \right|$$,

then

If the triangle PQR varies, then the minimum value of cos(P + Q) + cos(Q + R) + cos(R + P) is

|$$\overrightarrow{OX}$$ $$ \times $$ $$\overrightarrow{OY}$$| = ?

a₁₂ = ?

If a₄ = 28, then p + 2q =

A point charge $$+Q$$ is placed just outside an imaginary hemispherical surface of radius $$R$$ as shown in the figure. Which of the following statements is/are correct?

Two coherent monochromatic point sources $${S_1}$$ and $${S_2}$$ of wavelength $$\lambda = 600\,nm$$ are placed symmetrically on either side of the center of the circle as shown. The sources are separated by a distance $$d=1.8$$ $$mm.$$ This arrangement produces interference fringes visible as alternate bright and dark spots on the circumference of the circle. The angular separation between two consecutive bright spots is $$\Delta \theta .$$ Which of the following options is/are correct?

The instantaneous voltages at three terminals marked $$X,Y$$ and $$Z$$ are given by

$${V_x} = {V_0}\,\sin \,\omega t,$$

$${V_Y} = {V_0}\,\sin $$ $$\left( {\omega t + {{2\pi } \over 3}} \right)$$

and $$Vz = {V_0}\sin \left( {\omega t + {{4\pi } \over 3}} \right)$$

An ideal voltmeter is configured to read $$rms$$ value of the potential difference between its terminals. It is connected between points $$X$$ and $$Y$$ and then between $$Y$$ and $$Z.$$ The reading(s) of the voltmeter will be

A uniform magnetic field $$B$$ exist in the region between $$x=0$$ and $$x = {{3R} \over 2}$$ (region $$2$$ in the figure) pointing normally into the plane of the paper. A particle with charge $$+Q$$ and momentum $$p$$ directed along $$x$$-axis enters region $$2$$ from region $$1$$$ at point $${P_1}\left( y \right) = - R).$$ Which of the following option(s) is/are correct?

A person measures the depth of a well by measuring the time interval between dropping a stone and receiving the sound of impact with the bottom of the well. The error in his measurement of time is $$\delta T = 0.01$$ seconds and he measures the depth of the well to be $$L=20$$ meters. Take the acceleration due to gravity $$g = 10m{s^{ - 2}}$$ and the velocity of sound is $$300$$ $$m{s^{ - 1}}$$. Then the fractional error in the measurement, $$\delta L/L,$$ is closest to

A rocket is launched normal to the surface of the Earth, away from the sun, along the line joining the Sun and the Earth. The Sun is $$3 \times 10{}^5$$ times heavier than the earth and is at a distance $$2.5 \times {10^4}$$ times larger than the radius of the Earth. The escape velocity from Earth's gravitational field is $${V_c} = 11.2km\,{s^{ - 1}}.$$. The minimum initial velocity $$\left( {{v_s}} \right)$$ required for the rocket to be able to leave the sun-earth system is closest to (Ignore the the rotation and revoluation of the earth and the presence of any other planet)

Three vectors $$\overrightarrow P ,\overrightarrow Q $$ and $$\overrightarrow R $$ are shown in the figure. Let $$S$$ be any point on the vector $$\overrightarrow R .$$ The distance between the points $$P$$ and $$S$$ is $$b\left| {\overrightarrow R } \right|.$$ The general relation among vectors $$\overrightarrow P ,\overrightarrow Q $$ and $$\overrightarrow S $$ is :

A symmetric star shaped conducting wire loop is carrying a steady state current $${\rm I}$$ as shown in the figure. The distance between the diametrically opposite vertices of the star is $$4a.$$ The magnitude of the magnetic field at the center of the loop is

A photoelectric material having work-function $${\phi _0}$$ is illuminated with light of wavelength $$\lambda \left( {\lambda < {{he} \over {{\phi _0}}}} \right).$$ The fastest photoelectron has a de-Broglic wavelength $${\lambda _d}.$$ A change in wavelength of the incident light by $$\Delta \lambda $$ result in a change $$\Delta {\lambda _d}$$ in $${\lambda _d}.$$ Then the ratio $$\Delta {\lambda _d}/\Delta \lambda $$ is proportional to

Consider regular polygons with number of sides $$n=3,4,5....$$ as shown in the figure. The center of mass of all the polygons is at height $$h$$ from the ground. They roll on a horizontal surface about the leading vertex without slipping and sliding as depicted. The maximum increase in height of the locus of the center of mass for each polygon is $$\Delta $$. Then $$\Delta $$ depends on $$n$$ and $$h$$ as

Consider an expanding sphere of instantaneous radius R whose total mass remains constant. The expansion is such that the instantaneous density $$\rho $$ remains uniform throughout the volume. The rate of fractional change in density $$\left( {{1 \over \rho } {{d\rho } \over {dt}}} \right)$$ is constant. The velocity $$v$$ of any point on the surface of the expanding sphere is proportional to

A wheel of radius R and mass M is placed at the bottom of a fixed step of height R as shown in the figure. A constant force is continuously applied on the surface of the wheel so that it just climbs the step without slipping. Consider the torque $$\tau$$ about an axis normal to the plane of the paper passing through the point Q. Which of the following options is/are correct?

A rigid uniform bar AB of length L is slipping from its vertical position on a frictionless floor (as shown in the figure). At some instant of time, the angle made by the bar with the vertical is $$\theta$$. Which of the following statements about its motion is/are correct?

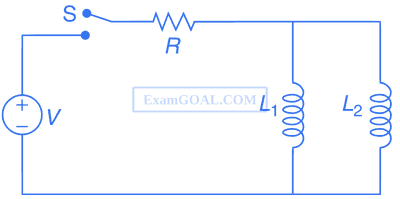
A source of constant voltage V is connected to a resistance R and two ideal inductors L₁ and L₂ through a switch S as shown. There is no mutual inductance between the two inductors. The switch S is initially open. At t = 0, the switch is closed and current begins to flow. Which of the following options is/are correct?

In Process 1, the energy stored in the capacitor E_C and heat dissipated across resistance E_D are related by

In Process 2, total energy dissipated across the resistance E_D is

The total kinetic energy of the ring is

The minimum value of $$\omega$$₀ below which the ring will drop down is