Chemistry
| Column - I | Column - II |
|---|---|
| (A) Carbonate | (p) Siderite |
| (B) Sulphide | (q) Malachite |
| (C) Hydroxide | (r) Bauxite |
| (D) Oxide | (s) Calamine |
| (t) Argentite |
$$X \to Y, \Delta _tG^o $$ = -193 kJ mol-1 is used for oxidizing M+ as M+ $$\to$$ M3+ + 2e-, Eo = -0.25 V
Under standard conditions, the number of moles of M+ oxidized when one mole of X is converted to Y is [F = 96500 C mol–1]
Column I
(A) Freezing water at 273 K and 1 atm
(B) Expansion of 1 mol of an ideal gas into a vacuum under isolated conditions.
(C) Mixing of equal volumes of two ideal gases at constant temperature and pressure in an isolated container.
(D) Reversible heating of H2(g) at 1 atm from 300K to 600K, followed by reversible cooling to 300K at 1 atm
Column II
(p) q = 0
(q) w = 0
(r) $$\Delta S_{sys}$$ < 0
(s) $$\Delta U$$ = 0
(t) $$\Delta G$$ = 0
[Atomic number: S = 16, Cl = 17, I = 53 and Xe = 54]
The total number of stereoisomers that can exist for M is ___________.

The number of resonance structure for N is _________.

The number of lone pairs of electrons in N2O3 is ___________.
For the octahedral complexes of Fe3+ in SCN$$-$$ (thiocyana-to-S) and in CN$$-$$ ligand environments, the difference between the spin-only magnetic moments in Bohr magnetons (when approximated to the nearest integer) is __________.
[Atomic number Fe = 26]
Compound(s) that on hydrogenation produce(s) optically inactive compound(s) is(are)
The major product of the following reaction is

In the following reaction, the major product is

The structure of D-(+)-glucose is

The structure of L-($$-$$)-glucose is
The major product of the reaction is

The correct statements about Cr2+ and Mn3+ is(are) (Atomic numbers of Cr = 24 and Mn = 25)
Fe3+ is reduced to Fe2+ by using
The % yield of ammonia as a function of time in the reaction
N2(g) + 3H2(g) $$\rightleftharpoons$$ 2NH3(g), $$\Delta$$H < 0 at (P, T1) is given below:

If this reactions is conducted at (P, T2), with T2 > T1, the % yield of ammonia as a function of time is represented by
Mathematics
$${5 \over 4}{\cos ^2}\,2x + {\cos ^4}\,x + {\sin ^4}\,x + {\cos ^6}\,x + {\sin ^6}\,x\, = \,2$$
in the interval $$\left[ {0,\,2\pi } \right]$$ is
| Column I | Column I | ||
|---|---|---|---|
| (A) | $\begin{array}{l}\text { In a triangle } \Delta X Y Z \text {, let } a, b \text { and } c \text { be the lengths of the sides } \\\text { opposite to the angles } X, Y \text { and } Z \text {, respectively. If } 2\left(a^2-b^2\right)=c^2 \\\text { and } \lambda=\frac{\sin (X-Y)}{\sin Z} \text {, then possible values of } n \text { for which } \cos (n \lambda) \\=0 \text { is (are) }\end{array}$ | (P) | 1 |
| (B) | $\begin{array}{l}\text { In a triangle } \triangle X Y Z \text {, let } a, b \text { and } c \text { be the lengths of the sides } \\\text { opposite to the angles } X, Y \text { and } Z \text {, respectively. If } 1+\cos 2 X-2 \\\cos 2 Y=2 \sin X \sin Y \text {, then possible value(s) of } \frac{a}{b} \text { is (are) }\end{array}$ | (Q) | 2 |
| (C) | $\begin{array}{l}\text { In } \mathbb{R}^2 \text {, let } \sqrt{3} \hat{i}+\hat{j}, \hat{i}+\sqrt{3} \hat{j} \text { and } \beta \hat{i}+(1-\beta) \hat{j} \text { be the position } \\\text { vectors of } X, Y \text { and } Z \text { with respect of the origin } \mathrm{O} \text {, respectively. If } \\\text { the distance of } \mathrm{Z} \text { from the bisector of the acute angle of } \overrightarrow{\mathrm{OX}} \text { with } \\\overrightarrow{\mathrm{OY}} \text { is } \frac{3}{\sqrt{2}} \text {, then possible value(s) of }|\beta| \text { is (are) }\end{array}$ | (R) | 3 |
| (D) | $\begin{array}{l}\text { Suppose that } F(\alpha) \text { denotes the area of the region bounded by } \\x=0, x=2, y^2=4 x \text { and } y=|\alpha x-1|+|\alpha x-2|+\alpha x \text {, } \\\text { where, } \alpha \in\{0,1\} \text {. Then the value(s) of } F(\alpha)+\frac{8}{2} \sqrt{2} \text {, when } \alpha=0 \\\text { and } \alpha=1 \text {, is (are) }\end{array}$ | (S) | 5 |
| (T) | 6 |
$$\,\,\,\,$$ $$\,\,\,\,$$ $$\,\,\,\,$$ Column $$I$$
(A)$$\,\,\,\,$$ In $${R^2},$$ If the magnitude of the projection vector of the vector $$\alpha \widehat i + \beta \widehat j$$ on $$\sqrt 3 \widehat i + \widehat j$$ and If $$\alpha = 2 + \sqrt 3 \beta ,$$ then possible value of $$\left| \alpha \right|$$ is/are
(B)$$\,\,\,\,$$ Let $$a$$ and $$b$$ be real numbers such that the function $$f\left( x \right) = \left\{ {\matrix{
{ - 3a{x^2} - 2,} & {x < 1} \cr
{bx + {a^2},} & {x \ge 1} \cr
} } \right.$$ if differentiable for all $$x \in R$$. Then possible value of $$a$$ is (are)
(C)$$\,\,\,\,$$ Let $$\omega \ne 1$$ be a complex cube root of unity. If $${\left( {3 - 3\omega + 2{\omega ^2}} \right)^{4n + 3}} + {\left( {2 + 3\omega - 3{\omega ^2}} \right)^{4n + 3}} + {\left( { - 3 + 2\omega + 3{\omega ^2}} \right)^{4n + 3}} = 0,$$ then possible value (s) of $$n$$ is (are)
(D)$$\,\,\,\,$$ Let the harmonic mean of two positive real numbers $$a$$ and $$b$$ be $$4.$$ If $$q$$ is a positive real nimber such that $$a, 5, q, b$$ is an arithmetic progression, then the value(s) of $$\left| {q - a} \right|$$ is (are)
$$\,\,\,\,$$ $$\,\,\,\,$$ $$\,\,\,\,$$ Column $$II$$
(p)$$\,\,\,\,$$ $$1$$
(q)$$\,\,\,\,$$ $$2$$
(r)$$\,\,\,\,$$ $$3$$
(s)$$\,\,\,\,$$ $$4$$
(t)$$\,\,\,\,$$ $$5$$
$$f\left( x \right) = \left\{ {\matrix{ {\left[ x \right],} & {x \le 2} \cr {0,} & {x > 2} \cr } } \right.$$ where $$\left[ x \right]$$ is the greatest integer less than or equal to $$x$$, if $$I = \int\limits_{ - 1}^2 {{{xf\left( {{x^2}} \right)} \over {2 + f\left( {x + 1} \right)}}dx,} $$ then the value of $$(4I-1)$$ is
$$\left( {1 + {e^x}} \right)y' + y{e^x} = 1.$$
If $$y(0)=2$$, then which of the following statement is (are) true?
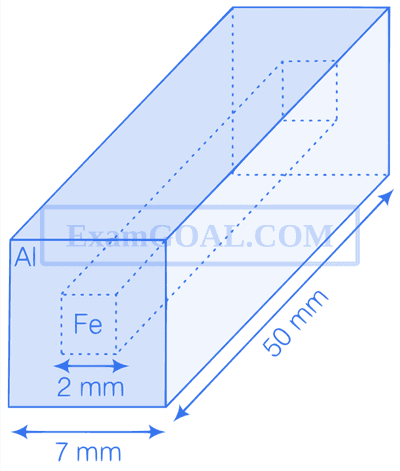
If the volume of the material used to make the container is minimum when the inner radius of the container is $$10 $$ mm,
then the value of $${V \over {250\pi }}$$ is
Let X and Y be two arbitrary, 3 $$\times$$ 3, non-zero, skew-symmetric matrices and Z be an arbitrary 3 $$\times$$ 3, non-zero, symmetric matrix. Then which of the following matrices is(are) skew symmetric?
Which of the following values of $$\alpha$$ satisfy the equation
$$\left| {\matrix{ {{{(1 - \alpha )}^2}} & {{{(1 + 2\alpha )}^2}} & {{{(1 + 3\alpha )}^2}} \cr {{{(2 + \alpha )}^2}} & {{{(2 + 2\alpha )}^2}} & {{{(2 + 3\alpha )}^2}} \cr {{{(3 + \alpha )}^2}} & {{{(3 + 2\alpha )}^2}} & {{{(3 + 3\alpha )}^2}} \cr } } \right| = - 648\alpha $$ ?
Let $$g:R \to R$$ be a differentiable function with $$g(0) = 0$$, $$g'(0) = 0$$ and $$g'(1) \ne 0$$. Let
$$f(x) = \left\{ {\matrix{ {{x \over {|x|}}g(x),} & {x \ne 0} \cr {0,} & {x = 0} \cr } } \right.$$
and $$h(x) = {e^{|x|}}$$ for all $$x \in R$$. Let $$(f\, \circ \,h)(x)$$ denote $$f(h(x))$$ and $$(h\, \circ \,f)(x)$$ denote $$f(f(x))$$. Then which of the following is (are) true?
Let $$f(x) = \sin \left( {{\pi \over 6}\sin \left( {{\pi \over 2}\sin x} \right)} \right)$$ for all $$x \in R$$ and g(x) = $${{\pi \over 2}\sin x}$$ for all x$$\in$$R. Let $$(f \circ g)(x)$$ denote f(g(x)) and $$(g \circ f)(x)$$ denote g(f(x)). Then which of the following is/are true?
Physics
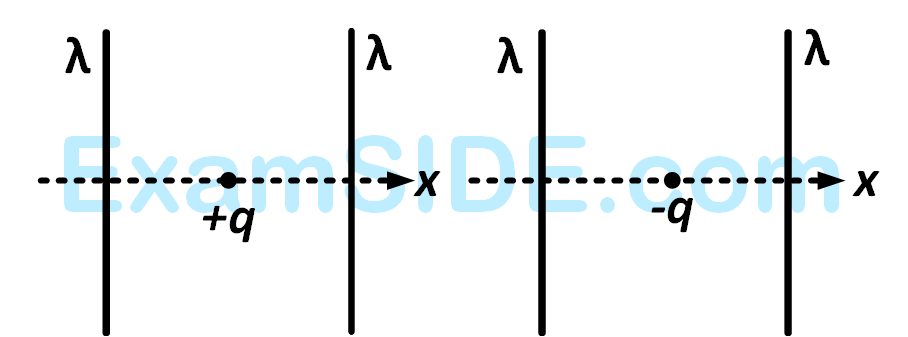

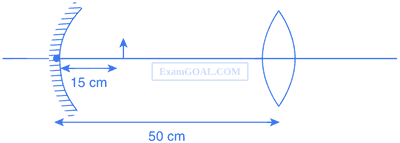

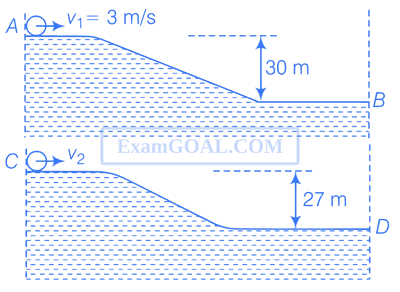
A nuclear power plant supplying electrical power to a village uses a radioactive material of half life T years as the fuel.
The amount of fuel at the beginning is such that the total power requirement of the village is 12.5 % of the electrical power available from the plant at that time. If the plant is able to meet the total power needs of the village for a maximum period of nT years, then the value of n is


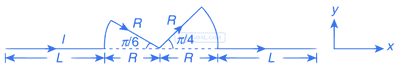
Match the nuclear processes given in Column I with the appropriate option(s) in Column II:

A particle of unit mass is moving along the x-axis under the influence of a force and its total energy is conserved. Four possible forms of the potential energy of the particle are given in Column I (a and U0 are constants). Match the potential energies in Column I to the corresponding statement(s) in Column II:
