According to Bohr's theory
E_n = Total energy, K_n = Kinetic Energy, V_n = Potential Energy, r_n = Radius of n^th orbit
Match the following

Column I

(A) V_n/K_n = ?
(B) If radius of n^th orbit $$ \propto E_n^x,x = ?$$
(C) Angular momentum in lowest orbital
(D) $${1 \over {{r_n}}} \propto {Z^y},y = ?$$

Column II

(p) 0
(q) -1
(r) -2
(s) 1

The species present in solution when CO₂ is dissolved in water are

MgSO₄ on reaction with NH₄OH and Na₂HPO₄ forms a white crystalline precipitate. What is its formula?

75.2 g of C₆H₅OH (phenol) is dissolved in a solvent of K_f = 14. If the depression in freezing point is 7 K then find the % of phenol that dimerises.

We have taken a saturated solution of AgBr. K_sp of AgBr is 12 $$\times$$ 10^-14. If 10^-7 mole of AgNO₃ are added to 1 litre of this solution find conductivity (specific conductance) of this solution in terms of 10^-7 S m^-1 units. Given, molar conductance of Ag⁺, Br^- and $$NO_3^-$$ are 6 $$\times$$ 10^-3 Sm² mol^-1, 8 $$\times$$ 10^-3 Sm² mol^-1 and 7 $$\times$$ 10^-3 Sm² mol^-1

A plane which is perpendicular to two planes $$2x - 2y + z = 0$$ and $$x - y + 2z = 4,$$ passes through $$(1, -2, 1).$$ The distance of the plane from the point $$(1, 2, 2)$$ is

Match the following :

Column $$I$$
(A) $$\int\limits_0^{\pi /2} {{{\left( {\sin x} \right)}^{\cos x}}\left( {\cos x\cot x - \log {{\left( {\sin x} \right)}^{\sin x}}} \right)dx} $$
(B) Area bounded by $$ - 4{y^2} = x$$ and $$x - 1 = - 5{y^2}$$
(C) Cosine of the angle of intersection of curves $$y = {3^{x - 1}}\log x$$ and $$y = {x^x} - 1$$ is
(D) Let $${{dy} \over {dx}} = {6 \over {x + y}}$$ where $$y(0)=0$$ then value of $$y$$ when $$x+y=6$$ is

Column $$II$$
(p) $$1$$
(q) $$0$$
(r) $$6\ln 2$$
(s) $${4 \over 3}$$

Let the definite integral be defined by the formula
$$\int\limits_a^b {f\left( x \right)dx = {{b - a} \over 2}\left( {f\left( a \right) + f\left( b \right)} \right).} $$ For more accurate result for
$$c \in \left( {a,b} \right),$$ we can use $$\int\limits_a^b {f\left( x \right)dx = \int\limits_a^c {f\left( x \right)dx + \int\limits_c^b {f\left( x \right)dx = F\left( c \right)} } } $$ so
that for $$c = {{a + b} \over 2},$$ we get $$\int\limits_a^b {f\left( x \right)dx = {{b - a} \over 4}\left( {f\left( a \right) + f\left( b \right) + 2f\left( c \right)} \right).} $$

$$\int\limits_0^{\pi /2} {\sin x\,dx = } $$

Let the definite integral be defined by the formula
$$\int\limits_a^b {f\left( x \right)dx = {{b - a} \over 2}\left( {f\left( a \right) + f\left( b \right)} \right).} $$ For more accurate result for
$$c \in \left( {a,b} \right),$$ we can use $$\int\limits_a^b {f\left( x \right)dx = \int\limits_a^c {f\left( x \right)dx + \int\limits_c^b {f\left( x \right)dx = F\left( c \right)} } } $$ so
that for $$c = {{a + b} \over 2},$$ we get $$\int\limits_a^b {f\left( x \right)dx = {{b - a} \over 4}\left( {f\left( a \right) + f\left( b \right) + 2f\left( c \right)} \right).} $$

If $$\mathop {\lim }\limits_{x \to a} {{\int\limits_a^x {f\left( x \right)dx - \left( {{{x - a} \over 2}} \right)\left( {f\left( x \right) + f\left( a \right)} \right)} } \over {{{\left( {x - a} \right)}^3}}} = 0,\,\,$$ then $$f(x)$$ is
of maximum degree

Let the definite integral be defined by the formula
$$\int\limits_a^b {f\left( x \right)dx = {{b - a} \over 2}\left( {f\left( a \right) + f\left( b \right)} \right).} $$ For more accurate result for
$$c \in \left( {a,b} \right),$$ we can use $$\int\limits_a^b {f\left( x \right)dx = \int\limits_a^c {f\left( x \right)dx + \int\limits_c^b {f\left( x \right)dx = F\left( c \right)} } } $$ so
that for $$c = {{a + b} \over 2},$$ we get $$\int\limits_a^b {f\left( x \right)dx = {{b - a} \over 4}\left( {f\left( a \right) + f\left( b \right) + 2f\left( c \right)} \right).} $$

If $$f''\left( x \right) < 0\,\forall x \in \left( {a,b} \right)$$ and $$c$$ is a point such that $$a < c < b,$$ and
$$\left( {c,f\left( c \right)} \right)$$ is the point lying on the curve for which $$F(c)$$ is
maximum, then $$f'(c)$$ is equal to

A curve $$y=f(x)$$ passes through $$(1,1)$$ and at $$P(x,y),$$ tangent cuts the $$x$$-axis and $$y$$-axis at $$A$$ and $$B$$ respectively such that $$BP:AP=3:1,$$ then

There are $$n$$ urns, each of these contain $$n+1$$ balls. The ith urn contains $$i$$ white balls and $$(n+1-i)$$ red balls. Let $${u_i}$$ be the event of selecting ith urn, $$i=1,2,3........,n$$ and $$w$$ the event of getting a white ball.

If $$P\left( {{u_i}} \right) \propto i,\,$$ where $$i=1,2,3,.......,n,$$ then $$\mathop {\lim }\limits_{n \to \infty } P\left( w \right) = $$

There are $$n$$ urns, each of these contain $$n+1$$ balls. The ith urn contains $$i$$ white balls and $$(n+1-i)$$ red balls. Let $${u_i}$$ be the event of selecting ith urn, $$i=1,2,3........,n$$ and $$w$$ the event of getting a white ball.

Let $$P\left( {{u_i}} \right) = {1 \over n},$$ if $$n$$ is even and $$E$$ denotes the event of choosing even numbered urn, then the value of $$P\left( {w/E} \right)$$ is

There are $$n$$ urns, each of these contain $$n+1$$ balls. The ith urn contains $$i$$ white balls and $$(n+1-i)$$ red balls. Let $${u_i}$$ be the event of selecting ith urn, $$i=1,2,3........,n$$ and $$w$$ the event of getting a white ball.

If $$P\left( {{u_i}} \right) = c,$$ (a constant) then $$P\left( {{u_n}/w} \right) = $$

Let $$\overrightarrow a = \widehat i + 2\widehat j + \widehat k,\,\overrightarrow b = \widehat i - \widehat j + \widehat k$$ and $$\overrightarrow c = \widehat i + \widehat j - \widehat k.$$ A vector in the plane of $$\overrightarrow a $$ and $$\overrightarrow b $$ whose projection on $$\overrightarrow c $$ is $${1 \over {\sqrt 3 }},$$ is

Let $$\theta \in \left( {0,{\pi \over 4}} \right)$$ and $${t_1} = {\left( {\tan \theta } \right)^{\tan \theta }},\,\,\,\,{t_2} = \,\,{\left( {\tan \theta } \right)^{\cot \theta }}$$, $${t_3}\, = \,\,{\left( {\cot \theta } \right)^{\tan \theta }}$$ and $${t_4}\, = \,\,{\left( {\cot \theta } \right)^{\cot \theta }},$$then

Let $${\overrightarrow A }$$ be vector parallel to line of intersection of planes $${P_1}$$ and $${P_2}.$$ Planes $${P_1}$$ is parallel to the vectors $$2\widehat j + 3\widehat k$$ and $$4\widehat j - 3\widehat k$$ and that $${P_2}$$ is parallel to $$\widehat j - \widehat k$$ and $$3\widehat i + 3\widehat j,$$ then the angle between vector $${\overrightarrow A }$$ and a given vector $$2\widehat i + \widehat j - 2\widehat k$$ is

Match the folowing :
(A)$$\,\,\,$$Two rays $$x + y = \left| a \right|$$ and $$ax - y=1$$ intersects each other in the
$$\,\,\,\,\,\,\,\,\,\,$$first quadrant in interval $$a \in \left( {{a_0},\,\,\infty } \right),$$ the value of $${{a_0}}$$ is
(B)$$\,\,\,$$ Point $$\left( {\alpha ,\beta ,\gamma } \right)$$ lies on the plane $$x+y+z=2.$$
$$\,\,\,\,\,\,\,\,\,\,\,$$Let $$\overrightarrow a = \alpha \widehat i + \beta \widehat j + \gamma \widehat k,\widehat k \times \left( {\widehat k \times \overrightarrow a } \right) = 0,$$ then $$\gamma = $$
(C)$$\,\,\,$$$$\left| {\int\limits_0^1 {\left( {1 - {y^2}} \right)dy} } \right| + \left| {\int\limits_1^0 {\left( {{y^2} - 1} \right)dy} } \right|$$
(D)$$\,\,\,$$If $$\sin A\,\,\sin B\,\,\sin C + \cos A\,\,\cos B = 1,$$ then the value of $$\sin C = $$

(p)$$\,\,\,$$ $$2$$
(q)$$\,\,\,$$ $${4 \over 3}$$
(r)$$\,\,\,$$ $$\left| {\int\limits_0^1 {\sqrt {1 - xdx} } } \right| + \left| {\int\limits_{ - 1}^0 {\sqrt {1 + xdx} } } \right|$$
(s)$$\,\,\,$$ $$1$$

$$\int {{{{x^2} - 1} \over {{x^3}\sqrt {2{x^4} - 2{x^2} + 1} }}dx = } $$

If $${{w - \overline w z} \over {1 - z}}$$ is purely real where $$w = \alpha + i\beta ,$$ $$\beta \ne 0$$ and $$z \ne 1,$$ then the set of the values of z is

Let $$a,\,b,\,c$$ be the sides of triangle where $$a \ne b \ne c$$ and $$\lambda \in R$$.
If the roots of the equation $${x^2} + 2\left( {a + b + c} \right)x + 3\lambda \left( {ab + bc + ca} \right) = 0$$ are real, then

Let $$a$$ and $$b$$ be the roots of the equation $${x^2} - 10cx - 11d = 0$$ and those $${x^2} - 10ax - 11b = 0$$ are $$c$$, $$d$$ then the value of $$a + b + c + d,$$ when $$a \ne b \ne c \ne d,$$ is

If $${a_n} = {3 \over 4} - {\left( {{3 \over 4}} \right)^2} + {\left( {{3 \over 4}} \right)^3} + ....{( - 1)^{n - 1}}{\left( {{3 \over 4}} \right)^n}\,\,and\,\,{b_n} = 1 - {a_n},$$, then find the least natural number $${n_0}$$ such that $${b_n}\,\, > \,\,{a_n}\,\forall \,n\,\, \ge \,\,{n_0}$$.

ABCD is a square of side length 2 units. $$C_1$$ is the circle touching all the sides of the square ABCD and $$C_2$$ is the circumcircle of square ABCD. L is a fixed line in the same plane and R is a fixed point.

If a circle is such that it touches the line L and the circle $$C_1$$ externally, such that both the circles are on the same side of the line, then the locus of centre of the circle is

ABCD is a square of side length 2 units. $$C_1$$ is the circle touching all the sides of the square ABCD and $$C_2$$ is the circumcircle of square ABCD. L is a fixed line in the same plane and R is a fixed point.

A line L' through A is drawn parallel to BD. Point S moves such that its distances from the BD and the vertex A are equal. If locus of S cuts L' at $$T_2$$ and $$T_3$$ and AC at $$T_1$$, then area of $$\Delta \,{T_1}\,{T_2}\,{T_3}$$ is

ABCD is a square of side length 2 units. $${C_1}$$ is the circle touching all the sides of the square ABCD and $${C_2}$$ is the circumcircle of square ABCD. L is a fixed line in the same plane and R is a fixed point.

If P is any point of $${C_1}$$ and Q is another point on $${C_2}$$, then

$${{P{A^2}\, + \,P{B^2}\, + P{C^2}\, + P{D^2}} \over {Q{A^2} + \,Q{B^2}\, + Q{C^2}\, + Q{D^2}}}$$ is equal to

The axis of a parabola is along the line $$y = x$$ and the distances of its vertex and focus from origin are $$\sqrt 2 $$ and $$2\sqrt 2 $$ respectively. If vertex and focus both lie in the first quadrant, then the equation of the parabola is

Let a hyperbola passes through the focus of the ellipse $${{{x^2}} \over {25}} + {{{y^2}} \over {16}} = 1$$. The transverse and conjugate axes of this hyperbola coincide with the major and minor axes of the given ellipse, also the produced of eccentricities of given ellipse and hyperbola is $$1$$, then

The equations of the common tangents to the parabola $$y = {x^2}$$ and $$y = - {\left( {x - 2} \right)^2}$$ is/are

Match the following : $$(3, 0)$$ is the pt. from which three normals are drawn to the parabola $${y^2} = 4x$$ which meet the parabola in the points $$P, Q $$ and $$R$$. Then

Column $${\rm I}$$
(A) Area of $$\Delta PQR$$
(B) Radius of circumcircle of $$\Delta PQR$$
(C) Centroid of $$\Delta PQR$$
(D) Circumcentre of $$\Delta PQR$$

Column $${\rm I}$$$${\rm I}$$
(p) $$2$$
(q) $$5/2$$
(r) $$(5/2, 0)$$
(s) $$(2/3, 0)$$

One angle of an isosceles $$\Delta $$ is $${120^ \circ }$$ and radius of its incircle $$ = \sqrt 3 $$. Then the area of the triangle in sq. units is

In $$\Delta ABC$$, internal angle bisector of $$\angle A$$ meets side $$BC$$ in $$D$$. $$DE \bot AD$$ meets $$AC$$ in $$E$$ and $$AB$$ in $$F$$. Then

Match the following

Column $$I$$

(A) $$\sum\limits_{i = 1}^\infty {{{\tan }^{ - 1}}\left( {{1 \over {2{i^2}}}} \right) = t,} $$ then tan $$t=$$

(B) Sides $$a, b, c$$ of a triangle $$ABC$$ are in $$AP$$ and
$$\cos {\theta _1} = {a \over {b + c}},\,\cos {\theta _2} = {b \over {a + c}},\cos {\theta _3} = {c \over {a + b}},$$
then $${\tan ^2}\left( {{{{\theta _1}} \over 2}} \right) + {\tan ^2}\left( {{{{\theta _3}} \over 2}} \right) = $$

(C) A line is perpendicular to $$x + 2y + 2z = 0$$ and
passes through $$(0, 1, 0)$$. The perpendicular distance of this line from the origin is

Column $$II$$

(p) $$1$$

(q) $${{\sqrt 5 } \over 3}$$

(r) $${2 \over 3}$$

$$f(x)$$ is cubic polynomial with $$f(2)=18$$ and $$f(1)=-1$$. Also $$f(x)$$ has local maxima at $$x=-1$$ and $$f'(x)$$ has local minima at $$x=0$$, then

Let $$f\left( x \right) = \left\{ {\matrix{ {{e^x},} & {0 \le x \le 1} \cr {2 - {e^{x - 1}},} & {1 < x \le 2} \cr {x - e,} & {2 < x \le 3} \cr } } \right.$$ and $$g\left( x \right) = \int\limits_0^x {f\left( t \right)dt,x \in \left[ {1,3} \right]} $$
then $$g(x)$$ has

For a twice differentiable function $$f(x),g(x)$$ is defined as $$4\sqrt {65} g\left( x \right) = \left( {f'{{\left( x \right)}^2} + f''\left( x \right)} \right)\,\,f\left( x \right)$$ on $$\,\,\,\left[ {a,\,\,\,e} \right].$$ If for $$a < b < c < d < e,\,f\left( a \right) = 0,f\left( b \right) = 2,f\left( c \right) = - 1,f\left( d \right) = 2,f\left( e \right) = 0$$ then find the minimum number of zeros of $$g(x)$$.

The value of $$5050{{\int\limits_0^1 {{{\left( {1 - {x^{50}}} \right)}^{100}}} dx} \over {\int\limits_0^1 {{{\left( {1 - {x^{50}}} \right)}^{101}}} dx}}$$ is.

A student performs an experiment for determination of $$\mathrm g\left(=\frac{4\mathrm\pi^2\mathcal l}{\mathrm T^2}\right)$$. The error in length $$\mathcal l$$ is $$\triangle\mathcal l$$ and in the time T is $$\triangle\mathrm T$$ and n is number of times the reading is taken.The reading of g is most accurate for

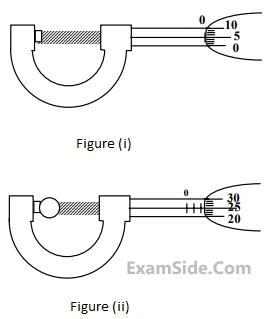
In a screw gauge, the zero of main scale coincides with the fifth division of circular scale in figure (i).The circular division of screw gauge is 50. It moves 0.5 mm on main scale in one rotation.The diameter of the ball in figure (ii) is

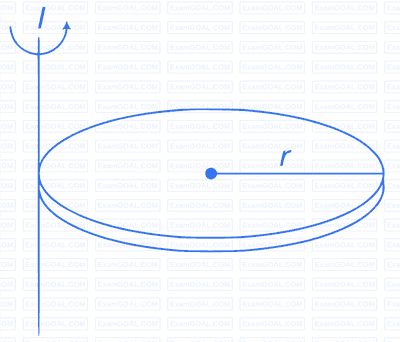
A solid sphere of radius $R$ has moment of inertia $I$ about its geometrical axis. If it is melted into a disc of radius $r$ and thickness $t$. If its moment of inertia about the tangential axis (which is perpendicular to plane of the disc), is also equal to $I$, then the value of $r$ is equal to