JEE Advanced 2018 Paper 1 Offline
Paper was held on
Sat, May 19, 2018 9:00 PM
Chemistry
1
The correct statement(s) regarding the binary transition metal carbonyl compounds is(are) (Atomic numbers : $$Fe = 26,Ni = 28$$)
2
A reversible cyclic process for an ideal gas is shown below. Here, $$P, V,$$ and $$T$$ are pressure, volume and temperature, respectively. The thermodynamic parameters $$q,w, H$$ and $$U$$ are heat, work, enthalpy and internal energy, respectively.

The correct option(s) is (are)

The correct option(s) is (are)
3
The compounds(s) which generate(s) $${N_2}$$ gas upon thermal decomposition below $${300^ \circ }C$$ is (are)
4
Consider an ionic solid $$MX$$ with $$NaCl$$ structure. Construct a new structure $$(Z)$$ whose unit cell is constructed from the unit cell of $$MX$$ following the sequential instructions given below. Neglect the charge balance.
$$(i)\,\,\,\,\,$$ Remove all the anions $$(X)$$ except the central one
$$(ii)\,\,\,\,$$ Replace all the face centered cations $$(M)$$ by anions $$(X)$$
$$(iii)\,\,$$ Remove all the corner cations $$(M)$$
$$(iv)\,\,\,\,$$ Replace the central anion $$(X)$$ with cation $$(M)$$
The value of $$\,\,\left( {{{number\,\,of\,\,anions} \over {number\,\,of\,\,cations}}} \right)\,\,$$ in $$Z$$ is ___________.
$$(i)\,\,\,\,\,$$ Remove all the anions $$(X)$$ except the central one
$$(ii)\,\,\,\,$$ Replace all the face centered cations $$(M)$$ by anions $$(X)$$
$$(iii)\,\,$$ Remove all the corner cations $$(M)$$
$$(iv)\,\,\,\,$$ Replace the central anion $$(X)$$ with cation $$(M)$$
The value of $$\,\,\left( {{{number\,\,of\,\,anions} \over {number\,\,of\,\,cations}}} \right)\,\,$$ in $$Z$$ is ___________.
5
Among the species given below, the total number of diamagnetic species is _____________.
$$H$$ atom, $$N{O_2}$$ monomer, $${O_2}^ - $$ (superoxide), dimeric sulphur in vapor phase, $$M{n_3}{O_4},\,\,$$ $${\left( {N{H_4}} \right)_2}\left[ {FeC{l_4}} \right],$$ $${\left( {N{H_4}} \right)_2}\left[ {NiC{l_4}} \right],\,$$ $$\,{K_2}Mn{O_4},\,$$$${K_2}Cr{O_4}$$
$$H$$ atom, $$N{O_2}$$ monomer, $${O_2}^ - $$ (superoxide), dimeric sulphur in vapor phase, $$M{n_3}{O_4},\,\,$$ $${\left( {N{H_4}} \right)_2}\left[ {FeC{l_4}} \right],$$ $${\left( {N{H_4}} \right)_2}\left[ {NiC{l_4}} \right],\,$$ $$\,{K_2}Mn{O_4},\,$$$${K_2}Cr{O_4}$$
6
The ammonia prepared by treating ammonium sulphate with calcium hydroxide is completely used by $$NiCl{}_2.6{H_2}O$$ to form a stable coordination compound. Assume that both the reactions are $$100\% $$ complete. If $$1584$$ $$g$$ of ammonium sulphate and $$952\,g$$ of $$NiC{l_2}.6{H_2}O$$ are used in the preparation, the combined weight (in grams) of gypsum and the nickel-ammonia coordination compound thus produced is _____________.
(Atomic weights in $$g$$ $$mo{l^{ - 1}}:$$ $$H = 1,N = 14,O = 16,$$ $$S = 32,Cl = 35.5,$$ $$Ca = 40,$$ $$Ni = 59$$
(Atomic weights in $$g$$ $$mo{l^{ - 1}}:$$ $$H = 1,N = 14,O = 16,$$ $$S = 32,Cl = 35.5,$$ $$Ca = 40,$$ $$Ni = 59$$
7
For the electrochemical cell,
$$\left. {Mg\left( s \right)} \right|M{g^{2 + }}\left( {aq,1\,M} \right)\left\| {C{u^{2 + }}} \right.\left( {aq,1M} \right)\left| {Cu\left( s \right)} \right.$$
the standard $$emf$$ of the cell is $$2.70$$ $$V$$ at $$300$$ $$K.$$ When the concentration of $$M{g^{2 + }}$$ is changed to $$x$$ $$M,$$ the cell potential changes to $$2.67$$ $$V$$ at $$300$$ $$K.$$ The value of $$x$$ is ___________.
(given, $${F \over R} = 11500\,K{V^{ - 1}},$$ where $$F$$ is the Faraday constant and $$R$$ is the gas constant, In $$(10=2.30)$$
$$\left. {Mg\left( s \right)} \right|M{g^{2 + }}\left( {aq,1\,M} \right)\left\| {C{u^{2 + }}} \right.\left( {aq,1M} \right)\left| {Cu\left( s \right)} \right.$$
the standard $$emf$$ of the cell is $$2.70$$ $$V$$ at $$300$$ $$K.$$ When the concentration of $$M{g^{2 + }}$$ is changed to $$x$$ $$M,$$ the cell potential changes to $$2.67$$ $$V$$ at $$300$$ $$K.$$ The value of $$x$$ is ___________.
(given, $${F \over R} = 11500\,K{V^{ - 1}},$$ where $$F$$ is the Faraday constant and $$R$$ is the gas constant, In $$(10=2.30)$$
8
Based on the compounds of group $$15$$ elements, the correct statement(s) is (are)
9
The reaction (s) leading to the formation of $$1,3,5$$-trimethylbenzene is (are)
10
In the following reaction sequence, the correct structure(s) of $$X$$ is (are)


11
Liquids A and B form ideal solution over the entire range of composition. At temperature $$T,$$ equimolar binary solution of liquids $$A$$ and $$B$$ has vapor pressure $$45$$ $$Torr.$$ At the same temperature, a new solution of $$A$$ and $$B$$ having mole fractions $${X_A}$$ and $${X_B}$$, respectively, has vapour pressure of $$22.5$$ $$Torr.$$ The value of $${x_A}/{x_B}$$ in the new solution is ___________.
(given that the vapor pressure of pure liquid $$A$$ is $$20$$ $$Torr$$ at temperature $$T$$)
(given that the vapor pressure of pure liquid $$A$$ is $$20$$ $$Torr$$ at temperature $$T$$)
12
The solubility of a salt of weak acid $$(AB)$$ at $$pH\,$$ $$3$$ is $$Y \times {10^{ - 3}}$$ $$mol\,{L^{ - 1}}.$$ The value of $$Y$$ is ________________.
(Given that the value of solubility product of $$AB$$ $$\left( {{K_{sp}}} \right) = 2 \times {10^{ - 10}}$$ and the value of ionization constant of $$HB$$ $$\left( {{K_a}} \right) = 1 \times {10^{ - 8}}$$)
(Given that the value of solubility product of $$AB$$ $$\left( {{K_{sp}}} \right) = 2 \times {10^{ - 10}}$$ and the value of ionization constant of $$HB$$ $$\left( {{K_a}} \right) = 1 \times {10^{ - 8}}$$)
13
A closed tank has two compartments $$A$$ and $$B,$$ both filled with oxygen (assumed to be ideal gas). The partition separating the two compartments is fixed and is a perfect heat insulator (Figure $$1.$$). If the old partition is replaced by a new partition which can slide and conduct heat but does NOT allow the gas to leak across (Figure $$2$$), the volume (in $${m^3}$$) of the compartment A after the system attains equilibrium is ______________.


14
The plot given below shows $$P-T$$ curves (where $$P$$ is the pressure and $$T$$ is the temperature) for two solvents $$X$$ and $$Y$$ and isomolal solutions of $$NaCl$$ in these solvents. $$NaCl$$ completely dissociates in both the solvents.

On addition of equal number of moles of a non-volatile solute $$S$$ in equal amount (in $$kg$$) of these solvents, the elevation of boiling point of solvent $$X$$ is three times that of solvent $$Y$$. Solute $$S$$ is known to undergo dimerization in these solvents. If the degree of dimerization is $$0.7$$ in solvent $$Y$$, the degree of dimerization in solvent $$X$$ is ___________.

On addition of equal number of moles of a non-volatile solute $$S$$ in equal amount (in $$kg$$) of these solvents, the elevation of boiling point of solvent $$X$$ is three times that of solvent $$Y$$. Solute $$S$$ is known to undergo dimerization in these solvents. If the degree of dimerization is $$0.7$$ in solvent $$Y$$, the degree of dimerization in solvent $$X$$ is ___________.
15
An organic acid P $$\left( {{C_{11}}{H_{12}}{O_2}} \right)$$ can easily be oxidized to a dibasic acid which reacts with ethylene glycol to produce a polymer dacron. Upon ozonolysis, P gives an aliphatic ketone as one of the products. P undergoes the following reaction sequences to furnish R via Q. The compound P also undergoes another set of reactions to produce S.

The compound R is

The compound R is
16
The compound $$Y$$ is
17
An organic acid P $$\left( {{C_{11}}{H_{12}}{O_2}} \right)$$ can easily be oxidized to a dibasic acid which reacts with ethylene glycol to produce a polymer dacron. Upon ozonolysis, P gives an aliphatic ketone as one of the products. P undergoes the following reaction sequences to furnish R via Q. The compound P also undergoes another set of reactions to produce S.

The compound S is

The compound S is
18
The compound $$Z$$ is
Mathematics
1
For a non-zero complex number z, let arg(z) denote the principal argument with $$-$$ $$\pi $$ < arg(z) $$ \le $$ $$\pi $$. Then, which of the following statement(s) is (are) FALSE?
2
In a $$\Delta $$PQR = 30$$^\circ $$ and the sides PQ and QR have lengths 10$$\sqrt 3 $$ and 10, respectively. Then, which of the following statement(s) is(are) TRUE?
3
Let P1 : 2x + y $$-$$ z = 3 and P2 : x + 2y + z = 2 be two planes. Then, which of the following statement(s) is(are) TRUE?
4
For every twice differentiable function $$f:R \to [ - 2,2]$$ with $${(f(0))^2} + {(f'(0))^2} = 85$$, which of the following statement(s) is(are) TRUE?
5
Let f : R $$ \to $$ R and g : R $$ \to $$ R be two non-constant differentiable functions. If f'(x) = (e(f(x) $$-$$ g(x))) g'(x) for all x $$ \in $$ R and f(1) = g(2) = 1, then which of the following statement(s) is (are) TRUE?
6
Let f : [0, $$\infty $$) $$ \to $$ R be a continuous function such that
$$f(x) = 1 - 2x + \int_0^x {{e^{x - t}}f(t)dt} $$ for all x $$ \in $$ [0, $$\infty $$). Then, which of the following statement(s) is (are) TRUE?
$$f(x) = 1 - 2x + \int_0^x {{e^{x - t}}f(t)dt} $$ for all x $$ \in $$ [0, $$\infty $$). Then, which of the following statement(s) is (are) TRUE?
7
The value of $${({({\log _2}9)^2})^{{1 \over {{{\log }_2}({{\log }_2}9)}}}} \times {(\sqrt 7 )^{{1 \over {{{\log }_4}7}}}}$$ is ....................
8
The number of 5 digit numbers which are divisible by 4, with digits from the set {1, 2, 3, 4, 5} and the repetition of digits is allowed, is .................
9
Let X be the set consisting of the first 2018 terms of the arithmetic progression 1, 6, 11, ...., and Y be the set consisting of the first 2018 terms of the arithmetic progression 9, 16, 23, .... . Then, the number of elements in the set X $$ \cup $$ Y is .........
10
The number of real solutions of the equation $$\eqalign{
& {\sin ^{ - 1}}\left( {\sum\limits_{i = 1}^\infty {} {x^{i + 1}} - x\sum\limits_{i = 1}^\infty {} {{\left( {{x \over 2}} \right)}^i}} \right) \cr
& = {\pi \over 2} - {\cos ^1}\left( {\sum\limits_{i = 1}^\infty {} {{\left( {{{ - x} \over 2}} \right)}^i} - \sum\limits_{i = 1}^\infty {} {{\left( { - x} \right)}^i}} \right) \cr} $$ lying in the interval $$\left( { - {1 \over 2},{1 \over 2}} \right)$$ is ........... .
(Here, the inverse trigonometric functions sin$$-$$1 x and cos$$-$$1 x assume values in $${\left[ { - {\pi \over 2},{\pi \over 2}} \right]}$$ and $${\left[ {0,\pi } \right]}$$, respectively.)
(Here, the inverse trigonometric functions sin$$-$$1 x and cos$$-$$1 x assume values in $${\left[ { - {\pi \over 2},{\pi \over 2}} \right]}$$ and $${\left[ {0,\pi } \right]}$$, respectively.)
11
For each positive integer n, let
$${y_n} = {1 \over n}(n + 1)(n + 2)...{(n + n)^{{1 \over n}}}$$.
For x$$ \in $$R, let [x] be the greatest integer less than or equal to x. If $$\mathop {\lim }\limits_{n \to \infty } {y_n} = L$$, then the value of [L] is ..............12
Let a and b be two unit vectors such that a . b = 0. For some x, y$$ \in $$R, let $$\overrightarrow c = x\overrightarrow a + y\overrightarrow b + \overrightarrow a \times \overrightarrow b $$. If | $$\overrightarrow c $$| = 2 and the vector c is inclined at the same angle $$\alpha $$ to both a and b, then the value of $$8{\cos ^2}\alpha $$ is ..............
13
Let a, b, c three non-zero real numbers such that the equation $$\sqrt 3 a\cos x + 2b\sin x = c,x \in \left[ { - {\pi \over 2},{\pi \over 2}} \right]$$, has two distinct real roots $$\alpha $$ and $$\beta $$ with $$\alpha + \beta = {\pi \over 3}$$. Then, the value of $${b \over a}$$ is ............
14
A farmer F1 has a land in the shape of a triangle with vertices at P(0, 0), Q(1, 1) and R(2, 0). From this land, a neighbouring farmer F2 takes away the region which lies between the sides PQ and a curve of the form y = xn (n > 1). If the area of the region taken away by the farmer F2 is exactly 30% of the area of $$\Delta $$PQR, then the value of n is .................
15
Let S be the circle in the XY-plane defined the equation x2 + y2 = 4.
Let E1E2 and F1F2 be the chords of S passing through the point P0 (1, 1) and parallel to the X-axis and the Y-axis, respectively. Let G1G2 be the chord of S passing through P0 and having slope$$-$$1. Let the tangents to S at E1 and E2 meet at E3, then tangents to S at F1 and F2 meet at F3, and the tangents to S at G1 and G2 meet at G3. Then, the points E3, F3 and G3 lie on the curve
Let E1E2 and F1F2 be the chords of S passing through the point P0 (1, 1) and parallel to the X-axis and the Y-axis, respectively. Let G1G2 be the chord of S passing through P0 and having slope$$-$$1. Let the tangents to S at E1 and E2 meet at E3, then tangents to S at F1 and F2 meet at F3, and the tangents to S at G1 and G2 meet at G3. Then, the points E3, F3 and G3 lie on the curve
16
Let S be the circle in the XY-plane defined the equation x2 + y2 = 4.
Let P be a point on the circle S with both coordinates being positive. Let the tangent to S at P intersect the coordinate axes at the points M and N. Then, the mid-point of the line segment MN must lie on the curve
Let P be a point on the circle S with both coordinates being positive. Let the tangent to S at P intersect the coordinate axes at the points M and N. Then, the mid-point of the line segment MN must lie on the curve
17
There are five students S1, S2, S3, S4 and S5 in a music class and for them there are five seats R1, R2, R3, R4 and R5 arranged in a row, where initially the seat Ri is allotted to the student Si, i = 1, 2, 3, 4, 5. But, on the examination day, the five students are randomly allotted the five seats.
(There are two questions based on Paragraph "A", the question given below is one of them)
The probability that, on the examination day, the student S1 gets the previously allotted seat R1, and NONE of the remaining students gets the seat previously allotted to him/her is
(There are two questions based on Paragraph "A", the question given below is one of them)
The probability that, on the examination day, the student S1 gets the previously allotted seat R1, and NONE of the remaining students gets the seat previously allotted to him/her is
18
There are five students S1, S2, S3, S4 and S5 in a music class and for them there are five seats R1, R2, R3, R4 and R5 arranged in a row, where initially the seat Ri is allotted to the student Si, i = 1, 2, 3, 4, 5. But, on the examination day, the five students are randomly allotted the five seats.
(There are two questions based on Paragraph "A", the question given below is one of them)
For i = 1, 2, 3, 4, let Ti denote the event that the students Si and Si+1 do NOT sit adjacent to each other on the day of the examination. Then, the probability of the event $${T_1} \cap {T_2} \cap {T_3} \cap {T_4}$$ is
(There are two questions based on Paragraph "A", the question given below is one of them)
For i = 1, 2, 3, 4, let Ti denote the event that the students Si and Si+1 do NOT sit adjacent to each other on the day of the examination. Then, the probability of the event $${T_1} \cap {T_2} \cap {T_3} \cap {T_4}$$ is
Physics
1
Three identical capacitors $${C_1},{C_2}$$ and $${C_3}$$ have a capacitance of $$1.0\,\mu F$$ each and they are unchanged initially. They are connected in a circuit as shown in the figure and $${C_1}$$ is then filled completely with a dielectric material of relative permittivity $${\varepsilon _r}.$$ The cell electromotive force $$\left( {emf} \right)\,\,{V_0} = 8V.$$ First the switch $${S_1}$$ is closed while the switch $${S_2}$$ is kept open. When the capacitor $${C_3}$$ is fully charged, $${S_1}$$ is opened and $${S_2}$$ is closed simultaneously. When all the capacitors reach equilibrium, the charge on $${C_3}$$ is found to be $$5\,\mu C.$$ The value of $${\varepsilon _r} = $$ _________________.


2
Consider a body of mass $$1.0$$ $$kg$$ at rest at the origin at time $$t=0.$$ A force $$\overrightarrow F = \left( {\alpha t \widehat i + \beta \widehat j} \right)$$ is applied on the body, where $$\alpha = 1.0N{s^{ - 1}}$$ and $$\beta = 1.0\,N.$$ The torque acting on the body about the origin at time $$t=1.0s$$ is $$\overrightarrow \tau .$$ Which of the following statements is (are) true?
3
A uniform capillary tube of inner radius $$r$$ is dipped vertically into a beaker filled with water. The water rises to a height $$h$$ in the capillary tube above the water surface in the beaker. The surface tension of water is $$\sigma .$$ The angle of contact between water and the wall of the capillary tube is $$\theta .$$ Ignore the mass of water in the meniscus. Which of the following statements is (are) true?
4
Two infinitely long straight wires lie in the $$xy$$-plane along the lines $$x = \pm R.$$ The wire located at $$x = + R$$ carries a constant current $${I_1}$$ and the wire located at $$x=-R$$ carries a constant current $${I_2}.$$ A circular loop of radius $$R$$ is suspended with its center at $$\left( {0,0,\sqrt 3 R} \right)$$ and in a plane parallel to the $$xy$$-plane. This loop carries a constant current $$I$$ in the clockwise direction as seen from above the loop. The current in the wire is taken to be positive if it is in the $$ + \widehat j$$ direction. which of the following statements regarding the magnetic field $$\overrightarrow B $$ is (are) true?
5
Two men are walking along a horizontal straight line in the same direction. The man in front walks at a speed $$1.0\,m{s^{ - 1}}$$ and the man behind walks at a speed $$2.0\,m{s^{ - 1}}.$$ A third man in standing at a height $$12$$ $$m$$ above the same horizontal line such that all three men are in a vertical plane. The two walking men are blowing identical whistles which emit a sound of frequency $$1430$$ $$Hz$$. The speed of sound in air is $$330\,m{s^{ - 1}}.$$ At the instant, when the moving men are $$10$$ $$m$$ apart, the stationary man is equidistant from them. The frequency of beats in $$Hz,$$ heard by the stationary man at this instant, is _____________.
6
A spring-block system is resting on a frictionless floor as shown in the figure. The spring constant is $$2.0\,N{m^{ - 1}}$$ and the mass of the block is $$2.0$$ $$kg.$$ Ignore the mass of the spring. Initially the spring is an unstretched condition. Another block of mass $$1.0$$ $$kg$$ moving with a speed of $$2.0$$ $$m{s^{ - 1}}$$ collides elastically with the first block. The collision is such that the $$2.0$$ $$kg$$ block does not hit the wall. The distance, in metres, between the two blocks when the spring returns to its unstretched position for the first time after the collision is __________.


7
One mole of a monatomic ideal gas undergoes a cyclic process as shown in the figure (where $$V$$ is the volume and $$T$$ is the temperature). Which of the statements below is (are) true?


8
Two vectors $$\overrightarrow A $$ and $$\overrightarrow B $$ are defined as $$\overrightarrow A $$ $$=$$ $$a\widehat i$$ and $$\overrightarrow B = a$$ $$\left( {\cos \,\omega T\widehat i + \sin \,\omega t\,\widehat j} \right),$$ where $$a$$ is a constant and $$\omega = \pi /6\,\,rad{s^{ - 1}}.$$ If $$\left| {\overrightarrow A + \overrightarrow B } \right| = \sqrt 3 \left| {\overrightarrow A - \overrightarrow B } \right|$$ at time $$t = \tau $$ for the first time, the value of $$\tau ,$$ in second, is ______________.
9
A ring and disc are initially at rest, side by side, at the top of an inclined plane which makes an angle $${60^ \circ }$$ with the horizontal. They start to roll without slipping at the same instant of time along the shortest path. If the time difference between their reaching the ground is $$\left( {2 - \sqrt 3 } \right)/\sqrt {10} \,\,s,$$ then the height of the top of the inclined plane, in metres is ______________ . Take $$g = 10\,\,m{s^{ - 2}}.$$
10
In the figure below, the switches $${S_1}$$ and $${S_2}$$ are closed simultaneously at $$t=0$$ and a current starts to flow in the circuit. Both the batteries have the same magnitude of the electromotive force (emf) and the polarities are as indicated in the figure. Ignore mutual inductance between the inductors. The current $$I$$ in the middle wire reaches its maximum magnitude $${I_{\max }}$$ at time $$t = \tau $$ . Which of the following statements is (are) true?


11
In the $$xy$$-plane, the region $$y > 0$$ has a uniform magnetic field $${B_1}\widehat k$$ and the region $$y < 0$$ has another uniform magnetic field $${B_2}\widehat k.$$ A positively charged particle is projected from the origin along the positive $$y$$-axis with speed $${v_0} = \pi \,m{s^{ - 1}}$$ at $$t=0,$$ as shown in the figure. Neglect gravity in this problem. Let $$t=T$$ be the time when the particle crosses the $$x$$-axis from below for the first time. If $${B_2} = 4{B_1},$$ the average speed of the particle, in $$m{s^{ - 1}},$$ along the $$x$$-axis in the time interval $$T$$ is ___________.
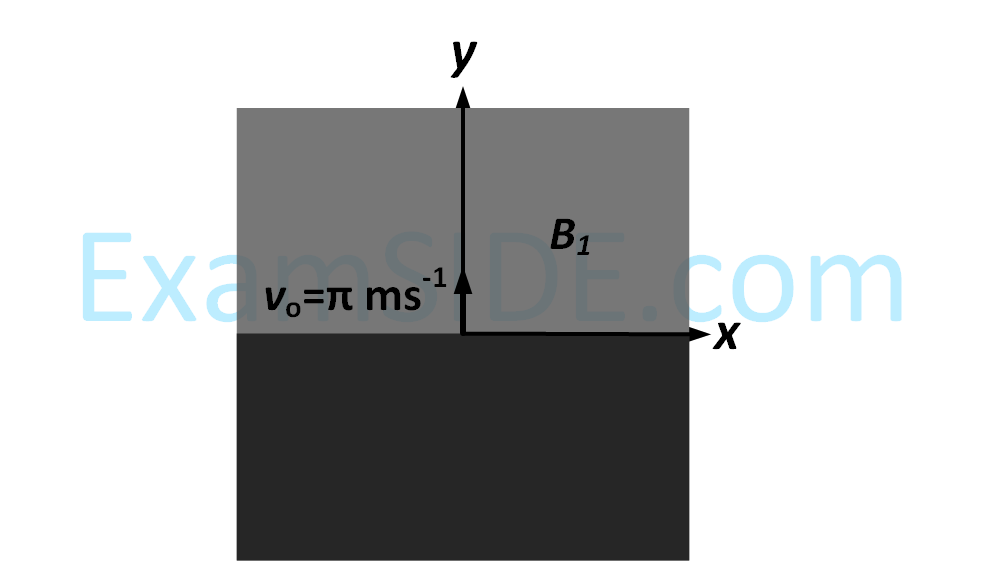

12
Two conducting cylinders of equal length but different radii are connected in series between two heat baths kept at temperatures $${T_1} = 300\,K$$ and $${T_2} = 100\,K$$, as shown in the figure. The radius of the bigger cylinder is twice that of the smaller once and the thermal conductivities of the materials of the smaller and the larger cylinders are $${K_1}$$ and $${K_2}$$ respectively. If the temperature at the junction of the two cylinders in the steady state is $$200$$ $$K,$$ then $${K_1}/{K_2} = $$ ______________.


13
Sunlight of intensity $$1.3$$ $$kW\,{m^{ - 2}}$$ is incident normally on a thin convex lens of focal length $$20$$ cm. Ignore the energy loss of light due to the lens and assume that the lens aperture size is much smaller than its focal length. The average intensity of light, in $$kW\,{m^{ - 2}},$$ at a distance $$22$$ cm from the lens on the other side is ___________.
14
In electromagnetic theory, the electric and magnetic phenomena are related to each other. Therefore, the dimensions of electric and magnetic quantities must also be related to each other. In the questions below, $$[E]$$ and $$[B]$$ stand for dimensions of electric and magnetic fields respectively, while $$\left[ {{\varepsilon _0}} \right]$$ and $$\left[ {{\mu _0}} \right]$$ stand for dimensions of the permittivity and permeability of free space respectively. $$\left[ L \right]$$ and $$\left[ T \right]$$ are dimensions of length and time respectively. All the quantities are given in $$SI$$ units.
The relation between $$\left[ {{\varepsilon _0}} \right]$$ and $$\left[ {{\mu _0}} \right]$$ is
The relation between $$\left[ {{\varepsilon _0}} \right]$$ and $$\left[ {{\mu _0}} \right]$$ is
15
In electromagnetic theory, the electric and magnetic phenomena are related to each other. Therefore, the dimensions of electric and magnetic quantities must also be related to each other. In the questions below, $$[E]$$ and $$[B]$$ stand for dimensions of electric and magnetic fields respectively, while $$\left[ {{\varepsilon _0}} \right]$$ and $$\left[ {{\mu _0}} \right]$$ stand for dimensions of the permittivity and permeability of free space respectively. $$\left[ L \right]$$ and $$\left[ T \right]$$ are dimensions of length and time respectively. All the quantities are given in $$SI$$ units.
The relation between $$[E]$$ and $$[B]$$ is
The relation between $$[E]$$ and $$[B]$$ is
16
If the measurement errors in all the independent quantities are known, then it is possible to determine the error in any dependent quantity. This is done by the use of series expansion and truncating the expansion at the first power of the error. For example, consider the relation $$z = x/y.$$ If the errors in $$x,y$$ and $$z$$ are $$\Delta x,\Delta y$$ and $$\Delta z,$$ respectively, then
$$$z \pm \Delta z = {{x \pm \Delta x} \over {y \pm \Delta y}} = {x \over y}\left( {1 \pm {{\Delta x} \over x}} \right){\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}}.$$$
The series expansion for $${\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}},$$ to first power in $$\Delta y/y.$$ is $$1 \pm \left( {\Delta y/y} \right).$$ The relative errors in independent variables are always added. So the error in $$z$$ will be
$$$\Delta z = z\left( {{{\Delta x} \over x} + {{\Delta y} \over y}} \right).$$$
The above derivation makes the assumption that $$\Delta x/x < < 1,$$ $$\Delta y/y < < 1.$$ Therefore, the higher powers of these quantities are neglected.
Consider the ratio $$r = {{\left( {1 - a} \right)} \over {1 + a}}$$ to be determined by measuring a dimensionless quantity $$a.$$ If the error in the measurement of $$a$$ is $$\Delta a\left( {\Delta a/a < < 1.} \right.$$ then what is the error $$\Delta r$$ in determining $$r$$?
$$$z \pm \Delta z = {{x \pm \Delta x} \over {y \pm \Delta y}} = {x \over y}\left( {1 \pm {{\Delta x} \over x}} \right){\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}}.$$$
The series expansion for $${\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}},$$ to first power in $$\Delta y/y.$$ is $$1 \pm \left( {\Delta y/y} \right).$$ The relative errors in independent variables are always added. So the error in $$z$$ will be
$$$\Delta z = z\left( {{{\Delta x} \over x} + {{\Delta y} \over y}} \right).$$$
The above derivation makes the assumption that $$\Delta x/x < < 1,$$ $$\Delta y/y < < 1.$$ Therefore, the higher powers of these quantities are neglected.
Consider the ratio $$r = {{\left( {1 - a} \right)} \over {1 + a}}$$ to be determined by measuring a dimensionless quantity $$a.$$ If the error in the measurement of $$a$$ is $$\Delta a\left( {\Delta a/a < < 1.} \right.$$ then what is the error $$\Delta r$$ in determining $$r$$?
17
If the measurement errors in all the independent quantities are known, then it is possible to determine the error in any dependent quantity. This is done by the use of series expansion and truncating the expansion at the first power of the error. For example, consider the relation $$z = x/y.$$ If the errors in $$x,y$$ and $$z$$ are $$\Delta x,\Delta y$$ and $$\Delta z,$$ respectively, then
$$$z \pm \Delta z = {{x \pm \Delta x} \over {y \pm \Delta y}} = {x \over y}\left( {1 \pm {{\Delta x} \over x}} \right){\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}}.$$$
The series expansion for $${\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}},$$ to first power in $$\Delta y/y.$$ is $$1 \pm \left( {\Delta y/y} \right).$$ The relative errors in independent variables are always added. So the error in $$z$$ will be
$$$\Delta z = z\left( {{{\Delta x} \over x} + {{\Delta y} \over y}} \right).$$$
The above derivation makes the assumption that $$\Delta x/x < < 1,$$ $$\Delta y/y < < 1.$$ Therefore, the higher powers of these quantities are neglected.
In an experiment the initial number of radioactive nuclei is $$3000.$$ It is found that $$1000 \pm 40$$ nuclei decayed in the first $$1.0s.$$ For $$\left| x \right| < < 1.$$ $$\ln \left( {1 + x} \right) = x$$ up to first power in $$x.$$ The error $$\Delta \lambda ,$$ in the determination of the decay constant $$\lambda ,$$ in $${s^{ - 1}},$$ is
$$$z \pm \Delta z = {{x \pm \Delta x} \over {y \pm \Delta y}} = {x \over y}\left( {1 \pm {{\Delta x} \over x}} \right){\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}}.$$$
The series expansion for $${\left( {1 \pm {{\Delta y} \over y}} \right)^{ - 1}},$$ to first power in $$\Delta y/y.$$ is $$1 \pm \left( {\Delta y/y} \right).$$ The relative errors in independent variables are always added. So the error in $$z$$ will be
$$$\Delta z = z\left( {{{\Delta x} \over x} + {{\Delta y} \over y}} \right).$$$
The above derivation makes the assumption that $$\Delta x/x < < 1,$$ $$\Delta y/y < < 1.$$ Therefore, the higher powers of these quantities are neglected.
In an experiment the initial number of radioactive nuclei is $$3000.$$ It is found that $$1000 \pm 40$$ nuclei decayed in the first $$1.0s.$$ For $$\left| x \right| < < 1.$$ $$\ln \left( {1 + x} \right) = x$$ up to first power in $$x.$$ The error $$\Delta \lambda ,$$ in the determination of the decay constant $$\lambda ,$$ in $${s^{ - 1}},$$ is
18
The potential energy of a particle of mass $$m$$ at a distance $$r$$ from a fixed point $$O$$ is given by $$V\left( r \right) = k{r^2}/2,$$ where $$k$$ is a positive constant of appropriate dimensions. This particle is moving in a circular orbit of radius $$R$$ about the point $$O$$. If $$v$$ is the speed of the particle and $$L$$ is the magnitude of its angular momentum about $$O,$$ which of the following statements is (are) true?