The carbon–based reduction method is NOT used for the extraction of

In the nuclear transmutation 9Be4 + X $$\to$$ 8Be4 + Y (X, Y) is (are) :

The standard reduction potential data at 25oC is given below: Eo (Fe3+ , Fe2+) = +0.77V; Eo (Fe2+ , Fe) = -0.44V; Eo (

An aqueous solution of X is added slowly to an aqueous solution of Y as shown in List – I. The variation in conductivity

The thermal dissociation equilibrium of CaCO3(s) is studied under different conditions CaCO3(s) $$\leftrightharpoons$$ C

The Ksp of Ag2CrO4 is 1.1 $$\times$$ 10-12 at 298 K. The solubility (in mol/L) of Ag2CrO4 in a 0.1 M AgNO3 solution is

In the following reaction, the product/products formed is/are

The major product(s) of the following reaction is(are)

After completion of the reactions (I and II), the organic compound(s) in the reaction mixtures is(are)

The correct statement(s) about O3 is(are)

The precipitate P contains

The coloured solution S contains

Compounds formed from P and Q are, respectively,

In the following reaction sequences V and W are, respectively,

The succeeding operations that enable this transformation of states are

The pair of isochoric processes among the transformation of states is

P and Q, respectively, are the sodium salts of

R, S and T respectively, are

The unbalanced chemical reactions given in List I show missing reagent or condition (?) which are provided in List II. M

Match the chemical conversions in List I with the appropriate reagents in List II and select the correct answer using th

match List $$I$$ with List $$II$$ and select the correct answer using the code given below the lists: $$\,\,\,\,$$ $$\

Let $\omega=\frac{\sqrt{3}+i}{2}$ and $P=\left\{\omega^n: n=1,2,3, \ldots\right\}$. Further $\mathrm{H}_1=\left\{z \in

Consider the lines $${L_1}:{{x - 1} \over 2} = {y \over { - 1}} = {{z + 3} \over 1},{L_2} : {{x - 4} \over 1} = {{y + 3}

Two lines $${L_1}:x = 5,{y \over {3 - \alpha }} = {z \over { - 2}}$$ and $${L_2}:x = \alpha ,{y \over { - 1}} = {z \over

A box $${B_1}$$ contains $$1$$ white ball, $$3$$ red balls and $$2$$ black balls. Another box $${B_2}$$ contains $$2$$ w

A box $${B_1}$$ contains $$1$$ white ball, $$3$$ red balls and $$2$$ black balls. Another box $${B_2}$$ contains $$2$$ w

Let $$f:\left[ {0,1} \right] \to R$$ (the set of all real numbers) be a function. Suppose the function $$f$$ is twice di

Let $$f:\left[ {0,1} \right] \to R$$ (the set of all real numbers) be a function. Suppose the function $$f$$ is twice di

Match List $$I$$ with List $$II$$ and select the correct answer using the code given below the lists: List $$I$$ $$P.$$

In a triangle $$PQR$$, $$P$$ is the largest angle and $$\cos P = {1 \over 3}$$. Further the incircle of the triangle tou

Let $$PQ$$ be a focal chord of the parabola $${y^2} = 4ax$$. The tangents to the parabola at $$P$$ and $$Q$$ meet at a p

Let $$PQ$$ be a focal chord of the parabola $${y^2} = 4ax$$. The tangents to the parabola at $$P$$ and $$Q$$ meet at a p

A line $$L:y=mx+3$$ meets $$y$$-axis at R$$(0, 3)$$ and the arc of the parabola $${y^2} = 16x,$$ $$0 \le y \le 6$$ at th

Circle (s) touching x-axis at a distance 3 from the origin and having an intercept of length $$2\sqrt 7 $$ on y-axis is

If $${3^x}\, = \,{4^{x - 1}},$$ then $$x\, = $$

Let $$S = {S_1} \cap {S_2} \cap {S_3}$$, where $${S_1} = \left\{ {z \in C:\left| z \right| < 4} \right\},{S_2} = \lef

Let $$S = {S_1} \cap {S_2} \cap {S_3}$$, where $${S_1} = \left\{ {z \in C:\left| z \right| < 4} \right\},{S_2} = \lef

$$a \in R$$ (the set of all real numbers), a $$\ne$$ $$-$$1, $$\mathop {\lim }\limits_{n \to \infty } {{({1^a} + {2^a} +

Let $$\omega$$ be a complex cube root of unity with $$\omega$$ $$\ne$$ 1 and P = [pij] be a n $$\times$$ n matrix with p

The function $$f(x) = 2\left| x \right| + \left| {x + 2} \right| - \left| {\left| {x + 2} \right| - 2\left| x \right|} \

Two non-conducting spheres of radii $${R_1}$$ and $${R_2}$$ and carrying uniform volume charge densities $$ + \rho $$ an

Two bodies, each of mass M, are kept fixed with a separation $$2L$$. A particle of mass m is projected from the midpoint

A particle of mass m is attached to one end of a mass-less spring of force constant k, lying on a frictionless horizonta

The magnitude of the normal reaction that acts on the block at the point Q is

The speed of the block when it reaches the point Q is

Match List I with List II and select the correct answer using the codes given below the lists: List I P. Boltzmann Const

Using the expression $$2d\sin \theta = \lambda $$, one calculates the values of d by measuring the corresponding angles

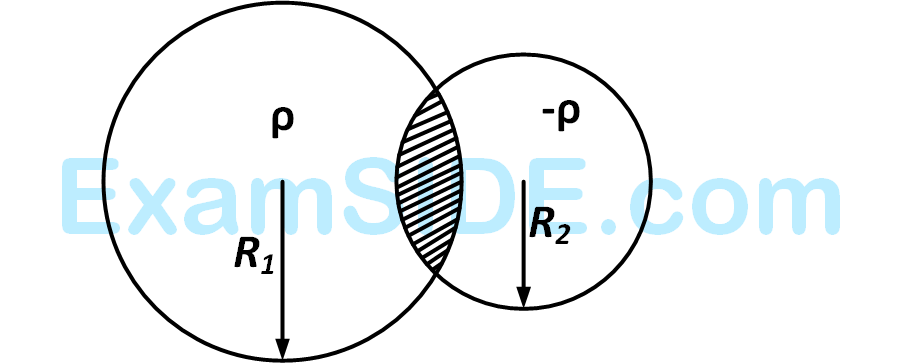
A steady current I flows along an infinitely long hollow cylindrical conductor of radius R. This cylinder is placed coax

Two vehicles, each moving with speed u on the same horizontal straight road, are approaching each other. Wind blows alon

The figure below shows the variation of specific heat capacity (C) of a solid as a function of temperature (T). The temp

The radius of the orbit of an electron in a hydrogen-like atom is 4.5a0, where a0 is the Bohr radius. Its orbital angula

If the direct transmission method with a cable of resistance 0.4 $$\Omega$$ km$$-$$1 is used, the power dissipation (in

In the method using the transformers, assume that the ratio of the number of turns in the primary to that in the seconda

The magnitude of the induced electric field in the orbit at any instant of time during the time interval of the magnetic

The change in the magnetic dipole moment associated with the orbit, at the end of the time interval of the magnetic fiel

The correct statement is

The kinetic energy (in keV) of the alpha particle, when the nucleus $$_{84}^{210}Po$$ at rest undergoes alpha decay, is

A right-angled prism of refractive index $$\mu$$1 is placed in a rectangular block of refractive index $$\mu$$2, which i

One mole of a monatomic ideal gas is taken along two cyclic processes E $$\to$$ F $$\to$$ G $$\to$$ E and E $$\to$$ F $$

Match List I of the nuclear processes with List II containing parent nucleus and one of the end products of each process