IIT-JEE 2007 Paper 2 Offline

MCQ (Single Correct Answer)

-1

STATEMENT 1

If there is no external torque on a body about its center of mass, then the velocity of the center of mass remains constant.

Because

STATEMENT 2

The linear momentum of an isolated system remains constant.

Statement-1 is True, Statement-2 is True; Statement-2 is a correct explanation for Statement-1.

Statement-1 is True, Statement-2 is True; Statement-2 is NOT a correct explanation for Statement-1.

Statement-1 is True, Statement-2 is False.

Statement-1 is False, Statement-2 is True.

IIT-JEE 2006

MCQ (Single Correct Answer)

-1

A solid sphere of radius $R$ has moment of inertia $I$ about its geometrical axis. If it is melted into a disc of radius $r$ and thickness $t$. If its moment of inertia about the tangential axis (which is perpendicular to plane of the disc), is also equal to $I$, then the value of $r$ is equal to

IIT-JEE 2006 Physics - Rotational Motion Question 1 English

$\frac{2}{\sqrt{15}} R$

$\frac{2}{\sqrt{5}} R$

$\frac{3}{\sqrt{15}} R$

$\frac{\sqrt{3}}{\sqrt{15}} R$

IIT-JEE 2001

MCQ (Single Correct Answer)

-1

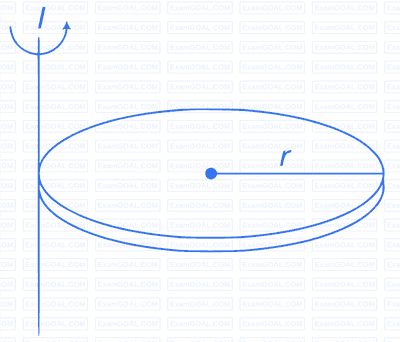
One quarter section is cut from a uniform circular disc of radius $R$. This section has a mass $M$. It is made to rotate about a line perpendicular to its plane and passing through the centre of the original disc. Its moment of inertia about the axis of rotation is

IIT-JEE 2001 Physics - Rotational Motion Question 2 English

$\dfrac{1}{2} MR^2$

$\dfrac{1}{4} MR^2$

$\dfrac{1}{8} MR^2$

$\sqrt{2} MR^2$

IIT-JEE 2000

MCQ (Single Correct Answer)

-1

A thin wire of length $L$ and uniform linear mass density $\rho$ is bent into a circular loop with centre at $O$ as shown. The moment of inertia of the loop about the axis $XX'$ is:

IIT-JEE 2000 Physics - Rotational Motion Question 3 English

$\frac{\rho L^3}{8\pi^2}$

$\frac{\rho L^3}{16\pi^2}$

$\frac{5\rho L^3}{16\pi^2}$

$\frac{3\rho L^3}{8\pi^2}$

JEE Advanced Subjects