A conducting square loop initially lies in the $X Z$ plane with its lower edge hinged along the $X$-axis. Only in the region $y \geq 0$, there is a time dependent magnetic field pointing along the $Z$-direction, $\vec{B}(t)=B_0(\cos \omega t) \hat{k}$, where $B_0$ is a constant. The magnetic field is zero everywhere else. At time $t=0$, the loop starts rotating with constant angular speed $\omega$ about the $X$ axis in the clockwise direction as viewed from the $+X$ axis (as shown in the figure). Ignoring self-inductance of the loop and gravity, which of the following plots correctly represents the induced e.m.f. $(V)$ in the loop as a function of time:

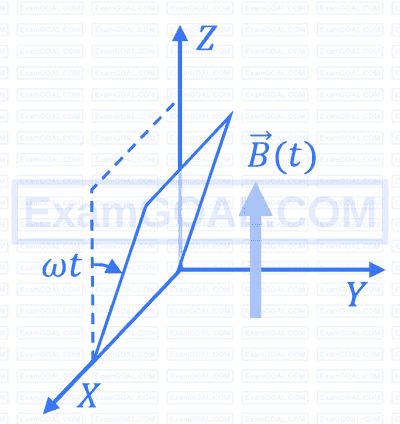
Figure 1 shows the configuration of main scale and Vernier scale before measurement. Fig. 2 shows the configuration corresponding to the measurement of diameter D of a tube. The measured value of D is:

A conducting square loop of side $L$, mass $M$ and resistance $R$ is moving in the $X Y$ plane with its edges parallel to the $X$ and $Y$ axes. The region $y \geq 0$ has a uniform magnetic field, $\vec{B}=B_0 \widehat{k}$. The magnetic field is zero everywhere else. At time $t=0$, the loop starts to enter the magnetic field with an initial velocity $v_0 \hat{\jmath} \mathrm{~m} / \mathrm{s}$, as shown in the figure. Considering the quantity $K=\frac{B_0^2 L^2}{R M}$ in appropriate units, ignoring self-inductance of the loop and gravity, which of the following statements is/are correct:

Length, breadth and thickness of a strip having a uniform cross section are measured to be 10.5 cm, 0.05 mm, and 6.0 μm, respectively. Which of the following option(s) give(s) the volume of the strip in cm³ with correct significant figures: