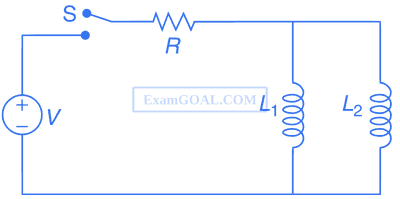
A source of constant voltage V is connected to a resistance R and two ideal inductors L₁ and L₂ through a switch S as shown. There is no mutual inductance between the two inductors. The switch S is initially open. At t = 0, the switch is closed and current begins to flow. Which of the following options is/are correct?

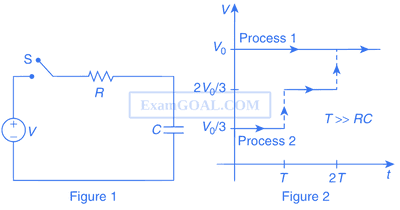
Consider a simple RC circuit as shown in Figure 1.

Process 1 : In the circuit the switch S is closed at t = 0 and the capacitor is fully charged to voltage V₀ (i.e. charging continues for time T >> RC). In the process some dissipation (E_D) occurs across the resistance R. The amount of energy finally stored in the fully charged capacitor is E_C.

Process 2 : In a different process the voltage is first set to $${{{V_0}} \over 3}$$ and maintained for a charging time T >> RC. Then, the voltage is raised to $${{2{V_0}} \over 3}$$ without discharging the capacitor and again maintained for a time T >> RC. The process is repeated one more time by raising the voltage to V₀ and the capacitor is charged to the same final voltage V₀ as in Process 1.

These two processes are depicted in Figure 2.

In Process 1, the energy stored in the capacitor E_C and heat dissipated across resistance E_D are related by

Consider a simple RC circuit as shown in Figure 1.

Process 1 : In the circuit the switch S is closed at t = 0 and the capacitor is fully charged to voltage V₀ (i.e. charging continues for time T >> RC). In the process some dissipation (E_D) occurs across the resistance R. The amount of energy finally stored in the fully charged capacitor is E_C.

Process 2 : In a different process the voltage is first set to $${{{V_0}} \over 3}$$ and maintained for a charging time T >> RC. Then, the voltage is raised to $${{2{V_0}} \over 3}$$ without discharging the capacitor and again maintained for a time T >> RC. The process is repeated one more time by raising the voltage to V₀ and the capacitor is charged to the same final voltage V₀ as in Process 1.

These two processes are depicted in Figure 2.

In Process 2, total energy dissipated across the resistance E_D is

One twirls a circular ring (of mass M and radius R) near the tip of one's finger as shown in Figure 1. In the process the finger never loses contact with the inner rim of the ring. The finger traces out the surface of a cone, shown by the dotted line. The radius of the path traced out by the point where the ring and the finger is in contact is r. The finger rotates with an angular velocity $$\omega$$₀. The rotating ring rolls without slipping on the outside of a smaller circle described by the point where the ring and the finger is in contact (Figure 2). The coefficient of friction between the ring and the finger is $$\mu$$ and the acceleration due to gravity is g.

The total kinetic energy of the ring is