In an electrostatic field, the electric displacement density vector, $$\overrightarrow D $$, is given by

$$\overrightarrow D (x,y,z) = ({x^3}\overrightarrow i + {y^3}\overrightarrow j + x{y^2}\overrightarrow k )$$ C/m²,

where, $$\overrightarrow i $$, $$\overrightarrow j $$, $$\overrightarrow k $$ are the unit vectors along x-axis, y-axis, and z-axis, respectively. Consider a cubical region R centered at the origin with each side of length 1 m, and vertices at ($$\pm$$ 0.5 m, $$\pm$$ 0.5 m, $$\pm$$ 0.5 m). The electric charge enclosed within R is __________ C (rounded off to two decimal places).

An electron (q₁) is moving in free space with velocity 10⁵ m/s towards a stationary electron (q₂) far away. The closest distance that this moving electron gets to the stationary electron before the repulsive force diverts its path is ___________ ×10^-8m.

[Given, mass of electron m = 9.11×10^-31 kg, charge of electron e = -1.6×10^-19 C , and permittivity $$\varepsilon_0=\left(1/36\mathrm\pi\right)\times10^{-9}\;\mathrm F/\mathrm m$$].

The parallel-plate capacitor shown in the figure has movable plates. The capacitor is charged so that the energy stored in it is E when the plate separation is d. The capacitor is then isolated electrically and the plates are moved such that the plate separation becomes 2d.

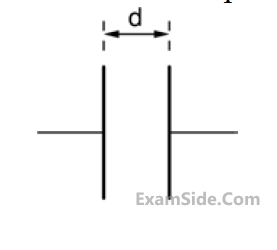
At this new plate separation, what is the energy stored in the capacitor, neglecting fringing effects?

A coaxial capacitor of inner radius 1 mm and outer radius 5 mm has a capacitance per unit length of 172 pF/m. If the ratio of outer radius to inner is doubled, the capacitance per unit length (in pF/m) is _____.