Complex Variable · Engineering Mathematics · GATE ECE
Marks 1
The value of the contour integral, $$\oint\limits_C {\left( {{{z + 2} \over {{z^2} + 2z + 2}}} \right)dz} $$, where the contour C is $$\left\{ {z:\left| {z + 1 - {3 \over 2}j} \right| = 1} \right\}$$, taken in the counter clockwise direction, is
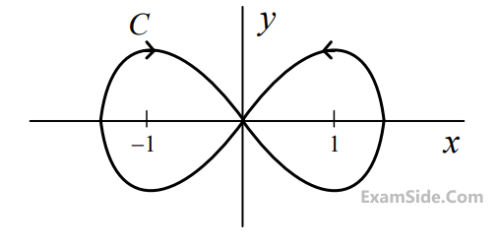
A simple closed path C in the complex plane is shown in the figure. If
$$\oint\limits_c {{{{2^z}} \over {{z^2} - 1}}dz = - i\pi A} $$,
where $$i = \sqrt { - 1} $$, then the value of A is ___________ (rounded off to two decimal places).

$$\left| z \right| = 3.$$ The value of the integral
$$\oint\limits_c {{{{z^2} - z + 4j} \over {z + 2j}}dz} $$ is
$$f(z) = {1 \over {{{\left( {z + 2} \right)}^2}{{\left( {z - 2} \right)}^2}}}$$ at z = 2 is
Marks 2
Which of the following statements involving contour integrals (evaluated counter-clockwise) on the unit circle $C$ in the complex plane is/are TRUE?
Let $z$ be a complex variable. If $f(z)=\frac{\sin(\pi z)}{z^{2}(z-2)}$ and $C$ is the circle in the complex plane with $|z|=3$ then $\oint\limits_{C} f(z)dz$ is _______.
Consider the following series :
$$\sum\limits_{n = 1}^\infty {{{{n^d}} \over {{c^n}}}} $$
For which of the following combinations of c, d values does this series converge?
 The value of the integral $${1 \over {\pi j}}\oint\limits_C {{{dz} \over {{z^2} - 1}}} $$ is ________________.
The value of the integral $${1 \over {\pi j}}\oint\limits_C {{{dz} \over {{z^2} - 1}}} $$ is ________________.If $$C$$ is defined as $$\left| z \right| = 3,$$ then the value of $${\rm I}$$ is
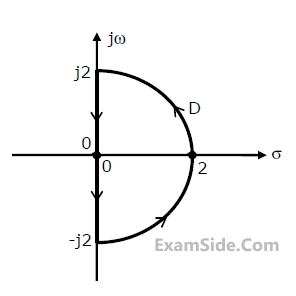
(i) the point $${z_0} = 2$$ inside the contour $$c,$$ and
(ii) the point $${z_0} = 2$$ outside the contour $$c,$$ respectively, are
$$\left| {z + 1} \right| = 1,$$ the value of $${1 \over {2\,\pi \,j}}\oint\limits_c {f\left( z \right)dz} $$ is