Continuous Time Linear Invariant System · Signals and Systems · GATE ECE
Marks 1
I. There is no bounded input bounded output (BIBO) stable system with a pole in the right half of the complex plane.
II. There is no causal and BIBO stable system with a pole in the right half of the complex plane.
Which one among the following is correct?
If G(s) is a stable transfer function, then $$F\left( s \right) = {1 \over {G\left( s \right)}}$$ is always a stable transfer function.
Marks 2
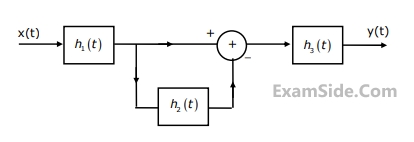
Let y(t) be the output of this filter. The maximum value of $$\left| {y(t)} \right|$$ is ________________________.
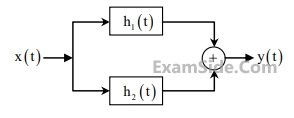
The impulse responses of the systems are
$${h_1}(t) = 2\delta (t + 2)\, - 3\delta (t + 1)$$
$${h_2}(t) = \delta (t - 2)$$
If the input x(t) is a unit step signal, then the energy of y(t) is__________________.
If the input to the system is $$x(t) = 8\cos \left( {20\pi t + \,{\pi \over 4}} \right) + \,16\sin \left( {40\pi t + {\pi \over 8}} \right) + 24\,\cos \left( {80\pi t + {\pi \over {16}}} \right)$$
Then the average power of the output signal y(t) is_____________.
The transfer function of the system is
The system is
Assuming zero initial conditions, the response y(t) of the above system for the input x(t) = $${e^{ - 2t}}$$ u(t) is given by
Where h(t) is shown in the graph.
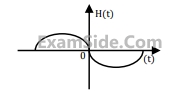
Which of the following four properties are possessed by the system?
BIBO: Bounded input gives a bounded output.
Causal: The system is casual.
LP: The system is low pass.
LTI: The system is linear and time- invariant.
Properties
P1 : Linear but NOT time-invariant
P2: Time-invariant but NOT linear
P3: Linear and time-invariant
Relations
R1: y(t) = $${t^2}$$ x(t)
R2: y(t) = t$$\left| {x(t)} \right|$$
R3: y(t) = $$\left| {x(t)} \right|$$
R4: y(t) = x(t-5)
$${h_1}$$(t), = 1;
$${h_2}$$(t), = U(t);
$${h_3}(t)\, = \,{{U(t)} \over {t + 1}}$$;
$${h_4}(t)\, = {e^{ - 3t}}U(t)$$ ,
where U (t) is the unit step function. Which of these system is time invariant, causal, and stable?
In the case of a linear time invariant system
List - 1
(1) Poles in the right half plane implies.
(2) Impulse response zero for $$t \le 0$$ implies.
List - 2
(A) Exponential decay of output
(B) System is causal
(C) No stored energy in the system
(D) System is unstable
List - 1
(A) $${a_1}{{{d^{2y}}} \over {d{x^2}}} + {a_2}y{{dy} \over {dx}} + {a_3}y = {a_4}$$
(B) $${a_1}{{{d^3}y} \over {d{x^3}}} + {a_2}y = {a_3}$$
(C) $$\eqalign{
& {a_1}{{{d_2}y} \over {d{x_2}}} + {a_2}x{{dy} \over {dx}} + {a_3}\,{x^2}y = 0 \cr
& \cr} $$
List - 2
(1) Non linear differential equation.
(2) Linear differential equation with constant coefficients.
(3) Linear homogeneous differential equation.
(4) Non - Linear homogeneous differential equation.
(5) Non - linear first order differential equation.
The voltage v(t) is

Marks 5
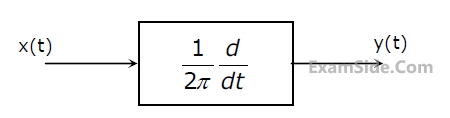
(a) Determine the autocorrelation Rxx ($$\tau $$) and the power spectral density Sxx(f).
(b) Find the output power spectral density Syy( f ).
(c) Evaluate Rxy(0) and Rxy(1/4).
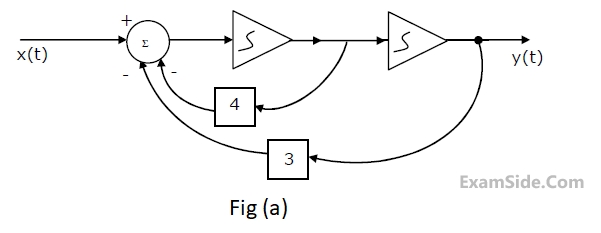
(a) Find the transfer function.
(b) For the step response of the system [i.e. find y(t) when x(t) is a unit step function and the initial conditions are zero]
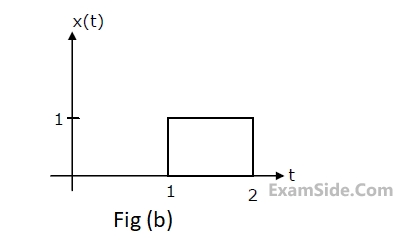
(c) Find y(t), if x(t) is as shown in Fig.(b), and the initial conditions are zero.
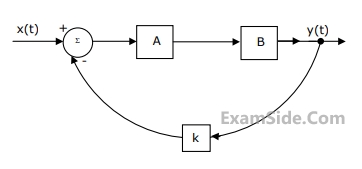
(a) Find the transfer function $${{Y(s)} \over {X(s)}}$$, when k=1
(b) Find the impulse response, when k = 0
(c) Find the value of k for which the system becomes unstable.
$$$\left[ {\matrix{ {Note:u(t)\, \equiv \,0} & {t\, \le \,0} \cr {\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \equiv 1} & {t\, > \,0} \cr } } \right]$$$
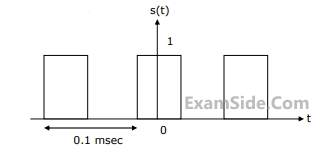
$${h_3}\,(t)\, = u(t)\,and\,x(t)\, = \,\sin \,2\,{\omega _0}t\, + \,\cos \,({\omega _0}t/2),$$ find the output y(t).