1
GATE ECE 2001
Subjective
+5
-0
A medium has breakdown strength of $$16$$ KV/m r.m.s. Its relative permeability is $$1.0$$ and relative permittivity is $$4.0$$. A plane electromagnetic wave is transmitted through the medium. Calculate the maximum possible power flow density and the associated magnetic filed.
2
GATE ECE 1999
Subjective
+5
-0
A plane wave in free space with
$$\overrightarrow E = \left( {\sqrt \pi } \right)\left( {10.0\,\widehat x + 11.8\,\widehat y} \right)\exp \left[ {j\left( {4\pi \times {{10}^8}\,t - k\,z} \right)} \right]$$
where $$\widehat x$$ and $$\widehat y$$ are unit vectors in the $$x$$- and $$y$$-directions respectively is incident normally on a semi-infinite block of ice as shown in Fig. For ice, $$\mu = {\mu _0},\,\,\,\sigma = 0$$ and $$\varepsilon = 9{\varepsilon _0}\left( {1 - j0.001} \right)$$.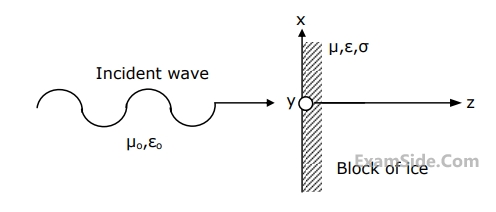
$$\overrightarrow E = \left( {\sqrt \pi } \right)\left( {10.0\,\widehat x + 11.8\,\widehat y} \right)\exp \left[ {j\left( {4\pi \times {{10}^8}\,t - k\,z} \right)} \right]$$
where $$\widehat x$$ and $$\widehat y$$ are unit vectors in the $$x$$- and $$y$$-directions respectively is incident normally on a semi-infinite block of ice as shown in Fig. For ice, $$\mu = {\mu _0},\,\,\,\sigma = 0$$ and $$\varepsilon = 9{\varepsilon _0}\left( {1 - j0.001} \right)$$.
(a) Calculate the average power density associated with the incident wave.
(b) Calculate the skin depth in ice.
(c) Estimate the average power density at a distance of 5 times the skins depth in the ice block, measured from the interface.

3
GATE ECE 1998
Subjective
+5
-0
A plane wave with $$\overrightarrow E = 10\,{e^{j\left( {\omega t - \beta z} \right)\,}}\,\,{\overrightarrow a _{_y}}$$ is incident normally on a thick plane conductor lying in the $$X - Y$$ plane. Its conductivity is $$6 \times {10^6}\,\,\,S/m\,\,\,$$ and surface impedance is $$5 \times {0^{ - 4}}\,\angle {45^0}\Omega $$. Determine the propagation constant and the skin depth in the conductor.
4
GATE ECE 1998
Subjective
+5
-0
The electric field vector of a wave is given as
$$$\vec E = {E_0}{\mkern 1mu} {e^{j\left( {\omega t + 3x - 4y} \right)}}{\mkern 1mu} {{8{{\vec a}_x} + 6{{\vec a}_y} + 5{{\vec a}_z}} \over {\sqrt {125} }}\,\,V/m$$$
Its frequency is 10 GHz.
(i) Investigate if this wave is a plane wave.
(ii) Determine its propagation constant, and
(iii) Calculate the phase velocity in $$y$$-direction.
Questions Asked from Uniform Plane Waves (Marks 5)
Number in Brackets after Paper Indicates No. of Questions
GATE ECE Subjects
Network Theory
Control Systems
Electronic Devices and VLSI
Analog Circuits
Digital Circuits
Microprocessors
Signals and Systems
Representation of Continuous Time Signal Fourier Series Fourier Transform Continuous Time Signal Laplace Transform Discrete Time Signal Fourier Series Fourier Transform Discrete Fourier Transform and Fast Fourier Transform Discrete Time Signal Z Transform Continuous Time Linear Invariant System Discrete Time Linear Time Invariant Systems Transmission of Signal Through Continuous Time LTI Systems Sampling Transmission of Signal Through Discrete Time Lti Systems Miscellaneous
Communications
Electromagnetics
General Aptitude