
A region in the form of an equilateral triangle (in $x-y$ plane) of height $L$ has a uniform magnetic field $\vec{B}$ pointing in the $+z$-direction. A conducting loop $\mathrm{PQR}$, in the form of an equilateral triangle of the same height $L$, is placed in the $x-y$ plane with its vertex $\mathrm{P}$ at $x=0$ in the orientation shown in the figure. At $t=0$, the loop starts entering the region of the magnetic field with a uniform velocity $\vec{v}$ along the $+x$-direction. The plane of the loop and its orientation remain unchanged throughout its motion.
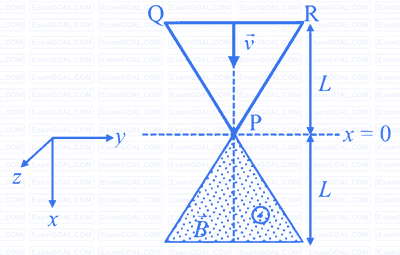
Which of the following graph best depicts the variation of the induced emf $(E)$ in the loop as a function of the distance $(x)$ starting from $x=0$ ?

[Given: The acceleration due to gravity $g=10 \mathrm{~m} \mathrm{~s}^{-2}$ and $e^{-1}=0.4$ ]
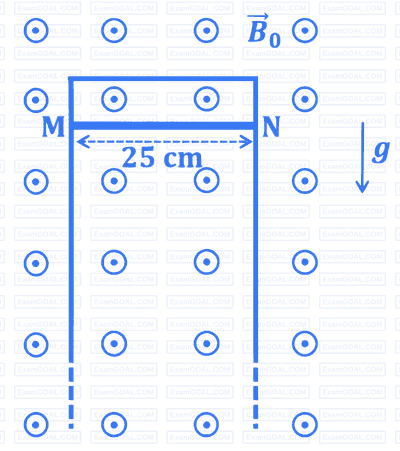
| List - I | List - II |
|---|---|
| (P) At $t=0.2 \mathrm{~s}$, the magnitude of the induced emf in Volt | (1) 0.07 |
| (Q) At $t=0.2 \mathrm{~s}$, the magnitude of the magnetic force in Newton | (2) 0.14 |
| (R) At $t=0.2 \mathrm{~s}$, the power dissipated as heat in Watt | (3) 1.20 |
| (S) The magnitude of terminal velocity of the rod in $\mathrm{m} \mathrm{s}^{-1}$ | (4) 0.12 |
| (5) 2.00 |
The figure shows certain wire segments joined together to form a coplanar loop. The loop is placed in a perpendicular magnetic field in the direction going into the plane of the figure. The magnitude of the field increases with time. $$I_1$$ and $$I_2$$ are the currents in the segments ab and cd. Then,




