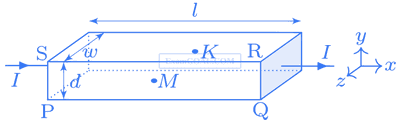
In a thin rectangular metallic strip a constant current I flows along the positive x-direction, as shown in the figure. The length, width and thickness of the strip are l, w and d, respectively.

A uniform magnetic field B is applied on the strip along the positive y-direction. Due to this, the charge carriers experience a net deflection along the z-direction. This results in accumulation of charge carriers on the surface PQRS and appearance of equal and opposite charges on the face opposite PQRS. A potential difference along the z-direction is thus developed. Charge accumulation continues until the magnetic force is balanced by the electric force. The current is assumed to be uniformly distributed on the cross section of the strip and carried by electrons.

A steady current I flows along an infinitely long hollow cylindrical conductor of radius R. This cylinder is placed coaxially inside an infinite solenoid of radius 2R. The solenoid has n turns per unit length and carries a steady current I. Consider a point P at a distance r from the common axis. The correct statement(s) is(are)

A particle of mass M and positive charge Q, moving with a constant velocity $${\overrightarrow u _1} = 4\widehat i$$ ms^$$-$$1 enters a region of uniform static magnetic field, normal to the xy plane. The region of the magnetic field extends from x = 0 to x = L for all values of y. After passing through this region, the particle emerges on the other side after 10 ms with a velocity $${\overrightarrow u _2} = 2\left( {\sqrt 3 \widehat i + \widehat j} \right)$$ ms^$$-$$1. The correct statement(s) is(are)