The Fourier transform of a signal h(t) is $$H(j\omega )$$ =(2 cos $$\omega $$) (sin 2$$\omega $$) / $$\omega $$. The value of h(0) is

The signal x(t) is described by $$x\left( t \right) = \left\{ {\matrix{ {1\,\,\,for\,\, - 1 \le t \le + 1} \cr {0\,\,\,\,\,\,\,\,\,\,\,\,\,\,otherwise} \cr } } \right.$$

Two of the angular frequencies at which its Fourier transform becomes zero are

For a signal x(t) the Fourier transform is X(f). Then the inverse Fourier transform of X(3f+2) is given by

Let x(t) and y(t) (with Fourier transforms X(f) and Y(f) respectively) be related as shown in Fig.(1) & (2).

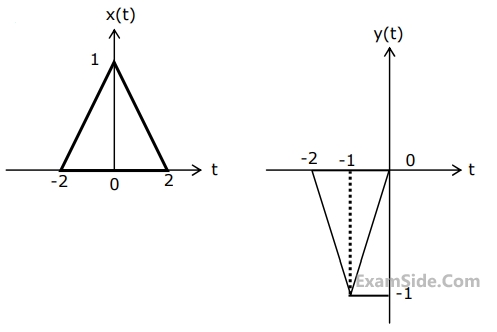
Then Y(f) is