The transfer function of the system $$Y\left( s \right)/U\left( s \right)$$ , whose state-space equations are given below is:
$$\eqalign{ & \left[ {\matrix{ {\mathop {{x_1}}\limits^ \bullet \left( t \right)} \cr {\mathop {{x_2}}\limits^ \bullet \left( t \right)} \cr } } \right] = \left[ {\matrix{ 1 & 2 \cr 2 & 0 \cr } } \right]\left[ {\matrix{ {{x_1}\left( t \right)} \cr {{x_2}\left( t \right)} \cr } } \right] + \left[ {\matrix{ 1 \cr 2 \cr } } \right]u\left( t \right) \cr & y\left( t \right) = \left[ {\matrix{ 1 & 0 \cr } } \right]\left[ {\matrix{ {{x_1}\left( t \right)} \cr {{x_2}\left( t \right)} \cr } } \right] \cr} $$

Consider the system described by the following state space representation
$$\eqalign{ & \left[ {\matrix{ {\mathop {{x_1}}\limits^ \bullet \left( t \right)} \cr {\mathop {{x_2}}\limits^ \bullet \left( t \right)} \cr } } \right] = \left[ {\matrix{ 0 & 1 \cr 0 & { - 2} \cr } } \right]\left[ {\matrix{ {{x_1}\left( t \right)} \cr {{x_2}\left( t \right)} \cr } } \right] + \left[ {\matrix{ 0 \cr 1 \cr } } \right]u\left( t \right) \cr & y\left( t \right) = \left[ {\matrix{ 1 & 0 \cr } } \right]\left[ {\matrix{ {{x_1}\left( t \right)} \cr {{x_2}\left( t \right)} \cr } } \right] \cr} $$

If $$u(t)$$ is a unit step input and $$\left[ {\matrix{ {{x_1}\left( 0 \right)} \cr {{x_2}\left( 0 \right)} \cr } } \right] = \left[ {\matrix{ 1 \cr 0 \cr } } \right],$$ the value of output $$y(t)$$ at $$t=1$$ sec (rounded off to three decimal places) is _____________.

Consider the following state - space representation of a linear time-invariant system.
$$\mathop x\limits^ \bullet \left( t \right) = \left[ {\matrix{ 1 & 0 \cr 0 & 2 \cr } } \right]\,\,x\left( t \right),\,\,y\left( t \right) = {c^T}x\left( t \right),\,c = \left[ {\matrix{ 1 \cr 1 \cr } } \right]$$ and
$$x\left( 0 \right) = \left[ {\matrix{ 1 \cr 1 \cr } } \right]$$

The value of $$y(t)$$ for $$t\,\,\, = \,\,{\log _e}2$$ ___________.

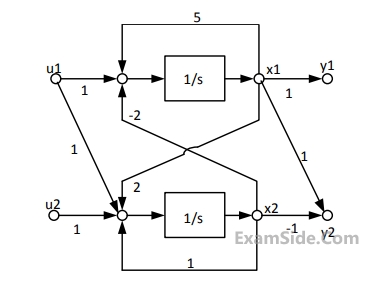
In the signal flow diagram given in the figure, $${u_1}$$ and $${u_2}$$ are possible inputs whereas $${y_1}$$ and $${y_2}$$ are possible outputs. When would the $$SISO$$ system derived from this diagram be controllable and observable?