Linear Time Invariant Systems · Signals and Systems · GATE EE
Marks 1
1
Suppose signal $y(t)$ is obtained by the time-reversal of signal $x(t)$, i.e., $y(t) = x(-t)$, $-\infty < t < \infty$. Which one of the following options is always true for the convolution of $x(t)$ and $y(t)$?
GATE EE 2024
2
Which of the following statement(s) is/are true?
GATE EE 2023
3
For the signals $$x(t)$$ and $$y(t)$$ shown in the figure, $$z(t)=x(t)*y(t)$$ is maximum at $$t=T_1$$. Then $$T_1$$ in seconds is __________ (Round off to the nearest integer)
GATE EE 2023
4
Consider a continuous-time system with input x(t) and output y(t) given by
$$y\left(t\right)=x\left(t\right)\cos\left(t\right)$$. This system is
GATE EE 2016 Set 1
5
The impulse response g(t) of a system G, is as shown in Figure (a). What is the maximum
value attained by the impulse response of two cascaded blocks of G as shown in Figure (b)?
GATE EE 2015 Set 1
6
Consider an LTI system with transfer function $$H\left(s\right)=\frac1{s\left(s+4\right)}$$.If the input to the system is cos(3t) and the steady state output is $$A\sin\left(3t+\alpha\right)$$, then the value
of A is
GATE EE 2014 Set 2
7
Consider an LTI system with impulse response $$h\left(t\right)=e^{-5t}u\left(t\right)$$ . If the output of the system is
$$y\left(t\right)=e^{-3t}u\left(t\right)-e^{-5t}u\left(t\right)$$ then the input, x(t), is given by
GATE EE 2014 Set 2
8
x(t) is nonzero only for $$T_x\;<\;t\;<\;T_x^1$$ , and similarly, y(t) is nonzero only for $$T_y\;<\;t\;<\;T_y^1$$ . Let
z(t) be convolution of x(t) and y(t). Which one of the following statements is TRUE?
GATE EE 2014 Set 1
9
The impulse response of a system is h(t) = tu(t). For an input u(t − 1), the output
is
GATE EE 2013
10
Two systems with impulse responses h1(t) and h2(t) are connected in cascade.
Then the overall impulse response of the cascaded system is given by
GATE EE 2013
11
Assuming zero initial condition, the response y(t) of the system given below to a
unit step input u(t) is
GATE EE 2013
12
A low–pass filter with a cut-off frequency of 30 Hz is cascaded with a high-pass
filter with a cut-off frequency of 20 Hz. The resultant system of filters will function
as
GATE EE 2011
13
Given two continuous time signals $$x\left(t\right)=e^{-t}$$ and $$y\left(t\right)=e^{-2t}$$ which exist for t > 0,
the convolution z(t) = x(t)*y(t) is
GATE EE 2011
14
The system represented by the input-output relationship $$y\left(t\right)=\int_{-\infty}^{5t}x\left(\tau\right)d\tau$$, t > 0 is
GATE EE 2010
15
For the system $$\frac2{\left(s+1\right)}$$,
the approximate time taken for a step response to reach 98%
of its final value is
GATE EE 2010
16
A linear Time Invariant system with an impulse response $$h(t)$$ produces output $$y(t)$$ when input $$x(t)$$ is applied. When the input $$x\left( {t - \tau } \right)$$ is applied to a system with response $$h\left( {t - \tau } \right)$$, the output will be
GATE EE 2009
17
The impulse response of a causal linear time-invariant system is given as $$h(t)$$. Now consider the following two statements:
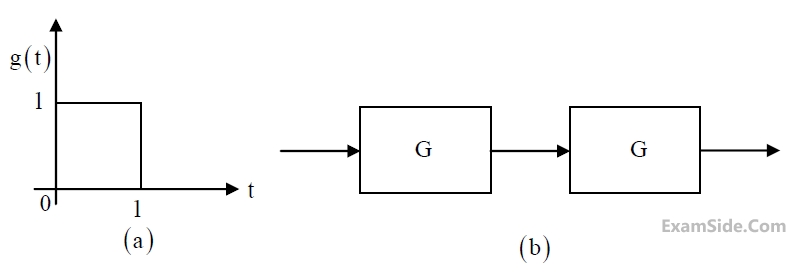
Statement-$$\left( {\rm I} \right)$$: Principle of superposition holds
Statement-$$\left( {\rm II} \right)$$: $$h\left( t \right) = 0$$ for $$t < 0$$
Which one of the following statements is correct?
GATE EE 2008
18
A signal $${e^{ - \alpha t}}\,\sin \left( {\omega t} \right)$$ is the input to a real Linear Time Invariant system. Given $$K$$ and $$\phi $$ are constants, the output of the system will be of the form $$K{e^{ - \beta t}}\,\sin \,\left( {\upsilon t + \phi } \right)$$ where
GATE EE 2008
19
A signal $${e^{ - \alpha t}}\,\sin \left( {\omega t} \right)$$ is the input to a real Linear Time Invariant system. Given $$K$$ and $$\phi $$ are constants, the output of the system will be of the form $$K{e^{ - \beta t}}\,\sin \,\left( {\upsilon t + \phi } \right)$$ where
GATE EE 2008
20
Let a signal $${a_1}\,\sin \left( {{\omega _1}t + {\phi _1}} \right)$$ be applied to a stable linear time-invariant system. Let the corresponding steady state output be represented as $${a_2}F\left( {{\omega _2}t + {\phi _2}} \right).$$ Then which of the following statements is true?
GATE EE 2007
21
$$s(t)$$ is step response and $$h(t)$$ is impulse response of a system. Its response $$y(t)$$ for any input $$u(t)$$ is given by
GATE EE 2002
22
If $$f(t)$$ is the step-response of a linear time-invariant system, then its impulse response is given by ___________
GATE EE 1994
23
$$s(t)$$ is step response and $$h(t)$$ is impulse response of a system. Its response $$y(t)$$ for any input $$u(t)$$ is given by
GATE EE 1993
Marks 2
1
The input $x(t)$ and the output $y(t)$ of a system are related as
$$ y(t) = e^{-t} \int\limits_{-\infty}^{t} e^{\tau} x(\tau) d\tau, \quad - \infty < t < \infty. $$
The system is
GATE EE 2024
2
Consider the discrete-time systems $T_1$ and $T_2$ defined as follows:
{ $T_1 x[ n ] = x[ 0 ] + x[ 1 ] + \cdots + x[ n ] $}
{ $T_2 x[ n ] = x[ 0 ] + \frac{1}{2} x[ 1 ] + \cdots + \frac{1}{2^n} x[ n ] $}
Which one of the following statements is true?
GATE EE 2024
3
Let a causal LTI system be governed by the following differential equation $$y(t) + {1 \over 4}{{dy} \over {dt}} = 2x(t)$$, where x(t) and y(t) are the input and output respectively. Its impulse response is
GATE EE 2022
4
Consider the system as shown below:
where y(t) = x(et). The system is
GATE EE 2022
5
For linear time invariant systems, that are Bounded Input Bounded stable, which one of
the following statement is TRUE?
GATE EE 2015 Set 2
6
The impulse response of a continuous time system is given by
h(t) = $$\delta$$(t − 1) + $$\delta$$(t − 3). The value of the step response at t = 2 is
GATE EE 2013
7
The input x(t) and output y(t) of a system are related as $$\int_{-\infty}^tx\left(\tau\right)\cos\left(3\tau\right)d\tau$$.The system is
GATE EE 2012
8
L et y[n] denote the convolution of h[n] and g[n], where $$h\left[n\right]=\left(1/2\right)^nu\left[n\right]$$ and g[n] is a causal
sequence. If y[0] = 1 and y[1] = 1/2, then g[1] equals
GATE EE 2012
9
The response h(t) of a linear time invariant system to an impulse $$\delta\left(t\right)$$, under
initially relaxed condition is $$h\left(t\right)=e^{-t}\;+\;e^{-2t}$$. The response of this system for a
unit step input u(t) is
GATE EE 2011
10
Given the finite length input x[n] and the corresponding finite length output y[n]
of an LTI system as shown below, the impulse response h[n] of the system is
GATE EE 2010
11
A cascade of 3 Linear Time Invariant systems is casual and unstable. From this, we conclude that
GATE EE 2009
12
The $$z$$$$-$$ transform of a signal $$x\left[ n \right]$$ is given by $$4{z^{ - 3}} + 3{z^{ - 1}} + 2 - 6{z^2} + 2{z^3}.$$ It is applied to a system, with a transfer function $$H\left( z \right) = 3{z^{ - 1}} - 2.$$ Let the output be $$y(n)$$. Which of the following is true?
GATE EE 2009
13
A system with input $$x(t)$$ and output $$y(t)$$ is defined by the input $$-$$ output relation:
$$y\left( t \right) = \int\limits_{ - \infty }^{ - 2t} {x\left( \tau \right)} d\tau .$$ The system will be
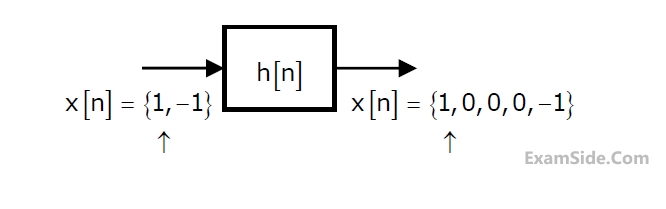
$$y\left( t \right) = \int\limits_{ - \infty }^{ - 2t} {x\left( \tau \right)} d\tau .$$ The system will be
GATE EE 2008
14
A signal $$x\left( t \right) = \sin c\left( {\alpha t} \right)$$ where $$\alpha $$ is a real constant $$\left( {\sin \,c\left( x \right) = {{\sin \left( {\pi x} \right)} \over {\pi x}}} \right)$$ is the input to a linear Time invariant system whose impulse response $$h\left( t \right) = \sin c\left( {\beta t} \right)$$ where $$\beta $$ is a real constant. If $$\min \left( {\alpha ,\,\,\beta } \right)$$ denotes the minimum of $$\alpha $$ and $$\beta $$, and similarly $$\max \left( {\alpha ,\,\,\beta } \right)$$ denotes the maximum of $$\alpha $$ and $$\beta $$, and $$K$$ is a constant, which one of the following statements is true about the output of the system?
GATE EE 2008
15
The transfer function of a linear time invariant system is given as $$G\left( s \right) = {1 \over {{s^2} + 3s + 2}}.$$ The steady state value of the output of this system for a unit impulse input applied at time instant $$t=1$$ will be
GATE EE 2008
16
$$X\left( z \right) = 1 - 3\,\,{z^{ - 1}},\,\,Y\left( z \right) = 1 + 2\,\,{z^{ - 2}}$$ are $$Z$$-transforms of two signals $$x\left[ n \right],\,\,y\left[ n \right]$$ respectively. A linear time invariant system has the impulse response $$h\left[ n \right]$$ defined by these two signals as $$h\left[ n \right] = x\left[ {n - 1} \right] * y\left[ n \right]$$ where $$ * $$ denotes discrete time convolution. Then the output of the system for the input $$\delta \left[ {n - 1} \right]$$
GATE EE 2007
17
A signal is processed by a causal filter with transfer function $$G(s).$$ For a distortion free output signal waveform, $$G(s)$$ must
GATE EE 2007
18
Consider the discrete-time system shown in the figure where the impulse response of $$G\left( z \right)$$ is
$$g\left( 0 \right) = 0,\,\,g\left( 1 \right) = g\left( 2 \right) = 1,\,g\left( 3 \right) = g\left( 4 \right) = .... = 0$$
$$g\left( 0 \right) = 0,\,\,g\left( 1 \right) = g\left( 2 \right) = 1,\,g\left( 3 \right) = g\left( 4 \right) = .... = 0$$
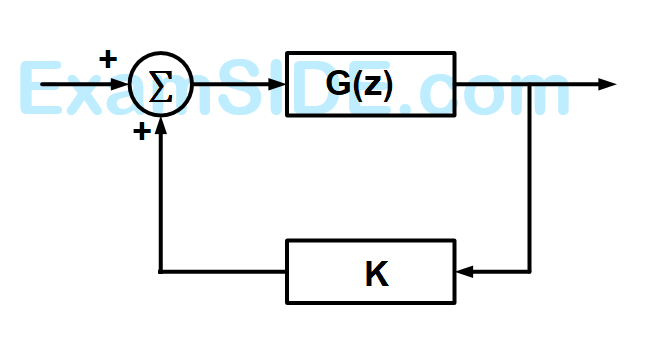
This system is stable for range of values of $$K$$
GATE EE 2007
19
A signal is processed by a causal filter with transfer function $$G(s).$$ For a distortion free output signal waveform, $$G(s)$$ must.
$$G\left( z \right) = a{z^{ - 1}} + \beta \,\,{z^{ - 3}}$$ is a low-pass digital filter with a phase characteristic same as that of the above question if
GATE EE 2007
20
$$x\left[ n \right] = 0;\,n < - 1,\,n > 0,\,x\left[ { - 1} \right] = - 1,\,x\left[ 0 \right]$$
$$\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2$$ is the input and
$$y\left[ n \right] = 0;\,n < - 1,\,n > 2,\,y\left[ { - 1} \right] = - 1,\, = y\left[ 1 \right],\,y\left[ 0 \right] = 3,\,y\left[ 2 \right]$$
$$\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, =- 2$$ is the output of a discrete-time $$LTI$$ system. The system impulse response $$h\left[ n \right]$$ will be
$$\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2$$ is the input and
$$y\left[ n \right] = 0;\,n < - 1,\,n > 2,\,y\left[ { - 1} \right] = - 1,\, = y\left[ 1 \right],\,y\left[ 0 \right] = 3,\,y\left[ 2 \right]$$
$$\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, =- 2$$ is the output of a discrete-time $$LTI$$ system. The system impulse response $$h\left[ n \right]$$ will be
GATE EE 2006
21
A continuous-time system is described by $$y\left( t \right) = {e^{ - |x\left( t \right)|}},$$ where $$y(t)$$ is the output and $$x(t)$$ is the input. $$y(t)$$ is bounded
GATE EE 2006
22
A discrete real all pass system has a pole at $$z = 2\angle {30^ \circ };\,$$ it, therefore,
GATE EE 2006
23
$$y\left[ n \right]$$ denotes the output and $$x\left[ n \right]$$ denotes the input of a discrete-time system given by the difference equation $$y\left[ n \right] - 0.8y\left[ {n - 1} \right] = x\left[ n \right] + 1.25\,x\left[ {n + 1} \right].$$ Its right-sided impulse response is
GATE EE 2006
24
In the system shown in Fig. the input $$x\left( t \right) = \sin t.$$ In the steady-state, the response $$y(t)$$ will be
GATE EE 2004
25
Given the relationship between the input $$u(t)$$ and the output $$y(t)$$ to be
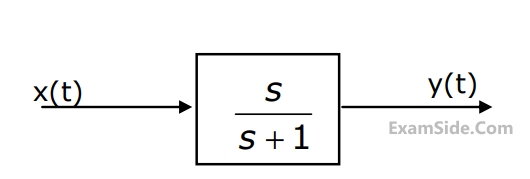
$$y\left( t \right) = \int\limits_0^t {\left( {2 + t - \tau } \right){e^{ - 3\left( {t - \tau } \right)}}} u\left( \tau \right)d\tau $$
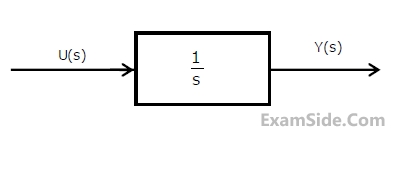
the transfer function $$Y\left( s \right)/U\left( s \right)$$ is
$$y\left( t \right) = \int\limits_0^t {\left( {2 + t - \tau } \right){e^{ - 3\left( {t - \tau } \right)}}} u\left( \tau \right)d\tau $$
the transfer function $$Y\left( s \right)/U\left( s \right)$$ is
GATE EE 2001
Marks 4
Marks 5
1
A single input single output system with $$y$$ as output and $$u$$ as input, is described by
$$${{{d^2}y} \over {d{t^2}}} + 2{{dy} \over {dt}} + 10y = 5{{d\,u} \over {dt}} - 3\,u$$$
For the above system find an input $$u(t),$$ with zero initial condition, that produces the same output as with no input and with the initial conditions.
$${{d\,y\left( {{0^ - }} \right)} \over {dt}} = - 4,\,\,\,y\left( {{0^ - }} \right) = 1$$
For the above system find an input $$u(t),$$ with zero initial condition, that produces the same output as with no input and with the initial conditions.
$${{d\,y\left( {{0^ - }} \right)} \over {dt}} = - 4,\,\,\,y\left( {{0^ - }} \right) = 1$$
GATE EE 2002
2
A first order system is initially at rest and excited by a step input at time $$t=0.$$ Its output becomes $$1.1$$ $$V$$ is in $$4$$ seconds and eventually reaches a steady state value of $$2V$$. Determine its time
GATE EE 1997
3
The impulse response of a network is $$h\left( t \right) = 1$$ for $$0 \le t < 1$$ and zero otherwise. Sketch the impulse response of two such networks in cascade, neglecting loading effects.
GATE EE 1992