Marks 1
1
Consider a unity-gain negative feedback system consisting of the plant G(s) (given below) and a proportional-integral controller. Let the proportional gain and integral gain be 3 and 1, respectively. For a unit step reference input, the final values of the controller output and the plant output, respectively, are
$$G(s) = {1 \over {s - 1}}$$
GATE EE 2023
2
The transfer function $$C(s)$$ of a compensator is given below:
$$C\left( s \right) = {{\left( {1 + {s \over {0.1}}} \right)\left( {1 + {s \over {100}}} \right)} \over {\left( {1 + s} \right)\left( {1 + {s \over {10}}} \right)}}$$
The frequency range in which the phase (lead) introduce by the compensator reaches the maximum is
GATE EE 2017 Set 2
3
The transfer function of a second order real system with a perfectly flat magnitude response of unity has a pole at $$\left( {2 - j3} \right).$$ List all the poles and zeros.
GATE EE 2015 Set 1
4
A lead compensator used for a closed loop controller has the following transfer function $${\textstyle{{K\left( {1 + {s \over a}} \right)} \over {\left( {1 + {s \over b}} \right)}}}\,\,\,$$ For such a lead compensator
GATE EE 2003
5
The phase lead compensation is used to
GATE EE 1998
6
A differentiator has transfer function whose
GATE EE 1994
7
The pole $$-$$ zero configuration of a phase lead compensator is given by
GATE EE 1994
Marks 2
1
A controller $D(s)$ of the form $\left(1+K_D s\right)$ is to be designed for the plant $G(s)=\frac{1000 \sqrt{2}}{s(s+10)^2}$ as shown in the figure. The value of $K_D$ that yields a phase margin of $45^{\circ}$ at the gain cross-over frequency of $10 \mathrm{rad} / \mathrm{sec}$ is _________(round off to one decimal place).
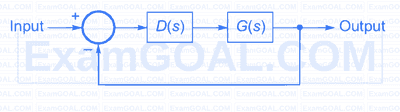

GATE EE 2025
2
The transfer function of a compensator is given as $${G_c}\left( s \right) = {{s + a} \over {s + b}}$$
$${G_c}\left( s \right)$$ is a lead compensator if
GATE EE 2012
3
The transfer function of a compensator is given as $${G_c}\left( s \right) = {{s + a} \over {s + b}}$$
The phase of the above lead compensator is maximum at
GATE EE 2012
4
The transfer function of two compensators are given below:
$${C_1} = {{10\left( {s + 1} \right)} \over {\left( {s + 10} \right)}},\,{C_2} = {{s + 10} \over {10\left( {s + 1} \right)}}$$
Which one of the following statements is correct?
GATE EE 2008
5
The system $$900/s(s+1)(s+9)$$ is to be such that its gain crossover frequency becomes same as its uncompensated phase crossover frequency and provides at $${45^0}$$ phase margin . To achieve this, one may use
GATE EE 2007
6
$$D\left( s \right) = {{\left( {0.5s + 1} \right)} \over {\left( {0.05s + 1} \right)}}$$ Maximum phase lead of the compensator is
GATE EE 2000