Which of the following statement(s) is/are correct?

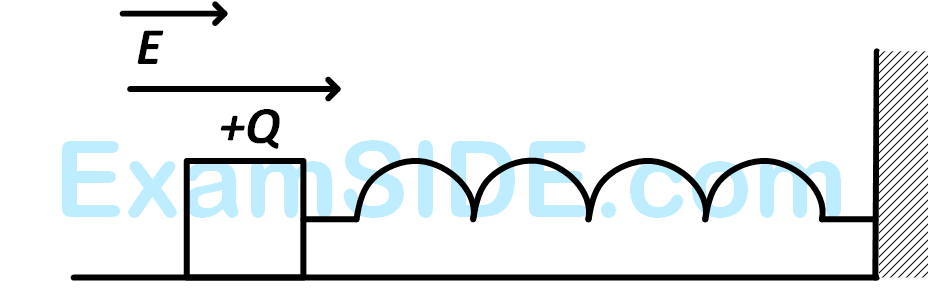
A wooden block performs $$SHM$$ on a frictionless surface with frequency, $${v_0}.$$ The block carries a charge $$+Q$$ on its surface . If now a uniform electric field $$\overrightarrow E $$ is switched- on as shown, then the $$SHM$$ of the block will be

Which of the field patterns given below is valid for electric field as well as for magnetic field ?

A point mass is subjected to two simultaneous sinusoidal displacements in x-direction, $${x_1}\left( t \right) = A\sin \omega t$$ and $${x_2}\left( t \right) = A\sin \left( {\omega t + {{2\pi } \over 3}} \right)$$. Adding a third sinusoidal displacement $${x_3}\left( t \right) = B\sin \left( {\omega t + \phi } \right)$$ brings the mass to a complete rest. The values of B and $$\phi $$ are