The vectors $\bar{a}, \bar{b}$ and $\bar{c}$ are such that $|\overline{\mathrm{a}}|=2,|\overline{\mathrm{~b}}|=4,|\overline{\mathrm{c}}|=4$. If the projection of $\overline{\mathrm{b}}$ on $\overline{\mathrm{a}}$ is equal to projection of $\overline{\mathrm{c}}$ on $\overline{\mathrm{a}}$ and $\overline{\mathrm{b}}$ is perpendicular to $\overline{\mathrm{c}}$, then the value of $|\overline{\mathrm{a}}+\overline{\mathrm{b}}-\overline{\mathrm{c}}|$ is
The values of $x$ for which the angle between the vectors $\overline{\mathrm{a}}=2 x^2 \hat{\mathrm{i}}+4 x \hat{\mathrm{j}}+\hat{\mathrm{k}}$ and $\overline{\mathrm{b}}=7 \hat{\mathrm{i}}-2 \hat{\mathrm{j}}+x \hat{\mathrm{k}}$ is obtuse, are
If $\overline{\mathrm{a}}, \overline{\mathrm{b}}, \overline{\mathrm{c}}$ are three coplanar vectors such that $|\overline{\mathrm{a}}|=1,|\overline{\mathrm{~b}}|=2, \overline{\mathrm{~b}} \cdot \overline{\mathrm{c}}=8$ and the angle between $\overline{\mathrm{b}}$ and $\overline{\mathrm{c}}$ is $45^{\circ}$ then the value of $|\overline{\mathrm{a}} \times(\overline{\mathrm{b}} \times \overline{\mathrm{c}})|$ is
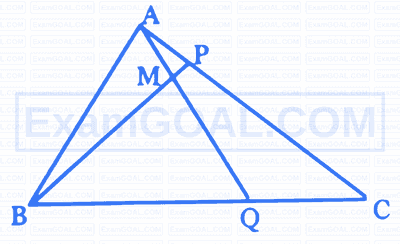
In the above figure, P divides AC in the ratio $3: 4$ and Q divides BC in the ratio $4: 3$. Then M divides AQ in the ratio