Linear Programming · Mathematics · MHT CET

A manufacturing company produces two items, A and B. Each toy should be processed by two machines, I and II. Machine I can be operated for maximum 10 hours 40 minutes. It takes 20 minutes for an item of A and 15 minutes for B. Machine II can be operated for a total time at 8 hours 20 minutes. It takes 5 minutes for an item A and 8 minutes for B . The profit per item of $A$ is $Rs 25$ and per item of $B$ is ₹ 18 . The formulation of an L.P.P. to maximize the profit (where $x$ is number of items A and $y$ is the number of item $B$ ) is

The solution for minimizing the function $\mathrm{z}=x+y$ under an L.P.P. with constraints $x+y \geq 2, x+2 y \leq 8, y \leq 3, x, y \geq 0$ is

In L.P.P., the maximum value of objective function $\mathrm{Z}=6 x+3 y$ subject to constraints $x+y \leq 5, x+2 y \geq 4,4 x+y \leq 12, x, y \geq 0$ is

The graph with correct feasible region of L.P.P. for the constraints $2 x+y \leqslant 10, y \leqslant x, y \leqslant 2, x, y \geqslant 0$ is …

The correct constraints for the given feasible region are ….

If the difference between the maximum and minimum values of the objective function $\mathrm{z}=7 x-8 y$, subject to the constraints $x+y \leqslant 20, y \geqslant 5, x, y \geqslant 0$ is $5 \mathrm{k}+200$, then the value of k is

The solution set for minimizing the function $\mathrm{z}=x+y$ with constraints $x+y \geqslant 2, x+2 y \leqslant 8, y \leqslant 3, x, y \geqslant 0$ contains

The L.P.P. , minimize $z=30 x+20 y, x+y \leq 8$, $x+2 y \geq 4,6 x+4 y \geq 12, x \geqslant 0, y \geqslant 0$ has

A scholarship amount is given by $\mathrm{z}=550 x+300 y$ and is to be distributed among $x$ boys and $y$ girls. From the graph given below the maximum amount of scholarship is __________

The shaded region in the following figure represents a solution set of

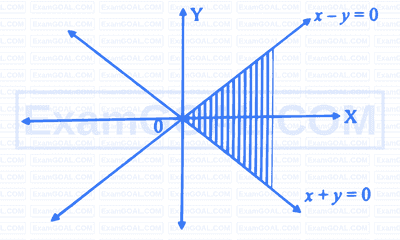
The feasible region for the constraints $x-2 \leqslant y, x \geqslant y-1, x \geqslant 2, y \leqslant 4, x, y \geqslant 0$, is _________

The feasible region represented by the given constraints $2 x+3 y \geq 12,-x+y \leq 3, x \leq 4, y \geq 3$ is denoted by

The shaded area in the given figure is a solution set for some system of inequalities. The maximum value of the function $\mathrm{z}=4 x+3 y$ subject to linear constraints given by the system is

Maximum value of $Z=100 x+70 y$ Subject to $2 x \geq 4, y \leq 3, x+y \leq 8, x, y \geq 0$ is

The graphical solution set of the system of inequations $2 x+3 y \leq 6, x+4 y \geq 4, x \geq 0, y \geq 0$ is given by

The region represented by the inequations $2 x+3 y \leqslant 18, x+y \geqslant 10, x \geqslant 0, y \geqslant 0$ is

A production unit makes special type of metal chips by combining copper and brass. The standard weight of the chip must be at least 5 gms. The basic ingredients i.e. copper and brass cost ₹8 and ₹ 5 per gm. The durability considerations dictate that the metal chip must no contain more than 4 gms of brass and should contain minimum 2 gms of copper. Then the minimum cost of the metal chip satisfying the above conditions is

For the following shaded region, the linear constraints are

The graphical solution set of the system of inequations $x+y \geq 1,7 x+9 y \leq 63, y \leq 5, x \leq 6$, $x \geq 0, y \geq 0$ is represented by

The function to be maximized is given by $Z=3 x+2 y$. The feasible region for this function is the shaded region given below, then the linear constraints for this region are given by

The maximum value of $z=4 x+2 y$, subject to the constraints $3 x+4 y \geqslant 12, x+y \leqslant 5, x, y \geqslant 0$ is

The maximum value of $z=x+y$, subjected to $x+y \leq 10,5 x+3 y \geq 15, x \leq 6, x, y \geq 0$

The maximum value of the objective function $\mathrm{z}=4 x+6 y$ subject to $3 x+2 y \leq 12, x+y \geq 4, x$, $y \geq 0$ is

The shaded region in the following figure is the solution set of the inequations

The maximum value of $\mathrm{Z}=x+y$, subjected to $x+y \leq 10,5 x+3 y \geq 15, x \leq 6, x, y \geq 0$

The shaded area in the figure below is the solution set for a certain linear programming problem, then the linear constraints are given by

The shaded region in the following figure is the solution set of the inequations

The point, at which the maximum value of $10 x+6 y$ subject to the constraints $x+y \leq 12$, $2 x+y \leq 20, x \geq 0, y \geq 0$ occurs, is

The shaded region in the following figure represents the solution set for a certain linear programming problem. Then linear constraints for this region are given by

The solution set of the inequalities $$4 x+3 y \leq 60, y \geq 2 x, x \geq 3, x, y \geq 0$$ is represented by region

The shaded area in the given figure is a solution set for some system of inequations. The maximum value of the function $$z=10 x+25 y$$ subject to the linear constraints given by the system is

If feasible region is as shown in the figure, then related inequalities are

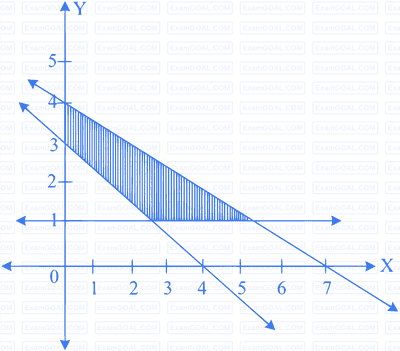
The maximum value of $$z=7 x+8 y$$ subject to the constraints $$x+y \leq 20, y \geq 5, x \leq 10, x \geq 0, y \geq 0$$ is

For a feasible region OCDBO given below, the maximum value of the objective function $$z=3 x+4 y$$ is

The maximum value of $$z=3 x+5 y$$ subject to the constraints $$3 x+2 y \leq 18, x \leq 4, y \leq 6, x, y \geq 0$$, is

For the following shaded area, the linear constraints except $$x,y \ge 0$$ are

The shaded area in the figure given below is a solution set of a system of inequations. The minimum value of objective function $$3 x+5 y$$, subject to the linear constraints given by this system of inequations is

The vertices of the feasible region for the constraints $$x+y \leq 4, x \leq 2, y \leq 1, x+y \geq 1, x, y \geq 0$$ are

The graphical solution set for the system of inequations $$x-2 y \leq 2,5 x+2 y \geq 10,4 x+5 y \leq 20, x \geq 0, y \geq 0$$ is given by

If feasible region is as shown in the figure, then the related inequalities are

Maximum value of $$Z=5 x+2 y$$, subject to $$2 x-y \geq 2, x+2 y \leq 8$$ and $$x, y \geq 0$$ is

The region represented by the inequalities $$x \geq 6, y \geq 3,2 x+y \geq 10, x \geq 0, y \geq 0$$ is

The common region of the solutions of the inequations $$x+2 y \geq 4,2 x-y \leq 6$$ and $$x, y>0$$ is

The minimum value of the objective function $$z=4 x+6 y$$ subject to $$x+2 y \geq 80,3 x+y \geq 75, x, y \geq 0$$ is

The maximum value of the objective function $$z=2 x+3 y$$ subject to the constraints $$x+y \leq 5,2 x+y \geq 4$$ and $$x \geq 0, y \geq 0$$ is

The common region of the solution of the inequations $$x+y \geq 5, y \leq 4, x \geq 2, x, y \geq 0$$ is

The maximum value of $$z=10 x+25 y$$ subject to $$0 \leq x \leq 3,0 \leq y \leq 3, x+y \leq 5$$ occurs at the point.

The objective function $$z=4 x+5 y$$ subjective to $$2 x+y \geq 7 ; 2 x+3 y \leq 15 ; y \leq 3, x \geq 0 ; y \geq 0$$ has minimum value at the point.

The shaded figure given below is the solution set for the linear inequations. Choose the correct option.

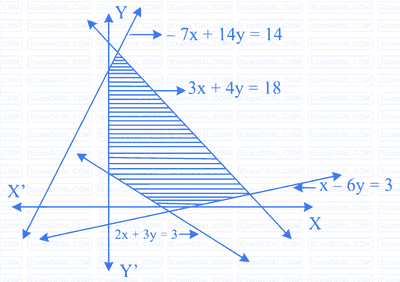
The solution set for the system of linear inequations $$x+y \geq 1 ; 7 x+9 y \leq 63 ; y \leq 5 ; x \leq 6, x \geq 0$$ and $$y \geq 0$$ is represented graphically in the figure. What is the correct option?

The shaded part of the given figure indicates the feasible region. Then the constraints are

The LPP to maximize $Z=x+y$, subject to $x+y \leq 1,2 x+2 y \geq 6, x \geq 0, y \geq 0$ has

The maximum value of $$Z=3 x+5 y$$, subject to $$3 x+2 y \leq 18, x \leq 4, y \leq 6, x, y \geq 0$$ is

The minimum value of $$Z=5 x+8 y$$ subject to $$x+y \geq 5,0 \leq x \leq 4, y \geq 2, x \geq 0, y \geq 0$$ is

If $z=a x+b y ; a, b>0$ subject to $x \leq 2, y \leq 2, x+y \geq 3, x \geq 0, y \geq 0$ has minimum value at $(2,1)$ only, then......

The maximum value of $Z=5 x+4 y$, Subject to $y \leq 2 x, x \leq 2 y, x+y \leq 3, x \geq 0, y \geq 0$ is ........

The maximum value of $z=6 x+8 y$ subject to $x-y \geq 0, x+3 y \leq 12, x \geq 0, y \geq 0$ is $\ldots \ldots$.

For L.P.P, maximize $z=4 x_1+2 x_2$ subject to $3 x_1+2 x_2 \geq 9, x_1-x_2 \leq 3, x_1 \geq 0, x_2 \geq 0$ has

The maximum value of $z=9 x+11 y$ subject to $3 x+2 y \leq 12,2 x+3 y \leq 12, x \geq 0, y \geq 0$ is $\ldots \ldots$.

The minimum value of $z=10 x+25 y$ subject to $0 \leq x \leq 3,0 \leq y \leq 3, x+y \geq 5$ is $\ldots$