1
GATE ME 2010
MCQ (Single Correct Answer)
+2
-0.6
For the epicyclic gear arrangement shown in the figure, $${{\omega _2} = 100}$$ rad/s clockwise (CW) and $${{\omega _{arm}} = 80}$$ rad/s counter clockwise (CCW). The angular velocity $${{\omega _5}}$$ (in rad/s) is
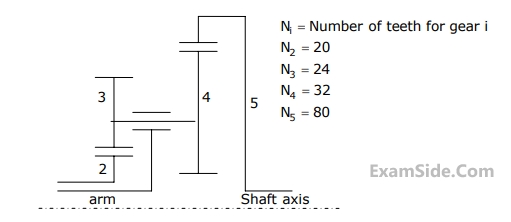

2
GATE ME 2009
MCQ (Single Correct Answer)
+2
-0.6
An epicyclic gear train is shown schematically in the adjacent figure The sun gear 2 on the input shaft is a 20 teeth external gear. The planet gear 3 is a 40 teeth external gear. The ring gear 5 is a 100 teeth internal gear. The ring gear 5 is fixed and the gear 2 is rotating at 60 rpm CCW (CCW $$=$$ counter-clockwise and CW $$=$$ clockwise)
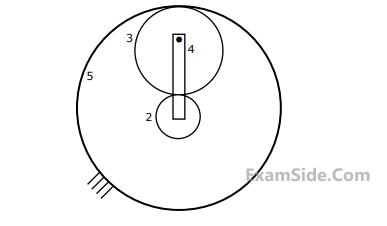
The arm 4 attached to the output shaft will rotate at

3
GATE ME 2006
MCQ (Single Correct Answer)
+2
-0.6
A planetary gear train has four gears and one carrier. Angular velocities of the gears are $${\omega _1},{\omega _2},{\omega _3},$$ and $${\omega _4}$$ respectively. The carrier rotates with angular velocity $${\omega _5}.$$
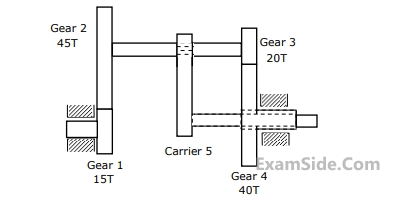

For $${{\omega _1} = 60}$$ rpm clockwise $$(CW)$$ when looked from the left, what is the angular velocity of the carrier and its direction so that Gear $$4$$ rotates in counter clockwise $$(CCW)$$ direction at twice the angular velocity of Gear $$1$$ when looked from the left?
4
GATE ME 2006
MCQ (Single Correct Answer)
+2
-0.6
A planetary gear train has four gears and one carrier. Angular velocities of the gears are $${\omega _1},{\omega _2},{\omega _3},$$ and $${\omega _4}$$ respectively. The carrier rotates with angular velocity $${\omega _5}.$$


What is the relation between the angular velocities of Gear $$1$$ and Gear $$4$$?
Questions Asked from Gears and Gear Trains (Marks 2)
Number in Brackets after Paper Indicates No. of Questions
GATE ME Subjects
Engineering Mechanics
Strength of Materials
Theory of Machines
Engineering Mathematics
Machine Design
Fluid Mechanics
Turbo Machinery
Heat Transfer
Thermodynamics
Production Engineering
Industrial Engineering
General Aptitude