Pert and Cpm · Industrial Engineering · GATE ME
Marks 1
Marks 2
A project involves eight activities with the precedence relationship and duration as shown in the table below. The slack for the activity D is __________hours (answer in integer).
$$ \begin{array}{|c|c|c|} \hline \text { Activity } & \text { Immediate predecessor } & \text { Duration(hours) } \\ \hline A & - & 4 \\ \hline B & A & 8 \\ \hline C & A & 5 \\ \hline D & B & 2 \\ \hline E & B & 7 \\ \hline F & C & 6 \\ \hline G & D & 3 \\ \hline H & E, F, G & 9 \\ \hline \end{array} $$A project consists of five activities (A, B, C, D and E). The duration of each activity follows beta distribution. The three time estimates (in weeks) of each activity and immediate predecessor(s) are listed in the table. The expected time of the project completion is ______ weeks (in integer).
|
Activity |
Time estimates (in weeks) |
Immediate predecessor(s) |
||
|
Optimistic time |
Most likely time |
Pessimistic time |
||
|
A |
4 |
5 |
6 |
None |
|
B |
1 |
3 |
5 |
A |
|
C |
1 |
2 |
3 |
A |
|
D |
2 |
4 |
6 |
C |
|
E |
3 |
4 |
5 |
B, D |
Activities A to K are required to complete a project. The time estimates and the immediate predecessors of these activities are given in the table. If the project is to be completed in the minimum possible time, the latest finish time for the activity G is ______ hours.
$$ \begin{array}{|c|c|c|} \hline \text { Activity } & \text { Time (hours) } & \text { Immediate predecessors } \\ \hline \text { A } & 2 & - \\ \hline \text { B } & 3 & - \\ \hline \text { C } & 2 & - \\ \hline \text { D } & 4 & \text { A } \\ \hline \text { E } & 5 & \text { B } \\ \hline \text { F } & 4 & \text { B } \\ \hline \text { G } & 3 & \text { C } \\ \hline \text { H } & 10 & \text { D, E } \\ \hline \text { I } & 5 & \text { F } \\ \hline \text { J } & 8 & \text { G } \\ \hline \text { K } & 3 & \text { H, I, J } \\ \hline \end{array} $$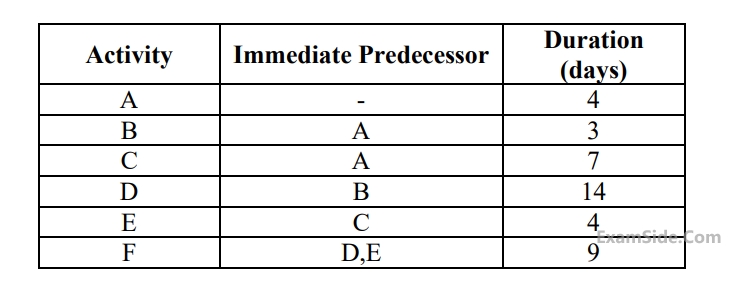
The minimum project completion time (in days) is ___________
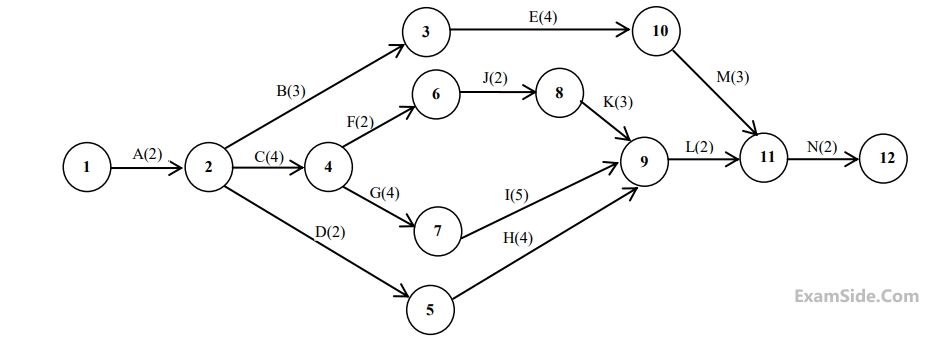
The minimum time (in days) for completion of the project is _____________
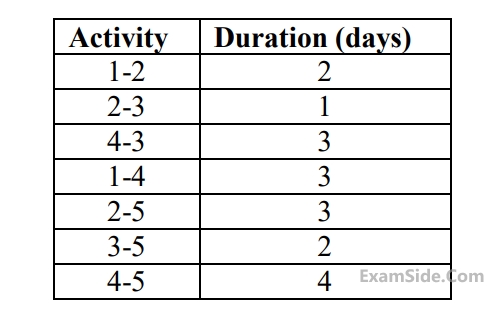
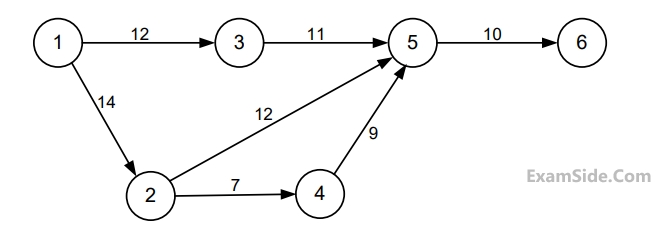
The critical path $$(CP)$$ in the network is
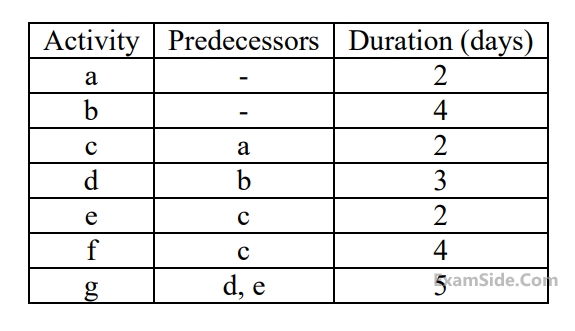
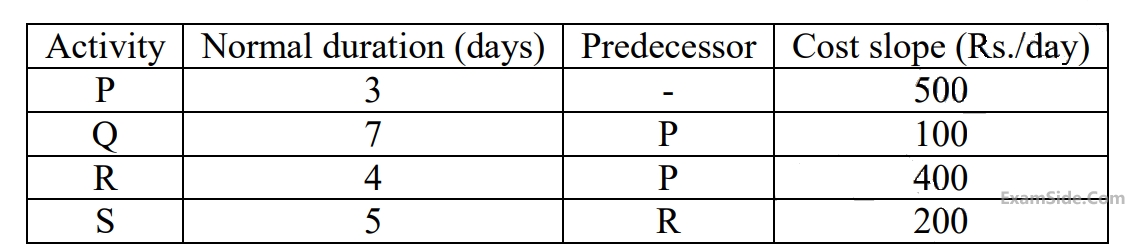
The normal cost of the project is Rs. $$10,000/-$$ and the overhead cost is Rs. $$200/-$$ per day. If the project duration has to be crashed down to $$9$$ days, the total cost (in Rupees) of the project is ______________.
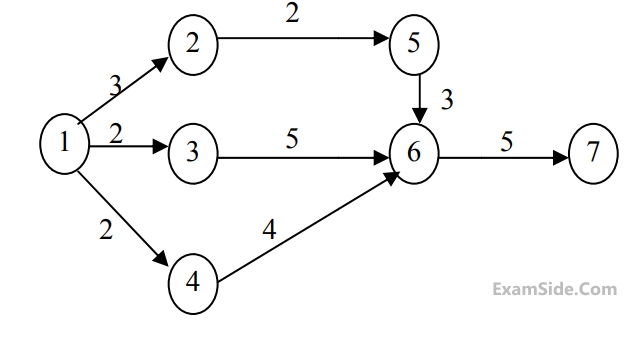
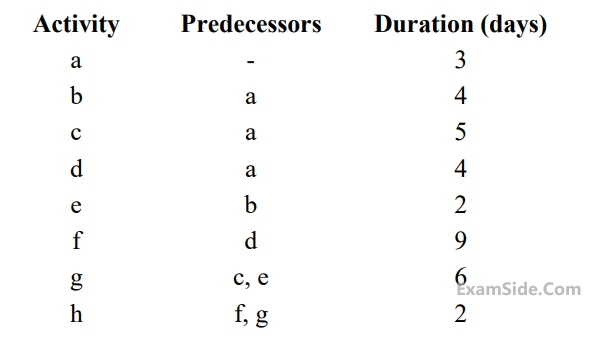
If the duration of activity $$''f ''$$ alone is changed from $$9$$ to $$10$$ days, then the

The critical path for the project is
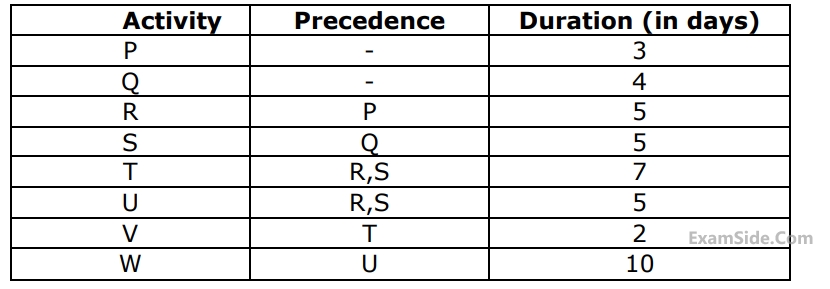

The optimistic time, most likely time and pessimistic time of all the activities are given in the table below :
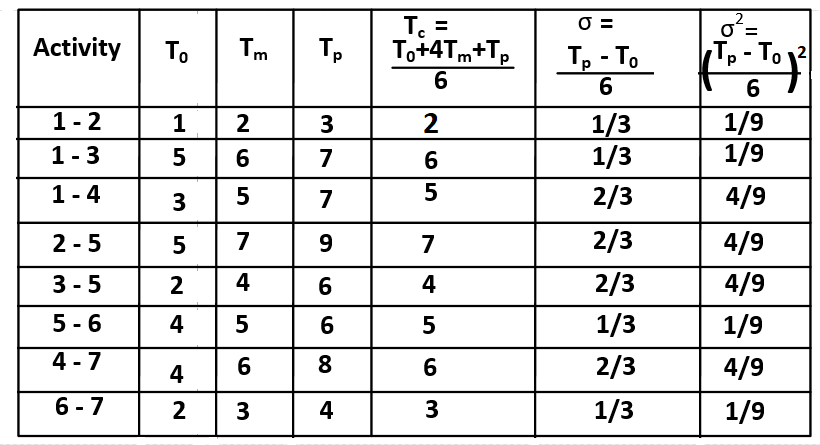
The standard deviation of the critical path is

The optimistic time, most likely time and pessimistic time of all the activities are given in the table below :

The critical path duration of the network (in days) is
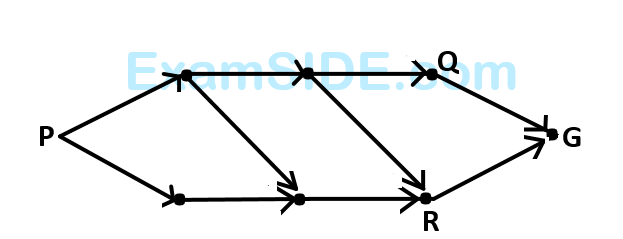
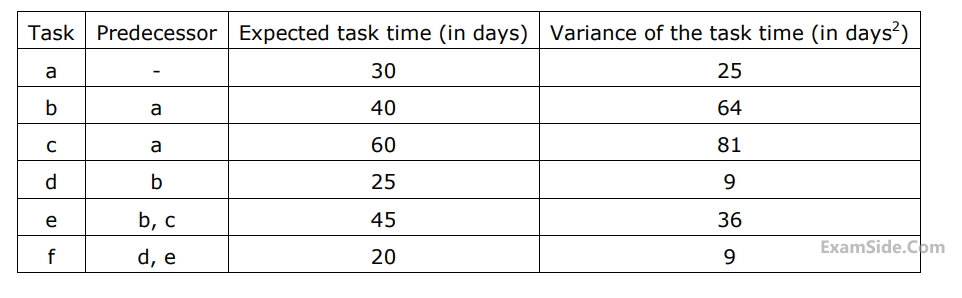
The expected completion time of the project is

The standard deviation of the critical path of the project is
The project can be completed
Marks 5
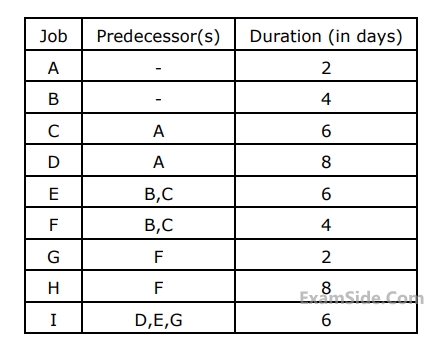
(a) Draw the activity-on-arc project network
(b) Determine all critical path(s) and their duration(s).
(c) What is the total float for jobs $$B$$ and $$D?$$
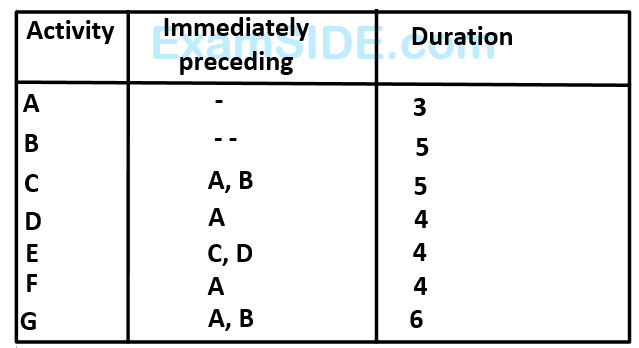
(a) Draw the network for the above project capturing the precedence relationships.
(b) Find the critical path and its duration.
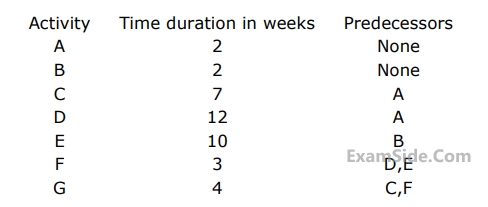
(a) Construct a $$PERT$$ network
(b) Find the critical path and estimate the project duration.
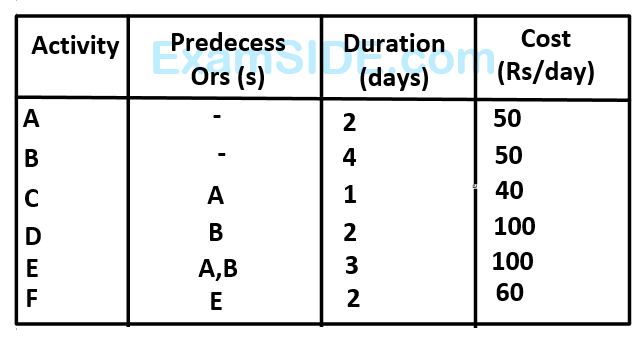
(a) What is the minimum duration of the project?
(b) Draw a Gantt chart for the early schedule
(c) Determine the peak requirement of money and the day on which it occurs in above schedule.
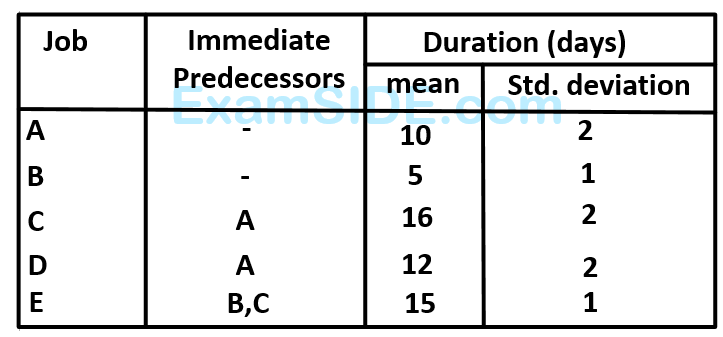
(i) Draw the project network in activity on arc mode
(ii) Under $$PERT$$ assumptions, determine the distribution of project duration.