Conduction · Heat Transfer · GATE ME

A plane, solid slab of thickness L, shown in the figure, has thermal conductivity k that varies with the spatial coordinate x as k = A + Bx, where A and B are positive constants (A > 0, B > 0). The slab walls are maintained at fixed temperatures of T(x = 0) = 0 and T(x = L) = T₀ > 0. The slab has no internal heat sources. Considering one-dimensional heat transfer, which one of the following plots qualitatively depicts the steady-state temperature distribution within the slab?

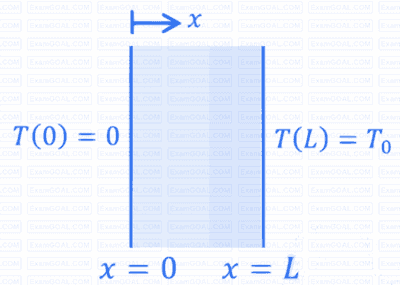
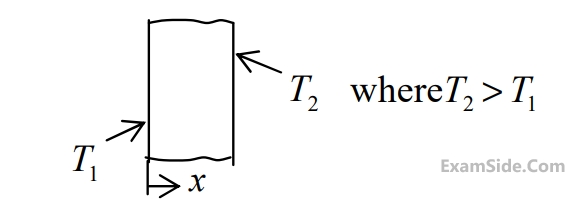
As $$x$$ increases, the state temperature gradient $$(dT/dx)$$ will

Consider a slab of 20 mm thickness. There is a uniform heat generation of $ \dot{q} = 100 \text{ MW/m}^3 $ inside the slab. The left and right faces of the slab are maintained at 150 °C and 110 °C, respectively. The plate has a constant thermal conductivity of 200 W/(m.K). Considering a 1-D steady state heat conduction, the location of the maximum temperature from the left face will be at ______mm (answer in integer).

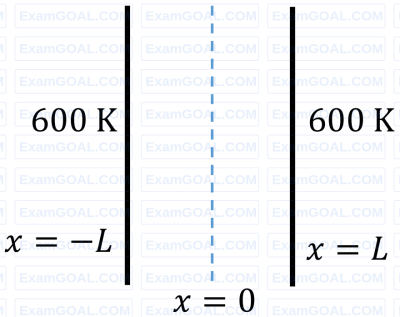
Consider steady state, one-dimensional heat conduction in an infinite slab of thickness 2L (L = 1 m) as shown in the figure. The conductivity (k) of the material varies with temperature as k = CT, where T is the temperature in K, and C is a constant equal to 2 W.m^-1K^-2. There is a uniform heat generation of 1280 kW/m³ in the slab. If both faces of the slab are maintained at 600 K, then the temperature at x = 0 is _______ K (in integer).

Consider a rod of uniform thermal conductivity whose one end (x = 0) is insulated and the other end (x = L) is exposed to flow of air at temperature T_∞ with convective heat transfer coefficient h. The cylindrical surface of the rod is insulated so that the heat transfer is strictly along the axis of the rod. The rate of internal heat generation per unit volume inside the rod is given as 

$\rm \dot q = \cos \frac{2 \pi x}{L}$

The steady-state temperature at the mid-location of the rod is given as T_A. What will be the temperature at the same location, if the convective heat transfer coefficient increases to 2h?

Consider a solid slab (thermal conductivity, k = 10 W∙m^-1∙K^-1) with thickness 0.2 m and of infinite extent in the other two directions as shown in the figure. Surface 2, at 300 K, is exposed to a fluid flow at a free stream temperature (T_∞) of 293 K, with a convective heat transfer coefficient (h) of 100 W∙m^-2∙K^-1. Surface 2 is opaque, diffuse and gray with an emissivity (ε) of 0.5 and exchanges heat by radiation with very large surroundings at 0 K. Radiative heat transfer inside the solid slab is neglected. The Stefan-Boltzmann constant is 5.67 × 10^-8 W∙m^-2∙K^-4. The temperature T₁ of Surface 1 of the slab, under steady-state conditions, is _________ K (round off to the nearest integer).

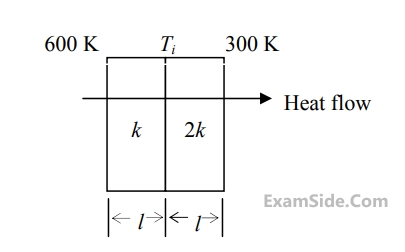
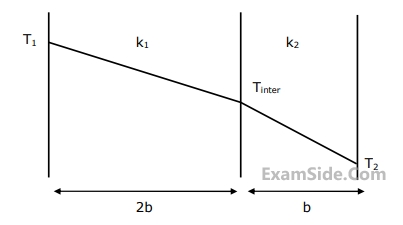
The interface temperature $${T_i}$$ (in $$K$$) of the composite wall is ___________

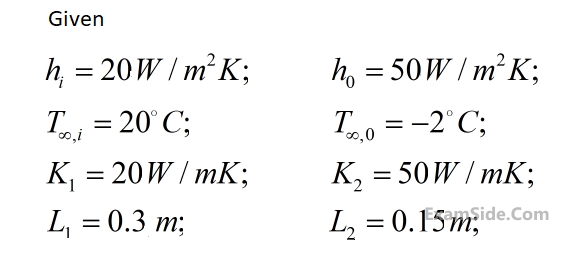
Assuming negligible contact resistance between the wall surfaces, the interface temp $$T(C)$$ of the two walls will be

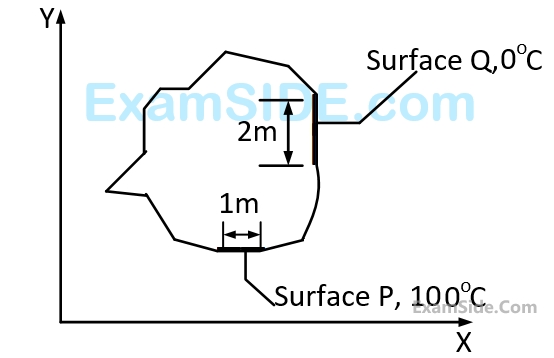
The location of maximum temp within the plate from left face is

The minimum temp within the plate in degree $$C$$ is

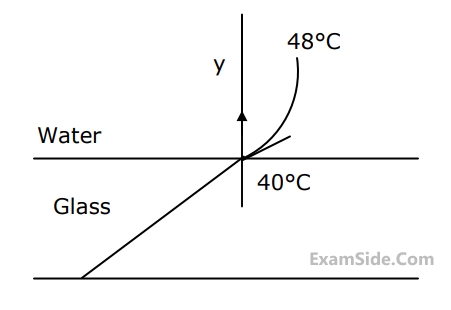
The heat transfer coefficient $$h$$ in $$W/{m^2}K$$ is

The value of the temperature gradient in the glass at the water-glass interface in $$K/m$$ is

Property
$$A.$$ Bulk modulus
$$B.$$ Thermal conductivity
$$C.$$ Heat transfer coefficient
$$D.$$ Heart flow rate

Units
$$1.$$ $$W/s$$
$$2.$$ $$N/{m^2}$$
$$3.$$ $$N/{m^3}$$
$$4.$$ $$W$$
$$5.$$ $$W/mK$$
$$6.$$ $$W/{m^2}K$$

Thermal conductivity of the materials are $$$\eqalign{ & {K_A} = {K_D} = 50\,\,\,W/mK, \cr & {K_B} = 10\,\,W/mK,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,{K_C} = 1\,\,W/mK \cr} $$$
The fluid temps and $$HTC$$(see fig) are $$$\eqalign{ & {T_1} = {200^ \circ }C,\,\,\,\,{h_1} = 50\,\,W/{m^2}K, \cr & T{}_2 = {25^ \circ }C,\,\,\,\,{h_2} = 10\,\,W/{m^2}K, \cr} $$$

Assuming one dimensional heat transfer condition. Determine the rate of heat transfer through the wall.

Inner radius of steel pipe $$=50mm,$$
Outer radius of the steel pipe $$=57mm,$$
Outer radius of insulation $$=157 mm,$$
Thermal conductivity of steel $$=43$$ $$W/mK$$
Thermal conductivity of insulating material $$=0.1$$ $$W/mK$$
Heat transfer coefficient on steam side $$ = 570W/{m^2}K$$
Heat transfer coefficient on air side $$ = 12W/{m^2}K,$$
Temperature of steam $$ = {500^ \circ }C$$
Temperature of surroundings $$ = {30^ \circ }C$$

Calculate the heat loss per meter length of pipe and temp of the outer surface of the insulation.