In the pipe network shown in the figure, all pipes have the same cross-section and can be assumed to have the same friction factor. The pipes connecting points W, N, and S with point J have an equal length L. The pipe connecting points J and E has a length 10L. The pressures at the ends N, E, and S are equal. The flow rate in the pipe connecting W and J is Q. Assume that the fluid flow is steady, incompressible, and the pressure losses at the pipe entrance and junction are negligible. Consider the following statements:

I : The flow rate in pipe connecting J and E is Q/21.

II: The pressure difference between J and N is equal to the pressure difference between J and E.

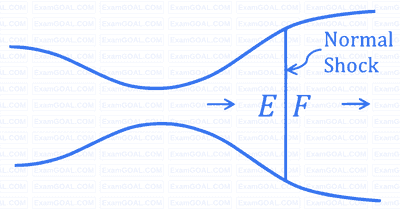
Steady, compressible flow of air takes place through an adiabatic converging-diverging nozzle, as shown in the figure. For a particular value of pressure difference across the nozzle, a stationary normal shock wave forms in the diverging section of the nozzle. If $E$ and $F$ denote the flow conditions just upstream and downstream of the normal shock, respectively, which of the following statement(s) is/are TRUE?

The velocity field of a certain two-dimensional flow is given by

V(𝑥, 𝑦) = 𝑘(𝑥𝑖̂ − 𝑦𝑗̂)

where 𝑘 = 2 s^-1. The coordinates 𝑥 and 𝑦 are in meters. Assume gravitational effects to be negligible.

If the density of the fluid is 1000 kg/m³ and the pressure at the origin is 100 kPa, the pressure at the location (2 m, 2 m) is _____________ kPa.

(Answer in integer)

Consider a unidirectional fluid flow with the velocity field given by

V(𝑥, 𝑦, 𝑧, 𝑡) = 𝑢(𝑥, 𝑡) 𝑖̂

where 𝑢(0,𝑡) = 1. If the spatially homogeneous density field varies with time 𝑡 as

𝜌(𝑡) = 1 + 0.2𝑒^−𝑡

the value of 𝑢(2, 1) is ______________. (Rounded off to two decimal places) Assume all quantities to be dimensionless.